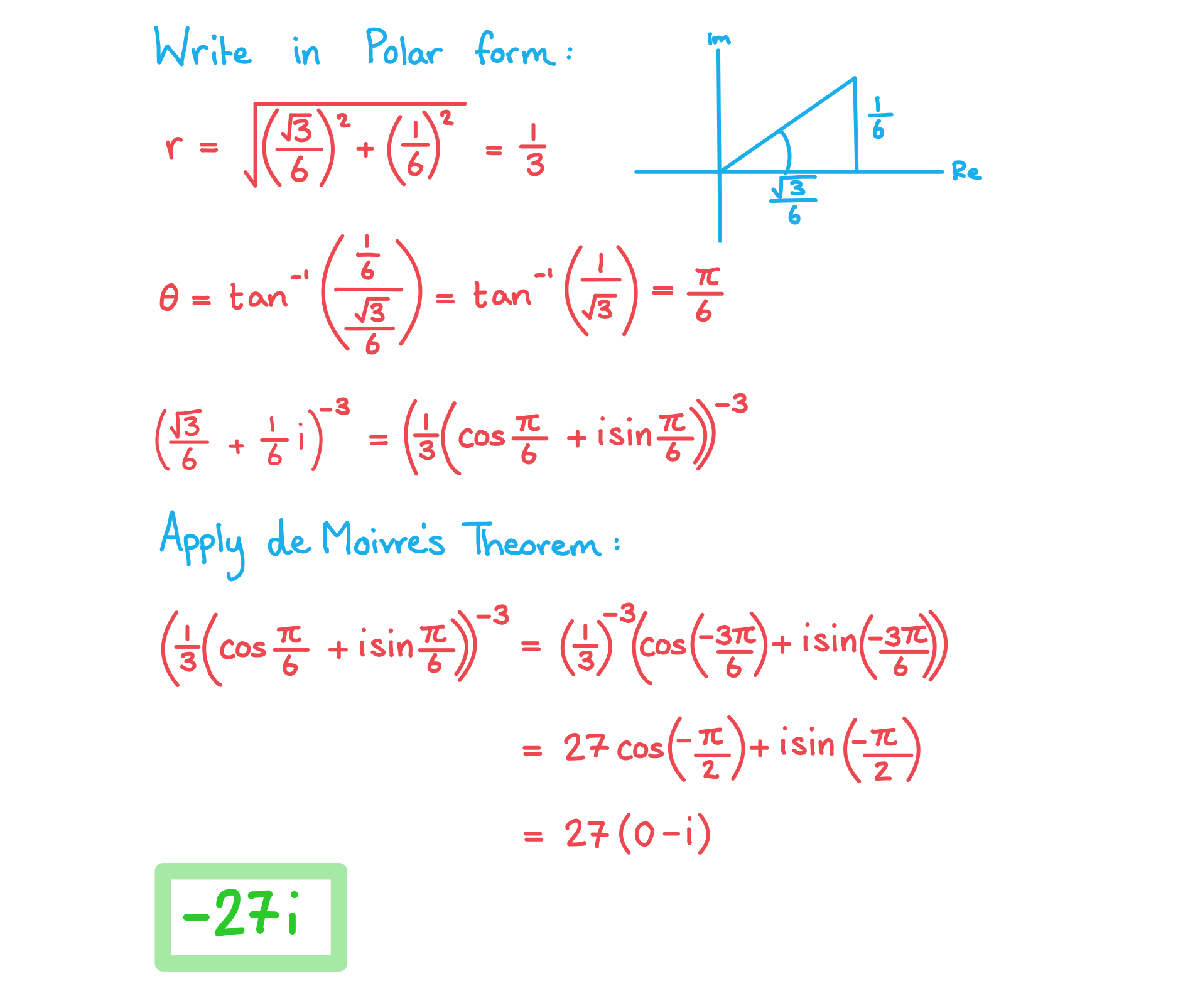1.2.2 de Moivre's Theorem | Edexcel A Level Further Maths: Core Pure Revision Notes 2017

De Moivre's Theorem

de Moivre’s theorem can be used to find powers of complex numbers
It states that for $z^{n} = [r (\cos θ + isin θ)]^{n} = r^{n} (\cos n θ + isin n θ)$
- Where
  - z ≠ 0
  - r is the modulus, |z|, r ∈ ℝ⁺
  - θ is the argument, arg z, θ ∈ ℝ
  - n ∈ ℝ
In Euler’s form this is simply:
- ${{(r e^{i θ})}^{n} = r}^{n} e^{i n θ}$
In words de Moivre’s theorem tells us to raise the modulus by the power of n and multiply the argument by n
In the formula booklet de Moivre’s theorem is given in both polar and Euler’s form:
- $[r (\cos θ + isin θ)]^{n} = r^{n} (\cos n θ + isin n θ) = r^{n} e^{i n θ}$

If a complex number is in Cartesian form you will need to convert it to either modulus-argument (polar) form or exponential (Euler’s) form first
- This allows de Moivre’s theorem to be used on the complex number
You may need to convert it back to Cartesian form afterwards
If a complex number is in the form $z = r (\cos (θ) - isin (θ))$ then you will need to rewrite it as $z = r (\cos (- θ) + isin (- θ))$ before applying de Moivre’s theorem
A useful case of de Moivre’s theorem allows us to easily find the reciprocal of a complex number:
- ^{$\frac{1}{z} = \frac{1}{r} (\cos (- θ) + isin (- θ) = \frac{1}{r} e^{- i θ}$}
- Using the trig identities cos(-θ) = cos(θ) and sin(-θ) = - sin(θ) gives
- $\frac{1}{z} = z^{- 1} = r^{- 1} [\cos (θ) - isin (θ)] = \frac{1}{r} [\cos (θ) - isin (θ)]$
In general
- $z^{- n} = r^{- n} [\cos (- n θ) + isin (- n θ)] = r^{- n} [\cos (n θ) - isin (n θ)]$

You may be asked to find all the powers of a complex number, this means there will be a repeating pattern
- This can happen if the modulus of the complex number is 1
- Keep an eye on your answers and look for the point at which they begin to repeat themselves

Find the value of ${(\frac{\sqrt{3}}{6} + \frac{1}{6} i)}^{- 3}$ , giving your answer in the form a + bi.

al-fm-1-2-2-de-moivres-theorem-we-solu