Multiple Angle Formulae
de Moivre’s theorem can be applied to prove identities such as . This allows expressions involving multiple angles to be written as a polynomial function of a single trig function which makes it easier to solve equations involving different angles.
How do I write sinkθ or coskθ in terms of powers of sinθ or cosθ?
- STEP 1
Use de Moivre’s theorem to write - STEP 2
Use the binomial expansion to expand - STEP 3
Useto simplify the expansion and group the real terms and the imaginary terms separately
- STEP 4
Equate the real parts of the expansion to cos kθ and equate the imaginary parts to sinkθ - STEP 5 (Depending on the question)
Useto write the identity in terms of sinθ only or cosθ depending on what the question asks
- coskθ can always be written as a function of just cosθ
- sinkθ can be written as a function of just sinθ when k is odd
- When k is even sinkθ will be a function of sinθ multiplied by a factor of cosθ
How do I write tankθ in terms of powers of tanθ?
- STEP 1
Find expressions for sinkθ and coskθ using the previous method - STEP 2
Use the identity - STEP 3
Divide each term in the fraction by the highest power of cosθ to write each term using powers of tanθ and secθ - STEP 4 (Depending on the question)
Write everything in terms of tanθ using the identity
Exam Tip
- You can use the substitutions c = cosθ and s = sinθ to shorten your working as long as you clearly state them and change back at the end of the proof
Worked example
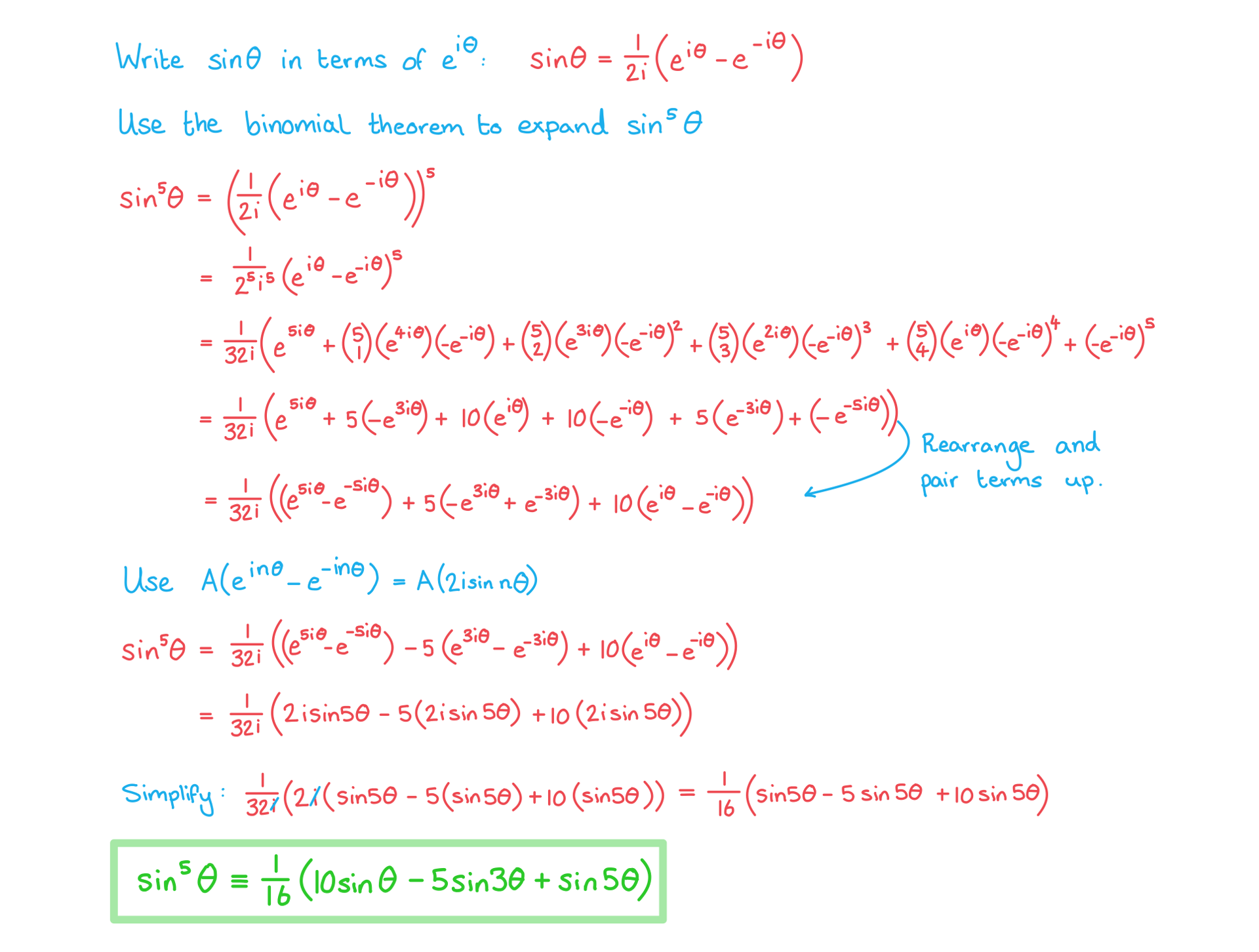
Prove that .