Approximating the Poisson Distribution (Edexcel International A Level (IAL) Maths): Revision Note
Exam code: YMA01
Calculating probabilities using a binomial or Poisson distribution can take a while. Under certain conditions we can use a normal distribution to approximate these probabilities. As we are going from a discrete distribution (binomial or Poisson) to a continuous distribution (normal) we need to apply continuity corrections.
Did this video help you?
Continuity Corrections
What are continuity corrections?
The binomial and Poisson distribution are discrete and the normal distribution is continuous
A continuity correction takes this into account when using a normal approximation
The probability being found will need to be changed from a discrete variable, X to a continuous variable, XN
For example, X = 4 for Poisson can be thought of as
for normal as every number within this interval rounds to 4
Remember that for a normal distribution the probability of a single value is zero so
How do I apply continuity corrections?
Think about what is largest/smallest integer that can be included in the inequality for the discrete distribution and then find its upper/lower bound
You add 0.5 as you want to include
in the inequality
You subtract 0.5 as you don't want to include
in the inequality
You subtract 0.5 as you want to include
in the inequality
You add 0.5 as you don't want to include
in the inequality
For a closed inequality such as
Think about each inequality separately and use above
Combine to give
Did this video help you?
Normal Approximation of Poisson
When can I use a normal distribution to approximate a Poisson distribution?
A Poisson distribution
can be approximated by a normal distribution
provided
is large
Remember that the mean and variance of a Poisson distribution are approximately equal, therefore the parameters of the approximating distribution will be:
The greater the value of λ in a Poisson distribution, the more symmetrical the distribution becomes and the closer it resembles the bell-shaped curve of a normal distribution
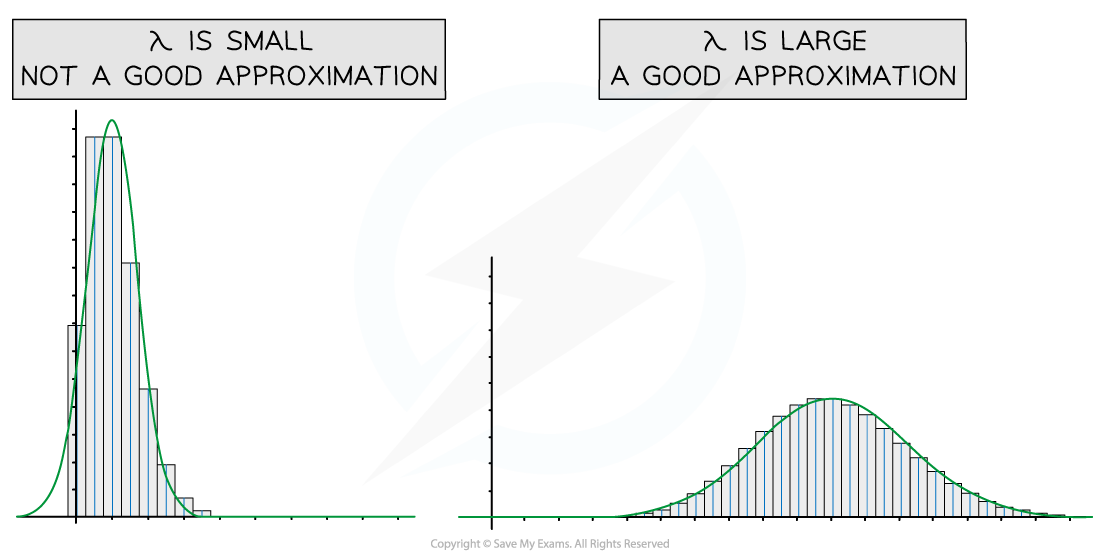
Why do we use approximations?
If there are a large number of values for a Poisson distribution there could be a lot of calculations involved and it is inefficient to work with the Poisson distribution
These days calculators can find Poisson probabilities so approximations are no longer necessary
However it can still be easier to work with a normal distribution
You can calculate the probability of a range of values quickly
You can use the inverse normal distribution function (most calculators don't have an inverse Poisson distribution function)
How do I approximate a probability?
STEP 1: Find the mean and variance of the approximating distribution
STEP 2: Apply continuity corrections to the inequality
STEP 3: Find the probability of the new corrected inequality
Find the standard normal probability and use the table of the normal distribution
The probability will not be exact as it is an approximation but provided λ is large enough then it will be a close approximation
Worked Example
The number of hits on a revision web page per hour can be modelled by the Poisson distribution with a mean of 40. Use a normal approximation to find the probability that there are more than 50 hits on the webpage in a given hour.
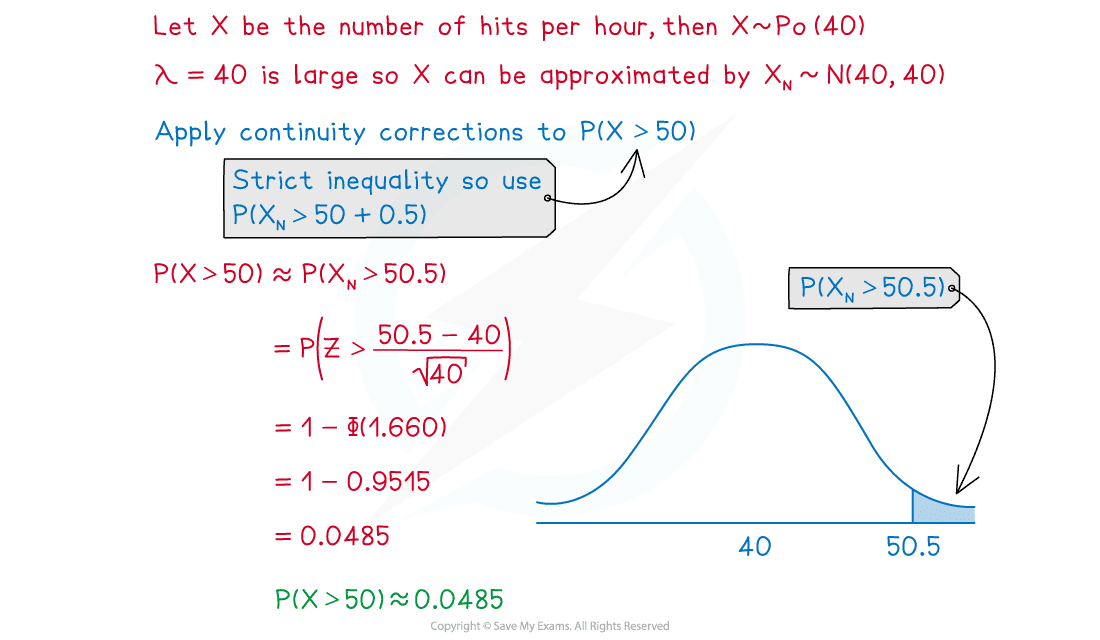
Examiner Tips and Tricks
The question will make it clear if an approximation is to be used, λ will be bigger than the values in the formula booklet.

Unlock more, it's free!
Did this page help you?