Geometric Series (Edexcel A Level Maths): Revision Note
Exam code: 9MA0
Did this video help you?
Geometric series
How do I find the sum of a geometric series?
A geometric series is the sum of the terms of a geometric sequence

The following formulae will let you find the sum of the first n terms of a geometric series:
or
a is the first term
r is the common ratio
The one on the left is more convenient if r < 1, the one on the right is more convenient if r > 1
The a and the r in those formulae are exactly the same as the ones used with geometric sequences
How do I prove the geometric series formula?
Learn this proof of the geometric series formula – you can be asked to give it in the exam:
Write out the sum once
Write out the sum again but multiply each term by r
Subtract the second sum from the first
All the terms except two should cancel out
Factorise and rearrange to make S the subject

What is the sum to infinity of a geometric series?
If (and only if!) |r| < 1, then the geometric series converges to a finite value given by the formula
S∞ is known as the sum to infinity
If |r| ≥ 1 the geometric series is divergent and the sum to infinity does not exist
Examiner Tips and Tricks
The geometric series formulae are in the formulae booklet – you don't need to memorise them
You will sometimes need to use logarithms to answer geometric series questions (see Exponential Equations)
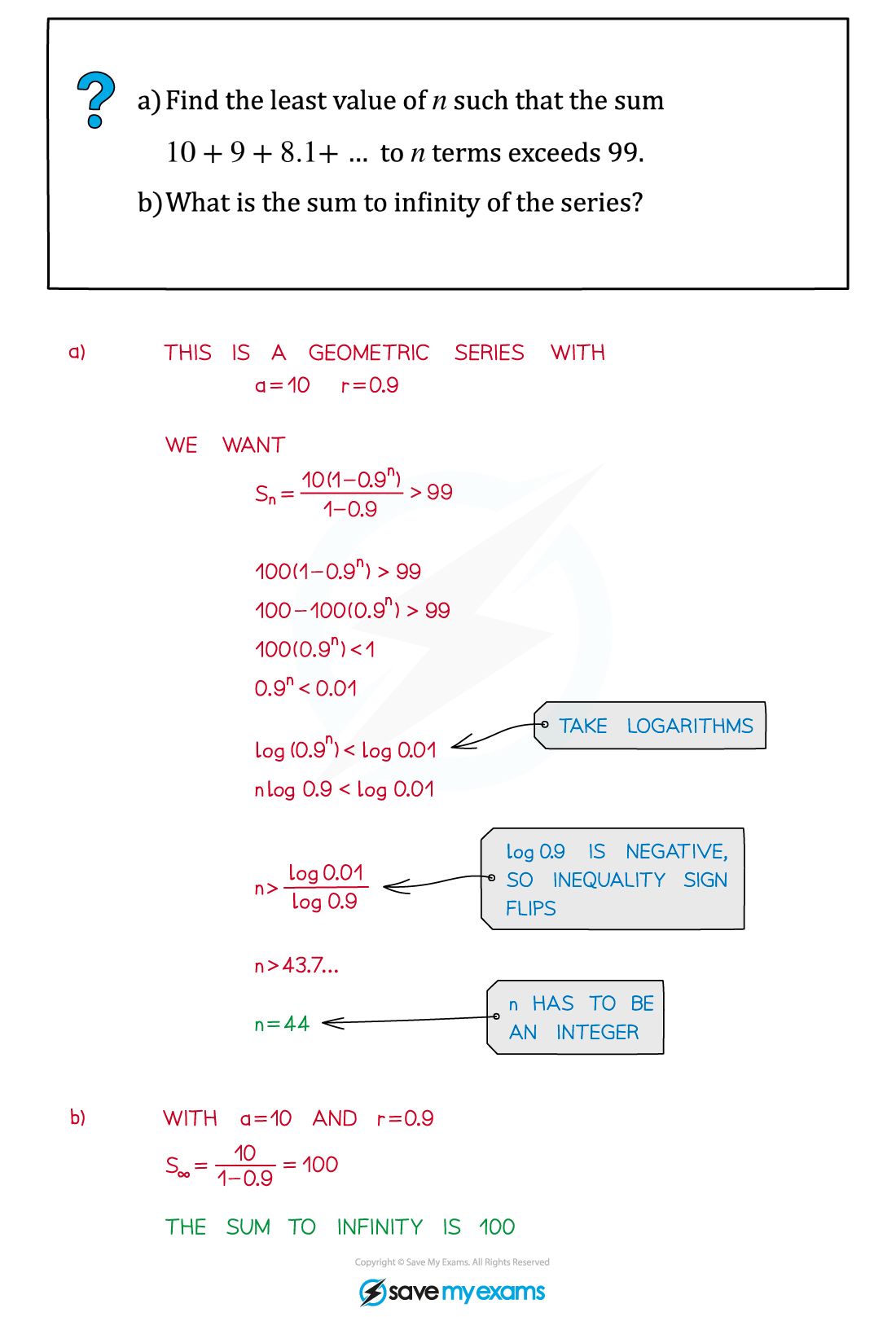
Worked Example

Unlock more, it's free!
Did this page help you?