Revision NotesExam QuestionsPast Papers
A LevelFurther MathsEdexcelCore PureRevision NotesFurther VectorsVector PlanesShortest Distances - Planes

Shortest Distances - Planes (Edexcel A Level Further Maths): Revision Note

Amber

Author

Amber

Last updated

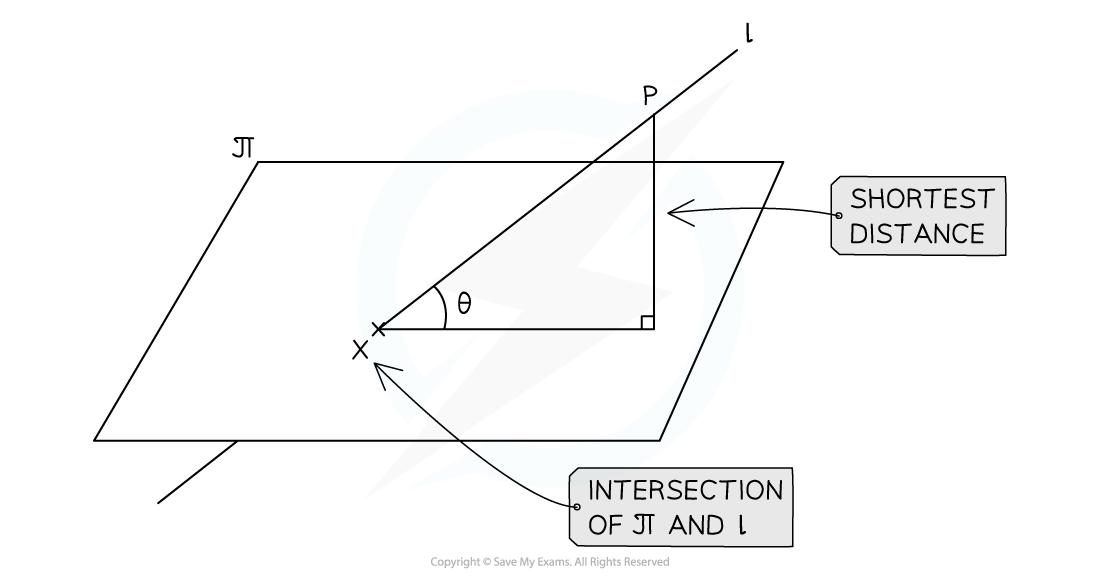
Shortest Distance between a Point & a Plane

How do I find the shortest distance between a given point on a line and a plane?

  • The shortest distance from any point on a line to a plane will always be the perpendicular distance from the point to the plane

  • Given a point, P, on the line l with equation bold r equals bold a plus lambda bold b and a plane capital pi with equation begin mathsize 16px style bold r times bold n equals d end style

    • STEP 1: Find the vector equation of the line perpendicular to the plane that goes through the point, P, on size 16px l

      • This will have the position vector of the point, P, and the direction vector n

    • STEP 2: Find the coordinates of the point of intersection of this new line with capital pi by substituting the equation of the line into the equation of the plane

    • STEP 3: Find the distance between the given point on the line and the point of intersection

      • This will be the shortest distance from the plane to the point

  • A question may provide the acute angle between the line and the plane 

    • Use right-angled trigonometry to find the perpendicular distance between the point on the line and the plane

      • Drawing a clear diagram will help

ZAFnkWOW_3-11-4-ib-hl-aa-shortest-dist-two-planes-diagram-1

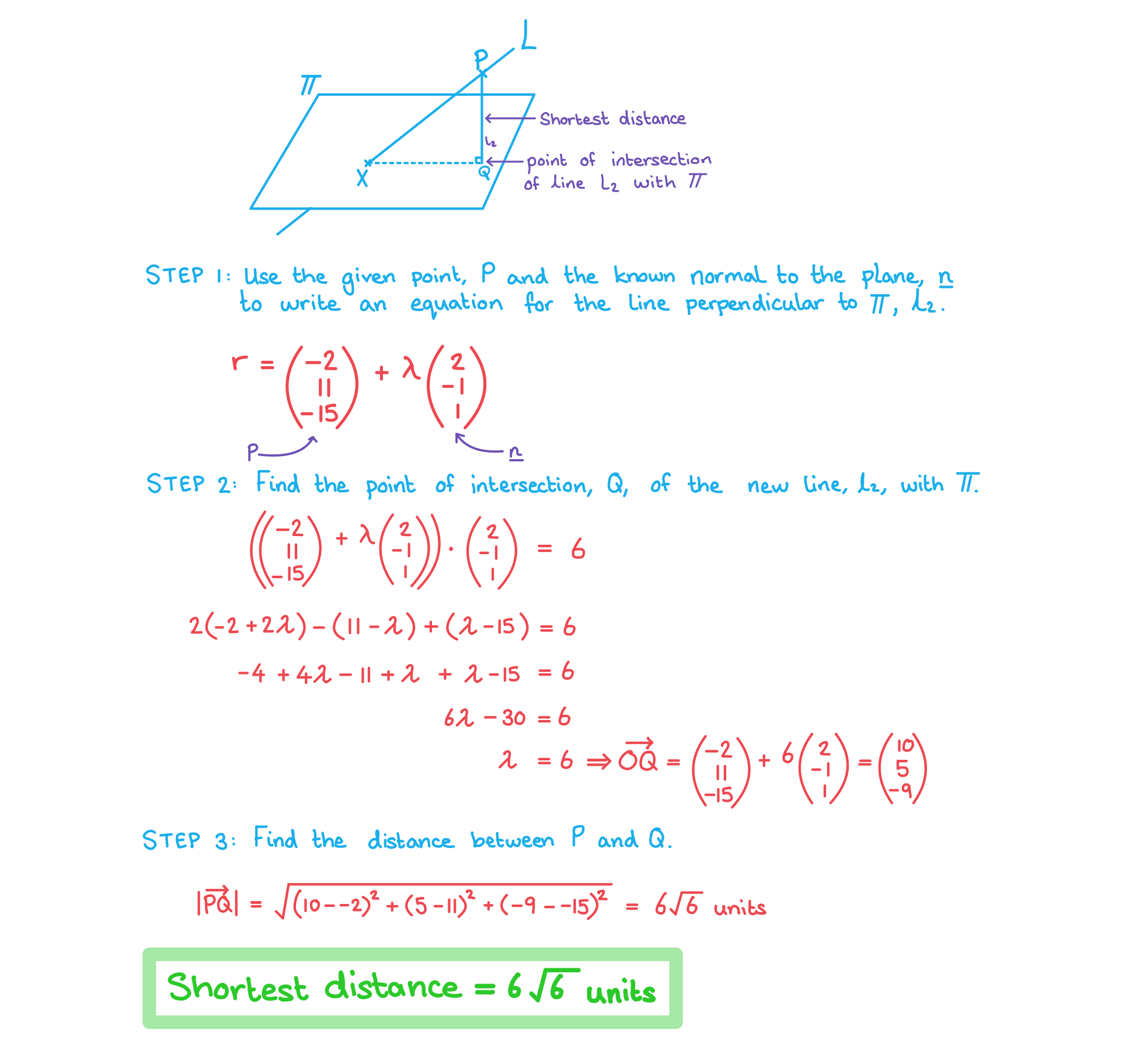
Worked Example

The plane capital pi has equation bold r times open parentheses table row 2 row cell negative 1 end cell row 1 end table close parentheses equals 6.

The line begin mathsize 16px style L end style has equation bold r equals open parentheses table row 2 row 3 row 1 end table close parentheses plus s open parentheses table row 1 row cell negative blank 2 end cell row 4 end table close parentheses.

The point P space left parenthesis negative 2 comma space 11 comma space minus 15 right parenthesis lies on the line begin mathsize 16px style L end style.

Find the shortest distance between the point P and the plane capital pi.

3-11-4-ib-hl-aa-shortest-dist-two-planes-we-1

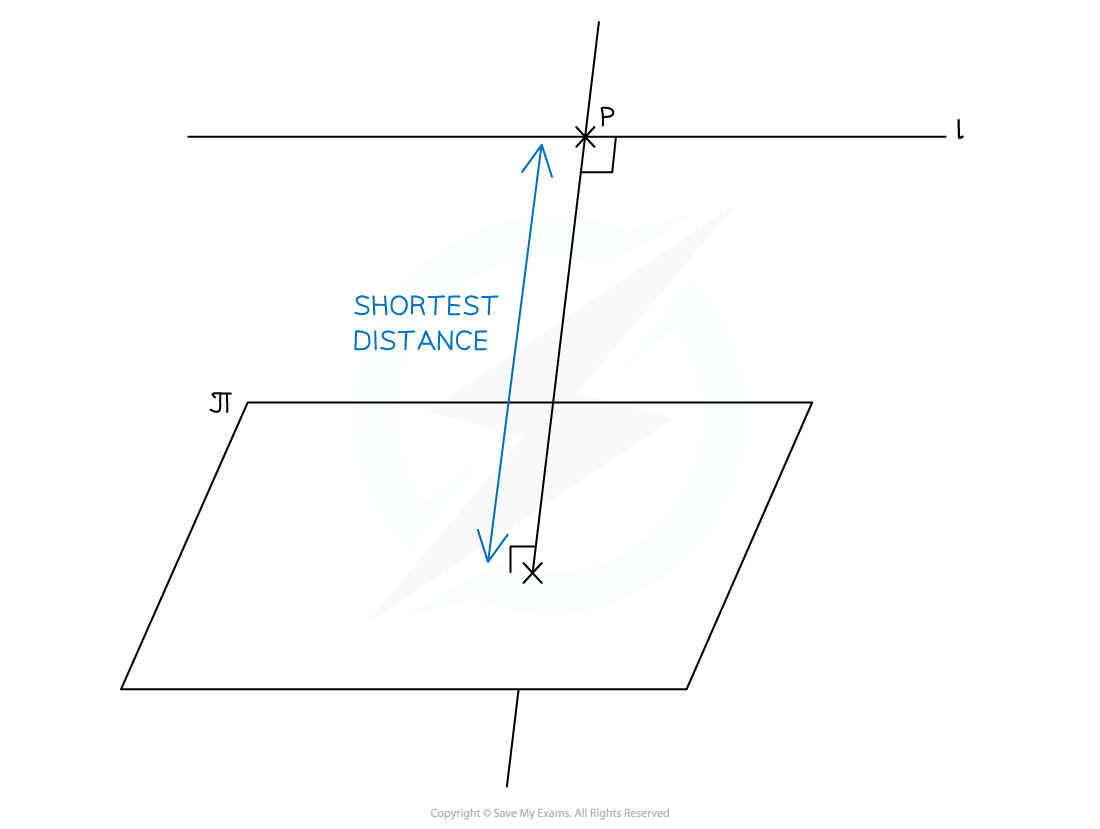
Shortest Distance between a Line & a Plane

How do I find the shortest distance between a plane and a line parallel to the plane?

  • The shortest distance between a line and a plane that are parallel to each other will be the perpendicular distance from the line to the plane

  • Given a line l subscript 1 with equation bold r equals bold a plus lambda bold b and a plane capital pi parallel to l subscript 1 with equation begin mathsize 16px style bold r times bold n equals d end style

    • Where n is the normal vector to the plane

    • STEP 1: Find the equation of the line l subscript 2 perpendicular to l subscript 1 and capital pi going through the point a in the form bold r equals bold a plus mu bold n

    • STEP 2: Find the point of intersection of the line size 16px l subscript 2 and capital pi

    • STEP 3: Find the distance between the point of intersection and the point,

3-11-4-ib-hl-aa-shortest-dist-two-planes-diagram-2-1

Shortest Distance between two Planes

How do I find the shortest distance between two parallel planes?

  • Two parallel planes will never intersect

  • The shortest distance between two parallel planes will be the perpendicular distance between them

  • Given a plane capital pi subscript 1 with equation begin mathsize 16px style bold r times bold n equals d end style and a plane capital pi subscript 2 with equation bold r equals bold a plus lambda bold b plus blank mu bold c then the shortest distance between them can be found

    • STEP 1: The equation of the line perpendicular to both planes and through the point a can be written in the form r = a + sn

    • STEP 2: Substitute the equation of the line into bold r times bold n equals d to find the coordinates of the point where the line meets capital pi subscript 1

    • STEP 3: Find the distance between the two points of intersection of the line with the two planes

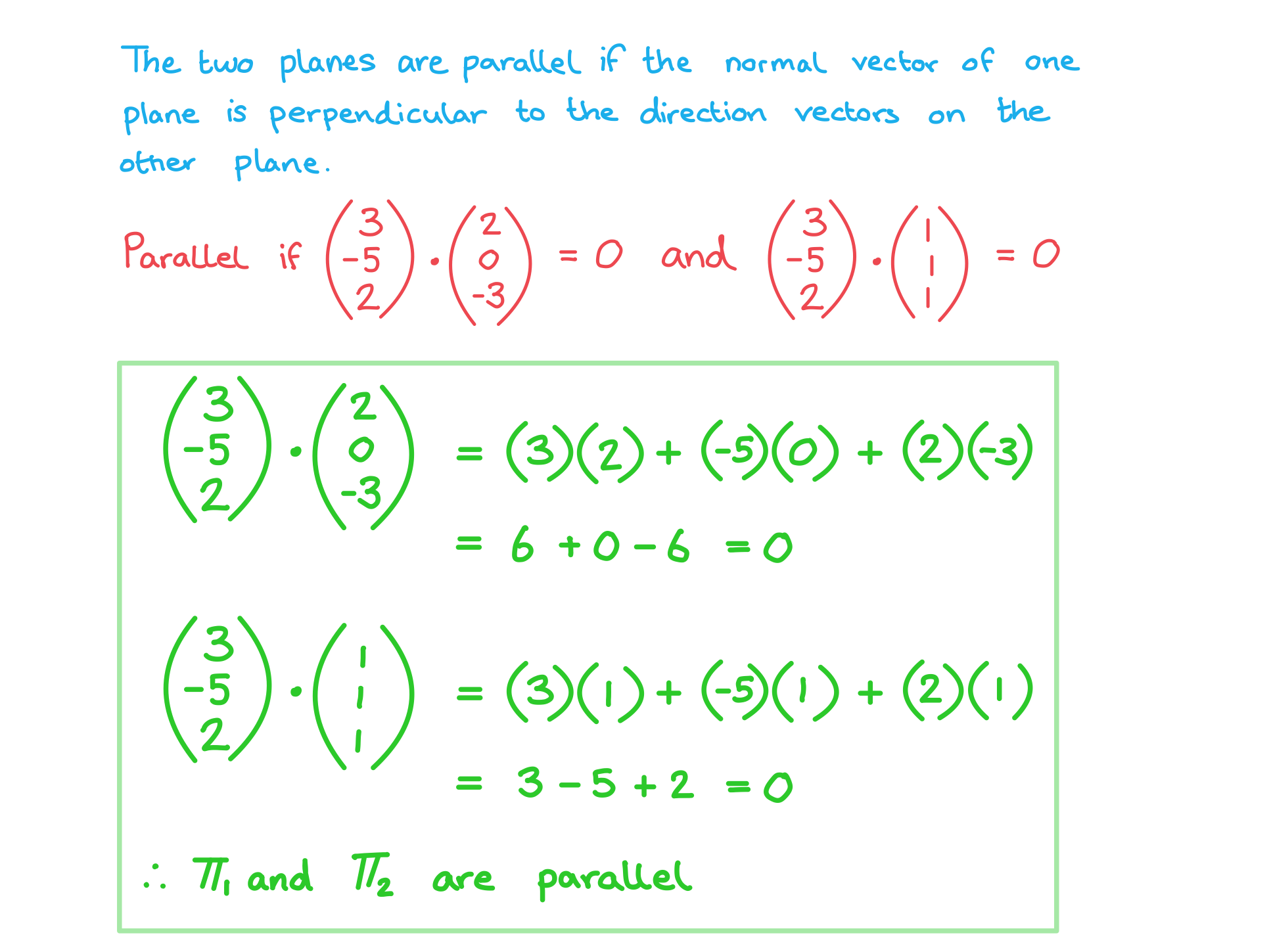
Consider the parallel planes defined by the equations:

capital pi subscript 1 blank colon space space bold r times open parentheses table row 3 row cell negative 5 end cell row 2 end table close parentheses space equals space 44,

capital pi subscript 2 blank colon space space bold r bold space equals space open parentheses table row 0 row 0 row 3 end table close parentheses space plus space lambda open parentheses table row 2 row 0 row cell negative 3 end cell end table close parentheses space plus space mu open parentheses table row 1 row 1 row 1 end table close parentheses.

a) Show that the two planes capital pi subscript 1 and capital pi subscript 2 are parallel.

al-fm-6-2-2-shortest-distance-between-two-planes-we-solution-a

 

b) Find the shortest distance between the two planes capital pi subscript 1 and capital pi subscript 2.

al-fm-6-2-2-shortest-distance-between-two-planes-we-solution-b

You've read 0 of your 5 free revision notes this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Combinations of PlanesNext:Polar Coordinates
    Amber

    Author: Amber

    Expertise: Maths Content Creator

    Amber gained a first class degree in Mathematics & Meteorology from the University of Reading before training to become a teacher. She is passionate about teaching, having spent 8 years teaching GCSE and A Level Mathematics both in the UK and internationally. Amber loves creating bright and informative resources to help students reach their potential.