Integration by Parts (Edexcel A Level Maths): Revision Note
Exam code: 9MA0
Did this video help you?
Integration by Parts
What is Integration by Parts?
For integrating the product of two functions - reverse product rule
Crucially the product is made from u and dv/dx (rather than u and v)
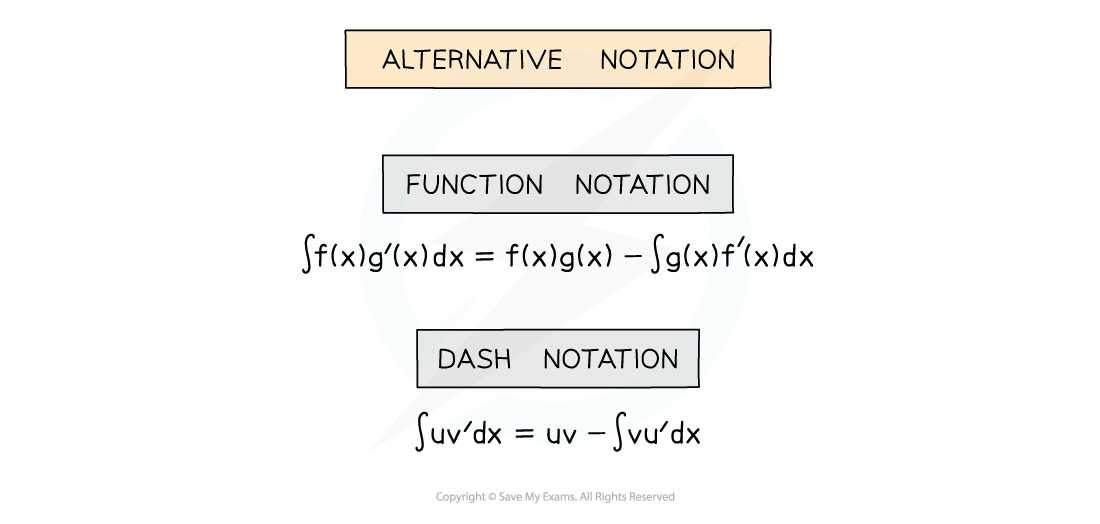
Alternative notation may be used
How do I use Integration by Parts?
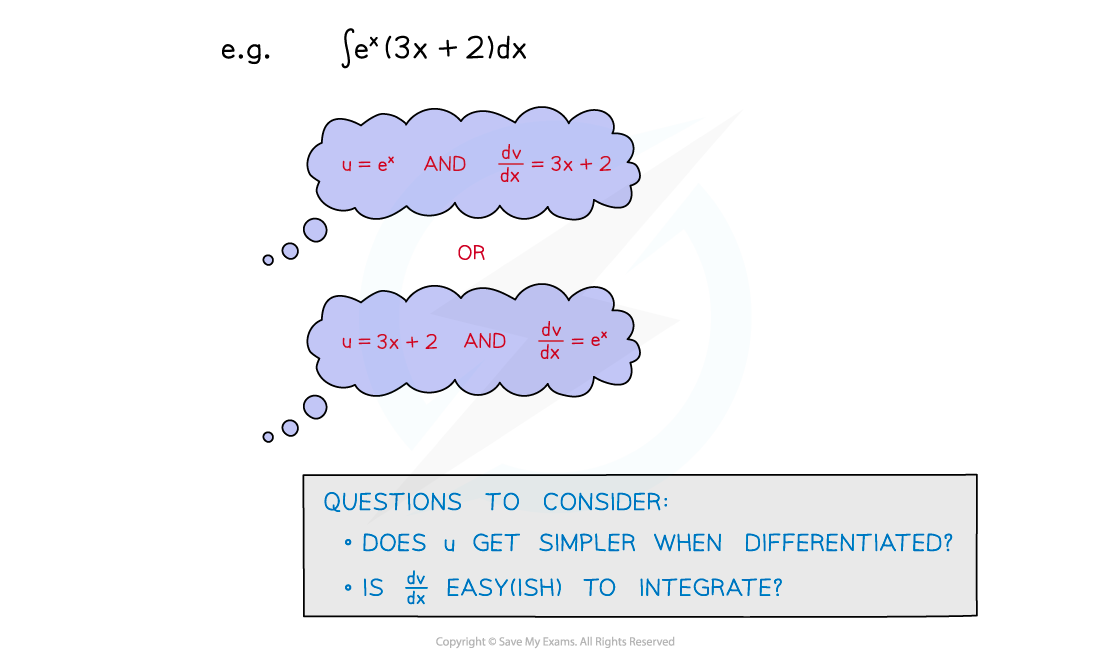
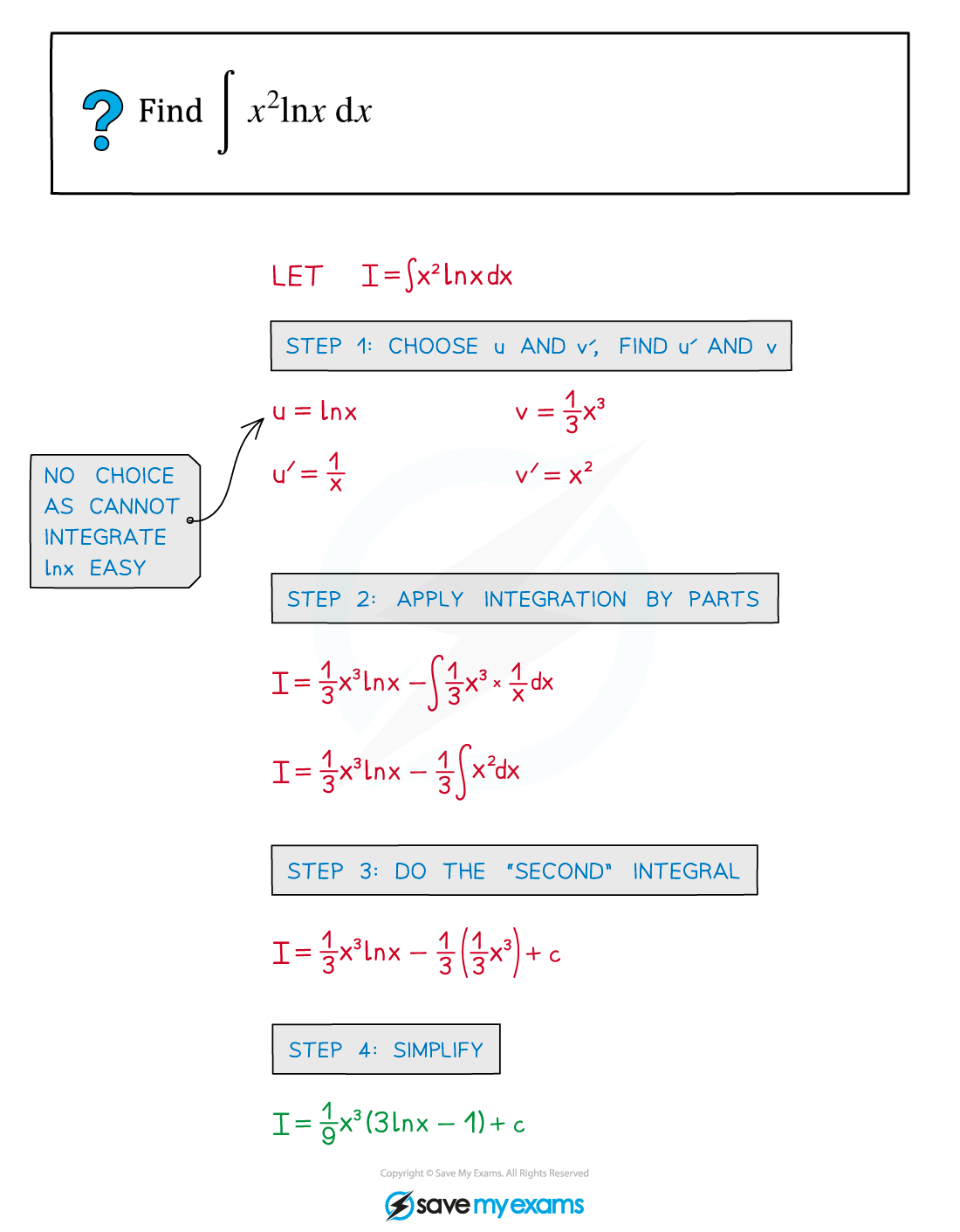
The hardest part is choosing u and dv/dx as there is no method for doing so
u, ideally, becomes simpler when differentiated but this is not always possible
dv/dx should be a function that can be integrated fairly easily
Be wary of functions that ‘cycle’/’repeat’ when differentiated/integrated
ex → ex
sin x → cos x → -sin x → -cos x → sin x
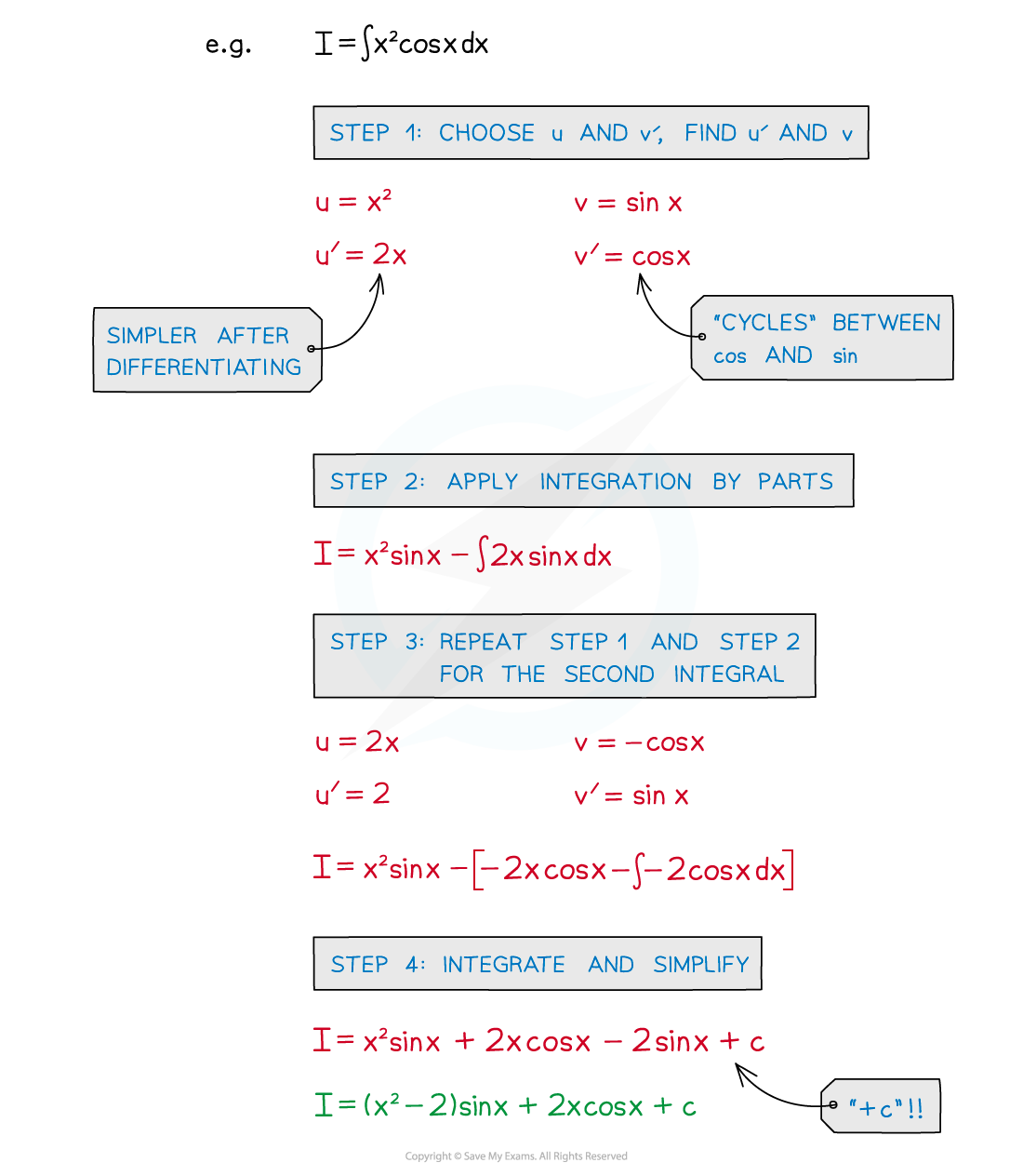
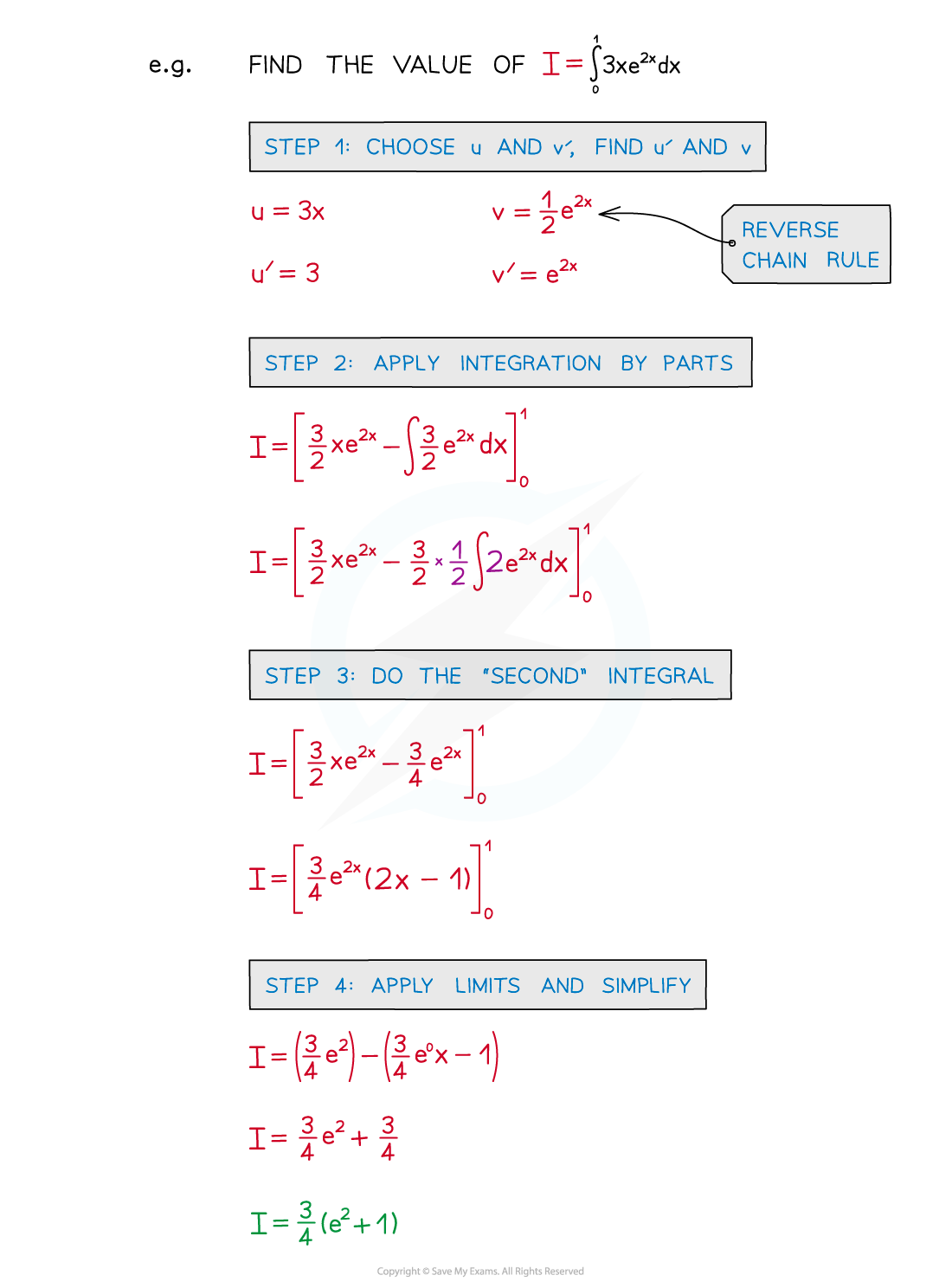
STEP 1: Choose u and v’, find u’ and v
STEP 2: Apply Integration by Parts
Simplify anything straightforward
STEP 3: Do the ‘second’ integral
If an indefinite integral remember “+c”, the constant of integration
STEP 4: Simplify and/or apply limits
What happens if I cannot integrate v × du/dx?
It is possible integration by parts may need to be applied more than once
ln x
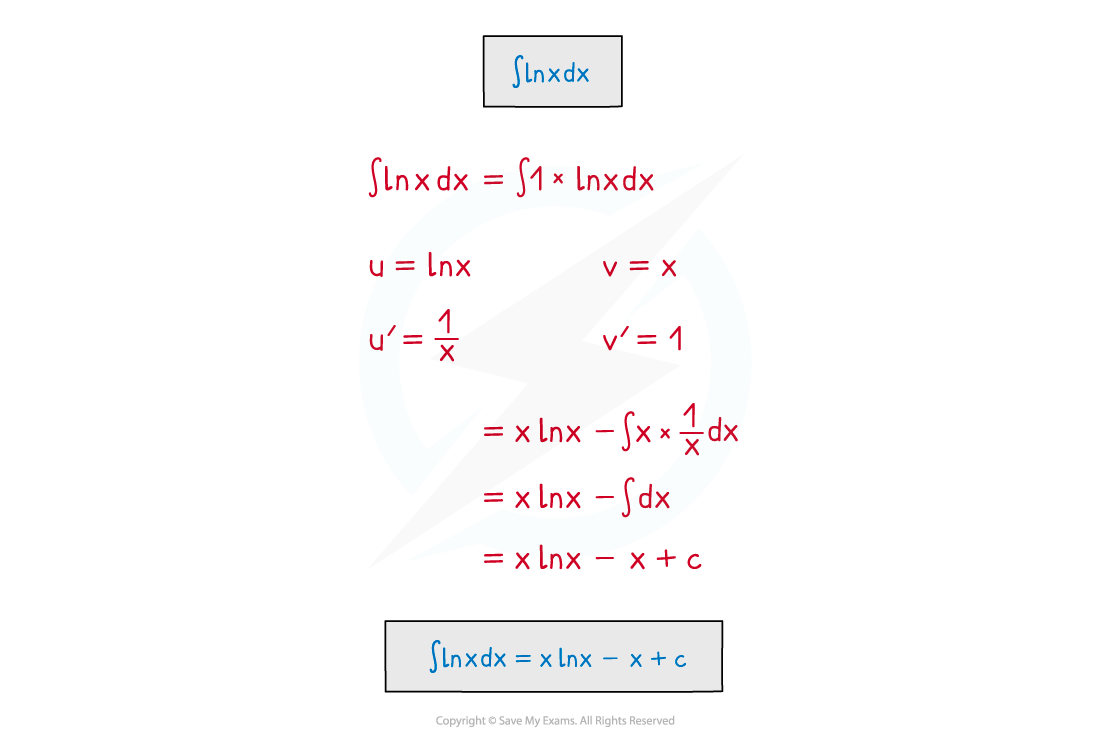
A classic ‘set piece’ in almost every A level maths textbook ever written!
In general, rewriting f(x) as 1×f(x) can be a powerful problem-solving technique
This could be a question in the exam
How do I find a definite integral using parts?
Examiner Tips and Tricks
Always think about what an elegant, slick, professional maths solution looks like – solutions normally get more complicated at first but quickly get simpler.
If your work is continuing to get more complicated, stop and check for an error.
Try to develop a sense of ‘having gone too far down the wrong path’.
This general advice is useful to remember:
Is the second integral harder than the first?
Try swapping your choice of u and dv/dx
It is rare to have to apply integration by parts more than twice
Worked Example
Unlock more revision notes. It's free!
By signing up you agree to our Terms and Privacy Policy.
Already have an account? Log in
Did this page help you?