Shapes of Linear, Quadratic & Cubic Graphs (AQA Level 3 Mathematical Studies (Core Maths)): Revision Note
Exam code: 1350
Shapes of Linear Graphs
What is the equation of a straight line?
is the equation for any straight line
is gradient given by “difference in
” ÷ “difference in
”
You may also see this written as
is the y-axis intercept
An alternative form is
Where
,
and
are integers

How do I find the equation of a straight line?
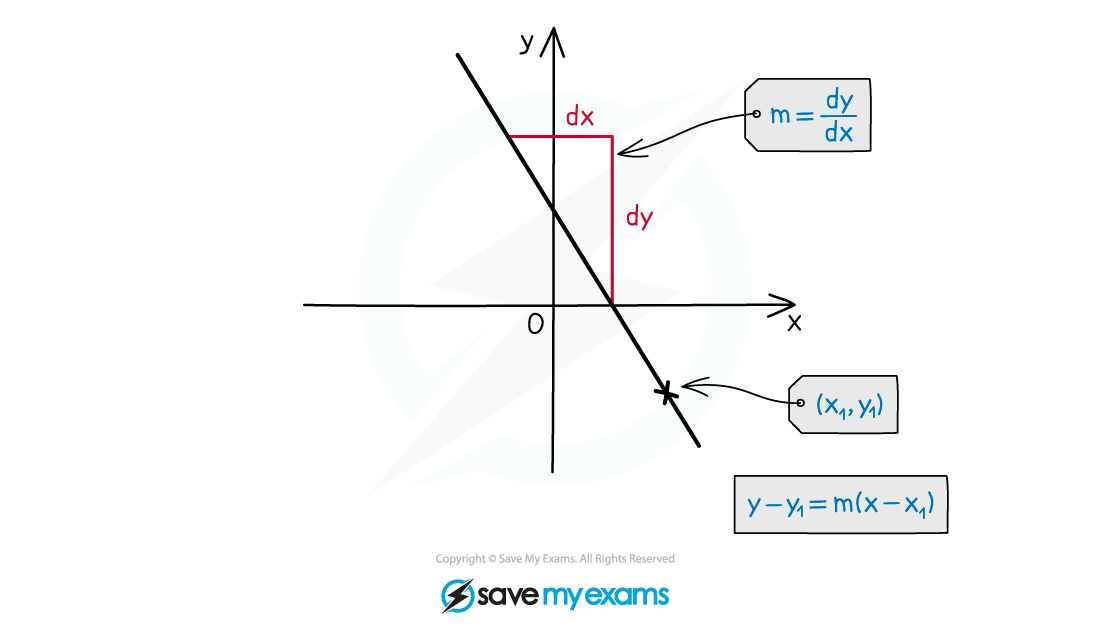
Two features of a straight line are needed
Gradient,
A point the line passes through,
The equation can then be found using
This can be arranged into either
or
How do I find the gradient of a straight line?
There are lots of ways to find the gradient of a line
Using two points on a line to find the change in
divided by change in
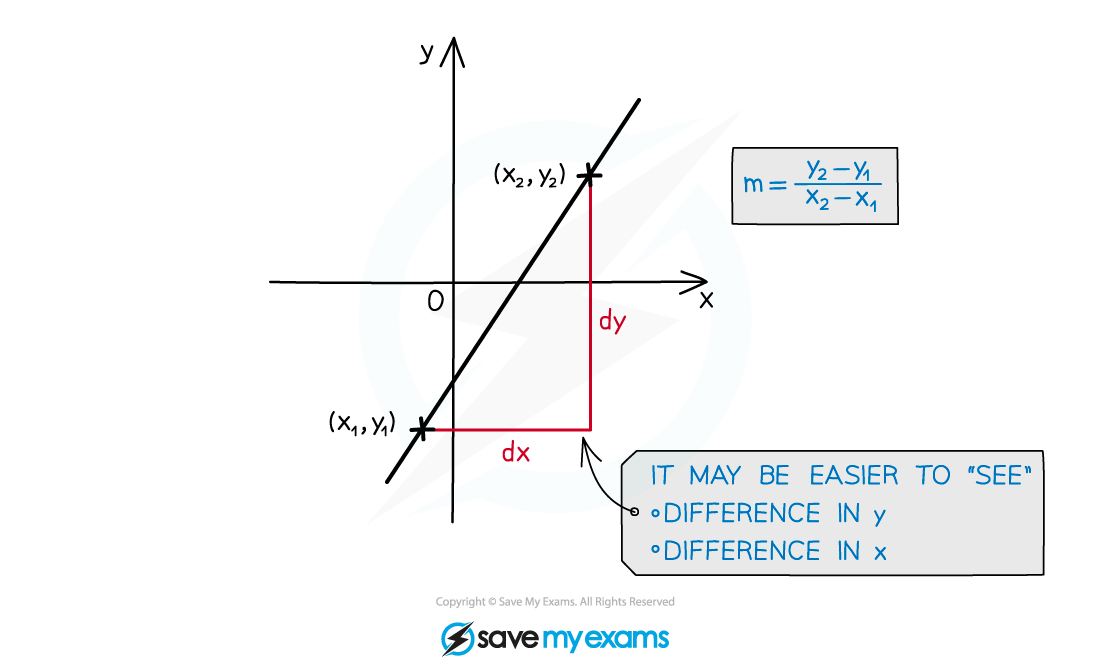
Using the fact that lines are parallel or perpendicular to another line
The gradient of parallel lines are equal
The gradient of perpendicular lines are negative reciprocals of each other
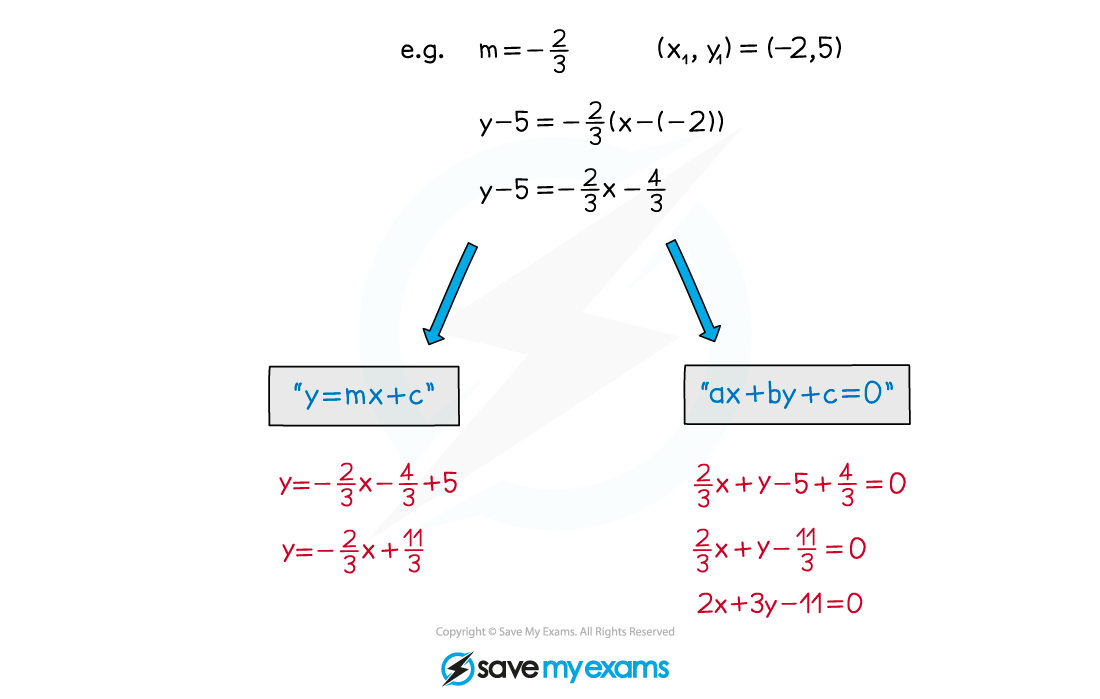
E.g.
and

Worked Example
The line passes through the points
and
.
Find the equation of , giving your answer in the form
where
and
are integers to be found.
First find the gradient of the line
Substitute the gradient and the coordinates of one of the points into
Multiply both sides of the equation by 2 to get rid of the fraction
Rearrange into the required form
Shapes of Quadratic Graphs
What is a quadratic?
A quadratic is a function of the form
where
is not zero
They are a very common type of function in mathematics, so it is important to know their key features
What does a quadratic graph look like?
The shape made by a quadratic graph is known as a parabola
The parabola shape of a quadratic graph can either look like a “u-shape” or an “n-shape”
A quadratic with a positive coefficient of
will be a u-shape
A quadratic with a negative coefficient of
will be an n-shape
A quadratic will always cross the
-axis
A quadratic may cross the
-axis twice, once, or not at all
The points where the graph crosses the
-axis are called the roots
If the quadratic is a u-shape, it has a minimum point (the bottom of the u)
If the quadratic is an n-shape, it has a maximum point (the top of the n)
Minimum and maximum points are both examples of turning points

How do I sketch a quadratic graph?
We could create a table of values for the function and then plot it accurately, however we often only require a sketch to be drawn, showing just the key features
The most important features of a quadratic are
Its overall shape; a u-shape or an n-shape
Its
-intercept
Its
-intercept(s), these are also known as the roots
Its minimum or maximum point (turning point)
If it is a positive quadratic (
in
is positive) it will be a u-shape
If it is a negative quadratic (
in
is negative) it will be an n-shape
The
-intercept of
will be
The roots, or the
-intercepts will be the solutions to
;
You can solve a quadratic by factorising, completing the square, or using the quadratic formula
There may be 2, 1, or 0 solutions and therefore 2, 1, or 0 roots
The minimum or maximum point of a quadratic can be found by completing the square
Once the quadratic has been written in the form
, the minimum or maximum point is given by
Be careful with the sign of the x-coordinate
E.g. if the equation is
then the minimum point is
but if the equation is
then the minimum point is
Worked Example
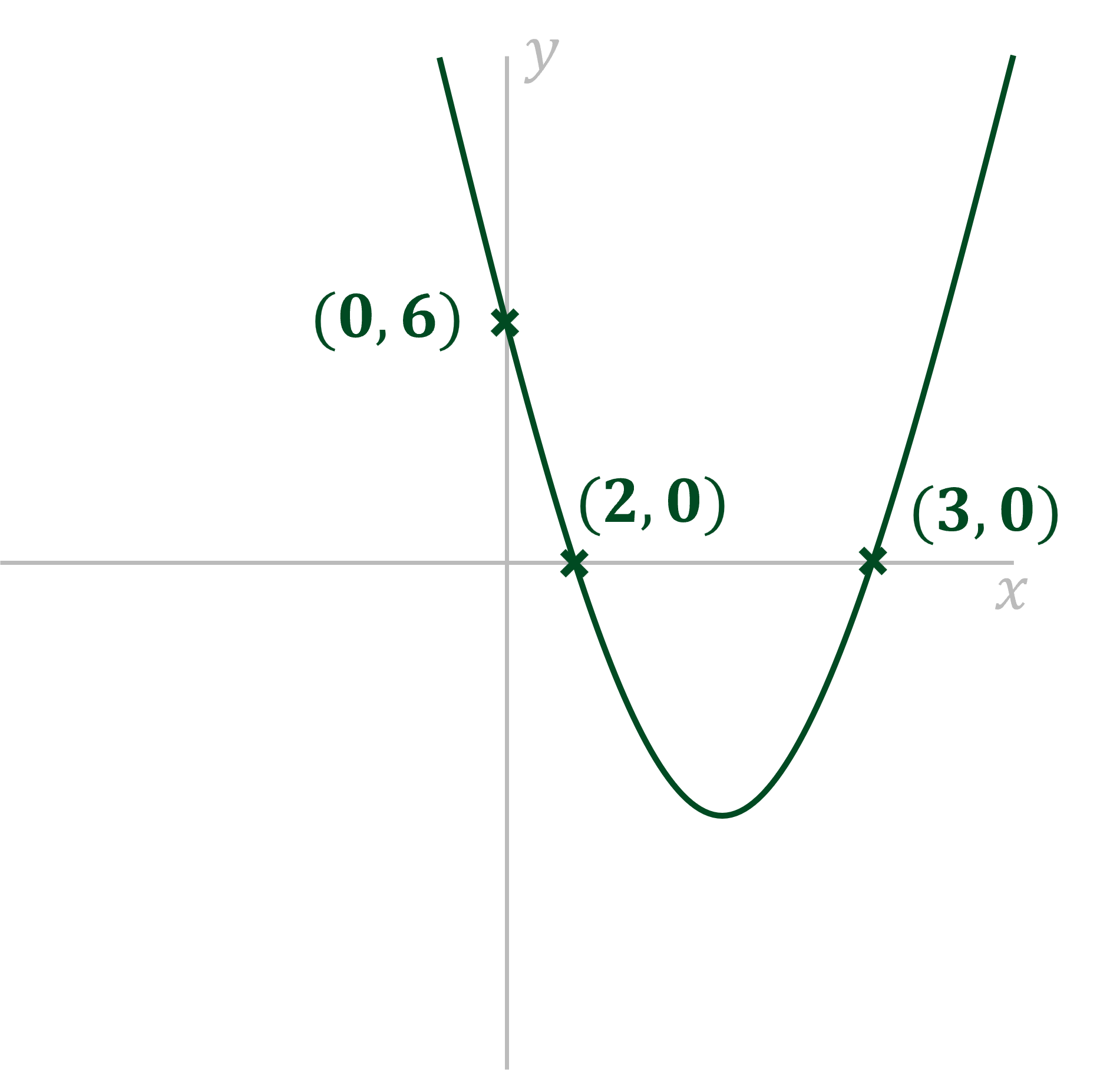
(a) Sketch the graph of showing the
and
intercepts.
It is a positive quadratic, so will be a u-shape
The at the end is the
-intercept, so this graph crosses the
-axis at
(0,6)
Factorise
Solve
So the roots of the graph are
(2,0) and (3,0)
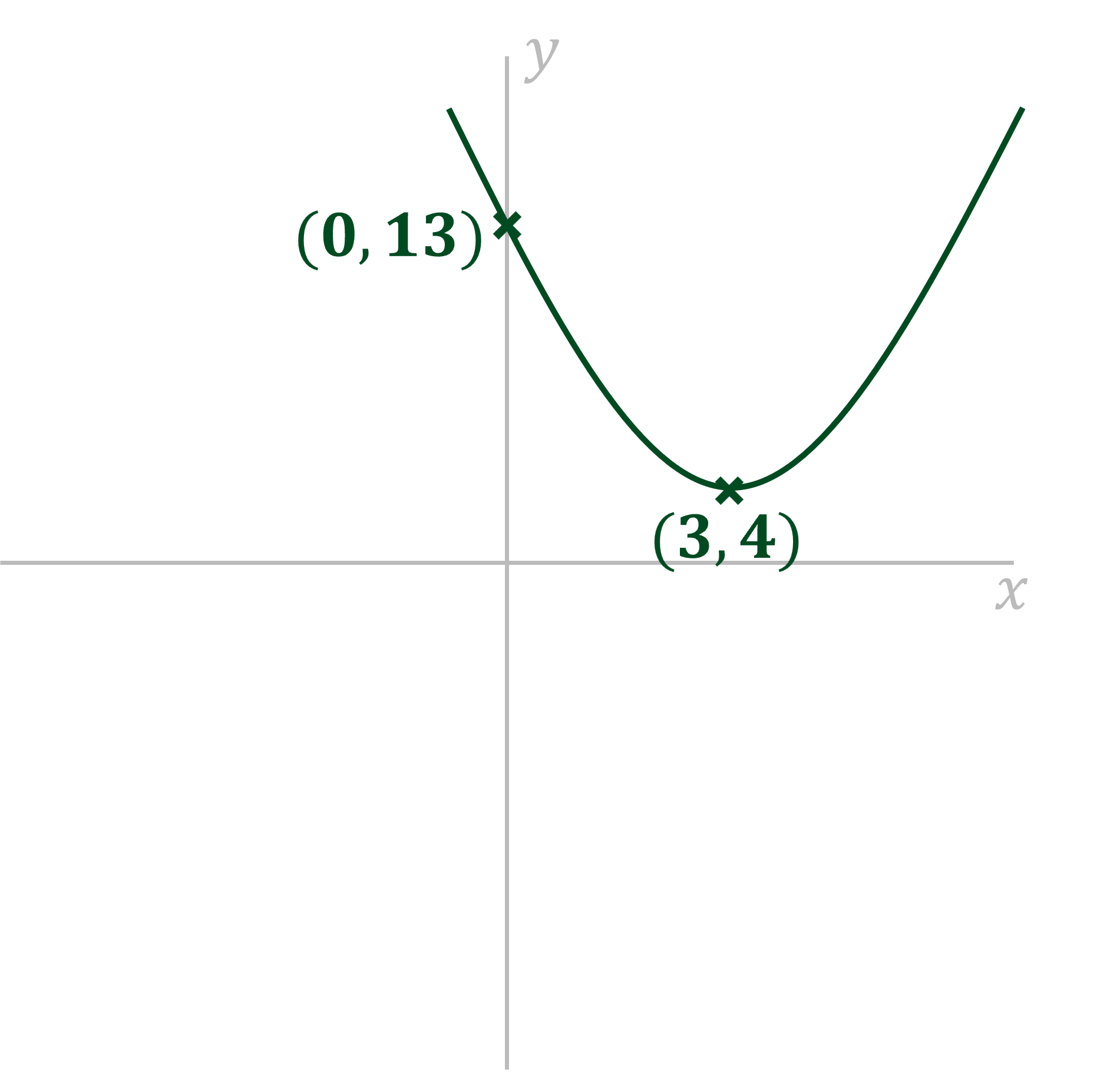
(b) Sketch the graph of showing the
-intercept and the turning point.
It is a positive quadratic, so will be a u-shape
The at the end is the
-intercept, so this graph crosses the y-axis at
(0,13)
We can find the minimum point (it will be a minimum as it is a positive quadratic) by completing the square
This shows that the minimum point will be
(3,4)
As the minimum point is above the -axis, this means the graph will not cross the
-axis, i.e. it has no roots
We could also show that there are no roots by trying to solve
If we use the quadratic formula, we will find that is the square root of a negative number
This is not a real number, which means there are no real solutions, and hence there are no roots
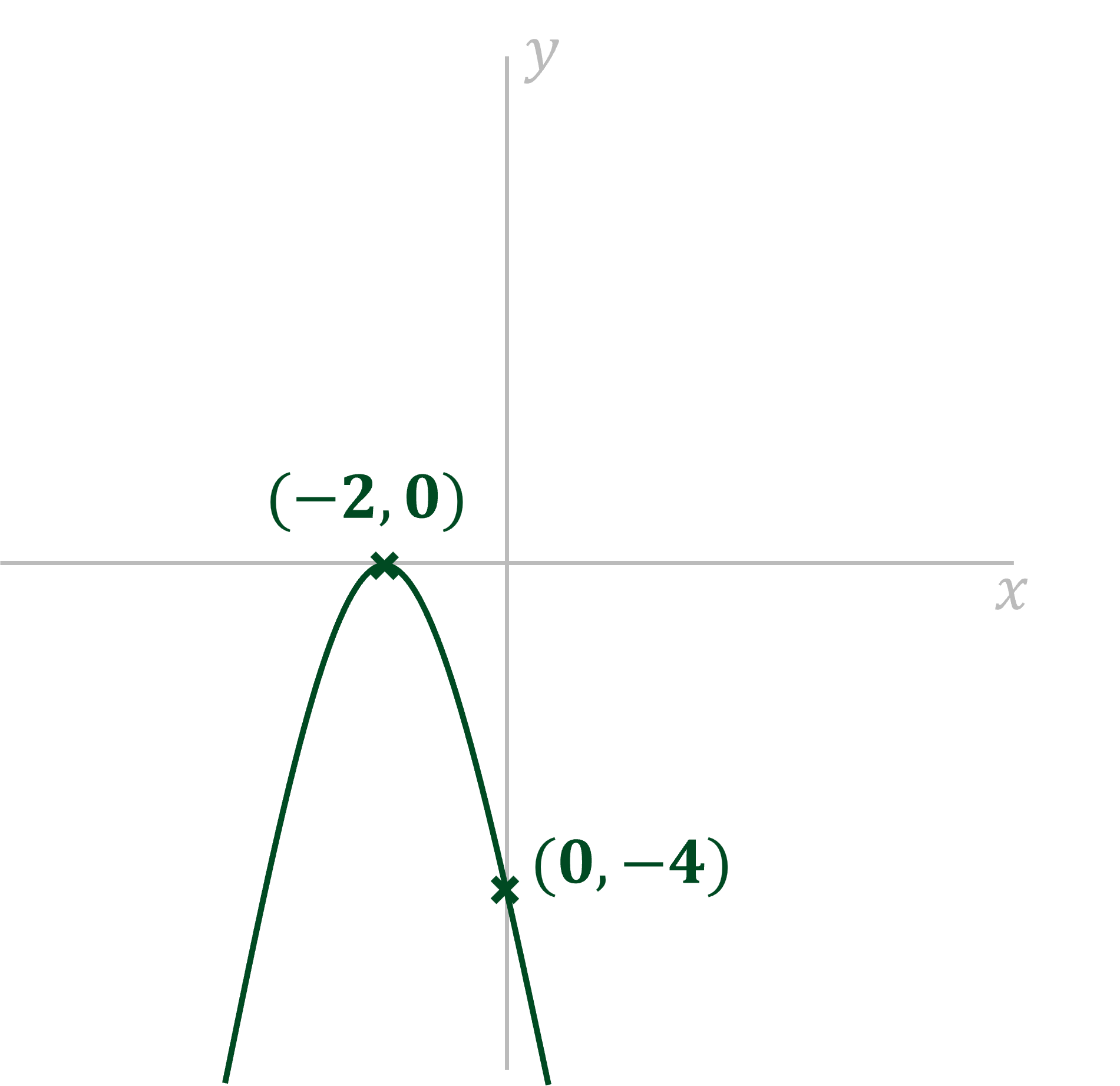
(c) Sketch the graph of showing the root(s),
-intercept, and turning point.
It is a negative quadratic, so will be an n-shape
The at the end is the
-intercept, so this graph crosses the
-axis at
(0, -4)
We can find the maximum point (it will be a maximum as it is a negative quadratic) by completing the square
This shows that the maximum point will be
(-2, 0)
As the maximum is on the -axis, there is only one root
We could also show that there is only one root by solving
If you use the quadratic formula, you will find that the two solutions for are the same number; in this case -2
Shapes of Cubic Graphs
What is a cubic?
A cubic polynomial is a function of the form
and
are constants
It is a polynomial of degree 3
So
and/or
could be zero
To sketch the graph of a cubic polynomial it will need to be in factorised form
E.g.
is the factorised form of
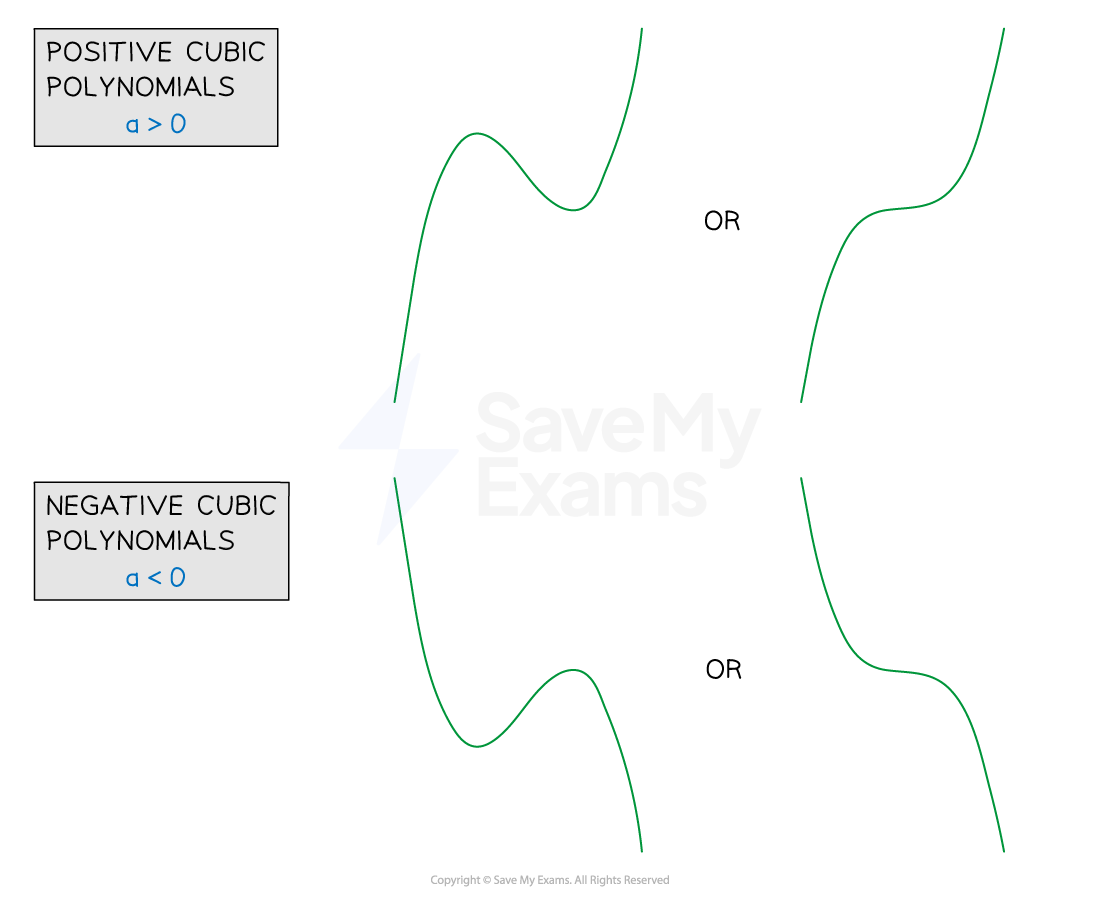
What does the graph of a cubic polynomial look like?
In general the graph of a cubic polynomial will take one of the four forms
All are smooth curves that take some practice to sketch!
The exact form a particular cubic polynomial will depend on:
The number (and value) of roots (
-axis intercepts) of the cubic polynomial
The
-axis intercept
The sign of the coefficient of the
term (
)
If
the graph is a positive cubic ('starts' in the third quadrant, 'ends' in the first)
If
the graph is a negative cubic ('starts' in the second quadrant, 'ends' in the fourth)
The turning points
How do I sketch the graph of a cubic polynomial?
STEP 1
Find the-axis intercept by setting
STEP 2
Find the-axis intercepts (roots) by setting
Any repeated roots will mean the graph touches, rather than crosses, the-axis
STEP 3
Consider the shape of the graph; is it a positive cubic or a negative cubic?
Where does the graph 'start' and 'end'?STEP 4
Consider where any turning points should goSTEP 5
Sketch the graph with a smooth curve, labelling points where the graph intercepts theand
axes
Worked Example
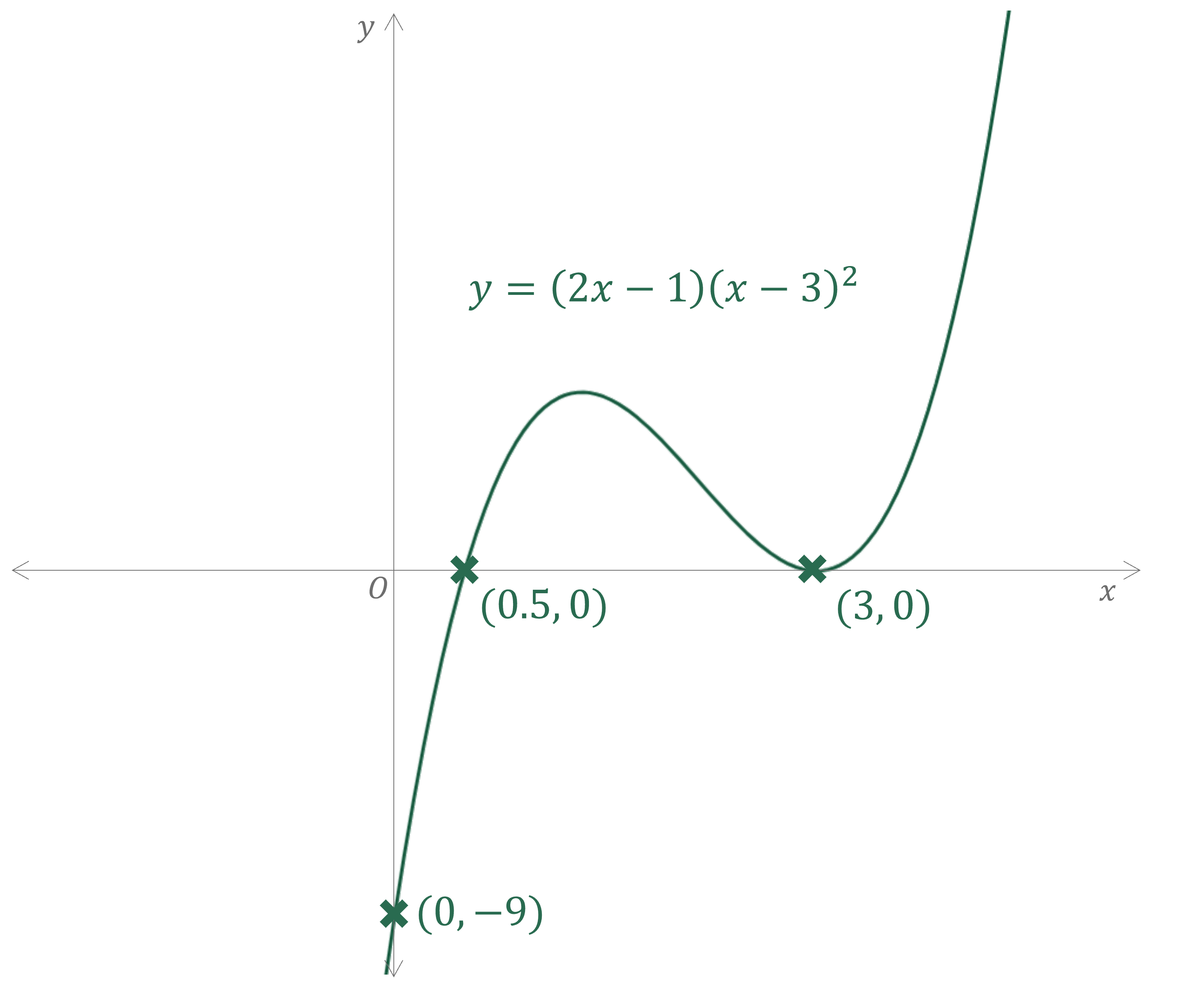
Sketch the graph of .
Find the -axis intercept by substituting in
Find the -axis intercepts by solving
Either bracket can be equal to zero
(repeated solution, as there are two brackets
Consider the shape, and the 'start' and 'end' points
so it is a positive cubic
is a repeated root so the graph will touch the
-axis at this point
Consider the turning points
One turning point (minimum) will need to be where the curve touches the -axis
The other (maximum) will need to be between the two roots and
Sketch a smooth curve with labelled intercepts

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?