Increasing & Decreasing Functions (OCR A Level Maths A): Revision Note
Exam code: H240
Did this video help you?
Increasing & decreasing functions
What are increasing and decreasing functions?
A function f(x) is increasing on an interval [a, b] if f'(x) ≥ 0 for all values of x such that a< x < b.
If f'(x) > 0 for all x values in the interval then the function is said to be strictly increasing
In most cases, on an increasing interval the graph of a function goes up as x increases
A function f(x) is decreasing on an interval [a, b] if f'(x) ≤ 0 for all values of x such that a < x < b
If f'(x) < 0 for all x values in the interval then the function is said to be strictly decreasing
In most cases, on a decreasing interval the graph of a function goes down as x increases
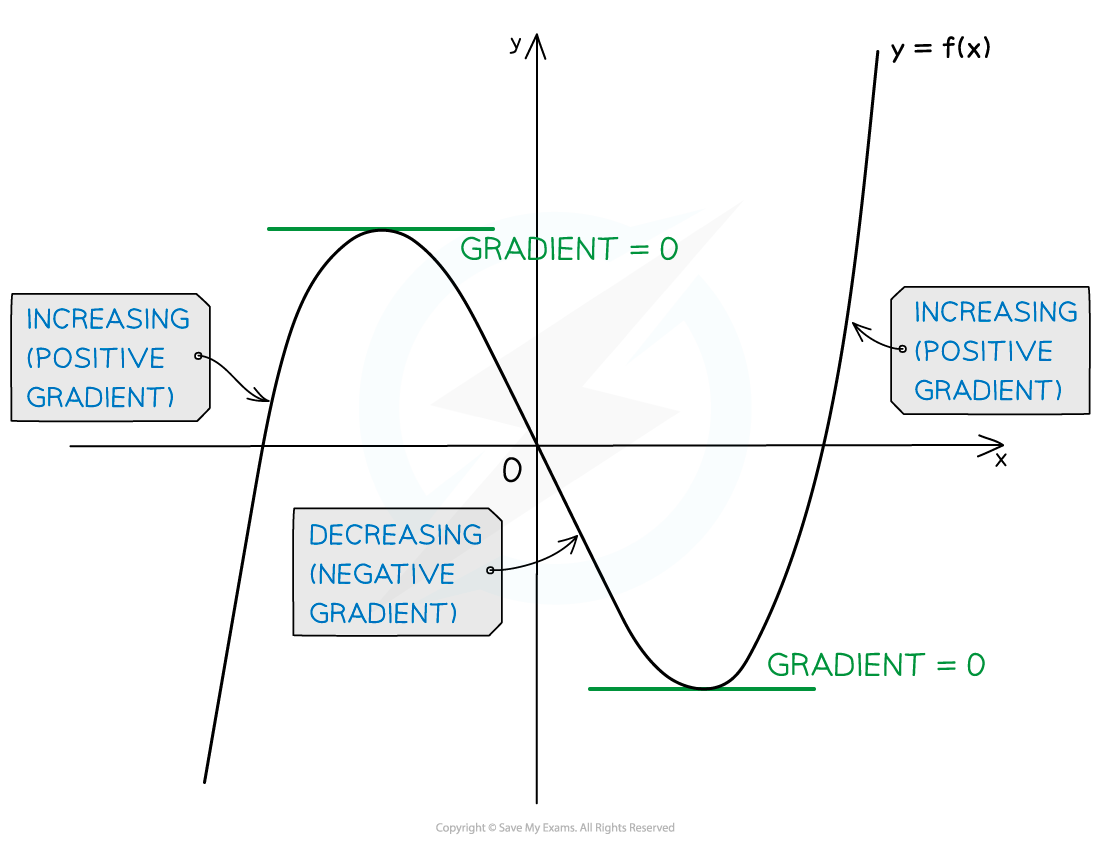
To identify the intervals on which a function is increasing or decreasing you need to:
Find the derivative f'(x)
Solve the inequalities f'(x) ≥ 0 (for increasing intervals) and/or f'(x) ≤ 0 (for decreasing intervals)
Examiner Tips and Tricks
On an exam, if you need to show a function is increasing or decreasing you can use either strict (<, >) or non-strict (≤, ≥) inequalities
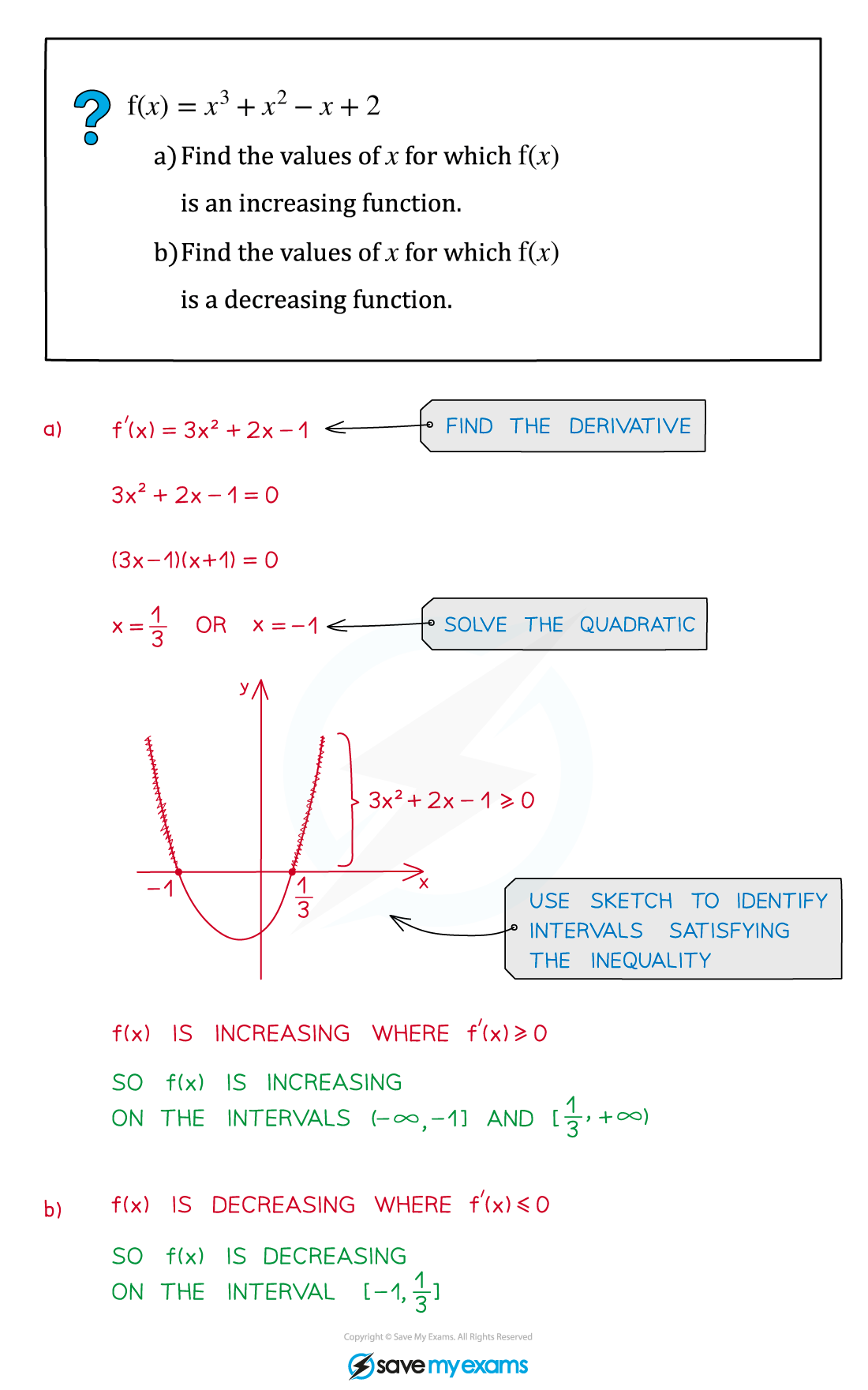
Worked Example

Unlock more, it's free!
Did this page help you?