A LevelMathsOCRMaths APureRevision NotesAlgebra & FunctionsFunctionsSolving Equations with Modulus Functions

Solving Equations with Modulus Functions (OCR A Level Maths A): Revision Note

Paul

Author

Paul

Last updated

OCR Maths A: PureCambridge (CIE) Maths: Pure 3AQA Maths: PureEdexcel Maths: Pure

Did this video help you?

Modulus Functions - Solving Equations

Modulus graphs and equations

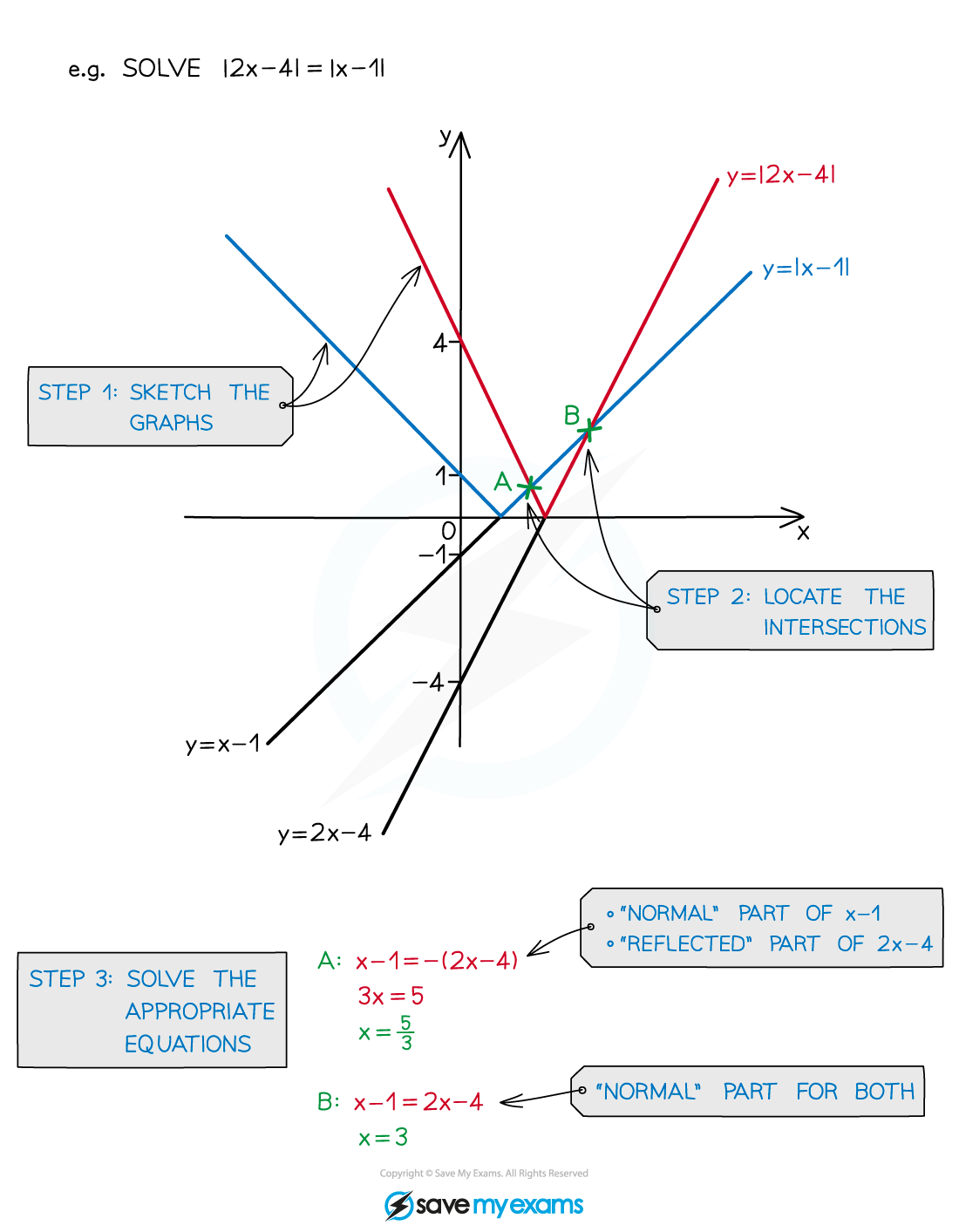
  • Two non-parallel straight-line graphs would intersect once

  • If modulus involved there could be more than one intersection

  • Deducing where these intersections are is crucial to solving equations

2-8-5-modulus-functions---solving-equations-notes-diagram-2_1

How do I solve modulus equations?

  • STEP 1        Sketch the graphs including any modulus (reflected) parts

    • (see Modulus Functions – Sketching Graphs)

  • STEP 2        Locate the graph intersections

  • STEP 3       Solve the appropriate equation(s) or inequality

    • For vertical line straight f left parenthesis x right parenthesis vertical line equals vertical line straight g left parenthesis x right parenthesis vertical line the two possible equations are straight f left parenthesis x right parenthesis equals straight g left parenthesis x right parenthesis and straight f left parenthesis x right parenthesis equals negative straight g left parenthesis x right parenthesis

2-8-5-modulus-functions---solving-equations-notes-diagram-32-8-5-modulus-functions---solving-equations-notes-diagram-3_1

Examiner Tips and Tricks

  • Sketching the graphs is important as solving algebraically can lead to invalid solutions.

  • For example, x = 1 is a solution to x minus 4 equals 2 x minus 5 but it is not a solution to vertical line x minus 4 vertical line equals 2 x minus 5 (substitute x = 1 into both sides and see why it does not work).

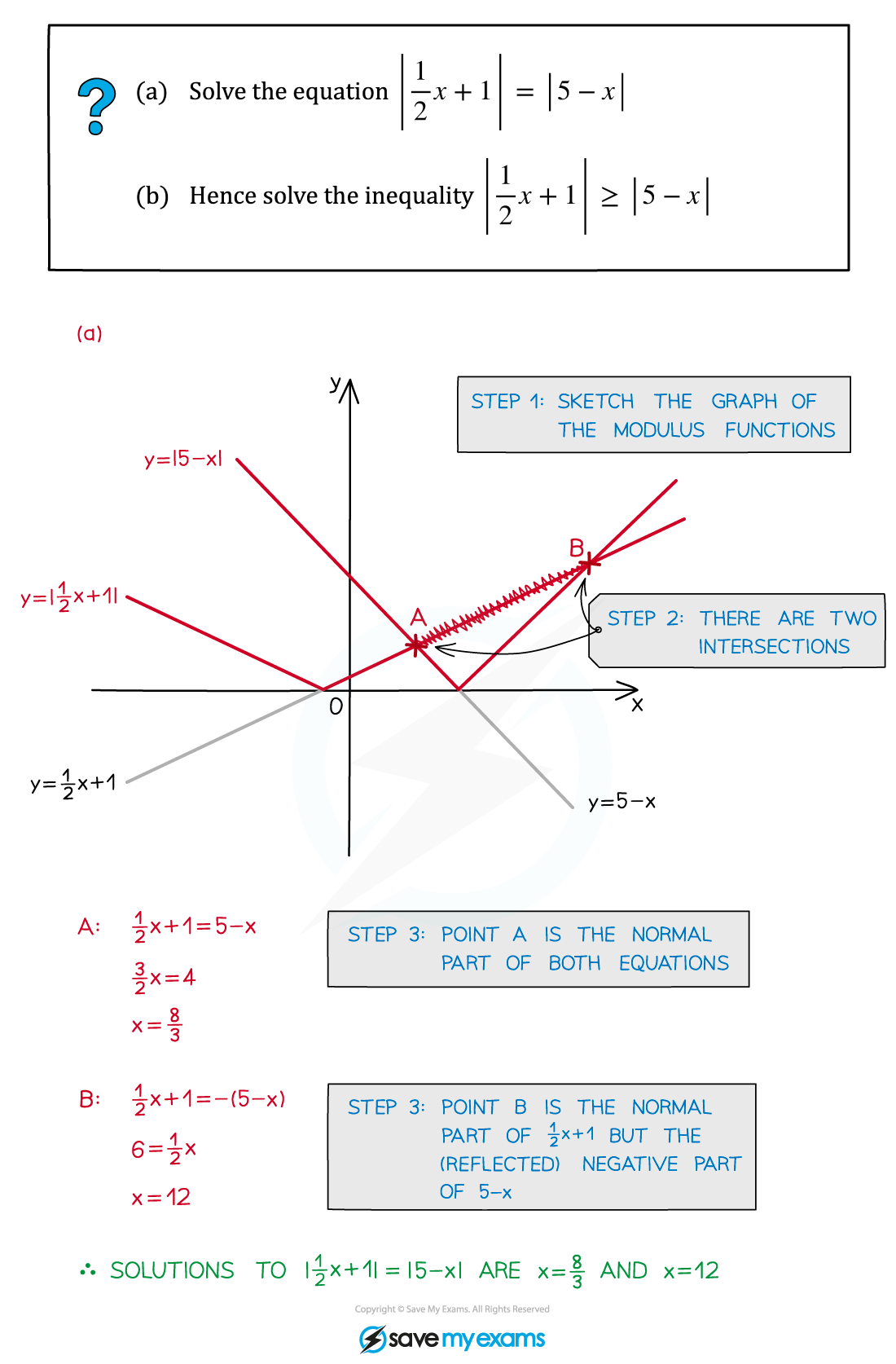
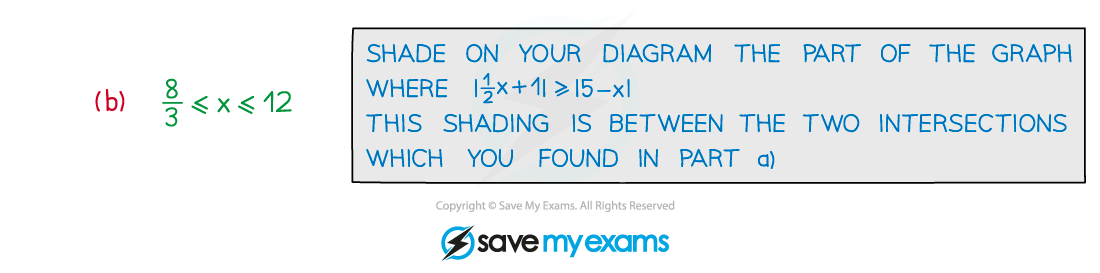
Worked Example

Modulus functions - Solving Equations Example Diagram 1, A Level & AS Level Pure Maths Revision Notes
Modulus functions - Solving Equations Example Diagram 2
👀 You've read 1 of your 5 free revision notes this week
An illustration of students holding their exam resultsUnlock more revision notes. It's free!

By signing up you agree to our Terms and Privacy Policy.

Already have an account? Log in

Test yourself

Did this page help you?

Previous:Sketching Graphs of Modulus FunctionsNext:Translations
Paul

Author: Paul

Expertise: Maths Content Creator

Paul has taught mathematics for 20 years and has been an examiner for Edexcel for over a decade. GCSE, A level, pure, mechanics, statistics, discrete – if it’s in a Maths exam, Paul will know about it. Paul is a passionate fan of clear and colourful notes with fascinating diagrams.

Download notes on Solving Equations with Modulus Functions