Further Parametric Equations (Edexcel International A Level (IAL) Maths: Pure 4): Exam Questions

Find an expression for in terms of for the parametric equations:

The graph defined by the parametric equations

is shown below.

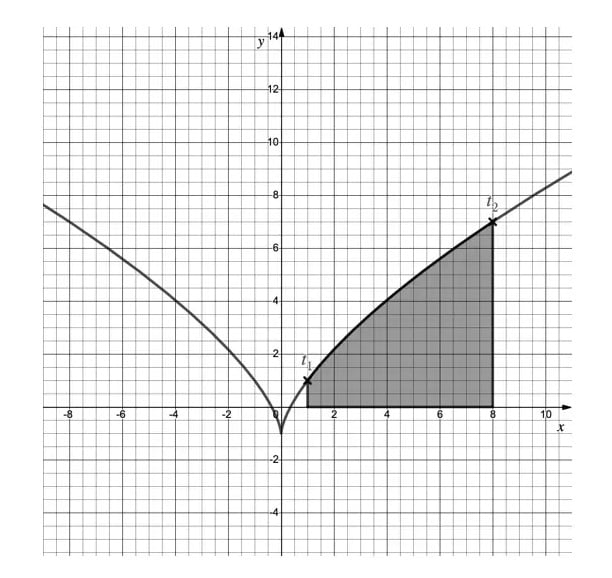
The point where has coordinates

The point where has coordinates

Find the values of and .

(i) Show that the shaded area can be found using the integral

(ii) Hence find the shaded area.

A crane swings a wrecking ball along a two-dimensional path defined by the parametric equations

as shown in the diagram below.

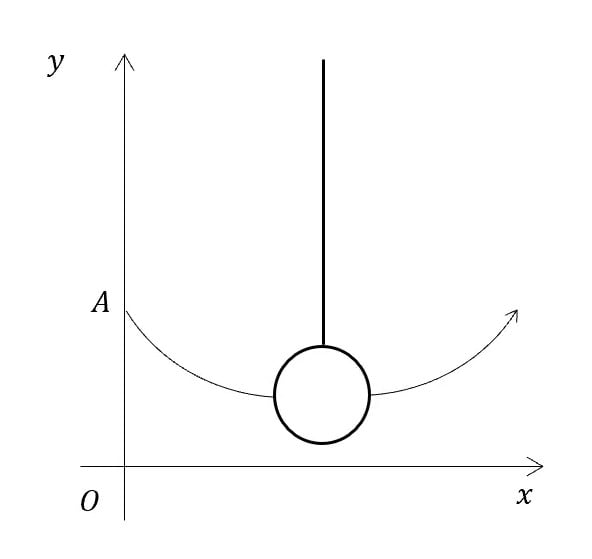
and are, respectively, the horizontal and vertical displacements in metres from the origin, , and is the time in seconds. Point indicates the initial position of the wrecking ball, at time .

Find the height of the wrecking ball after 0.3 seconds.

Find the minimum height of the wrecking ball during its motion.

Find the horizontal distances from point at the times when the wrecking ball is at a height of 2.9 m, giving your answers accurate to 1 decimal place.

The graph of the curve shown below is defined by the parametric equations

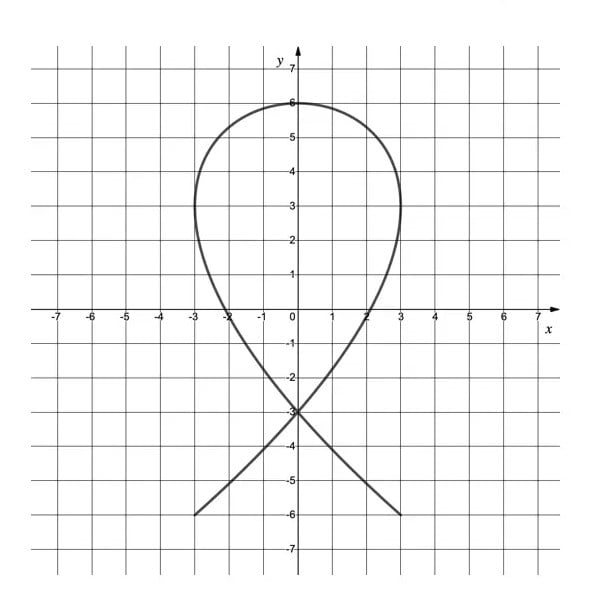
(i) Write down the value of at the point (0, 6) .

(ii) Write down the value of at the points (-3, 3) and (3, 3).

Find an expression for in terms of .

(i) Find the values of and at the point where .

(ii) Hence show the equation of the tangent to \( C \) at the point where is

The curve has parametric equations

Find an expression, in terms of , for .

(i) Find the gradient of the tangent to at the point .

(ii) Hence write down the gradient of the normal to at the point .

(iii) Find the equation of the normal to at the point .

The curve has parametric equations

Show that, in terms of ,

Show that the distance between the maximum and minimum points on C is

A company logo is in the shape of the symbol for infinity (∞) as shown on the graph below.

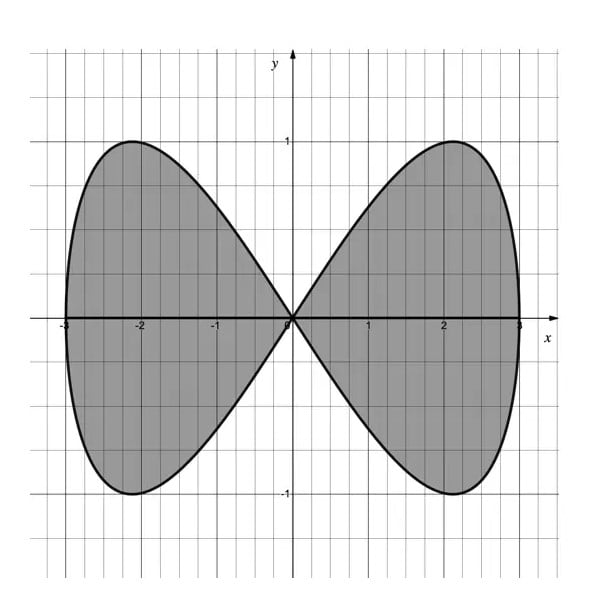
The company wishes to produce a sign of its logo and requires it to be painted, as indicated by the shading in the diagram.

The graph of the logo is defined by the parametric equations

where and are measured in metres.

(i) Show that when , , and that when , .

(ii) Find the coordinates of the point on the graph corresponding to .

(i) Using your results from part (a), along with the double angle formula show that the total area of the logo is given by

(ii) Hence find the total area of the logo that is to be painted.

A model car travels on a model track along the path of the curve shown in the diagram below. The curve is defined by the parametric equations

where and are, respectively, the horizontal and vertical displacements in metres from the origin , and is the time in seconds.

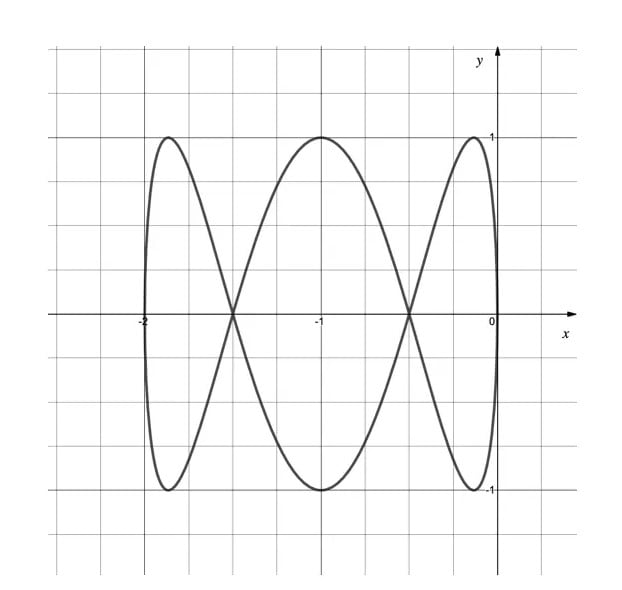
Verify that the starting position of the model car is at the origin, and find the position of the car at the times

(i) How many laps of the track does the model car complete?

(ii) Find the times at which the model car is at the point

The diagram below shows part of the curve with parametric equations

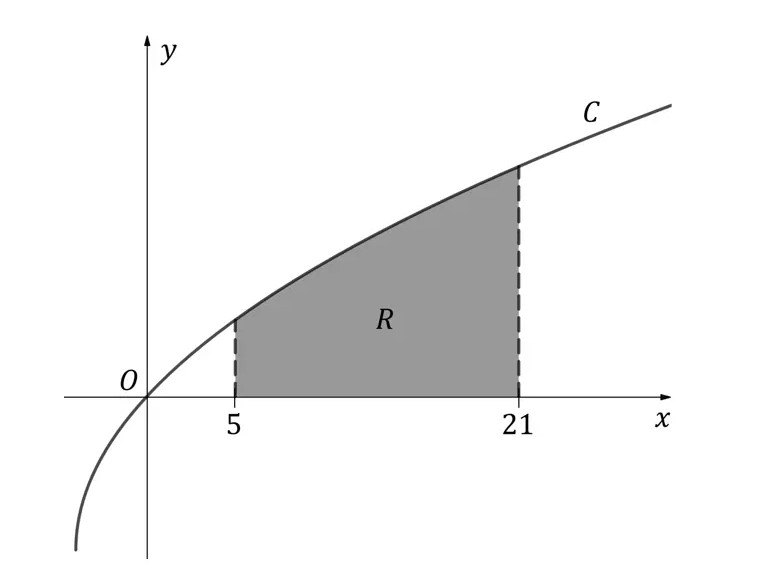
The point on the graph where has -coordinate 5.

The point on the graph where has -coordinate 21.

Determine the values of and , and find the coordinates of the corresponding points on .

The region shown in the diagram is bounded by , the -axis, and the lines and . Region is rotated through 360° about the -axis to form a solid of revolution.

(i) Show that the volume of the solid of revolution is given by the integral

(ii) Hence find the exact volume of the solid generated.

Find an expression for in terms of t for the parametric equations

Verify that the graph of against y passes through the point (0, 1) and find the gradient at that point.

The graph defined by the parametric equations

x=5t-1 y = t≥ 0

is shown below.

The point where t = t₁ has coordinates (-1,0).

The point where t = t₂ has coordinates (4, 1).

(i) Show that the shaded area can be found using the integral

(ii) Hence find the shaded area.

A crane swings a wrecking ball along a two-dimensional path defined by the parametric equations

x = 8t -4 y=16t²-16t + 5 0 ≤ t ≤ 1

as shown in the diagram below.

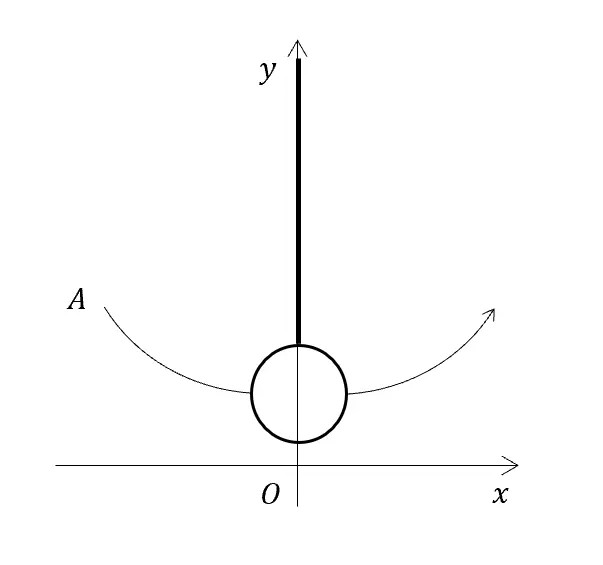
x and y are, respectively, the horizontal and vertical displacements in metres from the origin, 0, and t is the time in seconds. Point A indicates the initial position of the wrecking ball, at time t = 0.

Find a Cartesian equation of the curve in the form y = f(x), and state the domain of f(x).

Find the difference between the maximum and minimum heights of the wrecking ball during its motion.

The crane is positioned such that point A is 7 m horizontally from the wall the wrecking ball is to destroy.

Find the height at which the wrecking ball will strike the wall.

The graph of the curve C shown below is defined by the parametric equations

x = 2 cos 3 y = 5 sin 0 ≤ ≤ 2

Find an expression for in terms of .

(i) Show that the gradient of the tangent to C, at the point where , is .

(ii) Hence find the equation of the tangent to C at the point where .

The curve C has parametric equations

x = t>0

Find an expression, in terms of t, for

(i) Find the gradient of the tangent to C at the point where t =

(ii) Hence find the equation of the normal to C at the point where t =

The curve C has parametric equations

Show that at the point (0, 6), and find the value of at this point.

The tangent at the point (0, 6) is parallel to the normal at the point P.

Find the exact coordinates of point P

A curve C has parametric equations

The tangents to C at the points R and S meet at the point T, as shown in the diagram below.

Given that the x-coordinate of both points R and S is 5, find the area of the triangle RST.

A model car travels on a model track along the path of the curve shown in the diagram below. The curve is defined by the parametric equations

where and are, respectively, the horizontal and vertical displacements in metres from the origin , and is the time in seconds.

(i) Write down the coordinates of the starting position of the model car.

(ii) Indicate on the graph in which direction the model car travels.

(iii) How many laps of the track will the model car complete?

Find the times during the first lap at which the model car is at a “crossroads” – indicated by points and on the graph.

Find the speed of the model car at the start of the final lap.

The diagram below shows the curve with parametric equations

Find the coordinates of the points where intersects the -axis, and determine the corresponding values of .

The region shown in the diagram is bounded by and the -axis. Region is rotated through radians about the -axis to form a solid of revolution.

(i) Show that the volume of the solid of revolution is given by the integral

(ii) Hence find the exact volume of the solid generated.

The shaded area in the diagram below is bounded on three of its sides by the -axis, the -axis, and the line . On the remaining side, the boundary is defined by the parametric equations

Show that the shaded area is not a trapezium.

In your work, you may use without proof the result

A crane swings a wrecking ball along a two-dimensional path defined by the parametric equations

as shown in the diagram below.

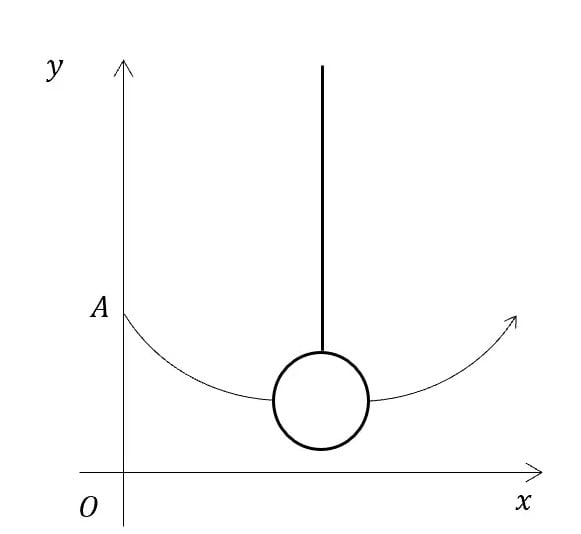
x and y are, respectively, the horizontal and vertical displacements in metres from the origin, O, and t is the time in seconds. Point A indicates the initial position of the wrecking ball.

(i) Write down the height of the wrecking ball when it is at point A.

(ii) Find the shortest distance between the wrecking ball and the ground during its motion.

The destruction of a building requires the wrecking ball to strike it at a height of 1.4 m whilst on the upward part of its path.
Find the horizontal distance from point A at which the ball hits the building.

The graph of the ellipse E shown below is defined by the parametric equations:

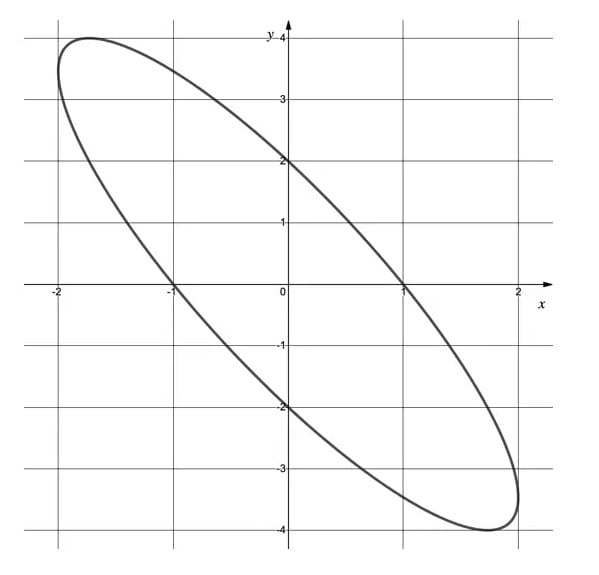
Find an expression for in terms of .

Find the equation of the tangent to E, at the point where , giving your answer in the form , where and are real numbers that should be given in exact form.