A LevelFurther MathsEdexcelCore PureRevision NotesFurther VectorsVector PlanesEquations of planes

Equations of planes (Edexcel A Level Further Maths): Revision Note

Exam code: 9FM0

Amber

Author

Amber

Last updated

Equation of a Plane in Vector Form

How do I find the vector equation of a plane?

  • A plane is a flat surface which is two-dimensional

    • Imagine a flat piece of paper that continues on forever in both directions

  • A plane in often denoted using the capital Greek letter Π

  • The vector form of the equation of a plane can be found using two direction vectors on the plane

    • The direction vectors must be

      • parallel to the plane

      • not parallel to each other

      • therefore they will intersect at some point on the plane

  • The formula for finding the vector equation of a plane is

    • bold italic r equals bold italic a plus s bold italic b plus t bold italic c

      • Where r is the position vector of any point on the plane

      • a is the position vector of a known point on the plane

      • b and c are two non-parallel direction (displacement) vectors parallel to the plane

      • s and t are scalars

  • The formula can also be written as

    • bold r equals bold a plus lambda left parenthesis bold b minus bold a right parenthesis plus mu left parenthesis bold c minus bold a right parenthesis equals left parenthesis 1 minus lambda minus mu right parenthesis bold a plus lambda bold b plus mu bold c

      • Where r is the position vector of any point on the plane

      • a, b, c are the position vectors of known points on the plane

      • λ and μ are scalars

    • These formulae are given in the formula booklet but you must make sure you know what each part means

  • As a could be the position vector of any point on the plane and b and c could be any non-parallel direction vectors on the plane there are infinite vector equations for a single plane

How do I determine whether a point lies on a plane?

  • Given the equation of a plane bold italic r blank equals blank open parentheses fraction numerator bold italic a subscript 1 over denominator table row cell bold italic a subscript 2 end cell row cell bold italic a subscript 3 end cell end table end fraction close parentheses plus lambda open parentheses fraction numerator bold italic b subscript 1 over denominator table row cell bold italic b subscript 2 end cell row cell bold italic b subscript 3 end cell end table end fraction close parentheses plus blank mu open parentheses fraction numerator bold italic c subscript 1 over denominator table row cell bold italic c subscript 2 end cell row cell bold italic c subscript 3 end cell end table end fraction close parentheses then the point r with position vector blank open parentheses fraction numerator x over denominator table row y row z end table end fraction close parentheses is on the plane if there exists a value of λ and μ such that

    • open parentheses fraction numerator x over denominator table row y row z end table end fraction close parentheses blank equals blank open parentheses fraction numerator bold italic a subscript 1 over denominator table row cell bold italic a subscript 2 end cell row cell bold italic a subscript 3 end cell end table end fraction close parentheses plus lambda open parentheses fraction numerator bold italic b subscript 1 over denominator table row cell bold italic b subscript 2 end cell row cell bold italic b subscript 3 end cell end table end fraction close parentheses plus blank mu open parentheses fraction numerator bold italic c subscript 1 over denominator table row cell bold italic c subscript 2 end cell row cell bold italic c subscript 3 end cell end table end fraction close parentheses

    • This means that there exists a single value of λ and μ that satisfy the three parametric equations:

      • x equals blank a subscript 1 plus lambda b subscript 1 plus blank mu c subscript 1 blank

      • y equals blank a subscript 2 plus lambda b subscript 2 plus blank mu c subscript 2 blank

      • z equals blank a subscript 3 plus lambda b subscript 3 blank plus blank mu c subscript 2

  • Solve two of the equations first to find the values of λ and μ that satisfy the first two equation and then check that this value also satisfies the third equation

  • If the values of λ and μ do not satisfy all three equations, then the point r does not lie on the plane

Examiner Tips and Tricks

  • The formula for the vector equation of a plane is given in the formula booklet, make sure you know what each part means

  • Be careful to use different letters, e.g. lambda and mu as the scalar multiples of the two direction vectors

Worked Example

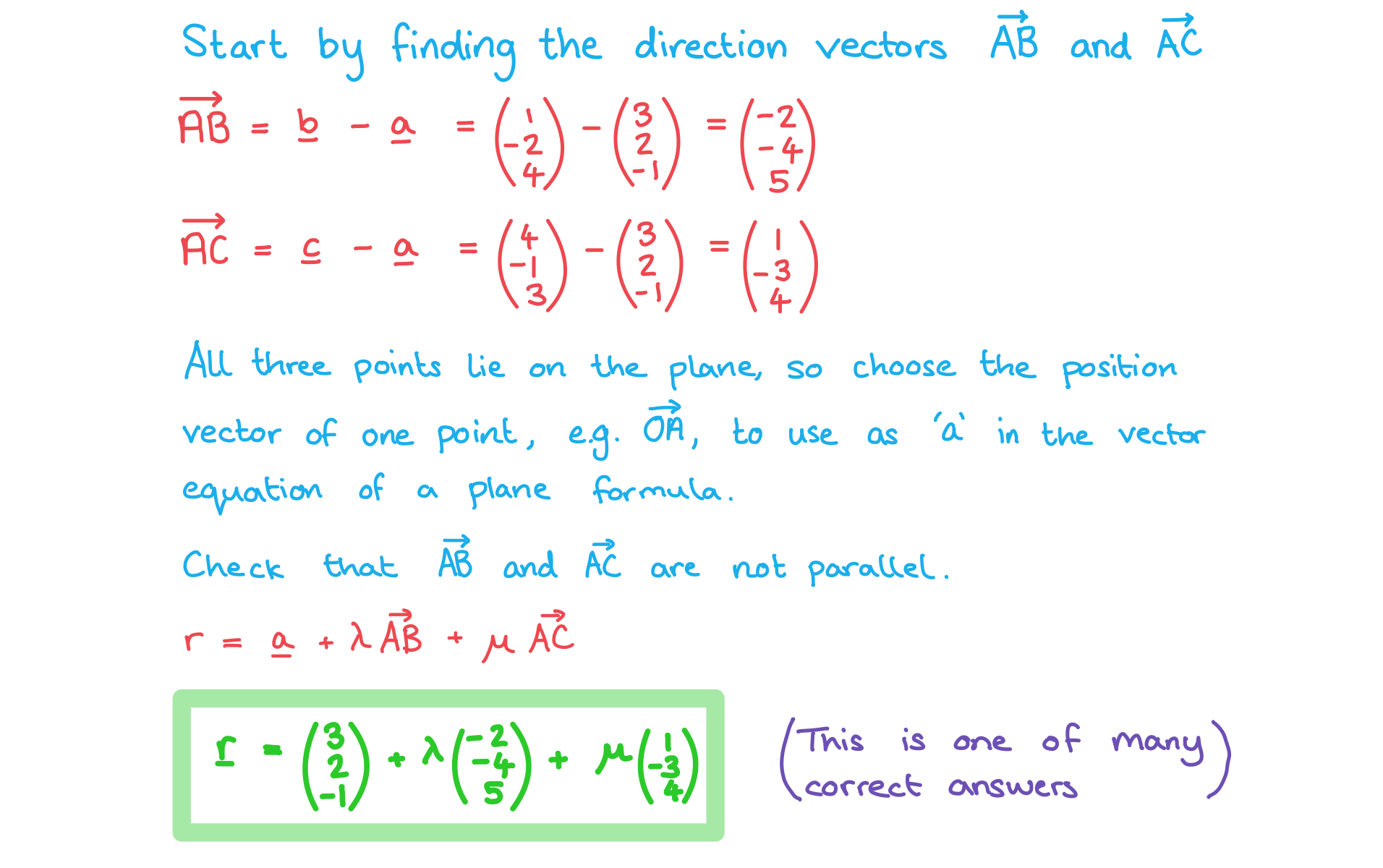
The points A, B and C have position vectors bold italic a equals 3 bold i plus 2 bold j minus bold k, bold italic b equals bold i minus 2 bold j plus 4 bold k, and bold italic c equals 4 bold i minus bold j plus 3 bold k respectively, relative to the origin O.

(a) Find the vector equation of the plane.

3-11-1-ib-aa-hl-vector-plane-vector-form-we-solution-a

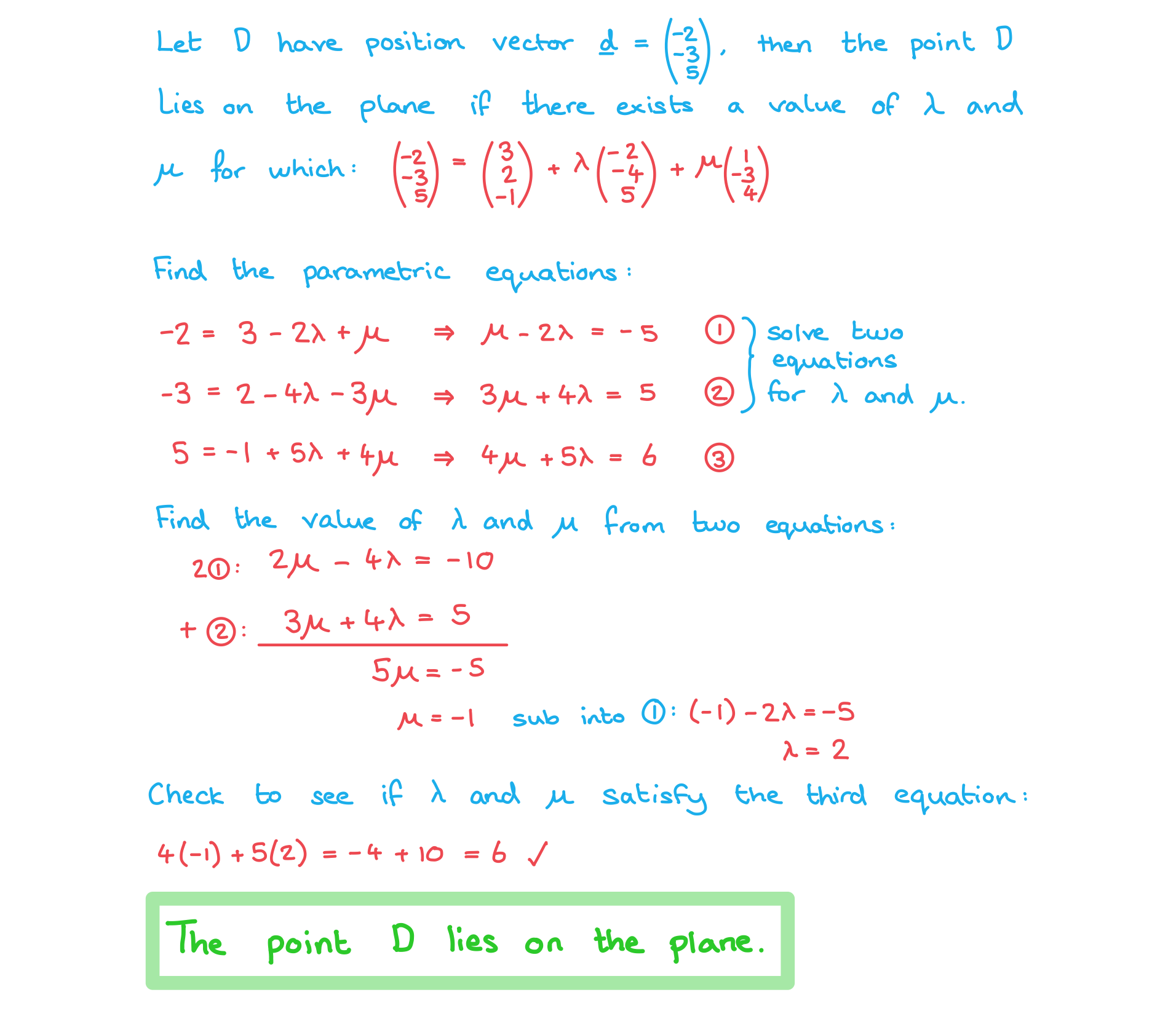
(b) Determine whether the point D with coordinates (-2, -3, 5) lies on the plane.

3-11-1-ib-aa-hl-vector-plane-vector-form-we-solution-b

Equation of a Plane in Cartesian Form

How do I find the vector equation of a plane in cartesian form?

  • The cartesian equation of a plane is given in the form

    • a x plus b y plus c z equals d

    • This is given in the formula booklet

  • A normal vector to the plane can be used along with a known point on the plane to find the cartesian equation of the plane

    • The normal vector will be a vector that is perpendicular to the plane

  • The scalar product of the normal vector and any direction vector on the plane will be zero

    • The two vectors will be perpendicular to each other

    • The direction vector from a fixed-point A to any point on the plane, R can be written as r – a

    • Then n ∙ (r – a) = 0 and it follows that (n ∙ r) – (n ∙ a) = 0

  • This gives the equation of a plane using the normal vector:

    • n ∙ r = a ∙ n

      • Where r is the position vector of any point on the plane

      • a is the position vector of a known point on the plane

      • n is a vector that is normal to the plane

    • This is given in the formula booklet

  • If the vector r is given in the form open parentheses table row x row y row z end table close parentheses and a and n are both known vectors given in the form open parentheses table row cell a subscript 1 end cell row cell a subscript 2 end cell row cell a subscript 3 end cell end table close parentheses and open parentheses table row cell n subscript 1 end cell row cell n subscript 2 end cell row cell n subscript 3 end cell end table close parentheses then the Cartesian equation of the plane can be found using:

    • bold italic n times bold italic r equals n subscript 1 x plus n subscript 2 y plus n subscript 3 z

    • bold italic a times bold italic n equals a subscript 1 n subscript 1 plus a subscript 2 n subscript 2 plus a subscript 3 n subscript 3

    • Therefore n subscript 1 x plus n subscript 2 y plus n subscript 3 z equals a subscript 1 n subscript 1 plus a subscript 2 n subscript 2 plus a subscript 3 n subscript 3

    • This simplifies to the form a x plus b y plus c z equals d

      • A version of this is given in the formula booklet

How do I find the equation of a plane in Cartesian form given the vector form?

  • Given the equation of the plane bold r equals bold a plus lambda bold b plus mu bold c

    • Form three equations

      • x equals a subscript 1 plus lambda b subscript 1 plus mu c subscript 1

      • y equals a subscript 2 plus lambda b subscript 2 plus mu c subscript 2

      • z equals a subscript 3 plus lambda b subscript 3 plus mu c subscript 3

  • Choose a pair of equations and use them to form an equation without μ

  • Choose another pair and form another equation without μ

  • Use your two expressions to form an equation without μ and λ

  • Rewrite the equation in the form a x plus b y plus c z plus d equals 0

Examiner Tips and Tricks

  • In an exam, using whichever form of the equation of the plane to write down a normal vector to the plane is always a good starting point

Worked Example

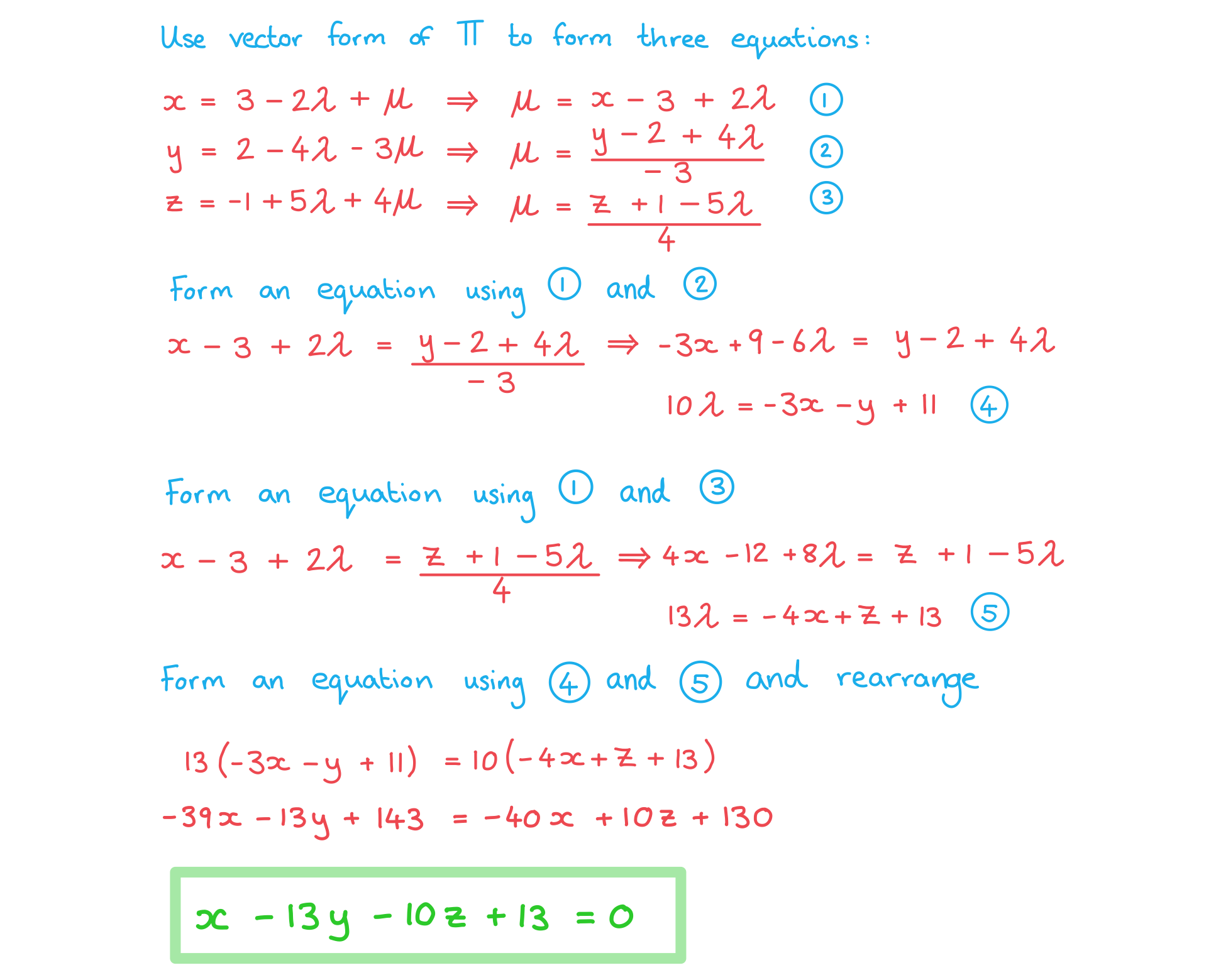
A plane straight capital pi has equation bold r equals open parentheses table row 3 row 2 row cell negative 1 end cell end table close parentheses plus lambda open parentheses table row cell negative 2 end cell row cell negative 4 end cell row 5 end table close parentheses plus mu open parentheses table row 1 row cell negative 3 end cell row 4 end table close parentheses. Find the equation of the plane in its Cartesian form.

al-fm-6-2-1-equation-of-plane-in-cartesian-form-1
👀 You've read 1 of your 5 free revision notes this week
An illustration of students holding their exam resultsUnlock more revision notes. It's free!

By signing up you agree to our Terms and Privacy Policy.

Already have an account? Log in

Test yourself

Did this page help you?

Previous:Shortest Distances - LinesNext:Combinations of Lines & Planes
Amber

Author: Amber

Expertise: Maths Content Creator

Amber gained a first class degree in Mathematics & Meteorology from the University of Reading before training to become a teacher. She is passionate about teaching, having spent 8 years teaching GCSE and A Level Mathematics both in the UK and internationally. Amber loves creating bright and informative resources to help students reach their potential.

Download notes on Equations of planes