Circle Theorems (OCR GCSE Maths: Higher): Exam Questions

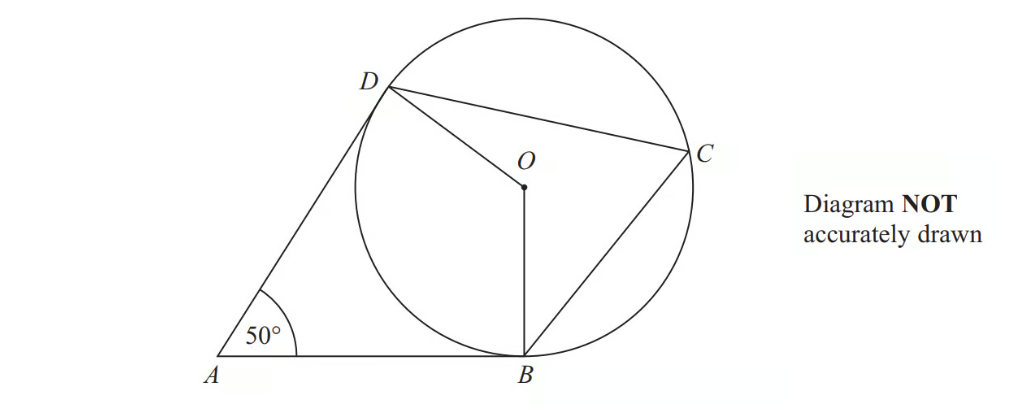
and  are points on the circumference of a circle, centre . and  are tangents to the circle.

Angle 
Angle 

Work out the size of angle .

and  are points on the circumference of a circle, centre .
 is a diameter of the circle.
and  intersect at E.

Angle 
Angle 

Work out the size of angle .
You must show all your working.

and  are points on a circle, centre .
is a tangent to the circle.

Angle 

Work out the size of angle .
Give reasons for each stage of your working.

and  are points on the circumference of a circle, centre .  is a straight line.
is the tangent at  to the circle.
Angle 

Work out the size of angle .
Give a reason for each stage of your working.

and  are points on a circle, centre .

 is a tangent to the circle.
 is a straight line.
Angle .

Find the size of angle , in terms of .
Give your answer in its simplest form.
Give reasons for each stage of your working.

and  are diameters of a circle, centre .

Prove that triangle  and triangle  are congruent.

and  are four points on the circumference of a circle.

and  are straight lines.
Prove that triangle  and triangle  are similar.
You must give reasons for each stage of your working.

A and B are points on the circumference of a circle, centre O. CA and CB are tangents to the circle.

Prove that triangle OAC is congruent to triangle OBC.

[4]

A, B, C and D are points on the circumference of a circle, centre O. AC is a diameter of the circle. Angle ABD = 58°. Angle CDB = 22°.

Work out the sizes of angle ACD and ACB, giving reasons for your answers.

Angle ACD = ...........................°

[2]

Angle ACB = ...........................°

[3]

In the diagram,

• A, B and C are points on the circumference of a circle

• O is the centre of the circle

• angle OAB = 15°

• angle BCO = 10°.

Calculate the acute angle AOC.

[4]

and are points on a circle.

is the tangent at to the circle.
Angle
Angle

Work out the size of angle .
Give reasons for your working.

[4]

and  are points on a circle.

and are straight lines.

Prove that  is not a diameter of the circle.

[4]

and are points on a circle.

• bisects angle .

• is a tangent to the circle.

Angle

Prove that

[3]

and  are points on a circle.
and  are straight lines.

Prove that triangle  is similar to triangle .
You must give reasons for your working.

and  are points on the circumference of a circle, centre .
is a tangent to the circle.

Show that
You must give a reason for each stage of your working.

Dylan was asked to give some possible values for  and .

He said, " could be 200 and  could be 110, because 200 − 110 = 90"

Is Dylan correct?
You must give a reason for your answer.

and  are points on the circumference of a circle centre .

Prove that angle  is twice the size of angle .

A, B and C are points on the circumference of a circle, centre O.
AOB is a diameter of the circle.

Prove that angle ACB is 90°
You must not use any circle theorems in your proof.

 is a regular pentagon.
and  are points on a circle, centre .
and  are tangents to the circle.
is a straight line.

Prove that .

A, B, C and D are points on the circumference of a circle, centre O.

Angle BAD = 112° and angle DCO = 33°.

Show that angle y = 35°. Give reasons for each stage of your working.

[4]

Work out angle z.

Give reasons for your answer.

Angle z = ......................... ° because ........................................................................................

[3]

A, B, C and D are points on the circumference of a circle, centre O.

Angle CAD = 28° and CD = 6.4cm. BD is a diameter of the circle.

Calculate the area of the circle.

...................... cm² [5]

and  are points on a circle.  is a tangent to the circle.

Angle 

Prove that angle  Give reasons for each stage in your working.

[5]

and  are points on a circle.

is the tangent at  to the circle. is parallel to .

Work out the size of angle . Give a reason for each stage of your working.

[5]

and  are points on a circle. , , and are straight lines. Work out the size of angle . Show your working clearly.

= ...................... [4]

and are points on a circle with centre .

Angle

The area of the shaded segment is 200cm²

Calculate the radius of the circle.
Give your answer correct to 3 significant figures.

...................................................... cm [5]

and are three points on the circumference of a circle, centre .

and are tangents to the circle. is a kite. Angle is

Prove that angle is 

[4]

and are points on a circle.

The tangent at is shown.
and are straight lines.

Prove that   

[4]

and are points on a circle.

is a tangent.

Assume that triangle is isosceles with

Prove that is parallel to .

[4]

In fact, triangle is equilateral.

Tick the two boxes for the statements that must be correct. 

[1]

Here is a cyclic quadrilateral.

Work out the size of angle .

.....................degrees

[4]

and are points on a circle.

and are straight lines.

Work out the size of angle.

You must show your working which may be on the diagram.

..........................degrees [4]