A LevelMathsCambridge (CIE)Probability & Statistics 1Revision NotesStatistical DistributionsWorking with DistributionsNormal Approximation of a Binomial Distribution

Normal Approximation of a Binomial Distribution (Cambridge (CIE) A Level Maths): Revision Note

Amber

Author

Amber

Last updated

Did this video help you?

Normal Approximation of Binomial

When can I use a normal distribution to approximate a binomial distribution?

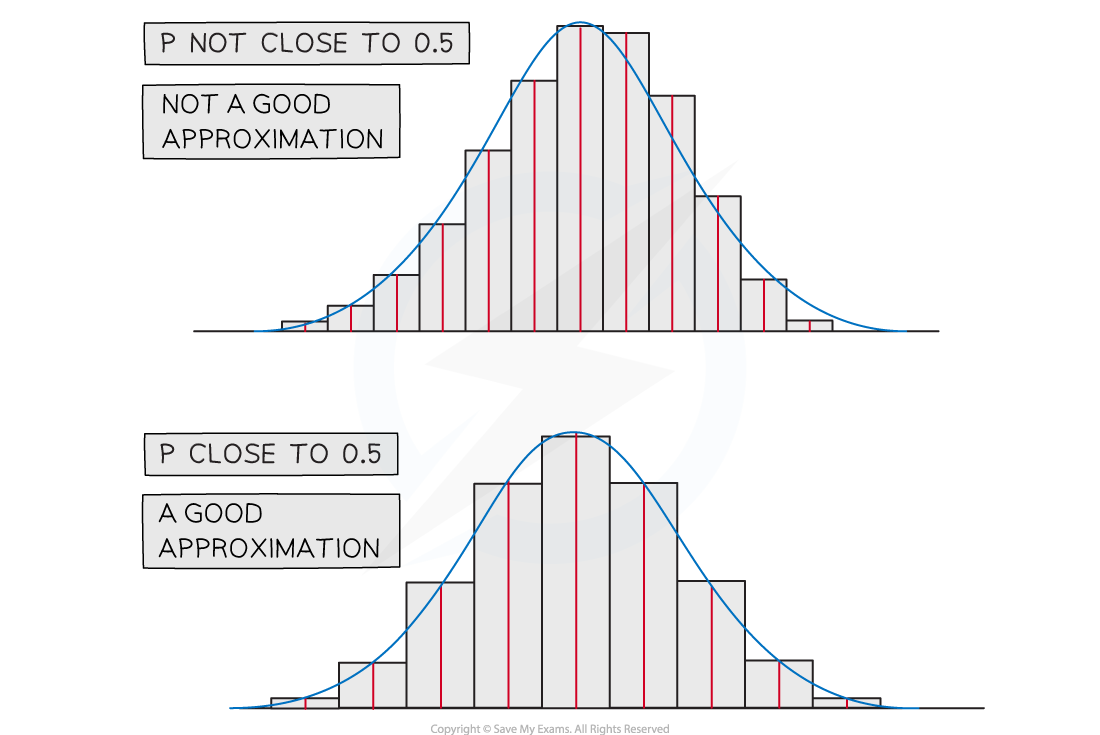
  • A binomial distribution begin mathsize 16px style X tilde straight B left parenthesis n comma p right parenthesis end style can be approximated by a normal distribution X subscript N tilde straight N left parenthesis mu comma sigma squared right parenthesis  provided

    • n is large

    • p is close to 0.5

      • bold italic n bold italic p bold greater than bold 5

      • bold italic n bold italic q bold greater than bold 5 bold space bold italic w bold italic h bold italic e bold italic r bold italic e bold space bold italic q bold equals bold 1 bold minus bold italic p

  • The mean and variance of a binomial distribution can be calculated by:

    • mu equals n p

    • sigma squared equals n p left parenthesis 1 minus p right parenthesis

4-4-2-normal-approximation-of-binomial-diagram-1

Why do we use approximations?

  • If there are a large number of values for a binomial distribution there could be a lot of calculations involved and it is inefficient to work with the binomial distribution

    • These days calculators can calculate binomial probabilities so approximations are no longer necessary

    • However it is easier to work with a normal distribution

      • You can calculate the probability of a range of values quickly

      • You can use the inverse normal distribution function (most calculators don't have an inverse binomial distribution function)

  • In your exam you must use the formula and not a calculator to find binomial probabilities so you are limited to small values of n

What are continuity corrections?

  • The binomial distribution is discrete and the normal distribution is continuous

  • A continuity correction takes this into account when using a normal approximation

  • The probability being found will need to be changed from a discrete variable, X,   to a continuous variable, XN

    • For example, X = 4 for binomial can be thought of as 3.5 less or equal than X subscript N less than 4.5 for normal as every number within this interval rounds to 4

    • Remember that for a normal distribution the probability of a single value is zero so straight P left parenthesis 3.5 less or equal than X subscript N less than 4.5 right parenthesis equals straight P left parenthesis 3.5 less than X subscript N less than 4.5 right parenthesis

How do I apply continuity corrections?

  • Think about what is largest/smallest integer that can be included in the inequality for the discrete distribution and then find its upper/lower bound

  • P left parenthesis X equals k right parenthesis almost equal to P left parenthesis k space minus 0.5 less than X subscript N less than k plus 0.5 right parenthesis

  • P left parenthesis X less or equal than k right parenthesis almost equal to P left parenthesis X subscript N less than k plus 0.5 right parenthesis

    • You add 0.5 as you want to include k in the inequality

  • P left parenthesis X less than k right parenthesis almost equal to P left parenthesis X subscript N less than k minus 0.5 right parenthesis

    • You subtract 0.5 as you don't want to include k in the inequality

  • P left parenthesis X greater or equal than k right parenthesis almost equal to P left parenthesis X subscript N greater than k minus 0.5 right parenthesis

    • You subtract 0.5 as you want to include k in the inequality

  • P left parenthesis X greater than k right parenthesis almost equal to P left parenthesis X subscript N greater than k plus 0.5 right parenthesis

    • You add 0.5 as you don't want to include k  in the inequality

  • For a closed inequality such as straight P left parenthesis a less than X less or equal than b right parenthesis

    • Think about each inequality separately and use above

    • P left parenthesis X greater than a right parenthesis almost equal to P left parenthesis X subscript N greater than a plus 0.5 right parenthesis

    • P left parenthesis X less or equal than b right parenthesis almost equal to P left parenthesis X subscript N less than b plus 0.5 right parenthesis

    • Combine to give

    • straight P left parenthesis a plus 0.5 less than X subscript N less than b plus 0.5 right parenthesis

How do I approximate a probability?

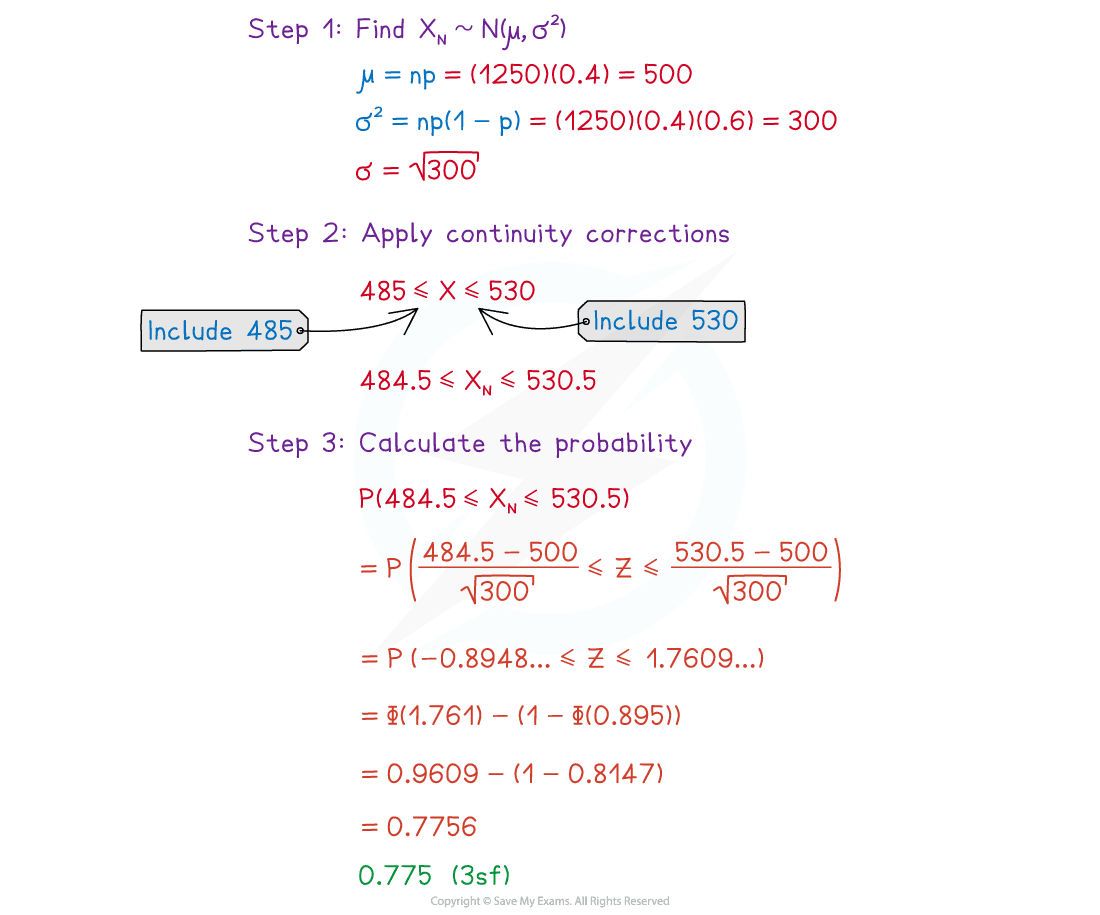
  • STEP 1: Find the mean and variance of the approximating distribution

    • mu equals n p

    • begin mathsize 16px style sigma squared equals n p left parenthesis 1 minus p right parenthesis end style

  • STEP 2: Apply continuity corrections to the inequality

  • STEP 3: Find the probability of the new corrected inequality

    • Find the standard normal probability and use the table of the normal distribution

  • The probability will not be exact as it is an approximate but provided n is large and p is close to 0.5 then it will be a close approximation

    • To decide if n is large enough and if p is close enough to 0.5 check that:

      • n p greater than 5 

      • n p greater than 5 where q equals 1 minus p

Worked Example

The random variable X tilde B left parenthesis 1250 comma space 0.4 right parenthesis.

Use a suitable approximating distribution to approximate straight P left parenthesis 485 less or equal than space X space less or equal than space 530 right parenthesis.

3-4-2-normal-approximation-of-binomial-we-solution-with-addition

Examiner Tips and Tricks

  • In the exam, the question will often tell you to use a normal approximation but sometimes you will have to recognise that you should do so for yourself. Look for the conditions mentioned in this revision note, n is large, p is close to 0.5, np > 5 and nq > 5.

You've read 1 of your 5 free revision notes this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

  • I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

    Beth
    IGCSE Student

  • This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

    Fathima
    A Level Student
Test yourself

Did this page help you?

Previous:Modelling with Distributions
Amber

Author: Amber

Expertise: Maths Content Creator

Amber gained a first class degree in Mathematics & Meteorology from the University of Reading before training to become a teacher. She is passionate about teaching, having spent 8 years teaching GCSE and A Level Mathematics both in the UK and internationally. Amber loves creating bright and informative resources to help students reach their potential.

Download notes on Normal Approximation of a Binomial Distribution