Parametric Differentiation (Cambridge (CIE) A Level Maths) : Revision Note

Parametric Differentiation

How do I find dy/dx from parametric equations? 

• Ensure you are familiar with Parametric Equations – Basics first

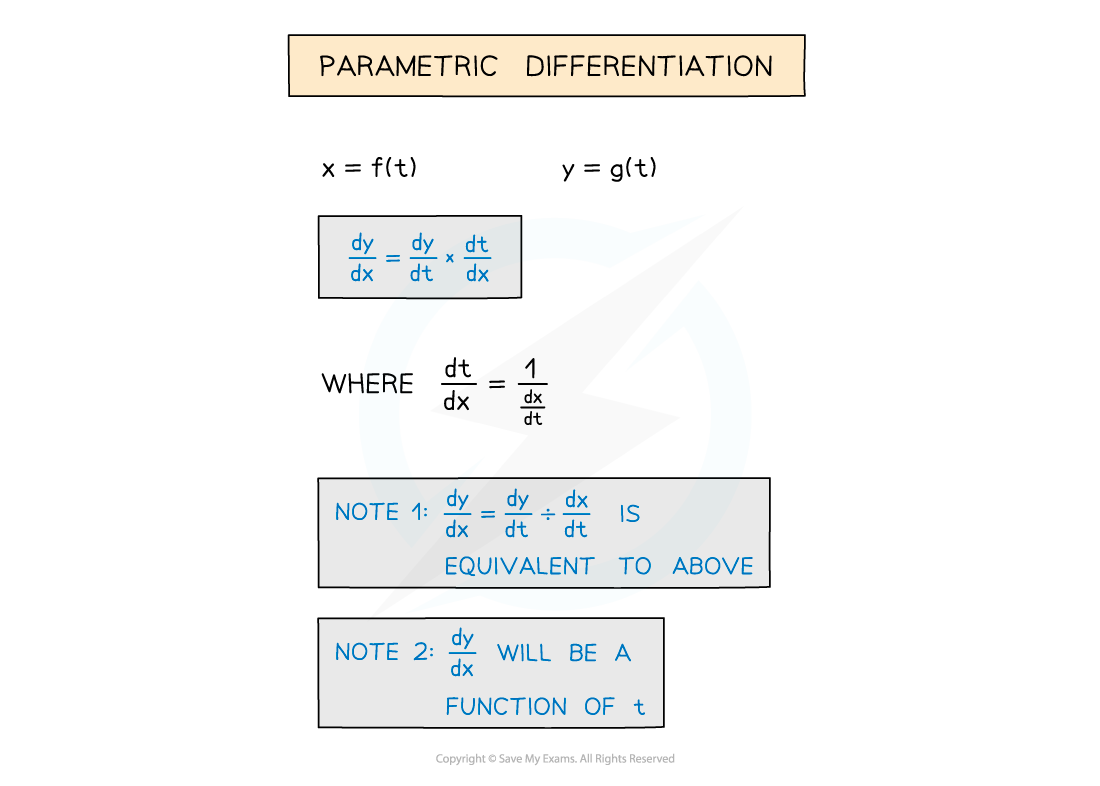
• This method uses the chain rule and the reciprocal property of derivatives

  • 

  • 

• Equivalently,

• will be in terms of t – this is fine

  • Questions usually involve finding gradients, tangents and normals

• The chain rule is always needed when there are three variables or more – see Connected Rates of Change

How do I find gradients, tangents and normals from parametric equations? 

• To find a gradient …

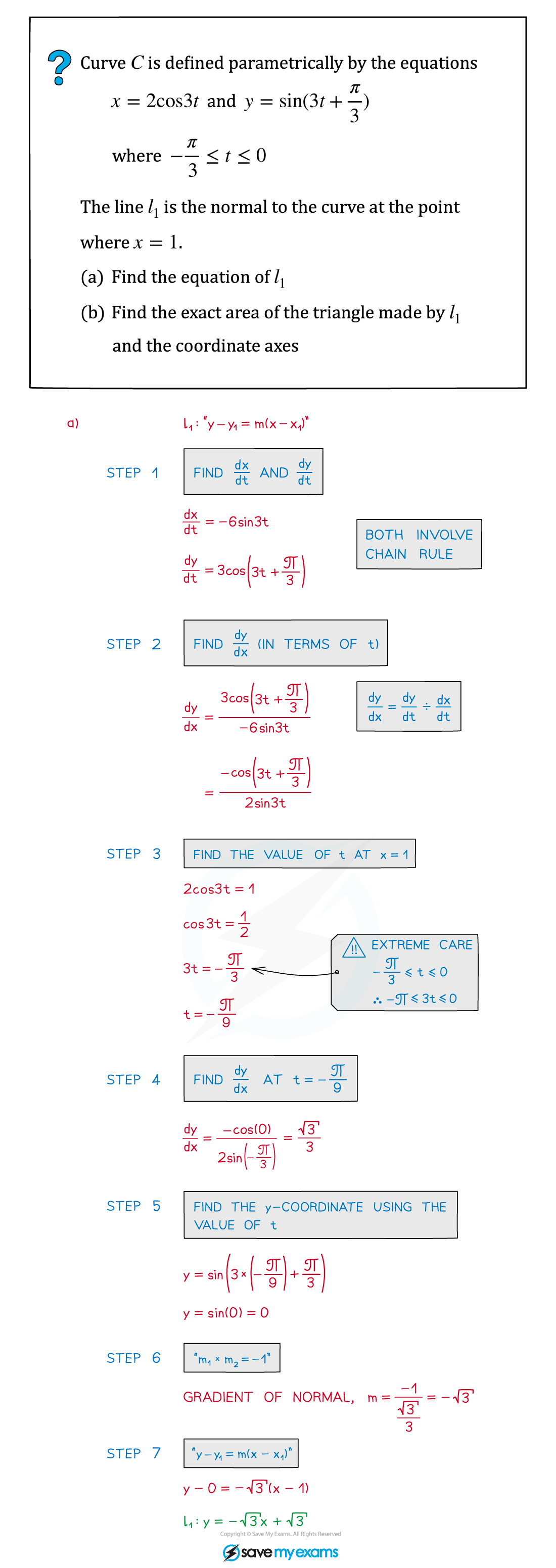
  • STEP 1: Find dx/dt and dy/dt

  • STEP 2: Find dy/dx in terms of t

  Using either dy/dx = dy/dt ÷ dx/dt

  or dy/dx = dy/dt × dt/dx where dt/dx = 1 ÷ dx/dt

  • STEP 3: Find the value of t at the required point

  • STEP 4: Substitute this value of t into dy/dx to find the gradient 

    

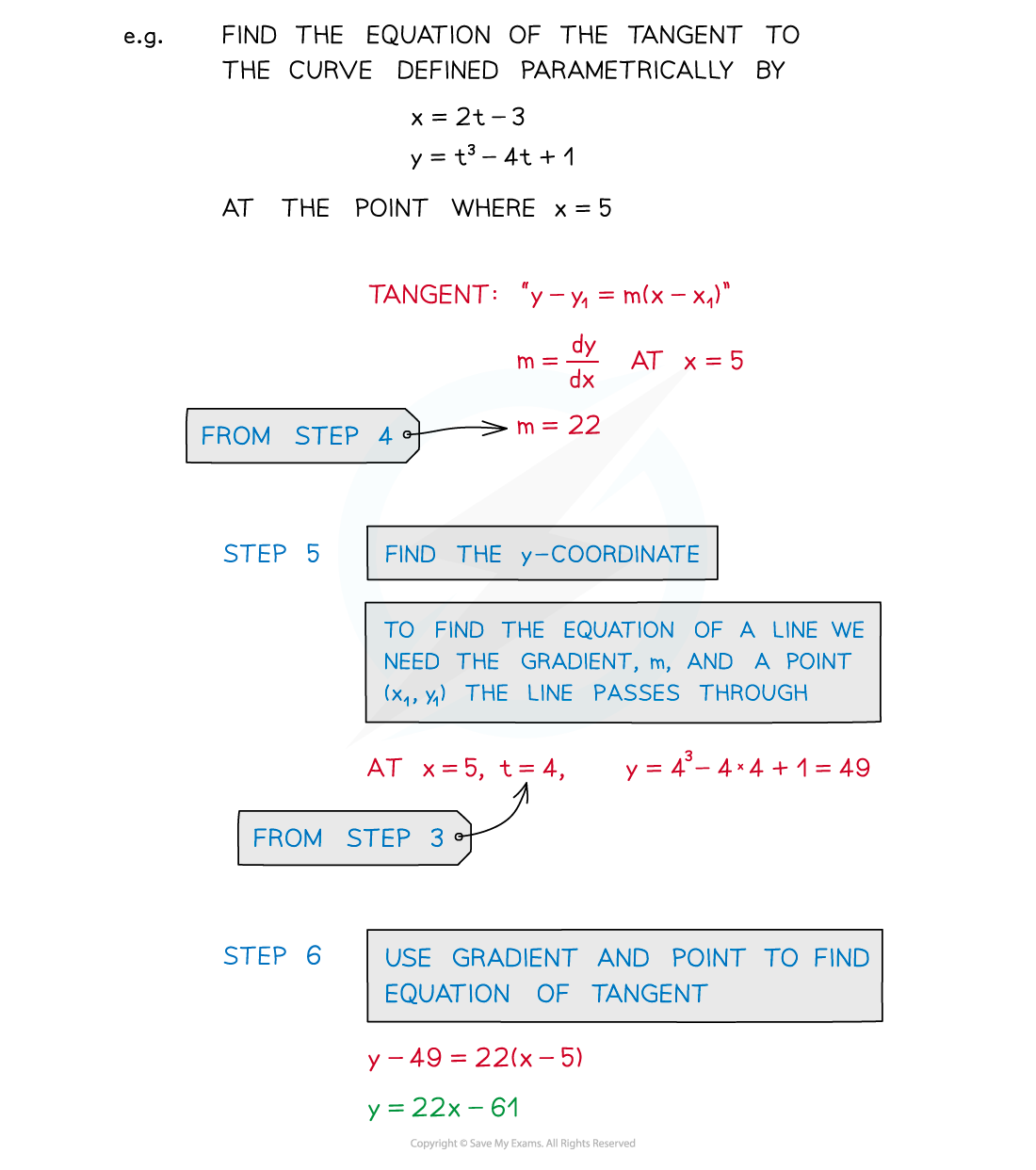
• to then go on to find the equation of a tangent …

  • STEP 5: Find the y coordinate

  • STEP 6: Use the gradient and point to find the equation of the tangent

• To find a normal...

  • STEP 7: Use perpendicular lines property to find the gradient of the normal m₁ × m₂ = -1

  • STEP 8: Use gradient and point to find the equation of the normal y - y₁ = m(x - x₁)

What else may I be asked to do?

• Questions may require use of tangents and normals as per the coordiante geometry sections

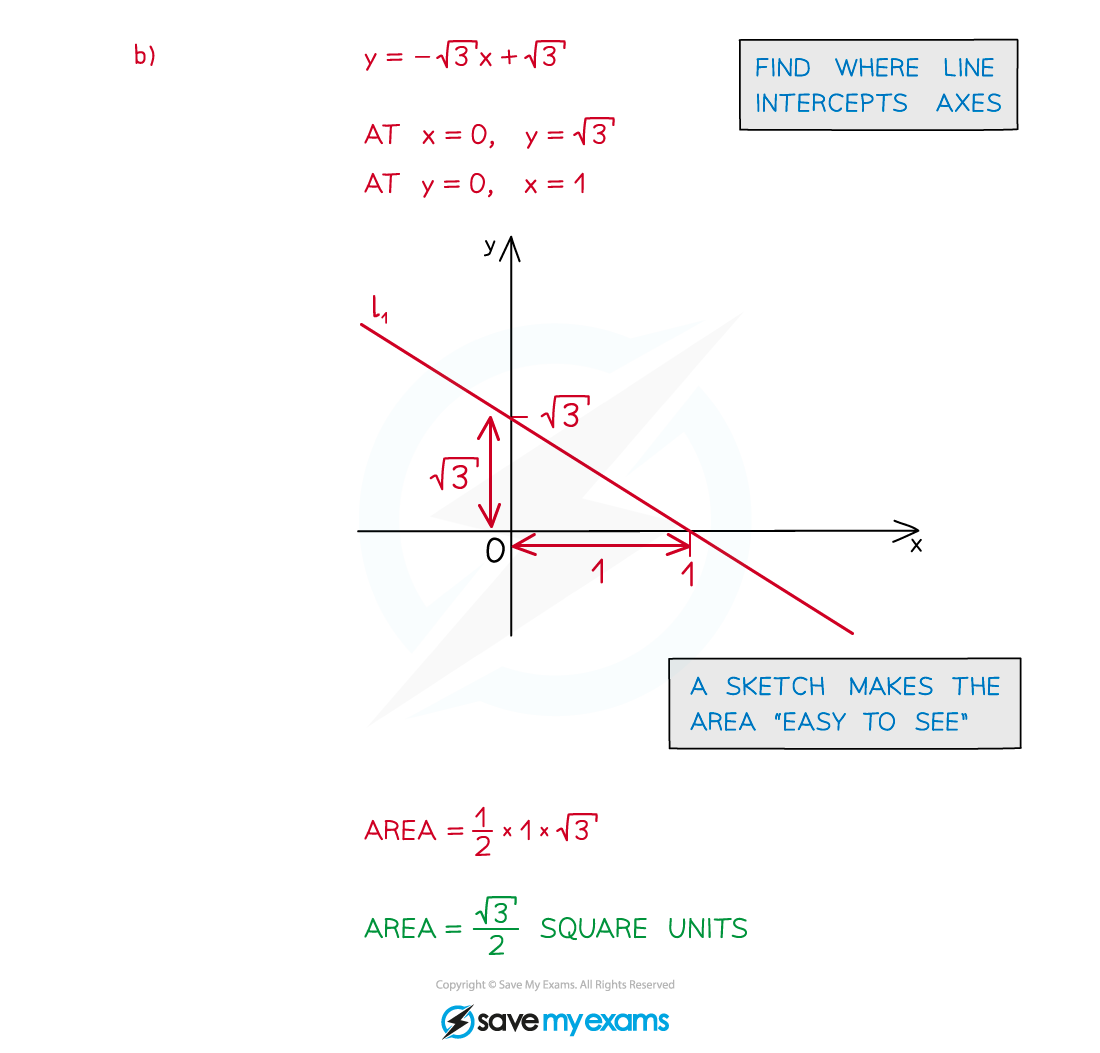
  • Find points of intersection between a tangent/normal and x/y axes

  • Find areas of basic shapes enclosed by axes and/or tangents/normal

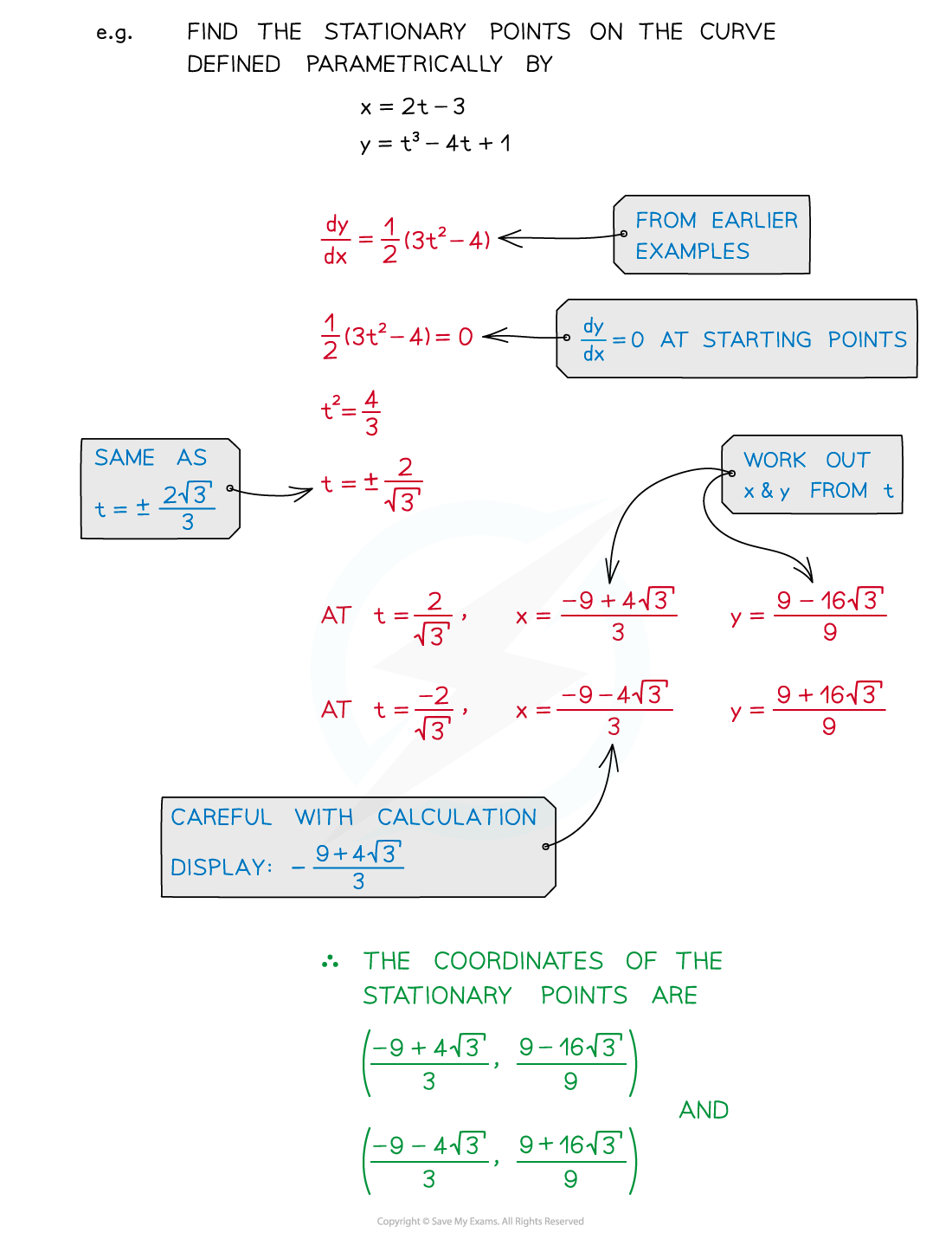
• Find stationary points (dy/dx = 0)

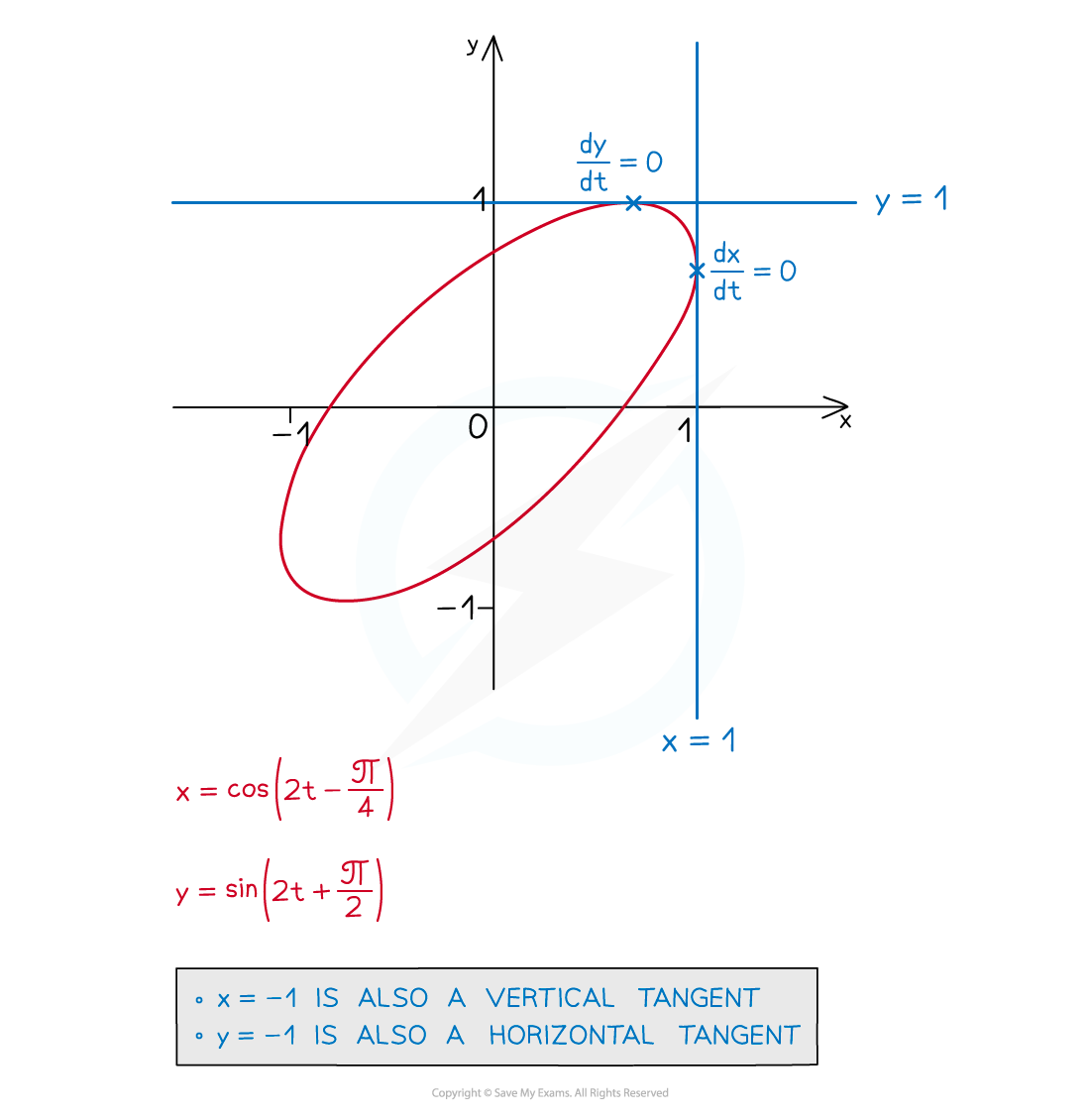
• You may also be asked about horizontal and vertical tangents

  • At horizontal (parallel to the x-axis) tangents, dy/dt = 0

  • At vertical (parallel to y-axis) tangents, dx/dt = 0

Just for fun …

• Try plotting the graph from the question below using graphing software

• Plenty of free online tools do this – for example Desmos and Geogebra

• Try changing the domain of t to -π/3 ≤ t ≤ π/3