Types of Graphs (Edexcel GCSE Maths)

Types of Graphs

What graphs do I need to know?

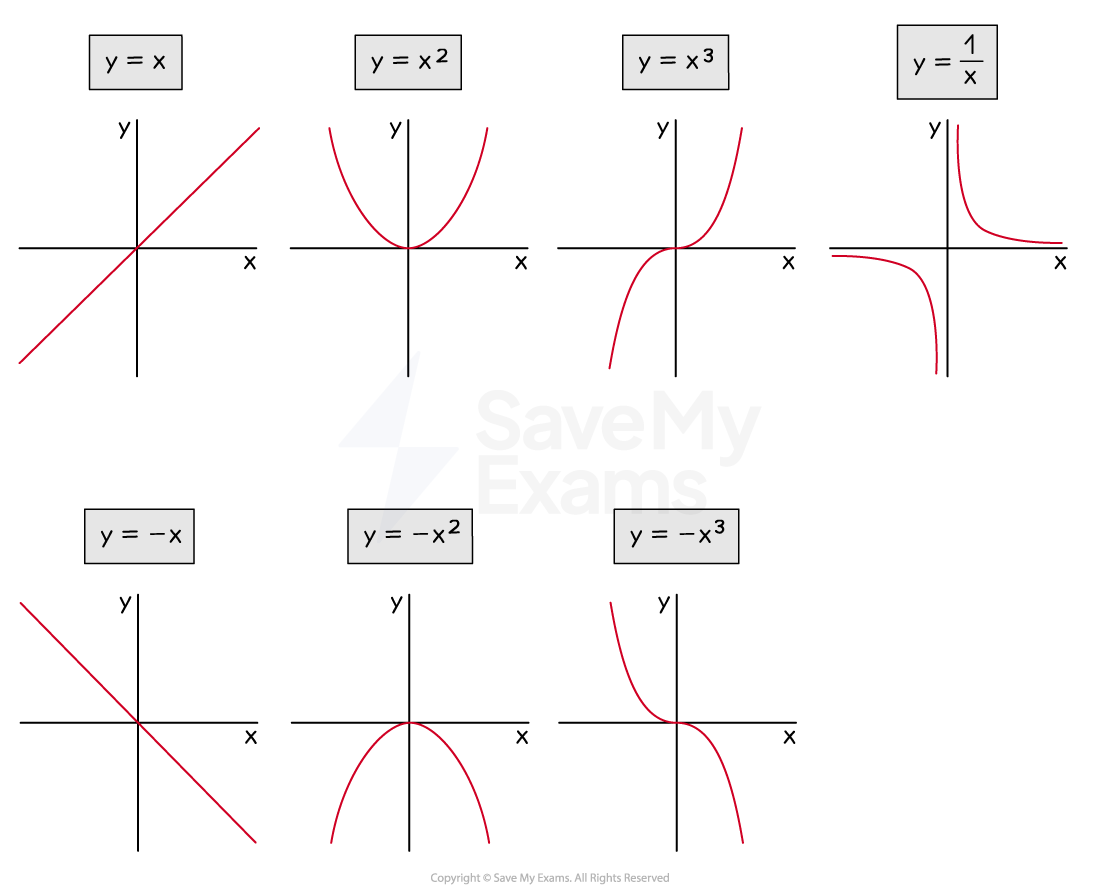
• You need to be able to recognise the following lines:

  •  Straight lines

    • y  = mx  + c

    • Such as y  = 3x  + 2, y  = 5x  - 1, ...

    • Two important ones are y =  x  and y =  -x

  • Horizontal lines

    • y  = c

    • Such as y  = 4, y  = -10, ...

  • Vertical lines

    • x  = k

    • Such as x  = 2, x  = -1, ...

• You need to be able to recognise quadratic graphs

  • y  = x²

  • y  = -x²

  • y  = ax²  + bx  + c

• You need to be able to recognise simple cubic graphs

  • y  = x³

  • y  = -x³

  • y  = ax³  + bx² + x + c

• You also need to be able to recognise reciprocal graphs

  • , where 

What does a quadratic graph look like?

• The equation of a quadratic graph is y  = ax²  + bx  + c

• A quadratic graph has either a u-shape or an n-shape

  • This type of shape is called a parabola 

• u-shapes are called positive quadratics

  • because the number in front of x² is positive

    • For example, y  = 2x²  + 3x  + 4

• n-shapes are called negative quadratics

  • because the number in front of x² is negative

    • For example, y  = -3x²  + 2x  + 4

• You can plot quadratic graphs using a table of values
   

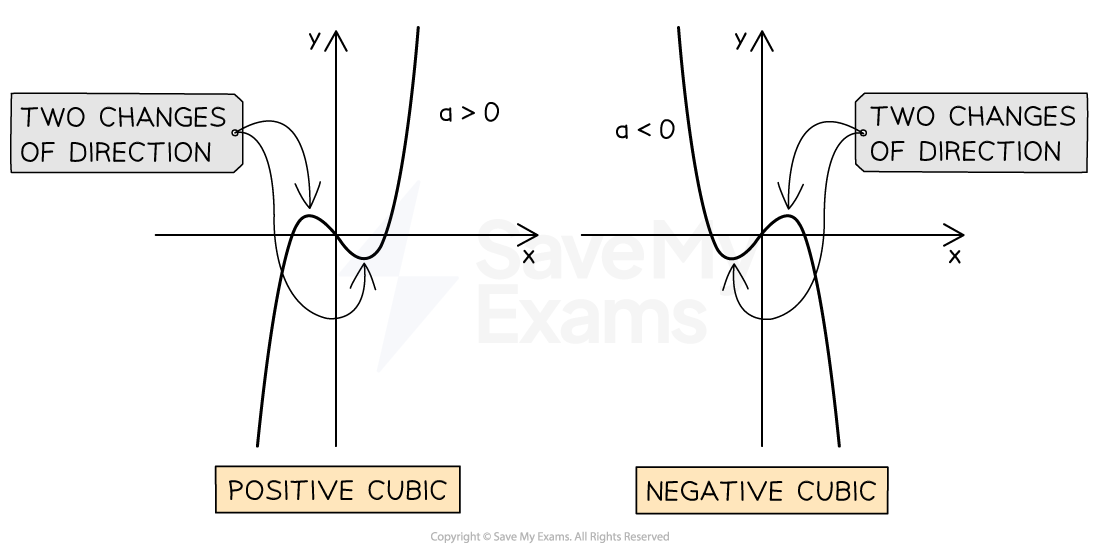
What does a cubic graph look like?

• The equation of a cubic graph is y  = ax³ + bx² + cx + d

• A cubic graph can have two points where it changes direction (turning points)

• A positive cubic goes uphill (from the bottom left to the top right)  

  • The number in front of x³ is positive

    • For example, y  = x³ - 3x² + 2x  + 1

• A negative cubic goes downhill (from the top left to the bottom right) 
  

  • The number in front of x³ is negative

    • For example, y  = -x³  + 2x² - x + 5

• You can plot cubic graphs using a table of values

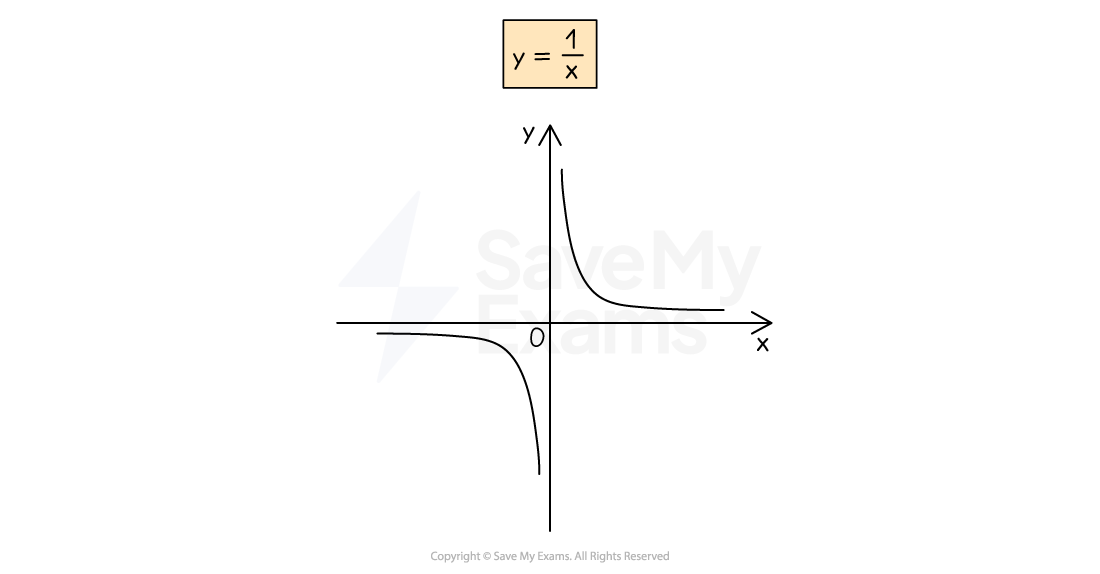
What does a reciprocal graph look like?

• The equation of the basic reciprocal graph is 

  • You cannot substitute in x  = 0 (division by zero is not allowed) 

    • 

  • You should not include x  = 0 in a table of values

• The shape of is shown below

  • It has two two curved branches

    • The branches are L-shaped

  • The branches never connect!