Types of Sequences (Edexcel GCSE Maths): Revision Note
Exam code: 1MA1
Did this video help you?
Types of sequences
What types of sequences are there?
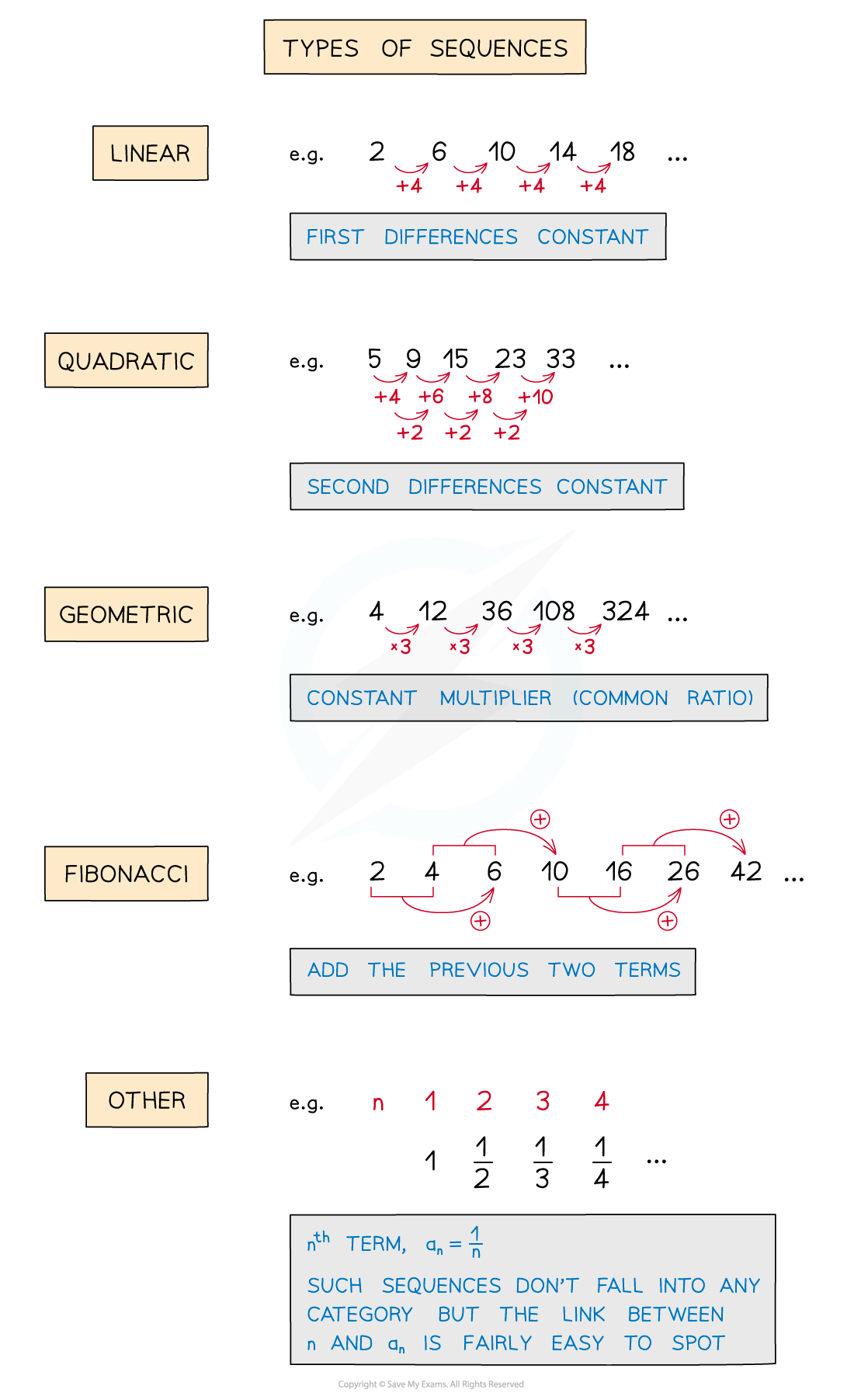
Linear sequences are the sequences that you are most likely to see in an exam question
These are sometimes called arithmetic sequences or progressions
A common difference is added to or subtracted from one term to get to the next term
Other types of sequences that you may also come across include
Quadratic sequences (square numbers)
Cube numbers
Triangular numbers
Geometric sequences
Fibonacci sequences
Another common type of sequence in exam questions, is fractions with combinations of the above
Look for anything that makes the position-to-term and/or the term-to-term rule easy to spot
What is a quadratic sequence?
A quadratic sequence is based around square numbers
The second differences are constant (the same)
Second differences are the differences between the first differences
For example, 2, 3, 6, 11, 18, …
1st Differences: 1 3 5 72nd Differences: 2 2 2
What is a geometric sequence?
A geometric sequence can also be referred to as a geometric progression and sometimes as an exponential sequence
In a geometric sequence, the term-to-term rule would be to multiply by a constant
This multiplier is called the common ratio and can be found by dividing any two consecutive terms
Consider the sequence 4, 8, 16, 32, 64, ...
The common ratio would be x2 (8 ÷ 4 or 16 ÷ 8 or 32 ÷ 16 and so on)
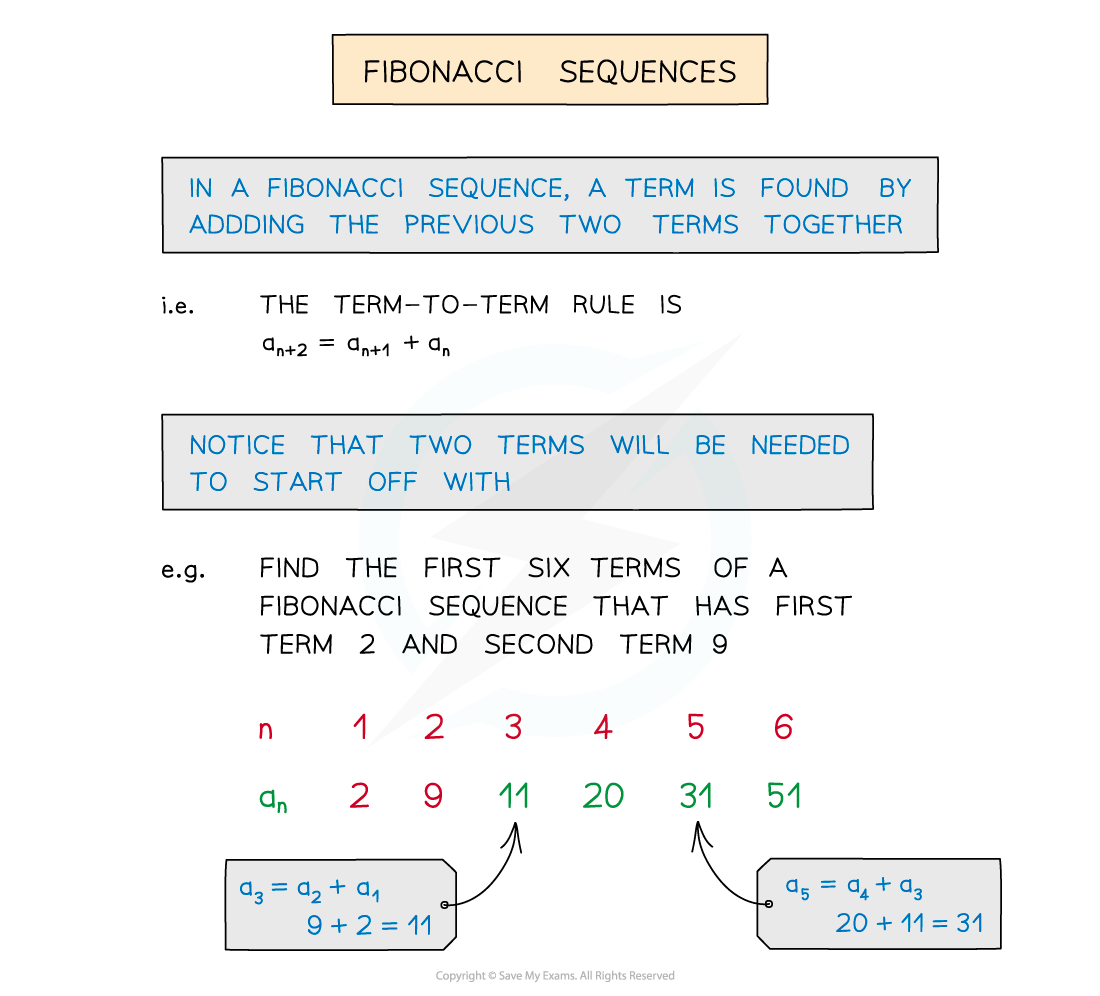
What is a Fibonacci sequence?
THE Fibonacci sequence is 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, ...
The sequence starts with the first two terms as 1
Each subsequent term is the sum of the previous two
Notice that two terms are needed to start a Fibonacci sequence
Any sequence that has the term-to-term rule of adding the previous two terms is called a Fibonacci sequence but the first two terms will not both be 1
Fibonacci sequences occur a lot in nature such as the number of petals of flowers
Problem solving with sequences
When the type of sequence is known it is possible to find unknown terms within the sequence
Possibly simultaneous equationsThis can lead to problems involving setting up and solving equations
Other problems may involve sequences that are related to common number sequences such as square numbers, cube numbers and triangular numbers
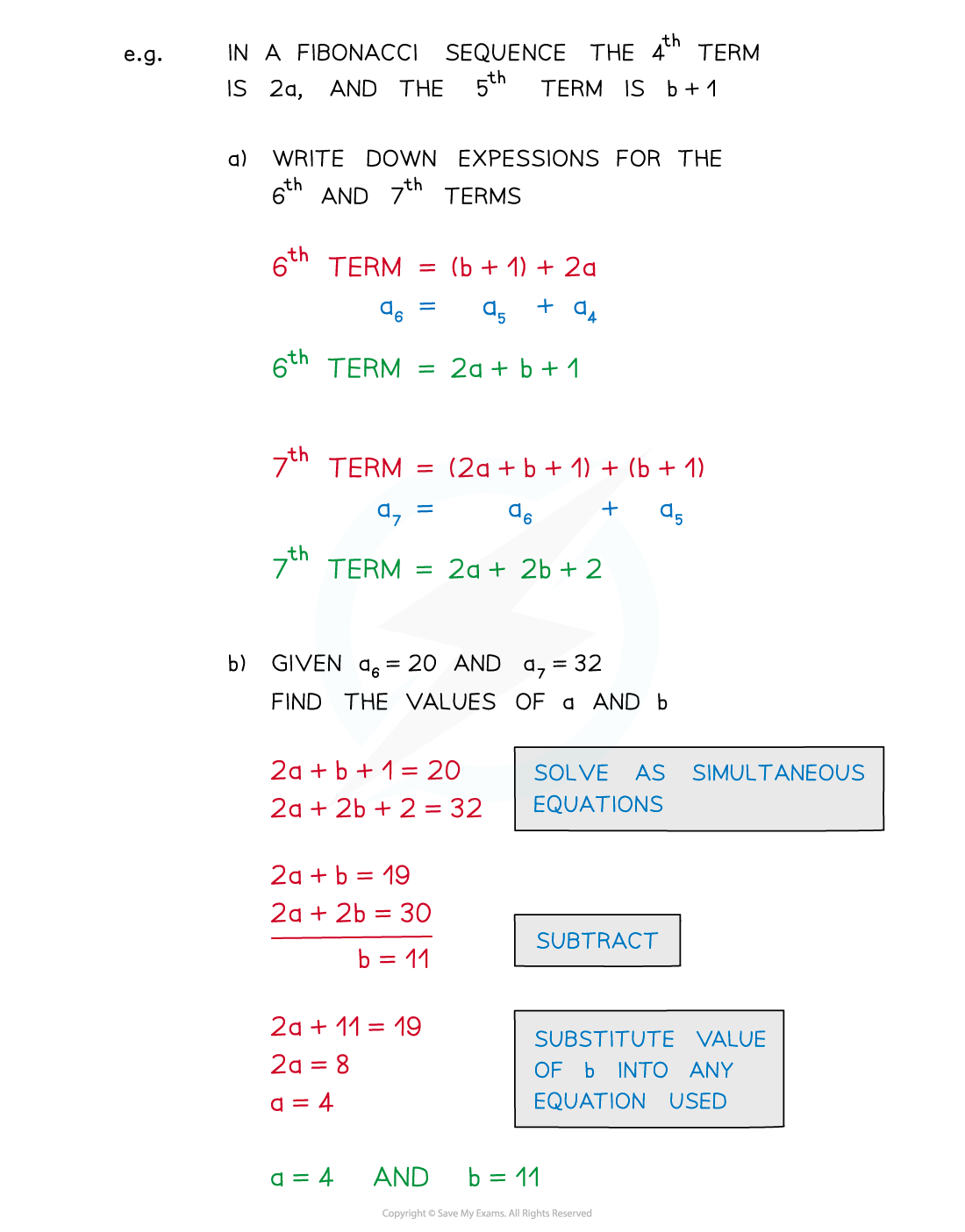
Worked Example
(a)
Identify the types of sequence below;
i) 4, 5, 9, 14, 23, 37, 60, ...
There is no common second difference so it is not quadratic
There is no common ratio so it is not geometric
Two terms add together to give the next term in the sequence
4 + 5 = 9
5 + 9 = 14 etc.
Fibonacci sequence
ii) 6, 10, 16, 24, 34, ...
First differences are not equal so it is not linear
Second differences are equal
6, 10, 16, 24, 34, ...
4 6 8 10
2 2 2
Quadratic sequence
iii) 12, 7, 2, -3, ...
There is a common first difference
12, 7, 2, -3, ...
-5 -5 -5
Linear sequence
(b) The 3rd and 6th terms in a Fibonacci sequence are 7 and 31 respectively.
Find the 1st and 2nd terms of the sequence.
Write down the terms of the sequence that you know in their correct position
n | 1 | 2 | 3 | 4 | 5 | 6 |
an |
|
| 7 |
|
| 31 |
We're told that this is a Fibonacci sequence, so two consecutive terms added together give the next term
Let the second term, a2 be x
We can write an expression for the 4th term by adding together the second and 3rd terms
We can write an expression for the 5th term by adding together the 3rd and 4th terms
n | 1 | 2 | 3 | 4 | 5 | 6 |
an |
| x | 7 | x + 7 | (x + 7) + 7 | 31 |
We know that the 6th term in the sequence is 31
Add together the expressions for the 4th and the 5th term and set it equal to 31
(x + 7) + (x + 7) + 7 = 31
Simplify
2x + 21 = 31
Solve for x
2x = 10
x = 5
Substitute the value for x into the terms of the sequence that you have expressions for
n | 1 | 2 | 3 | 4 | 5 | 6 |
an |
| 5 | 7 | 12 | 19 | 31 |
Find the 1st term in the sequence by subtracting the 2nd term from the 3rd term
7 - 5 = 2
First term = 2
Second term = 5

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?