Conversion Graphs

What is a conversion graph?

A conversion graph is a straight-line graph relating two quantities
- You can convert (change) between them by reading values off the graph
Common examples include
- Temperature
  - degrees Celsius (°C) and degrees Fahrenheit (°F)
- Currency
  - Dollars ($) and Yen (¥)
- Volume
  - Litres and gallons
- Prices
  - A taxi driver charging per kilometre driven
The gradient of a conversion graph represents the rate of change
- If the y-axis is the cost of a taxi journey (£) and the x-axis is the distance travelled (mile) then the gradient represents the cost per mile
  - A gradient of 5 means the cost increases by £5 for each mile travelled

How do I use a conversion graph?

Find the cost of 20kg using the conversion graph below
- Start at 20kg on the x-axis
- Draw a vertical line to the graph
- Then a horizontal line across to the y-axis
- Read off the value
  - $12
Find how many kilograms can be bought with $30
- Start at $30 on the y-axis
- Draw a horizontal line to the graph
- Then a verical line down to the x-axis
- Read off the value
  - 50kg
You can use proportion to find values that on not on the axes
- To find the cost of 120kg
  - 120kg = 6 × 20kg costs 6 × $12 = $72
  - 120kg = 50kg + 50kg + 20kg costs $30 + $30 + $12 = $72
- You can only do this if the graph starts at the origin

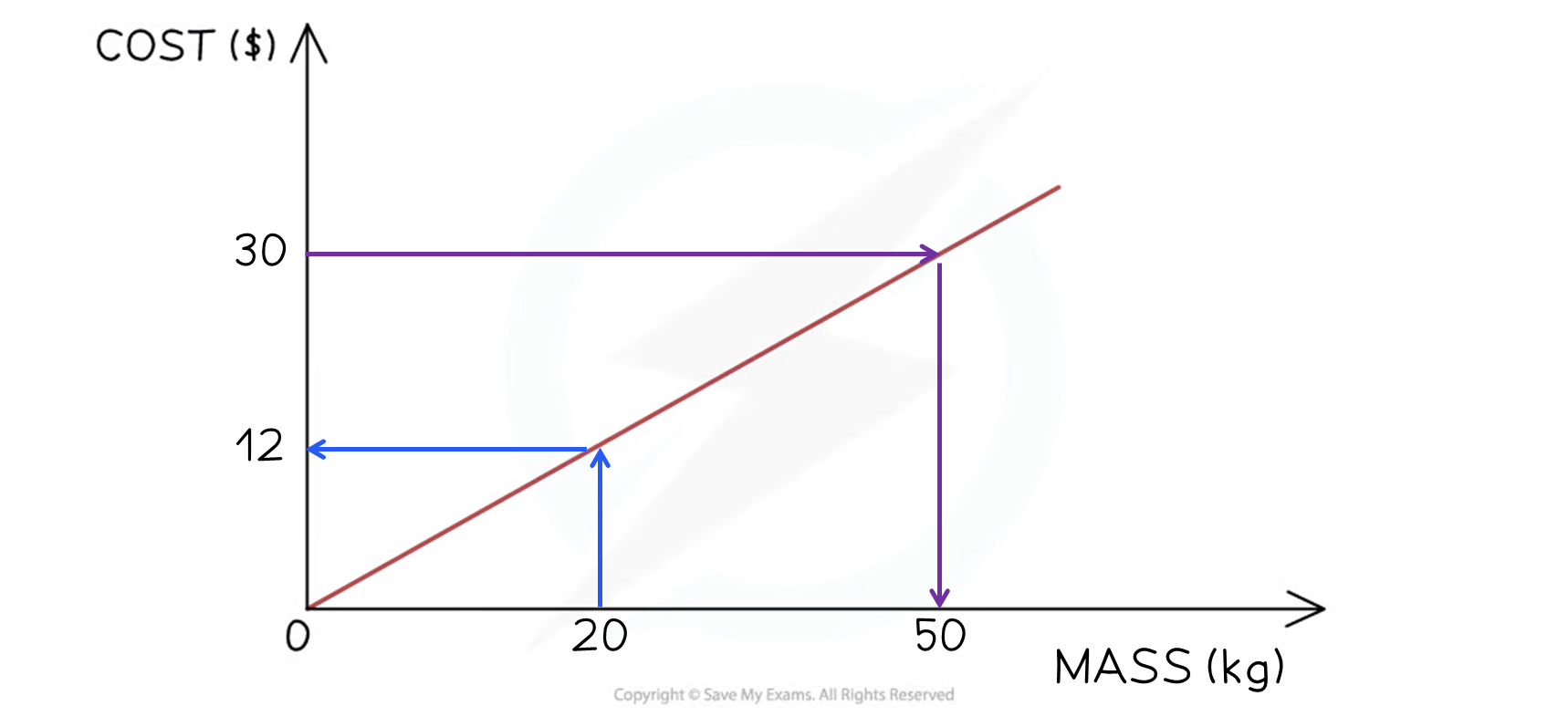
conversion-graph

How do I use a conversion graph that does not start at the origin?

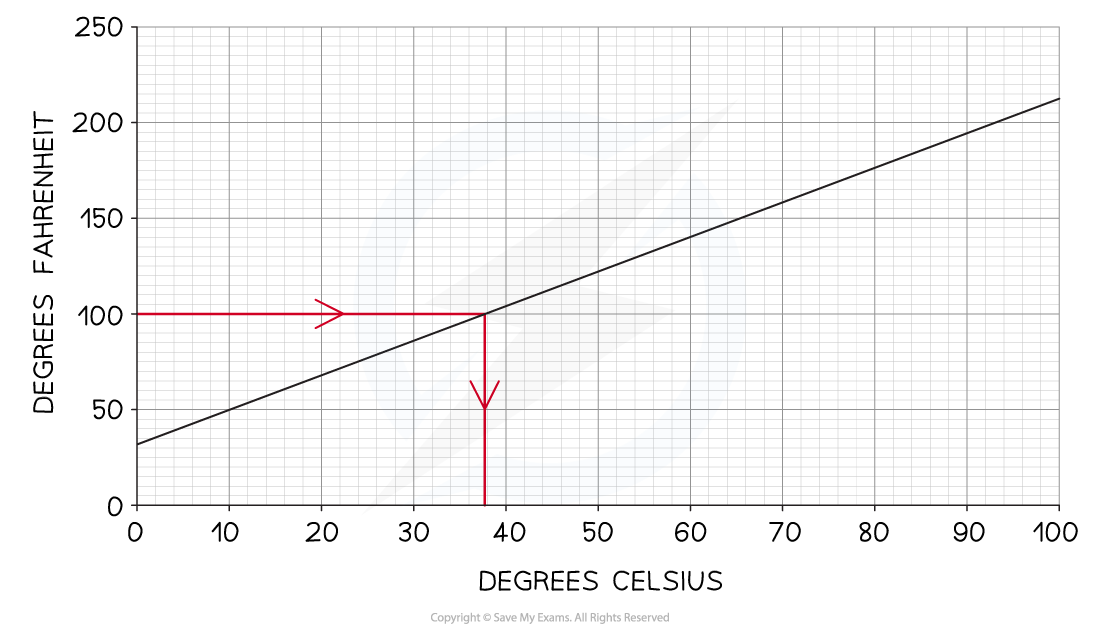
Convert 100°F into Celsius using the conversion graph below
- Start at 100°F on the y-axis
- Draw a horizontal line to the graph
- Then a vertical line down to the x-axis
- Read off the value
  - $\approx$ 37.5°C
  - Answers between 37°C and 38°C would be accepted
  - (The true answer is 37.8°C to 1 decimal place)
The graph starts at 32 on the y-axis
- This means that 0°C is 32°F
- This starting value sometimes represents a fixed cost when money is involved
  - It could represent the fixed charge for the cost of a taxi fare
To convert values that are not on the axis
- You would need to find an equation for the straight-line

A conversion graph for temperature in degrees Celsius and Fahrenheit

Examiner Tip

Always check the scales of the axes!

Worked example

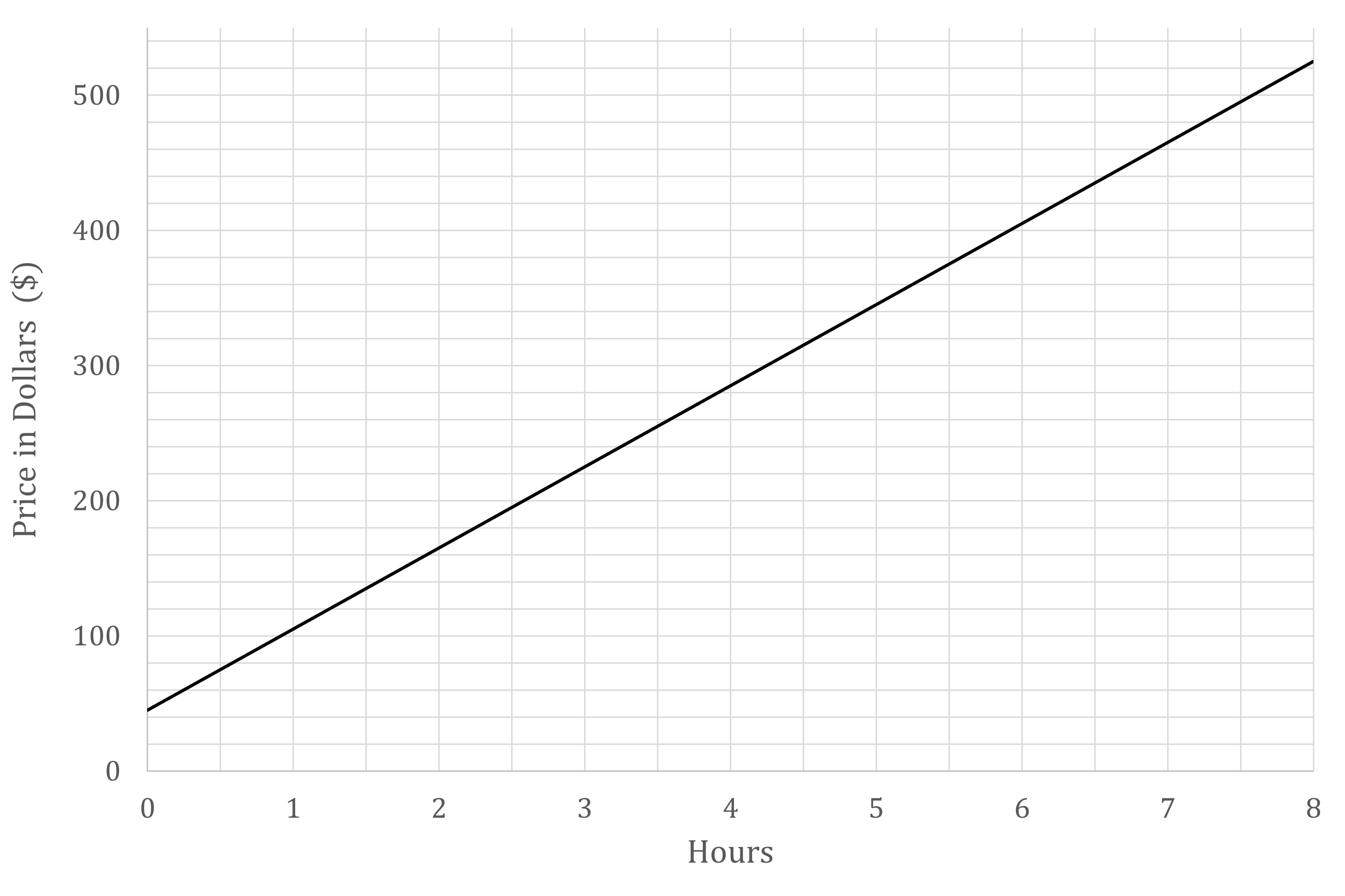
The graph below shows the price (in dollars, $) charged by a plumber for the time spent (in hours) on a particular job.

cie-igcse-conversion-graphs-we-1

(a)

Estimate the price charged for a job that takes 3 hours.

Draw a vertical line up from the x-axis at 3 hours
Then a horizontal line across to the y-axis
Read off the value

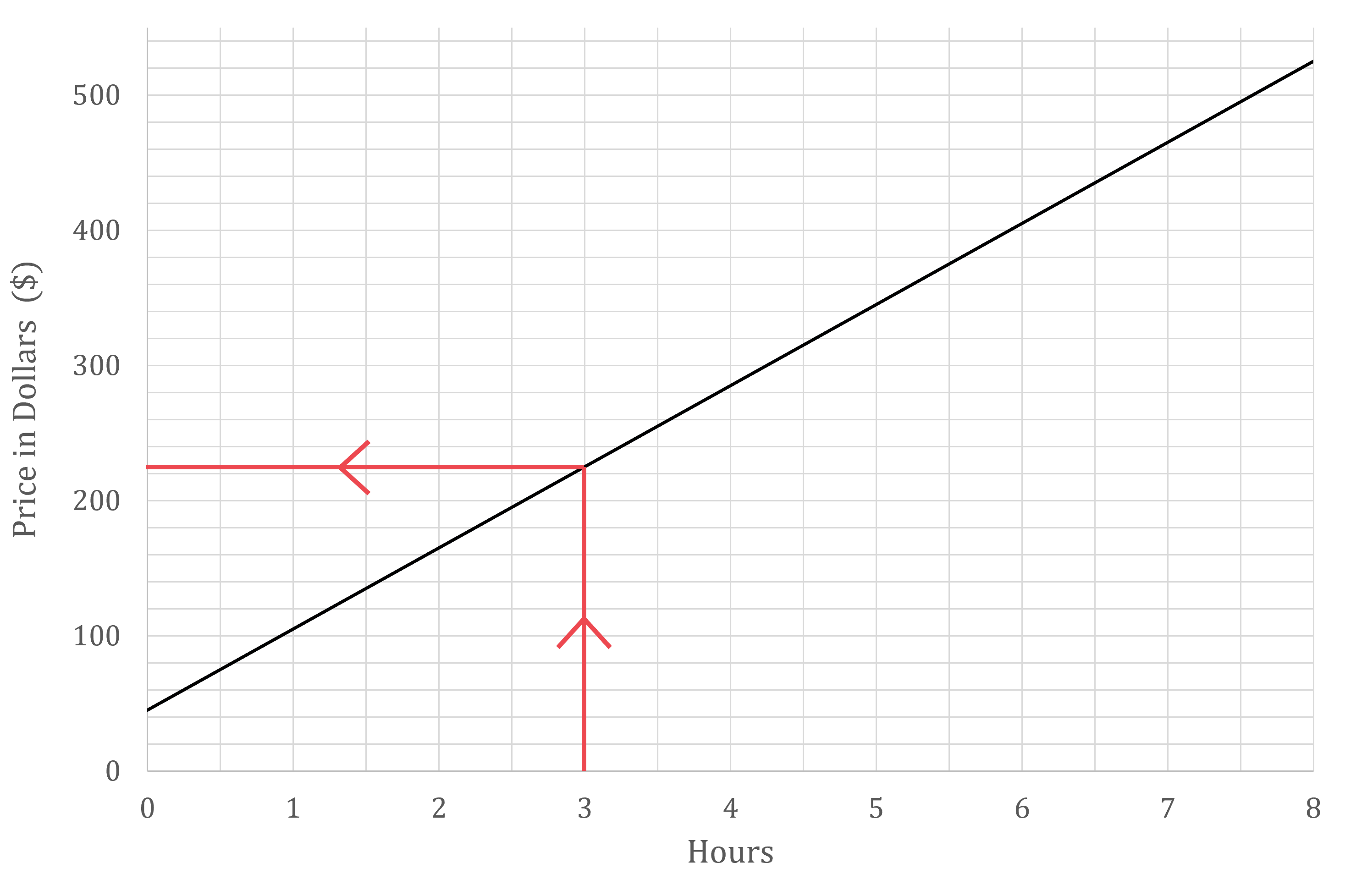
cie-igcse-conversion-graphs-we-2

Approximately $225

Answers between $220 and £230 are accepted

(b)

A particular job costs $320. Estimate, to the nearest half hour, how long this job took.

Draw a horizontal line across from the y-axis at $320
Draw a vertical line down to the x-axis
Read off the value to the nearest 0.5 hours

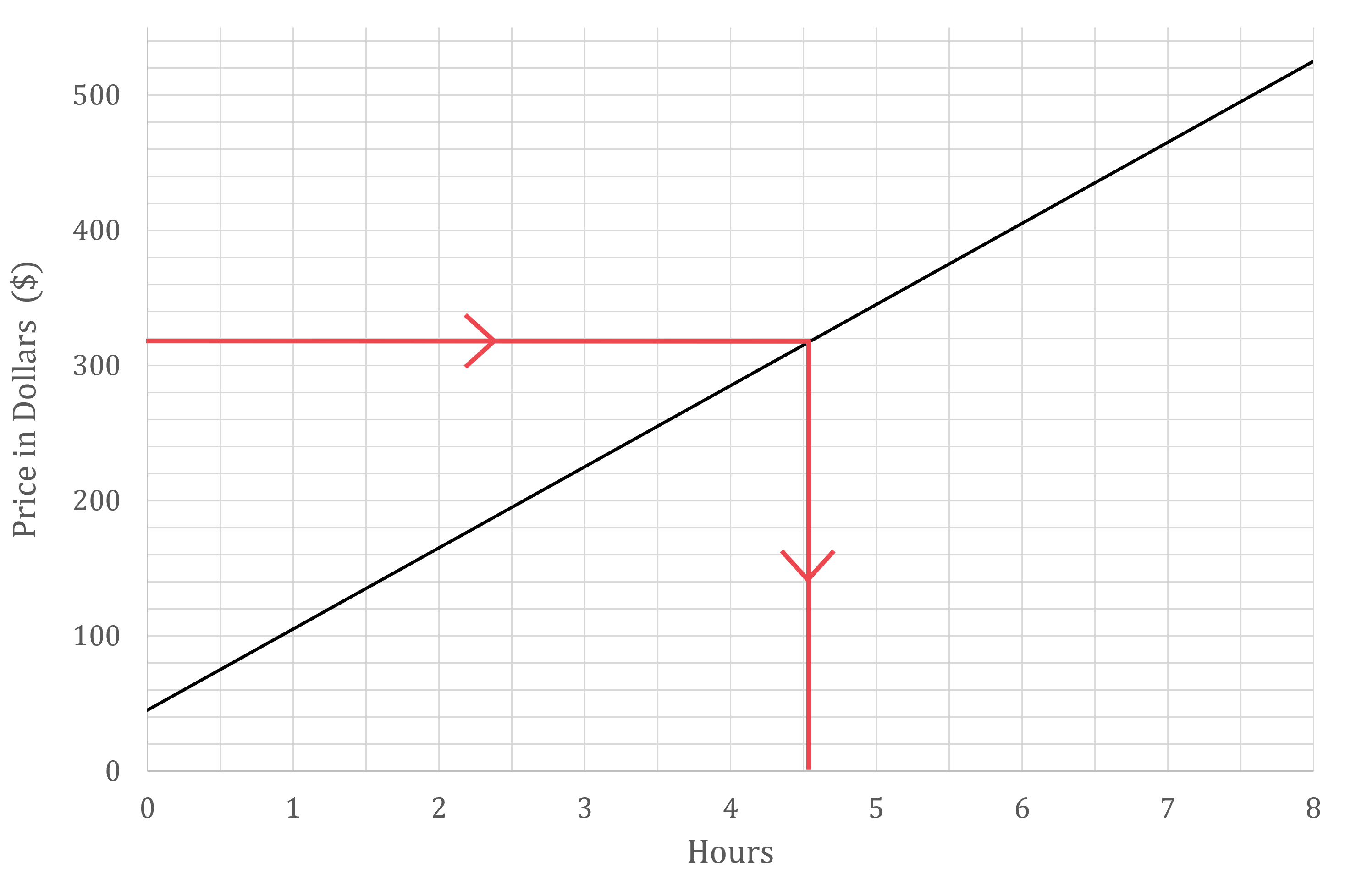
4.5 hours (to the nearest half hour)

(c)

The plumber charges a fixed callout fee for travelling to the customer and inspecting the job before starting any work.

Find the price of the callout fee.

Before starting work means 0 hours of work has been done
Find the price charged for 0 hours
This is the y-intercept of the graph

Approximately $45

Answers between $40 and £50 are accepted

Conversion Graphs (Edexcel GCSE Maths: Foundation)

Revision Note