Exponential Models (DP IB Applications & Interpretation (AI)): Revision Note
Did this video help you?
Exponential Models
What are the parameters of an exponential model?
An exponential model is of the form
or
for
Where e is the mathematical constant 2.718…
The value of
represents the boundary for the function
It can never be this value
The value of
or
describes the rate of growth or decay
The bigger the value of
or the absolute value of
the faster the function increases/decreases
What can be modelled as an exponential model?
Exponential growth or decay
Exponential growth is represented by
where
where
where
Exponential decay is represented by
where
where
where
They can be used when there is a constant percentage increase or decrease
Such as functions generated by geometric sequences
For example, suppose
is the value of a vehicle in dollars
years after it was purchased
The value of the car decreases by 5% each year
The initial value of the car is $24000+$6000 = $30000
The boundary is $6000
The car will never reach this value
Examples include:
V(t) is the value of car after t years
S(t) is the amount in a savings account after t years
B(t) is the amount of bacteria on a surface after t seconds
T(t) is the temperature of a kettle t minutes after being boiled
What are possible limitations of an exponential model?
An exponential growth model does not have a maximum
In real-life this might not be the case
The function might reach a maximum and stay at this value
Exponential models are monotonic
In real-life this might not be the case
The function might fluctuate
Examiner Tips and Tricks
These models are different to quadratic and cubic models, the constant term is not the initial value.
The initial value of
is
The initial value of
is
Worked Example
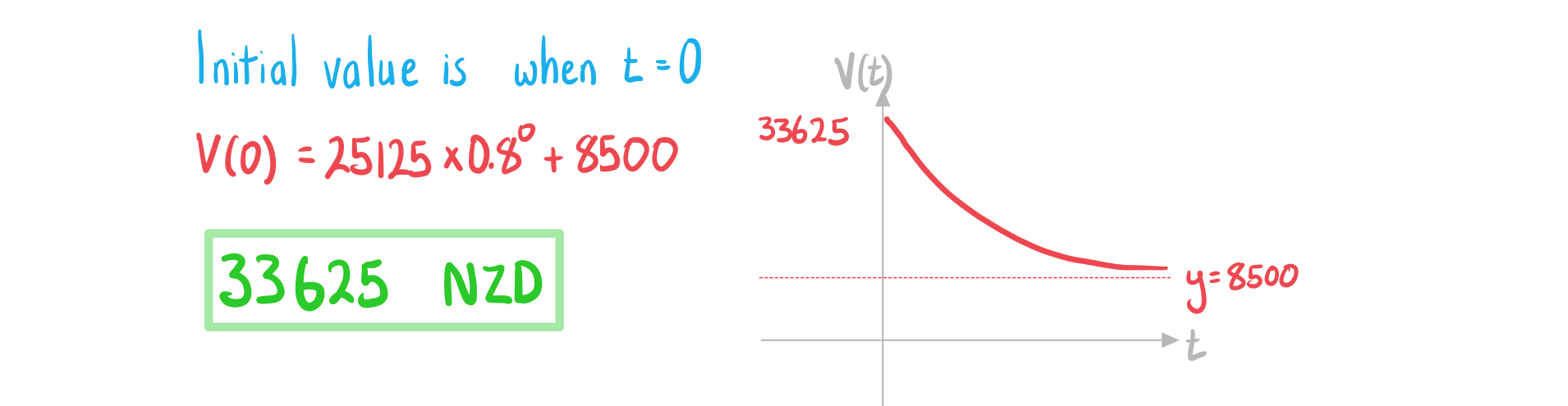
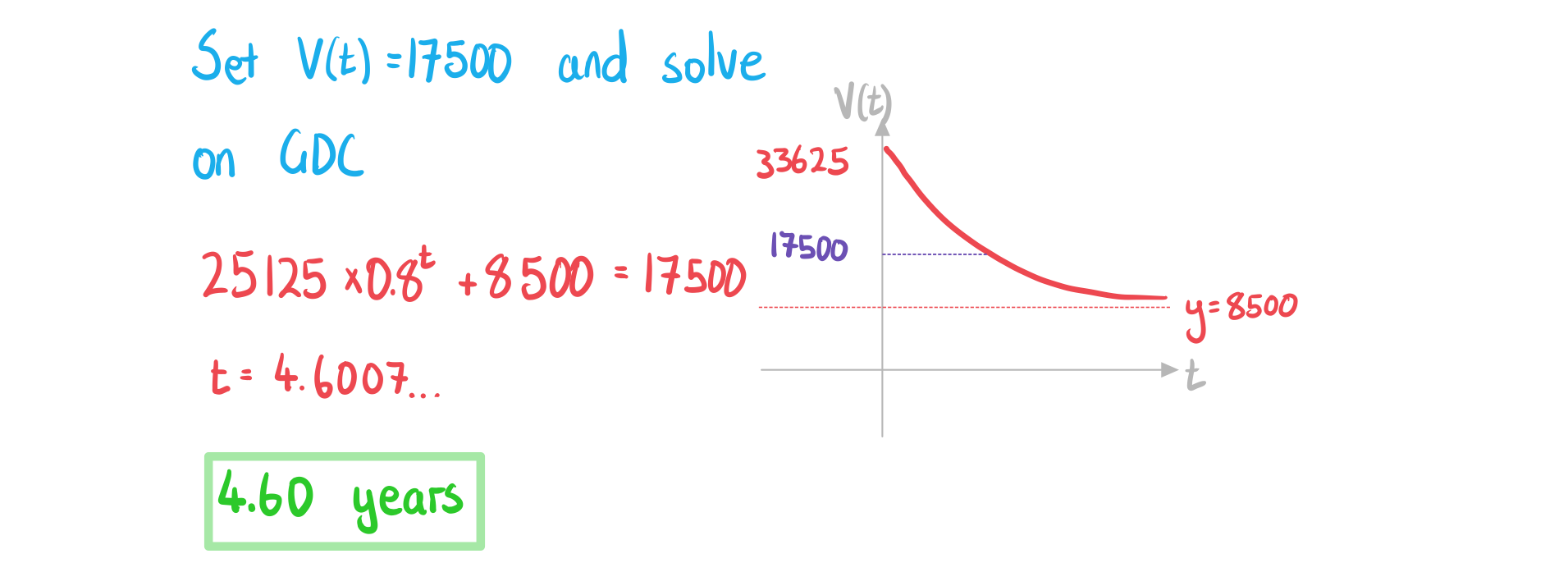
The value of a car, (NZD), can be modelled by the function
where is the age of the car in years.
a) State the initial value of the car.
b) Find the age of the car when its value is 17500 NZD.

Unlock more, it's free!
Did this page help you?