Study GuidesExam QuestionsPast PapersMock Exams
AP®PhysicsCollege BoardPhysics 1: Algebra-BasedExam QuestionsUnit 5: Torque & Rotational DynamicsRotational Kinematics

Rotational Kinematics (College Board AP® Physics 1: Algebra-Based): Exam Questions

1 hour23 questions

Select a download format for Rotational Kinematics

Scroll for more

Select an answer set to view for
Rotational Kinematics

Easy
Medium
1a
Sme Calculator2 marks

When considering the motion of a rotating door, the door is modeled as a rigid system.

i) Define the term rigid system.

ii) Justify why the door is modeled as a rigid system and not an object.

How did you do?

1b
Sme Calculator2 marks

i) Define the term angular position.

ii) Describe the difference between angular position and angular displacement.

How did you do?

1c
Sme Calculator4 marks
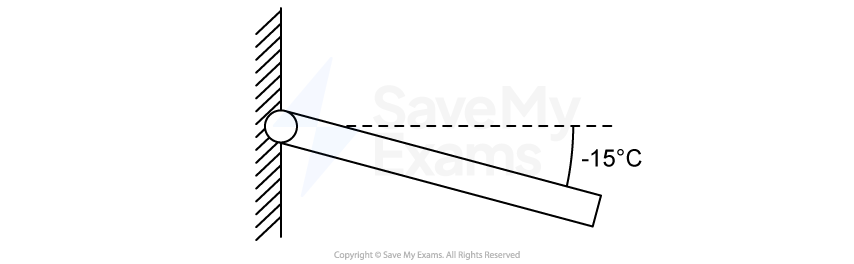
An above view of a door at an angle of 15 degrees below the horizontal.

Figure 1

The initial angular position of a swinging door is negative 15 degree, as shown in Figure 1. The door rotates until it reaches a final angular position of 30 degree.

i) Calculate the initial angular position of the door in radians, and state the direction.

ii) Calculate the angular displacement of the door in radians, and state the direction.

How did you do?

1d
Sme Calculator2 marks

When the door rotates from negative 15 degree to 30 degree, the door handle moves a linear distance of 0.75 space straight m.

Determine the distance of the handle from the door hinge.

How did you do?

Did this page help you?

2a1 mark

Define the term angular speed.

How did you do?

2b
Sme Calculator2 marks

A fairground wheel rotates clockwise at a constant rate of 1.5 space rpm.

Determine the angular speed of the wheel in rad divided by straight s.

How did you do?

2c
Sme Calculator2 marks
Ferris wheel diagram showing passenger entry at the base. Black passenger cars are evenly spaced around the wheel, with an arrow marking entry point.

Figure 1

A passenger enters a car at the bottom of the fairground wheel, as shown in Figure 1. At time t space equals space 0, the wheel begins to rotate.

i) Calculate the angular displacement, in rad, of the passenger at time t space equals space 30 space straight s.

ii) Draw a circle around a car on the diagram in Figure 1 to show the angular position of the passenger at time t space equals space 30 space straight s.

How did you do?

2d
Sme Calculator1 mark

The passenger leaves the car after the fairground wheel has completed 6 revolutions.

Calculate how long the passenger spends on the fairground wheel.

How did you do?

Did this page help you?

3a
Sme Calculator1 mark

Define the term angular acceleration.

How did you do?

3b
Sme Calculator3 marks

A car with 0.45 space straight m diameter tires is initially moving at a constant speed of 24 space straight m divided by straight s.

Calculate the angular velocity of the tires, and state the direction.

How did you do?

3c
Sme Calculator4 marks

The car decelerates uniformly from its initial speed of 24 space straight m divided by straight s to rest after the tires complete 42 revolutions.

i) Calculate the angular displacement of the tires.

ii) Calculate the angular acceleration of the tires, and state the direction.

How did you do?

3d
Sme Calculator2 marks

Calculate the linear distance traveled by the car as it comes to rest.

How did you do?

Did this page help you?

4a
Sme Calculator2 marks

State the conditions required for the rotational kinematic equations to be used.

How did you do?

4b
Sme Calculator2 marks

A disk starts spinning from rest when it undergoes a constant angular acceleration. After 10 space straight s, the disk spins with angular speed 18 space rad divided by straight s.

Calculate the angular acceleration of the disk.

How did you do?

4c
Sme Calculator2 marks

The disk continues to accelerate until it has rotated through an angular displacement of 360 space rad.

Determine the final angular speed of the disk.

How did you do?

4d
Sme Calculator2 marks

The disk has a radius of 20 space cm. Once the disk has reached its final angular speed, two coins are dropped from rest onto the disk. Coin 1 is dropped at a point 10 space cm from the disk's center. Coin 2 is dropped at the edge of the disk.

Indicate whether the tangential speed of Coin 2 is greater than, equal to, or less than the tangential speed of Coin 1. Justify your answer.

How did you do?

Did this page help you?

5a
Sme Calculator4 marks
Graph showing angle θ in radians versus time t in seconds. The line slopes upwards from (0,0) to (4,3), indicating a steady increase over time.

Figure 1

The graph in Figure 1 shows the angular position of a vinyl record as a function of time. Take counterclockwise to be the positive direction.

i) Describe the motion of the vinyl record using information from the graph in Figure 1.

ii) Using data from the graph in Figure 1, calculate the angular velocity of the vinyl record at t space equals space 4 space straight s.

How did you do?

5b
Sme Calculator6 marks
Graph depicting angular velocity in rad/s vs time in seconds; starts at 0, decreases linearly to -5 by 2s, then constant to 4s. Range: -10 to 10 rad/s.

Figure 2

The graph in Figure 2 shows the angular velocity of a different vinyl record as a function of time.

i) Describe the motion of the vinyl record using information from the graph in Figure 2.

ii) Using data from the graph in Figure 2, calculate the angular displacement of the vinyl record at t space equals space 4 space straight s.

iii) Using data from the graph in Figure 2, calculate the angular acceleration of the vinyl record between t space equals space 1 space straight s and t space equals space 3 space straight s.

How did you do?

5c
Sme Calculator2 marks

On the axes in Figure 3, sketch and label two lines to represent the angular accelerations of the vinyl records in part b) and c) as functions of time. Each line should be distinctly labeled.

Graph of angular acceleration (alpha) in radians per second squared versus time (t) in seconds, with values from -10 to 10 on the y-axis.

Figure 3

How did you do?

Did this page help you?

1
Sme Calculator2 marks
A horizontal beam attached to a hinge on a wall, and supported by a string at angle θ1. The string connects from point 1 on the wall to the beam's end, marked length L.

Figure 1

The left end of a uniform beam of mass M and length L is attached to a wall by a hinge, as shown in Figure 1. One end of a string with negligible mass is attached to the right end of the beam. The other end of the string is attached to the wall above the hinge at Point 1. The beam remains horizontal until the string is cut, and the beam begins to rotate about the hinge with negligible friction.

On the following axes, sketch the angular speed of the beam as a function of time for the time interval while the beam falls but before the beam becomes vertical.

Graph showing angular speed on the vertical axis and time on the horizontal axis, with origin labeled zero. Copyright Save My Exams.

How did you do?

Did this page help you?

2a
Sme Calculator4 marks
A circular disc with a vertical rod through its center.

Figure 1

The disk shown in Figure 1 spins about an axle at its center. During an experiment, a student discovers that, while the disk is spinning, friction between the axle and the disk exerts a constant torque on the disk. At time t space equals space 0 the disk has an initial counterclockwise (positive) angular velocity omega subscript 0. The disk later comes to rest at time t space equals space t subscript 1.

i) On the grid in Figure 2, sketch a graph to represent the disk's angular velocity as a function of time t from t space equals space 0 to t space equals space t subscript 1.

Graph showing angular velocity on the vertical axis and time on the horizontal axis, with both positive and negative angular velocity marked.

Figure 2

ii) On the grid in Figure 3, sketch a graph to represent the disk's angular acceleration as a function of time t from t space equals space 0 to t space equals space t subscript 1.

Graph depicting angular acceleration versus time, with vertical axis labeled 'Angular Acceleration', and horizontal axis labeled 't', ranging from 0 to t1.

Figure 3

How did you do?

2b
Sme Calculator4 marks

In another experiment, the disk again has an initial positive angular velocity omega subscript 0 at time t space equals space 0. At time t space equals space 1 half t subscript 1, the student starts dripping oil on the contact surface between the axle and the disk to reduce friction. As time passes, oil continues to reach the contact surface, reducing friction even further.

i) On the grid in Figure 4, sketch a graph to represent the disk's angular velocity as a function of time t from t space equals space 0 to t space equals space t subscript 1.

Graph showing angular velocity versus time, with a horizontal axis labelled "t" and vertical axis labelled "Angular velocity". Both ω₀ and -ω₀ are marked.

Figure 4

ii) On the grid in Figure 5, sketch a graph to represent the disk's angular acceleration as a function of time t from t space equals space 0 to t space equals space t subscript 1.

Graph showing angular acceleration vs time, with time on the x-axis from 0 to t1, and angular acceleration on the y-axis, remaining at 0.

Figure 5

How did you do?

Did this page help you?

3a
Sme Calculator2 marks
Diagram of a pulley system with two objects; Object 1 has mass m₀, Object 2 has mass 1.5m₀. Pulleys with radii r₀ and 2r₀ share an axle.

Figure 1

Two pulleys with different radii are attached to each other so that they rotate together about a horizontal axle through their common center. There is negligible friction in the axle. Object 1 has mass m subscript 0 and hangs from a light string wrapped around the larger pulley of radius 2 r subscript 0, while Object 2 has mass 1.5 m subscript 0 and hangs from another light string wrapped around the smaller pulley of radius r subscript 0, as shown in Figure 1.

At time t space equals space 0, the pulleys are released from rest. The magnitudes of the accelerations of Object 1 and Object 2 are a subscript 1 and a subscript 2 respectively.

Indicate whether a subscript 2 is greater than, equal to, or less than a subscript 1.

⎽⎽⎽⎽⎽⎽⎽⎽⎽⎽ a subscript 2 space greater than space a subscript 1‎ ‎ ‎ ‎ ‎ ‎ ‎ ‎ ‎ ‎ ⎽⎽⎽⎽⎽⎽⎽⎽⎽⎽ a subscript 2 space equals space a subscript 1 ‎ ‎ ‎‎ ‎ ‎ ‎ ‎ ‎ ‎ ⎽⎽⎽⎽⎽⎽⎽⎽⎽⎽ a subscript 2 space less than space a subscript 1

Justify your answer.

How did you do?

3b
Sme Calculator2 marks

At a later time t space equals space t subscript C, the string of Object 1 is cut while the objects are still moving and the pulley is still rotating.

On the axes below, sketch a graph of the angular velocity omega of the two-pulley system as a function of time t.

Graph with horizontal time axis marked 't' and vertical axis marked 'ω'. The grid is empty, and the time axis is labelled 't_c' at some point.

How did you do?

Did this page help you?