Reflections of Graphs (DP IB Applications & Interpretation (AI)): Revision Note
Did this video help you?
Reflections of graphs
What are reflections of graphs?
A reflection is when
the graph is flipped about one of the coordinate axes
Its orientation changes
The size of the graph remains unchanged
A particular reflection is specified by an axis of symmetry about which you reflect
This is the
-axis
This is the
-axis
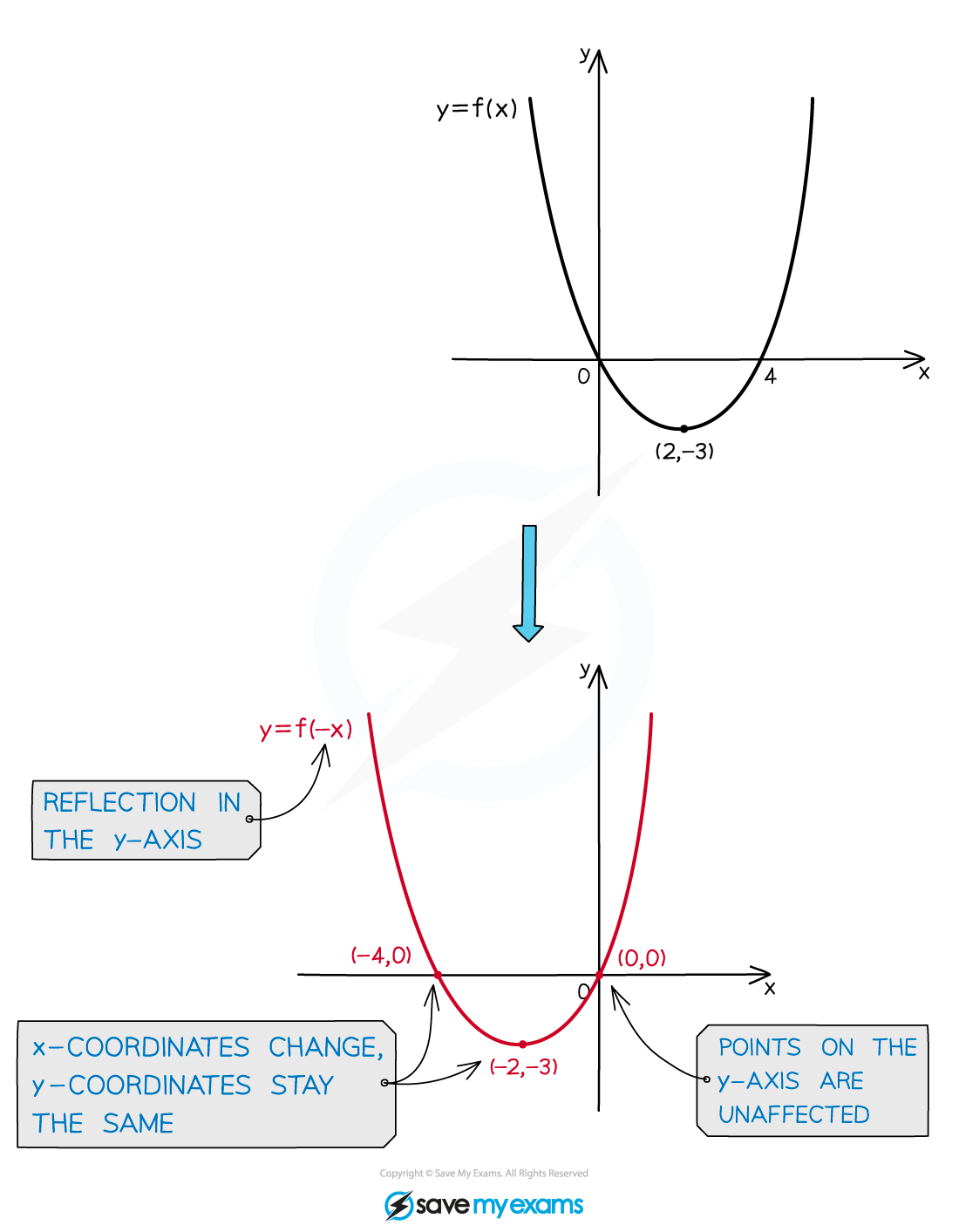
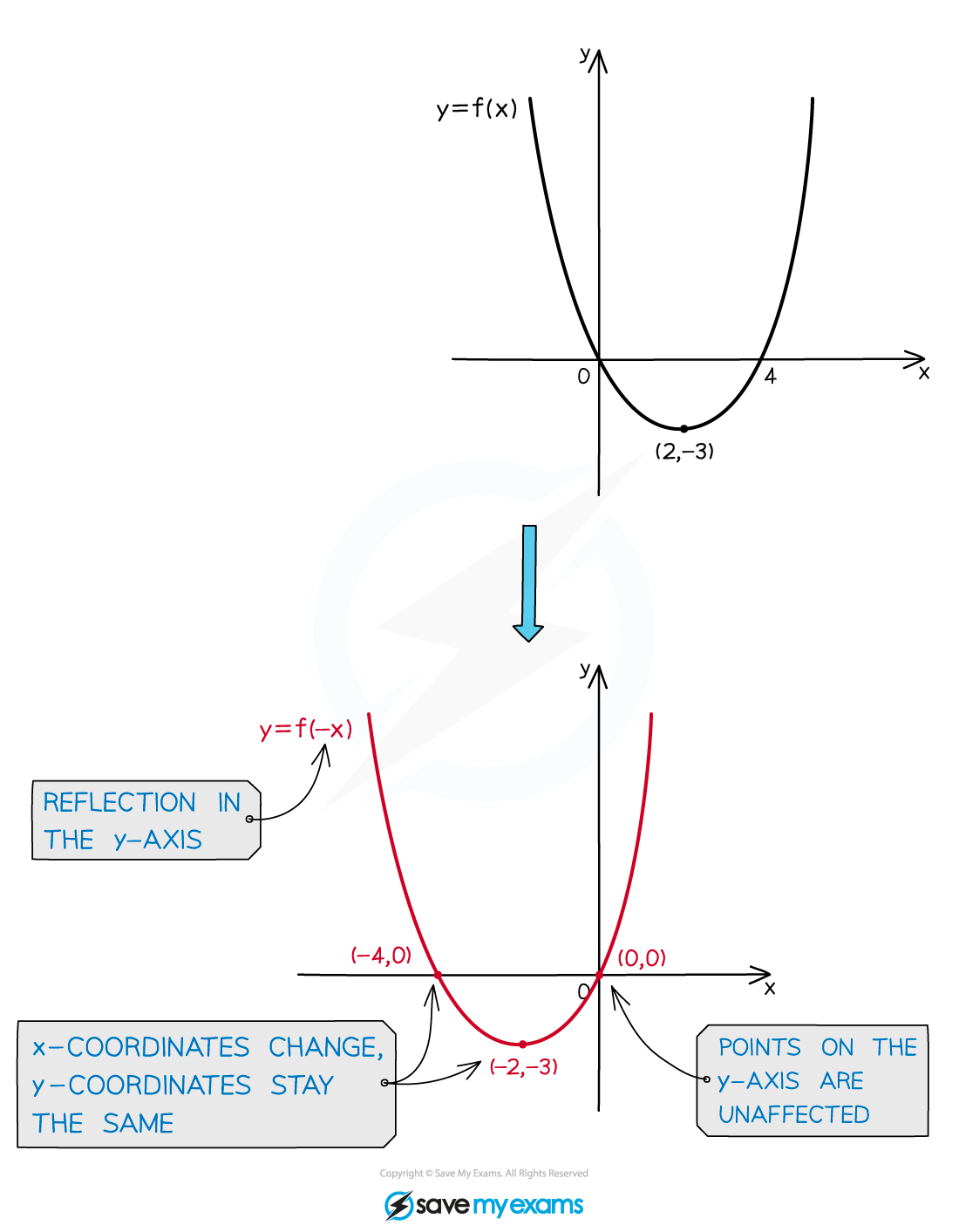
How do I find the graph equation after a reflection in the y-axis?
A horizontal reflection of the graph
about the y-axis is represented by the equation
Any vertical asymptotes change
becomes
Horizontal asymptotes stay the same
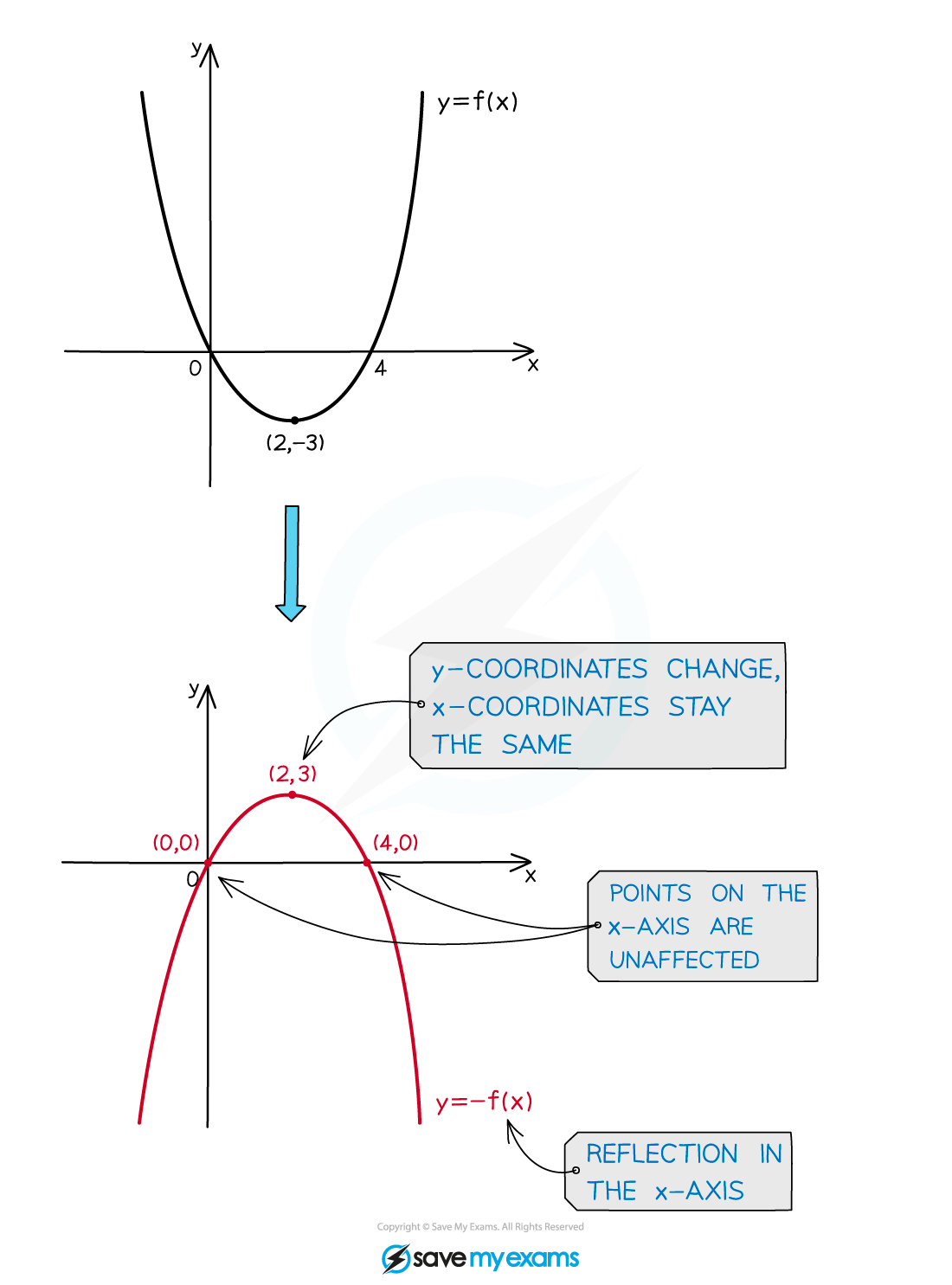
How do I find the graph equation after a reflection in the x-axis?
A vertical reflection of the graph
about the x-axis is represented by the equation
Any horizontal asymptotes change
becomes
Vertical asymptotes stay the same
Worked Example
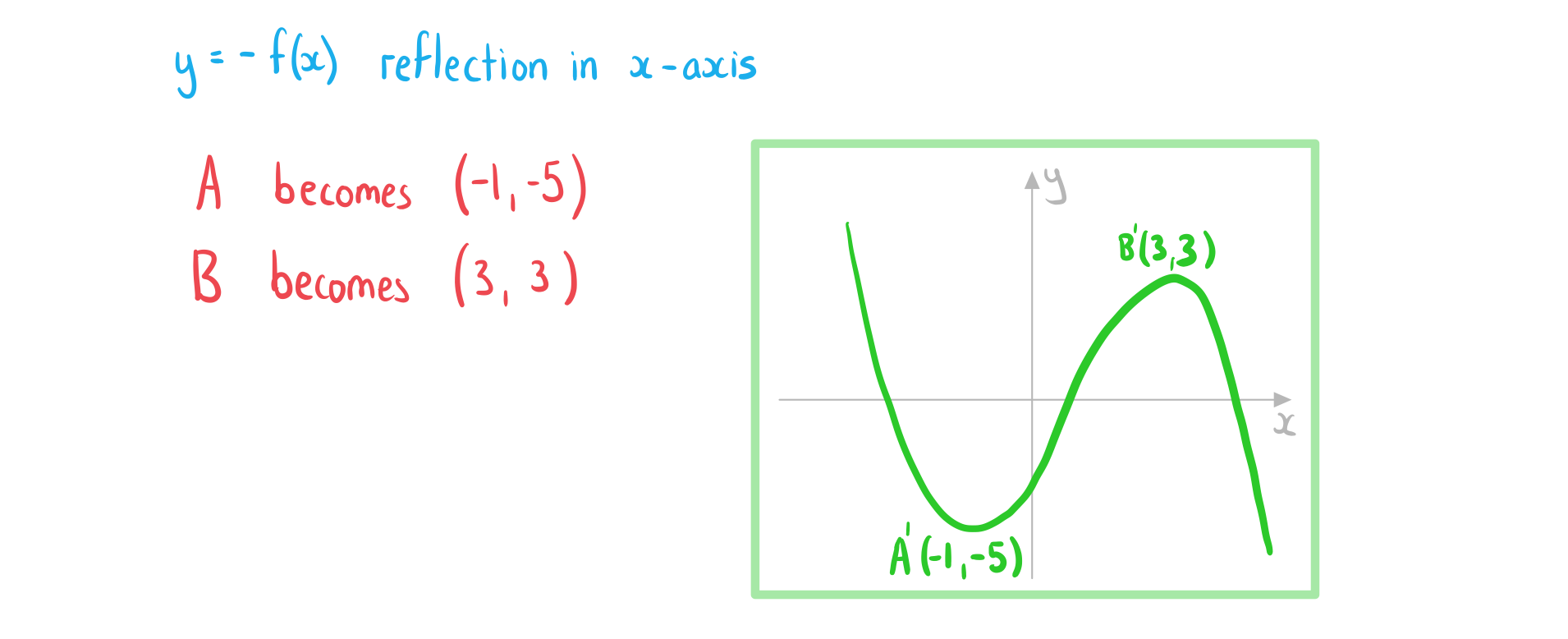
The diagram below shows the graph of .
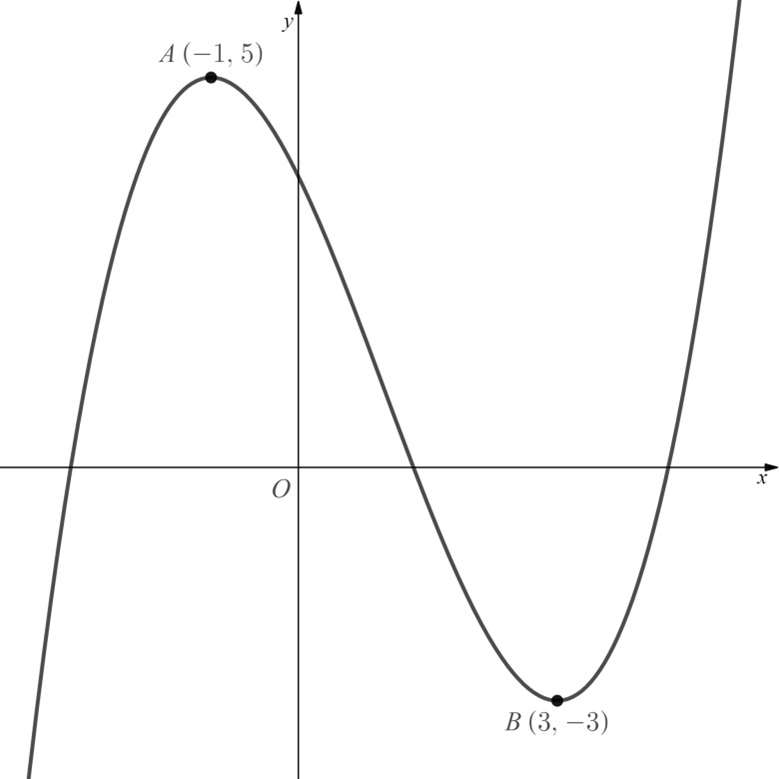
(a) Sketch the graph of .
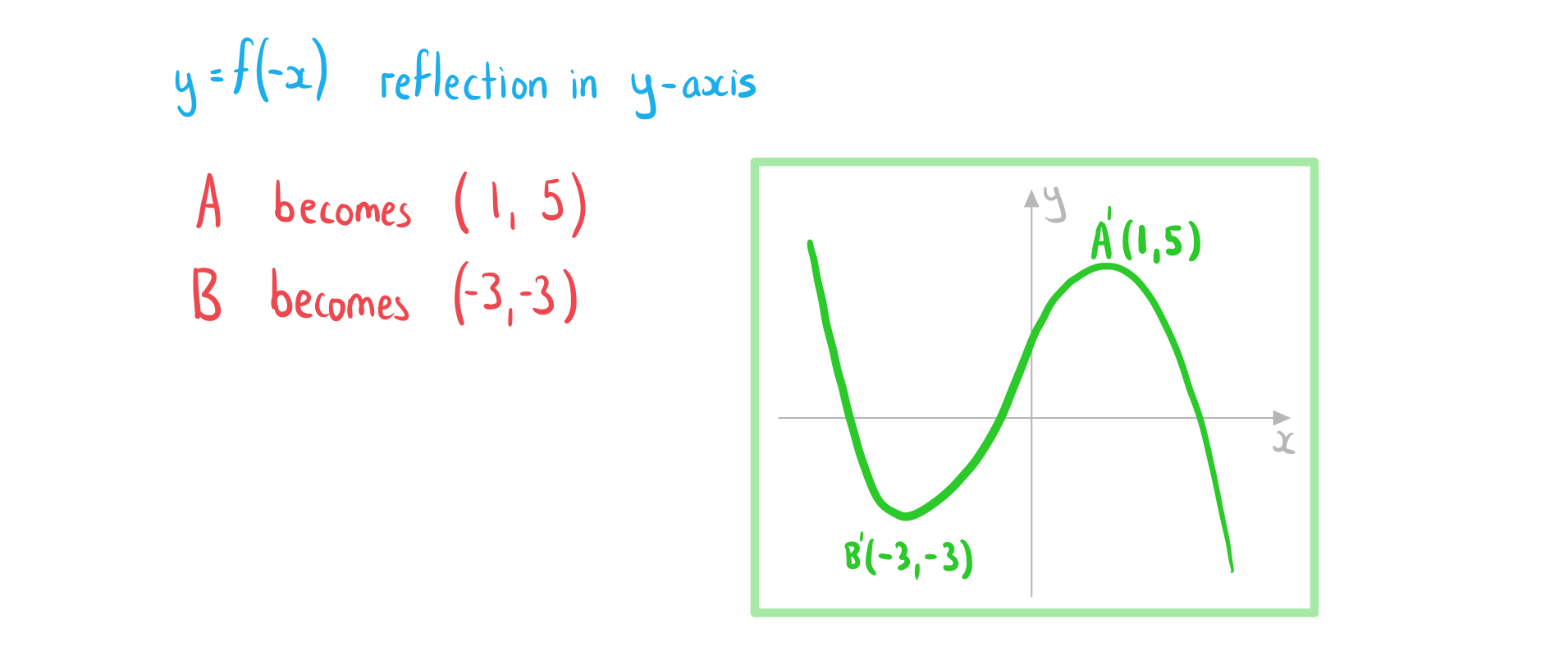
(b) Sketch the graph of .

Unlock more, it's free!
Did this page help you?