IBMathsDPAnalysis & Approaches (AA)SLRevision Notes3. Geometry & TrigonometryTrigonometric Equations & IdentitiesQuadratic Trigonometric Equations

Quadratic Trigonometric Equations (DP IB Analysis & Approaches (AA)): Revision Note

Amber

Author

Amber

Last updated

DP Analysis & Approaches (AA): SLDP Analysis & Approaches (AA): HL

Did this video help you?

Quadratic Trigonometric Equations

How are quadratic trigonometric equations solved?

  • A quadratic trigonometric equation is one that includes either sin squared space theta, cos squared space theta or tan to the power of 2 space end exponent theta

  • Often the identityspace sin squared space theta plus cos squared space theta equals 1 can be used to rearrange the equation into a form that is possible to solve

    • If the equation involves both sine and cosine then the Pythagorean identity should be used to write the equation in terms of just one of these functions

  • Solve the quadratic equation using your GDC, the quadratic equation or factorisation

    • This can be made easier by changing the function to a single letter

      • Such as changing 2 cos squared space theta minus 3 cos space theta minus 1 equals 0 to 2 c squared minus 3 c minus 1 equals 0

  • A quadratic can give up to two solutions

    • You must consider both solutions to see whether a real value exists

    • Remember that solutions for sin θ = k and cos θ = k only exist for -1 ≤ k ≤ 1  

    • Solutions for tan θ = k exist for all values of k

  • Find all solutions within the given interval

    • There will often be more than two solutions for one quadratic equation

    • The best way to check the number of solutions is to sketch the graph of the function

Examiner Tips and Tricks

  • Sketch the trig graphs on your exam paper to refer back to as many times as you need to!

  • Be careful to make sure you have found all of the solutions in the given interval, being super-careful if you get a negative solution but have a positive interval

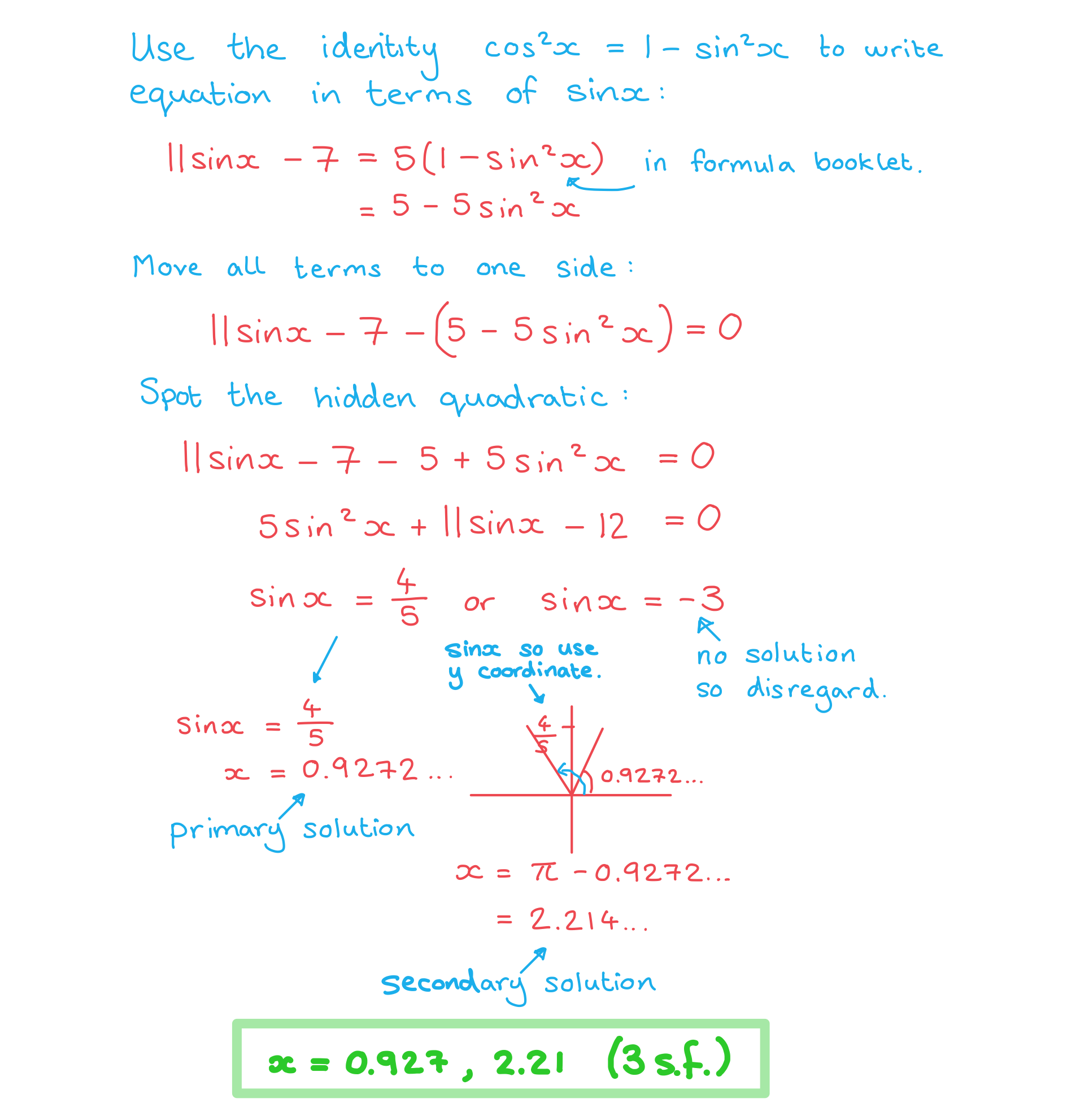
Worked Example

Solve the equation 11 sin space x blank – blank 7 blank equals 5 cos squared space x, finding all solutions in the range 0 space less or equal than space x space less or equal than space 2 straight pi.

aa-sl-3-6-5-quadratic-trig-equations-we-solution

You've read 1 of your 5 free revision notes this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

  • I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

    Beth
    IGCSE Student

  • This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

    Fathima
    A Level Student
Test yourselfFlashcards

Did this page help you?

Previous:Linear Trigonometric EquationsNext:Sampling & Data Collection
Amber

Author: Amber

Expertise: Maths Content Creator

Amber gained a first class degree in Mathematics & Meteorology from the University of Reading before training to become a teacher. She is passionate about teaching, having spent 8 years teaching GCSE and A Level Mathematics both in the UK and internationally. Amber loves creating bright and informative resources to help students reach their potential.

Download notes on Quadratic Trigonometric Equations