Binomial Expansion (AQA GCSE Further Maths) : Revision Note

Written by: Jamie Wood

Reviewed by: Dan Finlay

Updated on 5 March 2025

Pascal's Triangle

What is Pascal's Triangle?

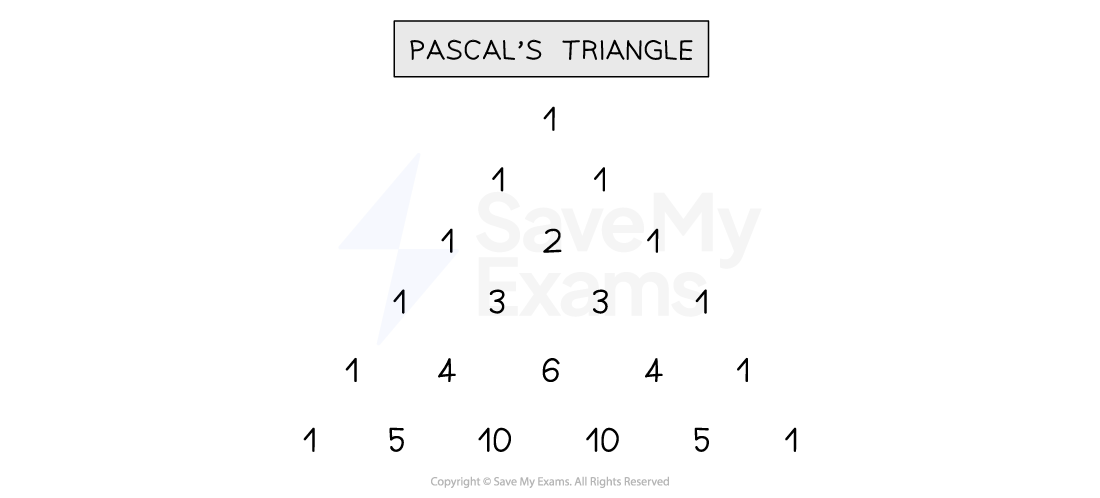
Pascal's Triangle is a triangle of number patterns (shown below)
- where each number is the sum of the two numbers above it
  - It can help to imagine zeros outside the triangle
- The triangle is symmetric

Binomial Expansion

What is a binomial?

A binomial is the sum or difference of two different terms
- e.g. $3 + 4 x$ or $p - q$
A binomial can be raised to a power, $n$
- This has the form ${(a + b)}^{n}$

How do I expand a binomial?

You can expand a binomial by multiplying out brackets, but the bigger the power, the longer this takes
- ${(a + b)}^{1} = a + b$
- ${(a + b)}^{2} = (a + b) (a + b)$
  - This gives $a^{2} + 2 a b + b^{2}$
- ${(a + b)}^{3} = (a + b) (a + b) (a + b)$
  - This gives $a^{3} + 3 a^{2} b + 3 a b^{2} + b^{3}$
- ${(a + b)}^{4} = (a + b) (a + b) (a + b) (a + b)$
  - This eventually gives $a^{4} + 4 a^{3} b + 6 a^{2} b^{2} + 4 a b^{3} + b^{4}$

The coefficients of the terms in the expansion of ${(a + b)}^{n}$ correspond to a row in Pascal's triangle
- ${(a + b)}^{0} = 1$
- ${(a + b)}^{1} = 1 a + 1 b$
- ${(a + b)}^{2} = 1 a^{2} + 2 a b + 1 b^{2}$
- ${(a + b)}^{3} = 1 a^{3} + 3 a^{2} b + 3 a b^{2} + 1 b^{3}$
- ${(a + b)}^{4} = 1 a^{4} + 4 a^{3} b + 6 a^{2} b^{2} + 4 a b^{3} + 1 b^{4}$

How do I expand a binomial using Pascal's triangle?

Using $a^{0} = b^{0} = 1$ , you can write down rules to expand ${(a + b)}^{n}$ without having to multiply out brackets
- It is the sum of terms in the form $Pascal coefficient \times a^{(. . .)} \times b^{(. . .)}$ where
  - the powers of $a$ decrease from $a^{n}$ to $a^{0}$
  - the powers of $b$ increase from $b^{0}$ to $b^{n}$
  - the Pascal coefficients come from the row starting with $1, n, . . .$
- There should be $(n + 1)$ terms in total
For example, to expand ${(a + b)}^{4}$ , there will be 4 + 1 = 5 terms as follows:

Power of $a$	$a^{4}$	$a^{3}$	$a^{2}$	$a^{1}$	$a^{0}$
Power of $b$	$b^{0}$	$b^{1}$	$b^{2}$	$b^{3}$	$b^{4}$
Pascal's triangle row	1	4	6	4	1
${(a + b)}^{4} =$	$1 a^{4} b^{0}$	$+ 4 a^{3} b^{1}$	$+ 6 a^{2} b^{2}$	$+ 4 a^{1} b^{3}$	$+ 1 a^{0} b^{4}$

This simplifies to
- ${(a + b)}^{4} = a^{4} + 4 a^{3} b + 6 a^{2} b^{2} + 4 a b^{3} + b^{4}$

How do I expand binomials with harder terms?

You need to be familiar with index laws, e.g.:
- ${(2 x)}^{3} = 2^{3} x^{3} = 8 x^{3}$
- ${(\frac{x}{2})}^{3} = \frac{x^{3}}{2^{3}} = \frac{x^{3}}{8}$
For example
- To expand ${(2 x + 3)}^{4}$
  - Imagine ${(a + b)}^{4}$ where $a = 2 x$ and $b = 3$
  - Put brackets around $(2 x)$ and $(3)$
  - Then use the rules above
- ${(2 x + 3)}^{4} = 1 {(2 x)}^{4} {(3)}^{0} + 4 {(2 x)}^{3} {(3)}^{1} + 6 {(2 x)}^{2} {(3)}^{2} + 4 {(2 x)}^{1} {(3)}^{3} + 1 {(2 x)}^{0} {(3)}^{4}$
- Apply the index laws carefully
  - $= 2^{4} x^{4} \times 1 + 4 \times 2^{3} x^{3} \times 3 + 6 \times 2^{2} x^{2} \times 3^{2} + 4 \times 2 x \times 3^{3} + 3^{4}$
  - $= 16 x^{4} + 96 x^{3} + 216 x^{2} + 216 x + 81$
Note that the final coefficients are not symmetric
- even though the Pascal coefficients used in the working are

How do I expand binomials with negative terms?

You need to be familiar with powers of negatives:
- ${(- 2)}^{2} = 4$
  - Even powers are positive
- ${(- 2)}^{3} = - 8$
  - Odd powers are negative
For example, ${(2 x - 3)}^{4}$ is ${(a + b)}^{4}$ with $a = (2 x)$ and $b = (- 3)$
- ${(2 x - 3)}^{4} = 1 {(2 x)}^{4} {(- 3)}^{0} + 4 {(2 x)}^{3} {(- 3)}^{1} + 6 {(2 x)}^{2} {(- 3)}^{2} + 4 {(2 x)}^{1} {(- 3)}^{3} + 1 {(2 x)}^{0} {(- 3)}^{4}$
  - $= 2^{4} x^{4} \times 1 + 4 \times 2^{3} x^{3} \times (- 3) + 6 \times 2^{2} x^{2} \times 9 + 4 \times 2 x \times (- 27) + 81$
  - $= 16 x^{4} - 96 x^{3} + 216 x^{2} - 216 x + 81$
- The signs alternate between positive and negative

Examiner Tips and Tricks

Check that the pairs of powers in each term of your working sum to the power of the binomial
- e.g. for ${(a + b)}^{4} = 1 a^{4} b^{0} + 4 a^{3} b^{1} + 6 a^{2} b^{2} + 4 a^{1} b^{3} + 1 a^{0} b^{4}$ the sums are 4+0, 3+1, 2+2, etc.

Worked Example

Expand and simplify ${(3 x - 2)}^{5}$ .

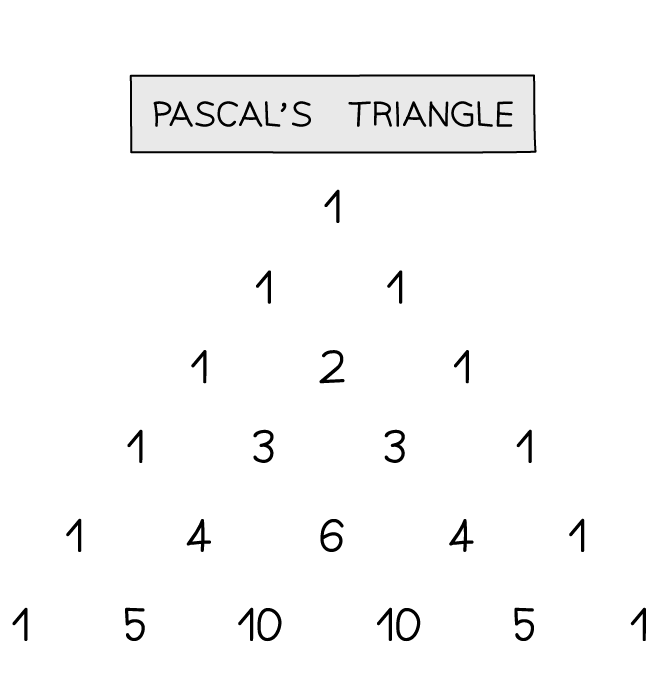
As the power of the binomial is 5, you need the row from Pascal's triangle that starts with 1, 5, ...
(You are not expected to remember this, but you are expected to be able to write out Pascal's triangle to work out the fifth row)

$\begin{matrix} 1 \\ 1 & 1 \\ 1 & 2 & 1 \\ 1 & 3 & 3 & 1 \\ 1 & 4 & 6 & 4 & 1 \end{matrix} \begin{matrix} \end{matrix} \begin{matrix} \end{matrix}$

$\begin{matrix} 1 & 5 & 10 & 10 & 5 & 1 \end{matrix}$

Write out the expansion of ${(a + b)}^{5}$ with decreasing powers of a and increasing power of b

${(a + b)}^{5} = 1 a^{5} b^{0} + 5 a^{4} b^{1} + 10 a^{3} b^{2} + 10 a^{2} b^{3} + 5 a^{1} b^{4} + 1 a^{0} b^{5}$

Remember that $b^{0} = a^{0} = 1$ and $b^{1} = b, a^{1} = a$

Substitute in $a = (3 x)$ and $b = (- 2)$

${(3 x - 2)}^{5} = 1 {(3 x)}^{5} + 5 {(3 x)}^{4} (- 2) + 10 {(3 x)}^{3} {(- 2)}^{2} + 10 {(3 x)}^{2} {(- 2)}^{3} + 5 (3 x) {(- 2)}^{4} + 1 {(- 2)}^{5}$

Use index laws to simplify each term (remember to apply the power to the number as well as the letter)

${(3 x - 2)}^{5} = (3^{5} x^{5}) + 5 (3^{4} x^{4}) (- 2) + 10 (3^{3} x^{3}) (4) + 10 (3^{2} x^{2}) (- 8) + 5 (3 x) (16) + (- 32)$

Calculate the numerical values, being careful with negative numbers

${(3 x - 2)}^{5} = 243 x^{5} - 810 x^{4} + 1080 x^{3} - 720 x^{2} + 240 x - 32$

Check that the signs alternate between positive and negative, and that powers of x decrease from 5 to 1 to 0 (a constant term)

${(3 x - 2)}^{5} = 243 x^{5} - 810 x^{4} + 1080 x^{3} - 720 x^{2} + 240 x - 32$

You've read 0 of your 5 free revision notes this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Test yourself

Did this page help you?

Previous:Forming & Solving EquationsNext:Problem Solving with Binomial Expansion

Binomial Expansion (AQA GCSE Further Maths) : Revision Note

Pascal's Triangle

What is Pascal's Triangle?

Binomial Expansion

What is a binomial?

How do I expand a binomial?

How are binomials related to Pascal's triangle?

How do I expand a binomial using Pascal's triangle?

How do I expand binomials with harder terms?

How do I expand binomials with negative terms?

You've read 0 of your 5 free revision notes this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

1. Number

Surds

Simplifying Surds

Rationalising Denominators

Counting Principles

Counting Principles

2. Algebra & Functions

Algebra Toolkit

Expanding & Factorising

Factorising Quadratics

Quadratics Factorising Methods

Completing the Square

Solving Quadratic Equations

Equating Coefficients

Forming & Solving Equations

Binomial Expansion

Binomial Expansion

Problem Solving with Binomial Expansion

Algebraic Fractions

Algebraic Fractions

Rearranging Formulae

Rearranging Formulae

Indices

Indices

Algebraic Proof

Algebraic Proof

Polynomials & Factor Theorem

Factor Theorem

Applications of Factor Theorem

Shapes of Graphs

Quadratic & Polynomial Graphs

Exponential Graphs

Solving Equations using Graphs

Simultaneous Equations

Linear Simultaneous Equations

Quadratic Simultaneous Equations

Simultaneous Equations with 3 Variables

Solving Inequalities

Linear Inequalities

Quadratic Inequalities

Functions

Functions Toolkit

Composite & Inverse Functions

Sequences

Finding nth Terms of Sequences

Using nth Terms of Sequences

3. Coordinate Geometry

Coordinate Geometry & Straight Lines

Coordinate Geometry

Gradients

Equation of a Straight Line

Equation of a Circle

Equation of a Circle

Tangents to Circles

4. Calculus

Differentiation

Definition of Gradient

Differentiating Powers of x

Applications of Differentiation

Tangents & Normals

Increasing & Decreasing Functions

Second Derivatives

Stationary Points & Turning Points

Modelling with Differentiation inc. Optimisation

5. Matrix Transformations

Matrix Multiplication

Introduction to Matrices