GCSEFurther MathsAQARevision Notes4. CalculusApplications of DifferentiationTangents & Normals

Tangents & Normals (AQA GCSE Further Maths)

Revision Note

Test yourself
Jamie Wood

Written by: Jamie Wood

Reviewed by: Dan Finlay

Finding a Tangent

Using the derivative to find a tangent

  • At any point on a curve, the tangent is the line that touches the point and has the same gradient as the curve at that point

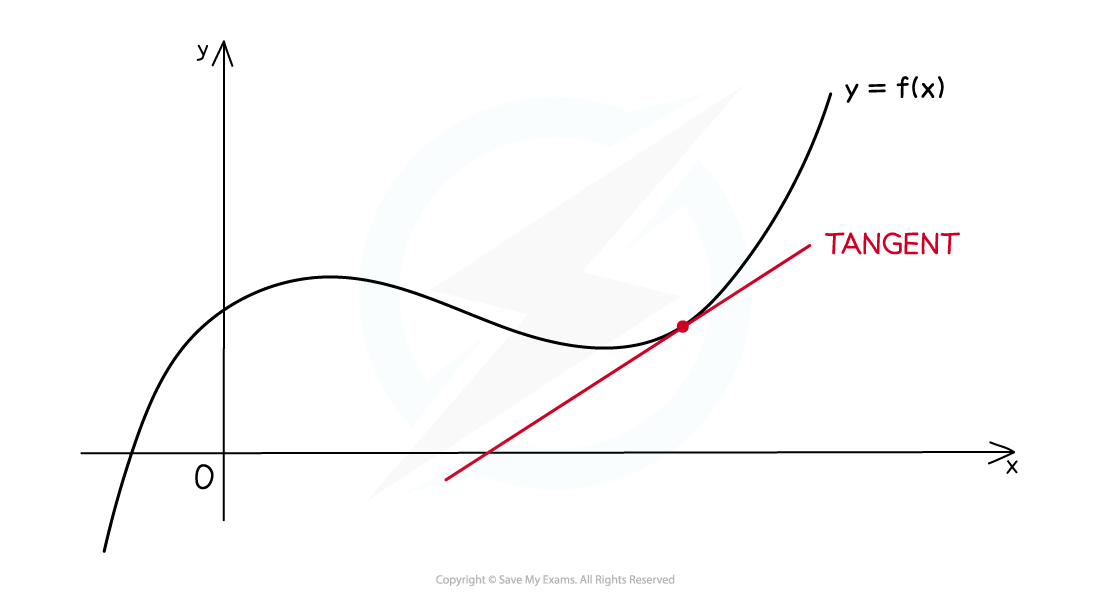
Grad Tang Norm Illustr 2, A Level & AS Maths: Pure revision notes

  • When given a curve, you can find the equation of the tangent to the curve at the point open parentheses a comma space b close parentheses by:

    • Finding the derivative (gradient) of the curve at point x equals a

      • This is also the gradient of the tangent line

      • You can find this by differentiating the equation of the curve, and substituting in x equals a

    • Substituting the value of the gradient open parentheses m close parentheses into the equation of the tangent, in the form y equals m x plus c

    • To find the full equation of the tangent, substitute in the point open parentheses a comma space b close parentheses as y and x and solve to find c

    • You could alternatively use the form y minus y subscript 1 equals m open parentheses x minus x subscript 1 close parentheses for the equation of a line where open parentheses x subscript 1 comma space y subscript 1 close parentheses is the point open parentheses a comma space b close parentheses and m is still the gradient

  • Sometimes, you may not be told the full coordinate; just the x-value

    • In this case, substitute the x-value into the equation of the curve (not the derivative) to find the full coordinate, and then follow the method above

Examiner Tips and Tricks

  • A good sketch of the curve and the tangent at a point can help you spot if the tangent will have a positive or negative gradient; helping you to check your answer

Worked Example

Work out the equation of the tangent to the curve y equals 2 x squared minus 6 x plus 10 at the point where x equals 1.
Write your answer in the form y equals m x plus c.

Find the derivative of the curve.

table row cell fraction numerator d y over denominator d x end fraction end cell equals cell space 4 x space minus space 6 end cell end table 

To find the gradient of the curve at the point where x space equals space 1 comma substitute x space equals space 1 into the derivative of the curve.

table row cell m space end cell equals cell space 4 open parentheses 1 close parentheses space minus space 6 space equals space minus 2 end cell end table

This is the same as the gradient of the tangent to the curve at the point where x space equals space 1, so the equation of the line is in the form y space equals space minus 2 x space plus space c. 

To find the value of size 16px c we will need to know the full coordinate at the point where x space equals space 1. We can find this by substituting x space equals space 1 into the equation for the curve (be careful to substitute it into the original equation and not your differentiated version).

y space equals space 2 open parentheses 1 close parentheses squared space minus 6 open parentheses 1 close parentheses space plus space 10

Simplify to find the value of y and hence, the full coordinate.

table row cell y space end cell equals cell space 2 space minus space 6 space plus space 10 space equals space 6 end cell end table

Substitute x space equals space 1 and size 16px y size 16px space size 16px equals size 16px space size 16px 6 into the equation of the line.

6 space equals space minus 2 open parentheses 1 close parentheses space plus space c

Solve this equation to find size 16px c size 16px.
table row cell 6 space end cell equals cell space minus 2 space plus space c end cell row cell c space end cell equals cell space 8 end cell end table

Write out the equation of the tangent to the curve in the form given in the question.

bold italic y bold equals bold minus bold 2 bold italic x bold space bold plus bold space bold 8

Finding a Normal

Using the derivative to find a normal

  • At any point on a curve, the normal is the line that goes through the point and is perpendicular to the tangent at that point

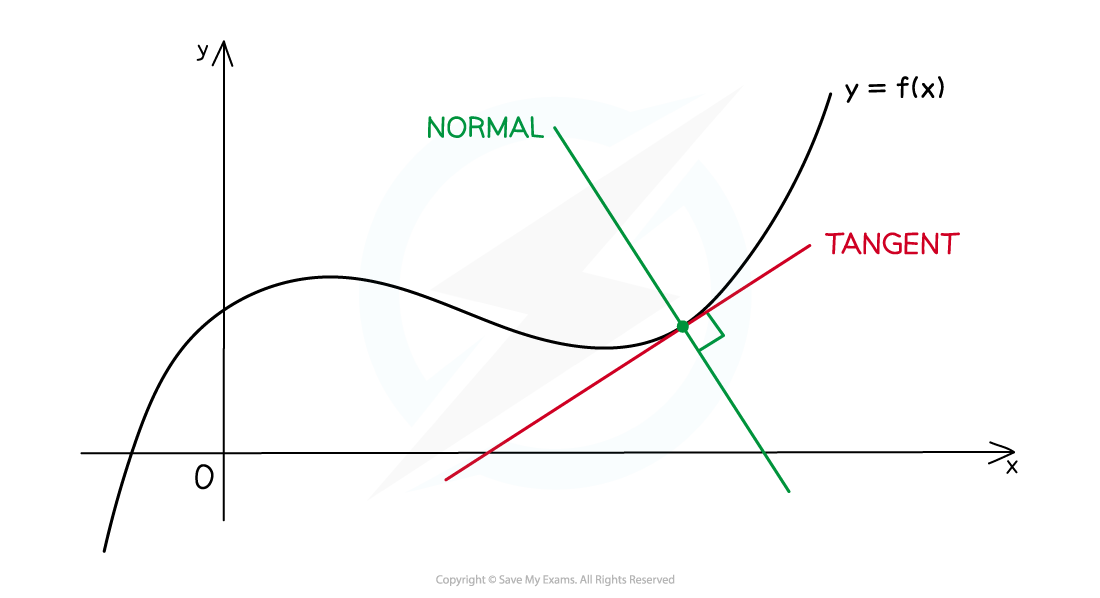
Grad Tang Norm Illustr 3, A Level & AS Maths: Pure revision notes

  • The process for finding a normal to a curve is the same as finding a tangent to a curve, but with one extra step

  • When given a curve, you can find the equation of the normal to the curve at the point open parentheses a comma space b close parentheses by:

    • Finding the derivative (gradient) of the curve at point x equals a

      • This is also the gradient of the tangent line

      • You can find this by differentiating the equation of the curve, and substituting in x equals a

    • EXTRA STEP: Find the negative reciprocal of the gradient

      • This will be the gradient of the normal

      • The negative reciprocal of g is fraction numerator negative 1 over denominator g end fraction

        • e.g. If the gradient of the tangent is 3, the gradient of the normal will be negative 1 third

    • Substituting the value of the gradient of the normal open parentheses m close parentheses into the equation of the normal, in the form y equals m x plus c

    • To find the full equation of the normal, substitute in the point open parentheses a comma space b close parentheses as y and x and solve to find c

    • You could alternatively use the form y minus y subscript 1 equals m open parentheses x minus x subscript 1 close parentheses for the equation of a line where open parentheses x subscript 1 comma space y subscript 1 close parentheses is the point open parentheses a comma space b close parentheses and m is still the gradient

  • Sometimes, you may not be told the full coordinate; just the x-value

    • In this case, substitute the x-value into the equation of the curve (not the derivative) to find the full coordinate, and then follow the method above

Examiner Tips and Tricks

  • It sounds obvious, but read the question carefully to see if you need to find a normal or a tangent! (and check again at the end!)

    • It is a very common mistake, especially under exam pressure

Worked Example

Work out the equation of the normal to the curve y equals 4 over x at the point where x equals 1.
Give your answer in the form a x plus b y plus c equals 0 where a, b, and c are integers.

Find the derivative of the curve, you will need to rewrite the equation of the curve using index form first.

table row cell y space end cell equals cell space 4 x to the power of negative 1 end exponent end cell row cell fraction numerator d y over denominator d x end fraction end cell equals cell space minus 4 x to the power of negative 2 end exponent space space equals space minus 4 over x squared end cell end table 

To find the gradient of the curve at the point where x space equals space 1 comma substitute x space equals space 1 into the derivative of the curve.

table row cell g space end cell equals cell space minus fraction numerator 4 space over denominator 1 squared end fraction space equals space minus 4 end cell end table

This is the same as the gradient of the tangent to the curve at the point where x space equals space 1, to find the gradient of the normal to the curve at the point where x space equals space 1 find the negative reciprocal of g.

table row cell m space end cell equals cell space minus 1 over g equals space minus fraction numerator 1 over denominator negative 4 end fraction space equals space 1 fourth end cell end table

So the equation of the normal is in the form y space equals space 1 fourth x space plus space c. 

To find the value of size 16px c we will need to know the full coordinate at the point where x space equals space 1. We can find this by substituting x space equals space 1 into the equation for the curve (be careful to substitute it into the original equation and not your differentiated version).

y space equals 4 over 1 space equals space 4

Substitute x space equals space 1 and y space equals space 4 into the equation of the normal.

4 space equals space 1 fourth open parentheses 1 close parentheses space plus space c

Solve this equation to find size 16px c size 16px.

table row 4 equals cell space 1 fourth space plus space c end cell row cell c space end cell equals cell space 15 over 4 end cell end table

Write out the equation of the normal to the curve in the form table row cell y space end cell equals cell space m x space plus space c end cell end table and then rearrange to the form given in the question.

y space equals space 1 fourth x space plus space 15 over 4

Multiply each term by 4.

4 y space equals space x space plus space 15

Subtract size 16px 4 size 16px y from both sides.

bold italic x bold space bold minus bold 4 bold italic y bold space bold plus bold space bold 15 bold space bold equals bold space bold 0

Last updated:

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Differentiating Powers of xNext:Increasing & Decreasing Functions
    Author
    Reviewer
    Jamie Wood

    Author: Jamie Wood

    Expertise: Maths

    Jamie graduated in 2014 from the University of Bristol with a degree in Electronic and Communications Engineering. He has worked as a teacher for 8 years, in secondary schools and in further education; teaching GCSE and A Level. He is passionate about helping students fulfil their potential through easy-to-use resources and high-quality questions and solutions.

    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.