Volumes of Revolution (Cambridge (CIE) A Level Maths) : Revision Note

Volumes of revolution around the x-axis

What is a volume of revolution around the x-axis? 

• A solid of revolution is formed when an area bounded by a function  (and other boundary equations) is rotated 360° around the x-axis

• A volume of revolution is the volume of this solid formed

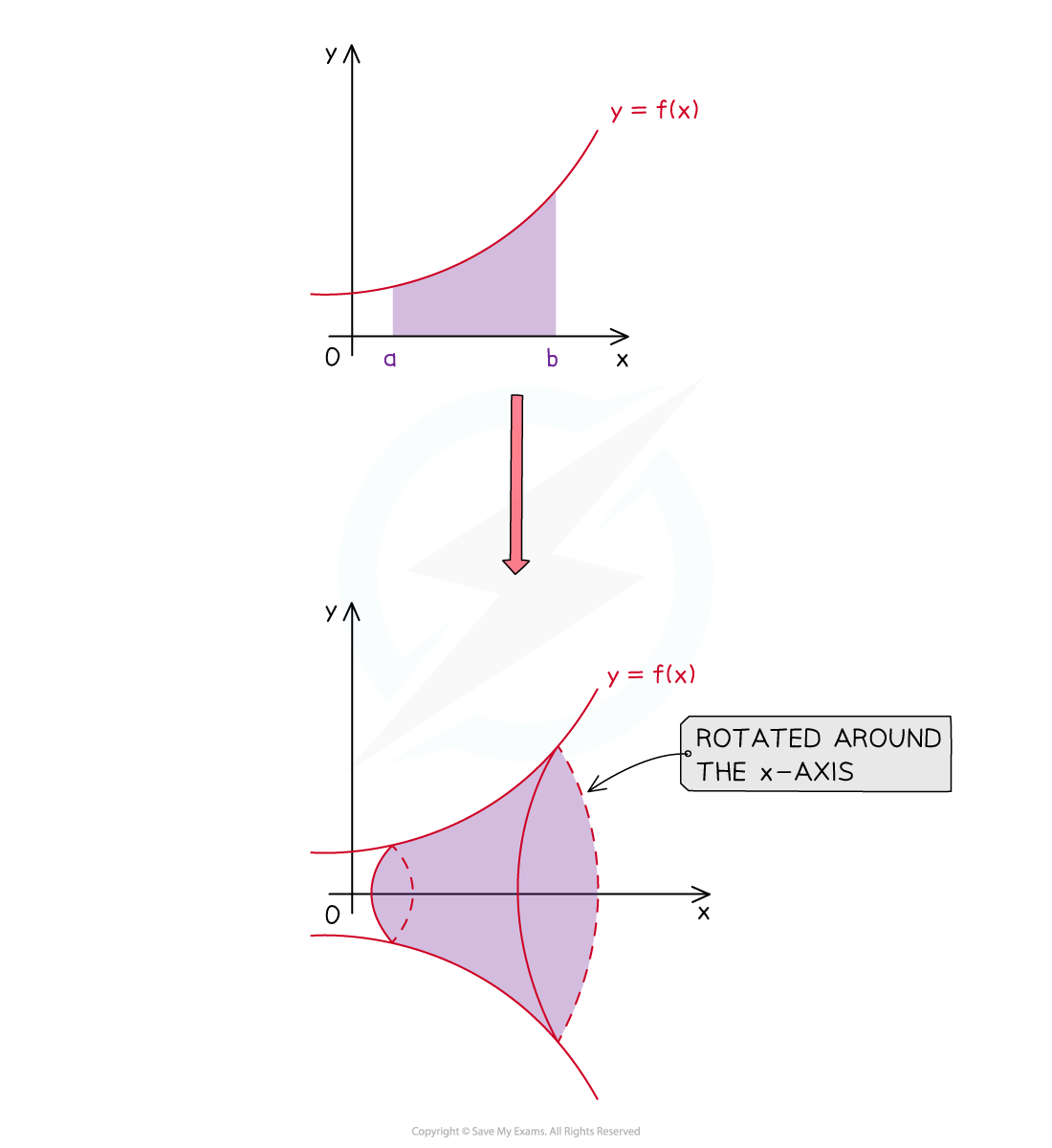
Example of a solid of revolution that is formed by rotating the area bounded by the function , the lines  and   and the -axis about the -axis

How do I find the volume of revolution around the x-axis? 

• To find the volume of revolution created when the area bounded by the function , the lines  and , and the x-axis is rotated 360° about the x-axis use the formula

• The formula may look complicated or confusing at first due to the y and dx

  • remember that y is a function of x

  • once the expression for y is substituted in, everything will be in terms of x

• π is a constant so you may see this written either inside or outside the integral

• This is not given in the formulae booklet

  • The formulae booklet does list the volume formulae for some common 3D solids – it may be possible to use these depending on what information about the solid is available

How do I solve problems involving volumes of revolution around the x-axis? 

• Visualising the solid created is helpful

  • Try sketching some functions and their solids of revolution to help

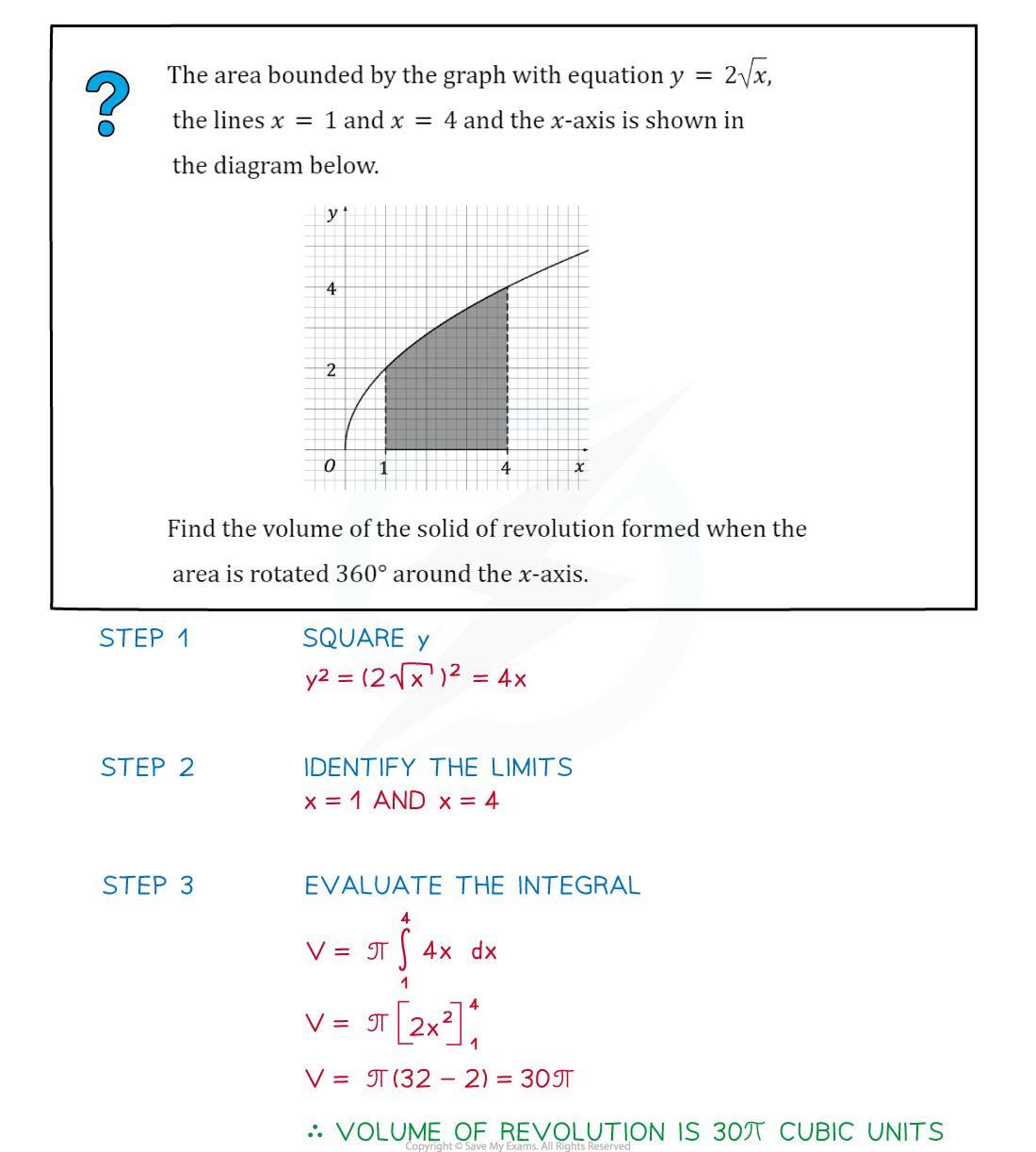
•  STEP 1 Square y            

  • Do this first without worrying about π or the integration and limits

• STEP 2 Identify the limits a and b (which could come from a graph)

• STEP 3 Use the formula by evaluating the integral and multiplying by π

  • The answer may be required in exact form (leave in terms of π)

    • If not, round to three significant figures (unless told otherwise)

• Trickier questions may give you the volume and ask for the value of an unknown constant elsewhere in the problem

Volumes of revolution around the y-axis

What is a volume of revolution around the y-axis? 

• A solid of revolution is formed when an area bounded by a function  (and other boundary equations) is rotated 360° around the y-axis

• A volume of revolution is the volume of this solid formed

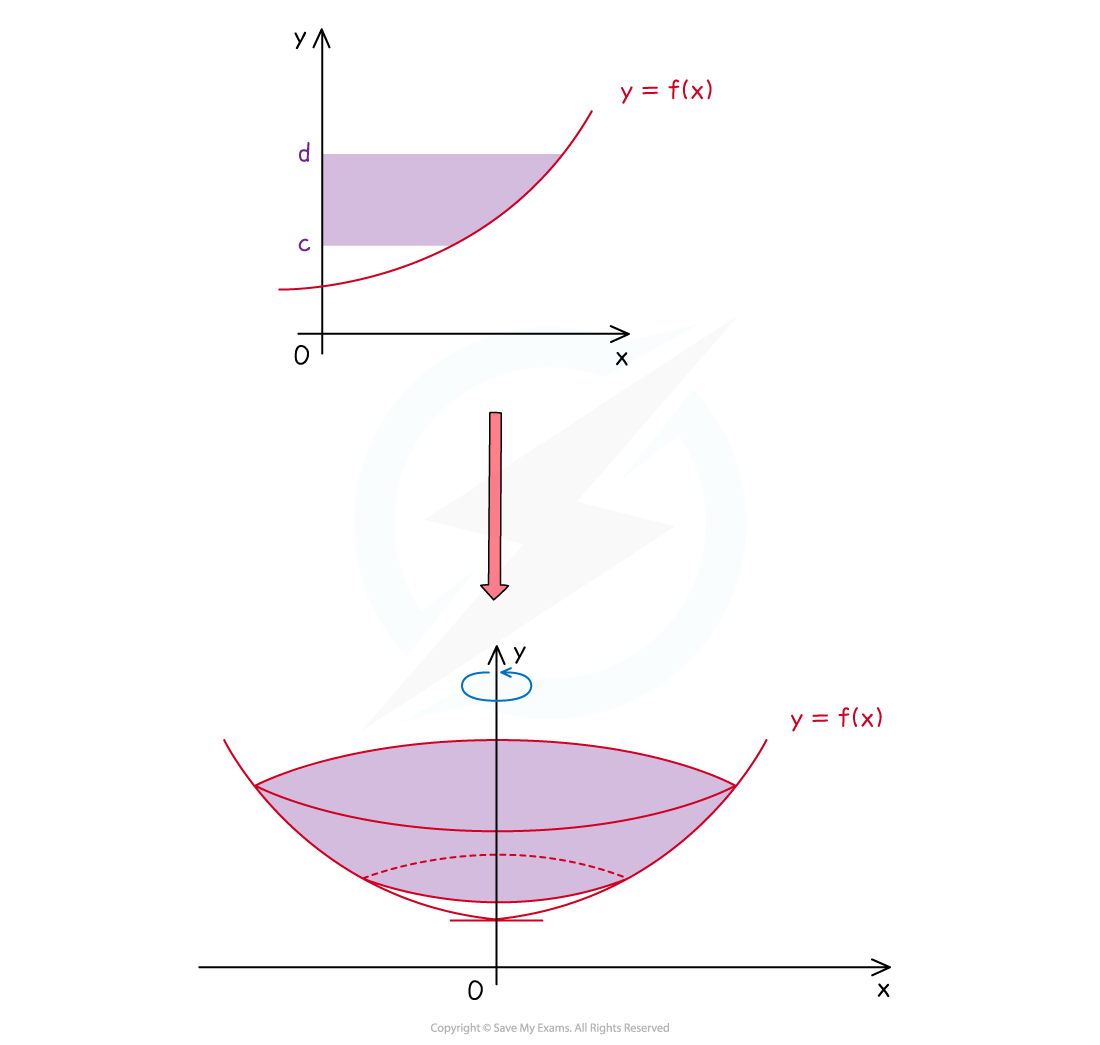
Example of a solid of revolution that is formed by rotating the area bounded by the function , the lines and  and the -axis about the -axis

How do I find the volume of revolution around the y-axis? 

• To find the volume of revolution created when the area bounded by the function , the lines  and , and the y-axis is rotated 360° about the y-axis use the formula

• Note that although the function may be given in the form  it will first need rewriting in the form  

• This is not given in the formulae booklet

How do I solve problems involving volumes of revolution around the y-axis? 

• Visualising the solid created is helpful

  • Try sketching some functions and their solids of revolution to help 

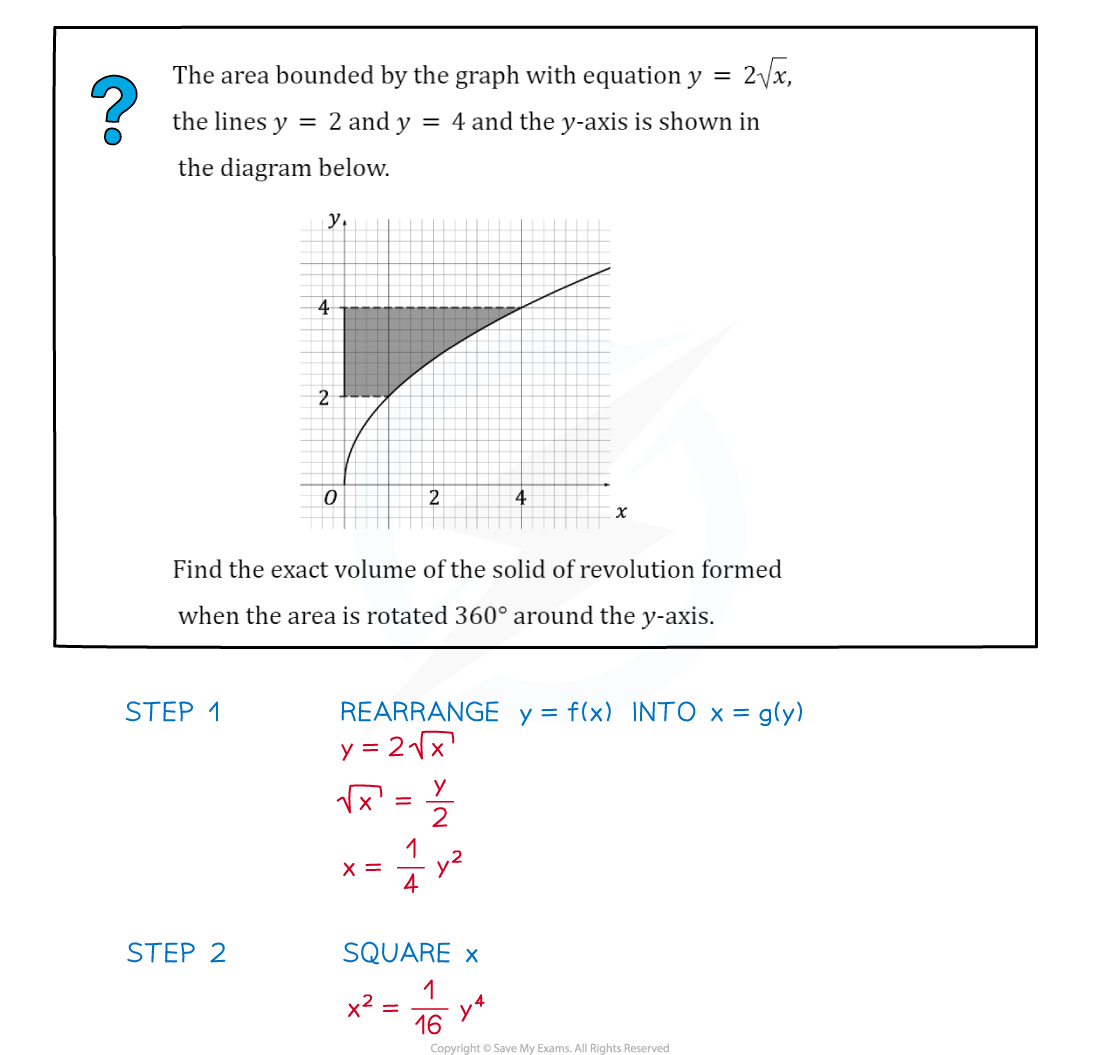
• STEP 1 Rearrange  into the form  (if necessary)

  • This is finding the inverse function 

• STEP 2 Square x

  • Do this first without worrying about π or the integration and limits

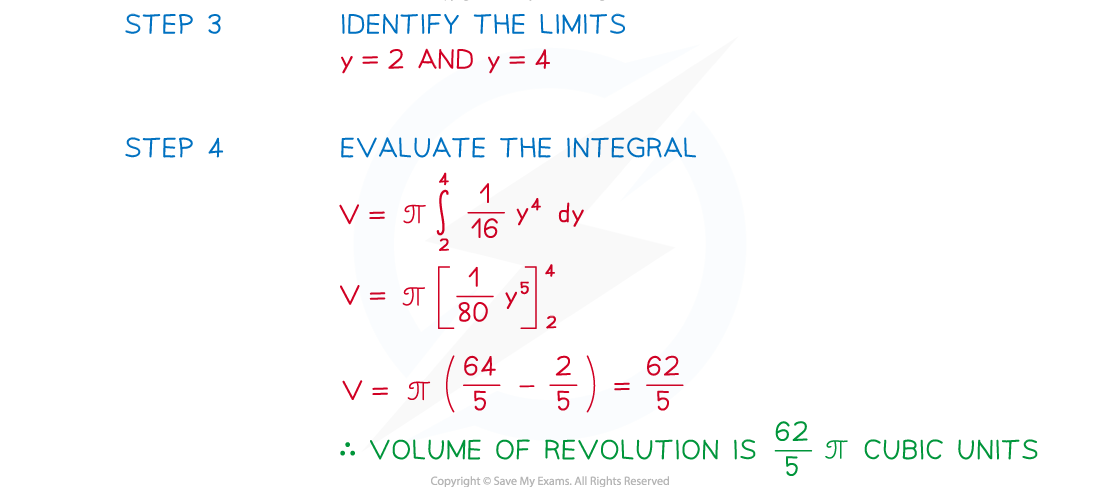
• STEP 3 Identify the limits c and d (which could come from a graph)

• STEP 4 Use the formula by evaluating the integral and multiplying by π

  • The answer may be required in exact form (leave in terms of π)

    • If not, round to three significant figures (unless told otherwise) 

• Trickier questions may give you the volume and ask for the value of an unknown constant elsewhere in the problem