A LevelMathsCambridge (CIE)Pure 3Revision NotesAlgebra & FunctionsPartial FractionsPartial Fractions with Quadratic Denominators

Partial Fractions with Quadratic Denominators (Cambridge (CIE) A Level Maths): Revision Note

Exam code: 9709

Last updated

Did this video help you?

Quadratic Denominators

What is meant by partial fractions with quadratic denominators?

  • For linear denominators the denominator of the original fraction can be factorised such that the denominator becomes a product of linear terms of the form left parenthesis a x space plus space b right parenthesis

  • With squared linear denominators, the same applies, except that some (usually just one) of the factors on the denominator may be squared, i.e. left parenthesis a x space plus space b right parenthesis squared

  • In both the above cases it can be shown that the numerators of each of the partial fractions will be a constant left parenthesis A comma space B comma space C comma space etc right parenthesis

  • For this course, quadratic denominators refer to fractions that have one linear factor and one quadratic factor (that cannot be factorised) on the denominator

    • the denominator of the quadratic partial fraction will be of the form left parenthesis a x squared space plus space b x space plus space c right parenthesis; very often b space equals space 0 leaving it as left parenthesis a x squared space plus space c right parenthesis

    • the numerator of the quadratic partial fraction could be of linear form, left parenthesis A x space plus space B right parenthesis

How do I find partial fractions involving quadratic denominators?

  •  STEP 1          Factorise the denominator as far as possible (if not already done so)

  • Sometimes the numerator can be factorised too

  • STEP 2          Split the fraction into a sum with

    • the linear denominator having an (unknown) constant numerator

    • the quadratic denominator having an (unknown) linear numerator

  • STEP 3          Multiply through by the denominator to eliminate fractions

  • STEP 4          Substitute values into the identity and solve for the unknown constants

    • Use the root of the linear factor as a value of x to find one of the unknowns

    • Use x space equals space 0 to find another one of the unknowns

    • Use any value of x (keep it small and simple) to find the final unknown

    • If the linear factor is then you'll need to use any two other values of  to form simultaneous equations

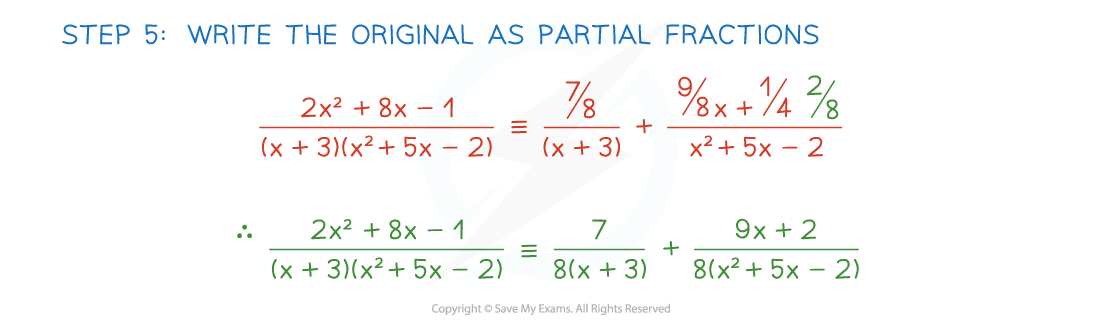
  • STEP 5          Write the original as partial fractions

  • In harder problems there may be more than one linear or quadratic factor

    • In such cases, values of x, whatever order they’re used in, will not always eliminate all but one of the unknowns

    • Simultaneous equations will need to be used

Worked Example

1-3-3-cie-fig1-we-solution-quad-den
1-3-3-cie-fig1-we-solution-quad-den_2

Examiner Tips and Tricks

  • You can check your final answer by substituting a value of x in to both the left and right-hand sides and seeing if they’re equal

    • Choose a small value of x  to keep things simple but not a value that would make a denominator zero

👀 You've read 1 of your 5 free revision notes this week
An illustration of students holding their exam resultsUnlock more revision notes. It's free!

By signing up you agree to our Terms and Privacy Policy.

Already have an account? Log in

Test yourself

Did this page help you?

Previous:Partial Fractions with Squared Linear DenominatorsNext:Further Modelling with Functions
Download notes on Partial Fractions with Quadratic Denominators