Particular Solutions (Cambridge (CIE) A Level Maths): Revision Note
Exam code: 9709
Did this video help you?
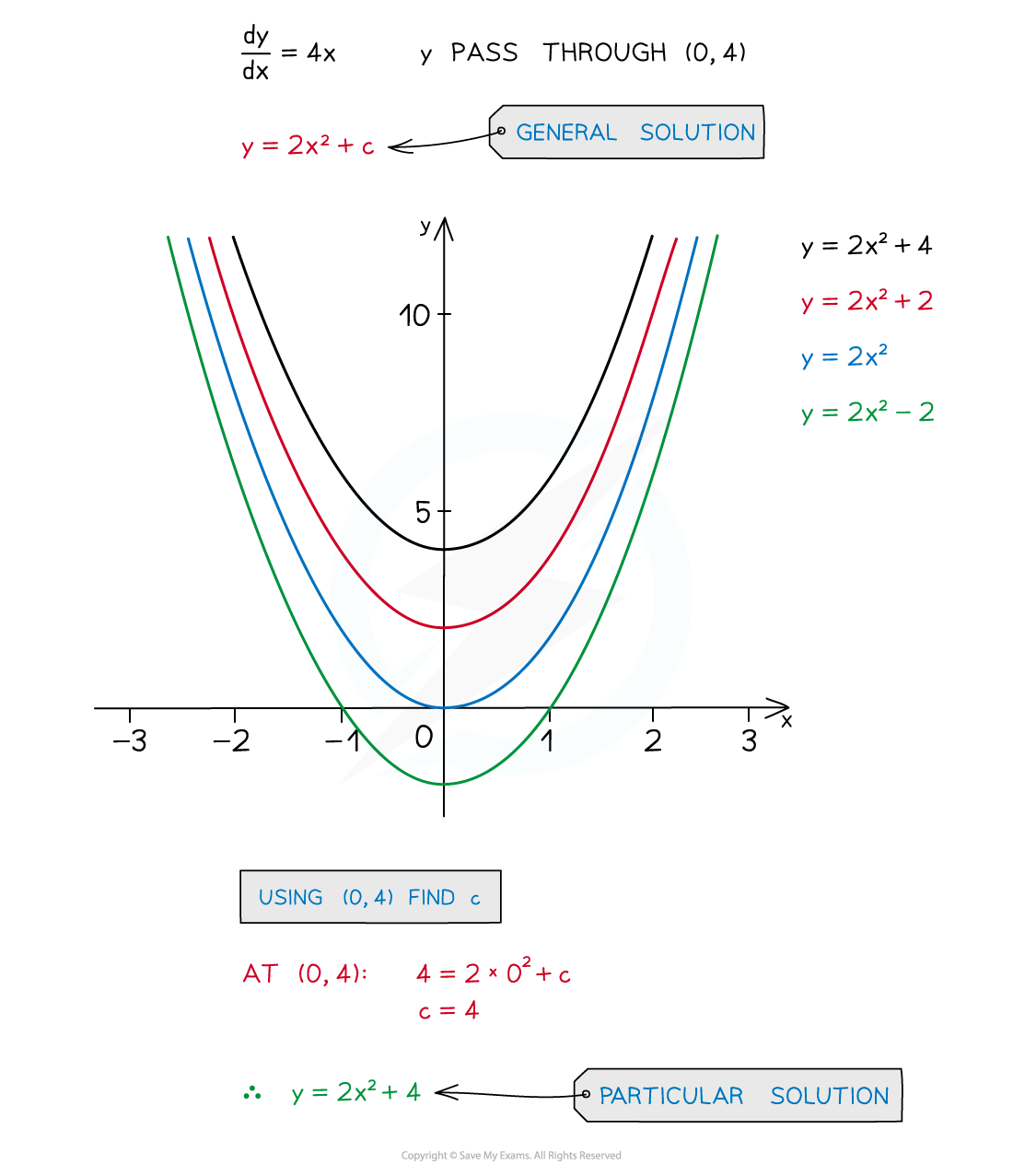
Particular solutions
What is a particular solution?
Ensure you are familiar with General Solutions first
With extra information, the constant of integration, c, can be found
This means the particular solution (from the family of solutions) can be found
What is a boundary condition and an initial condition?
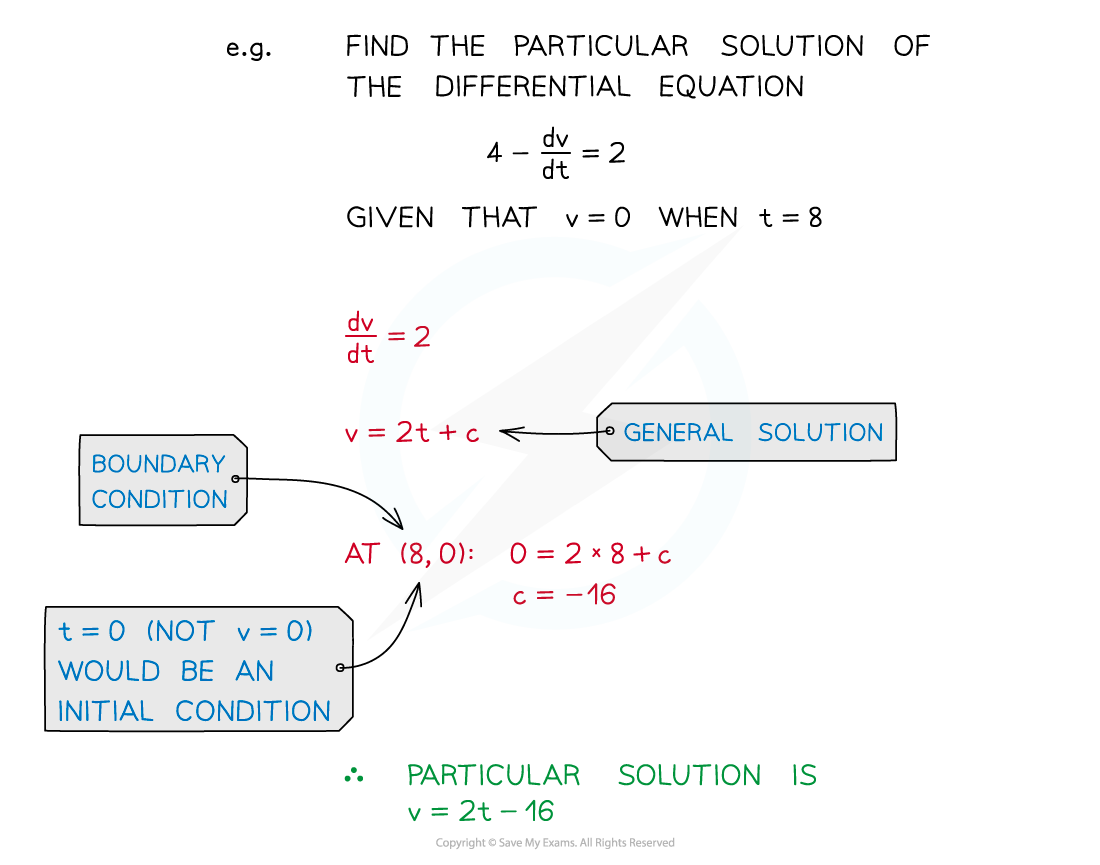
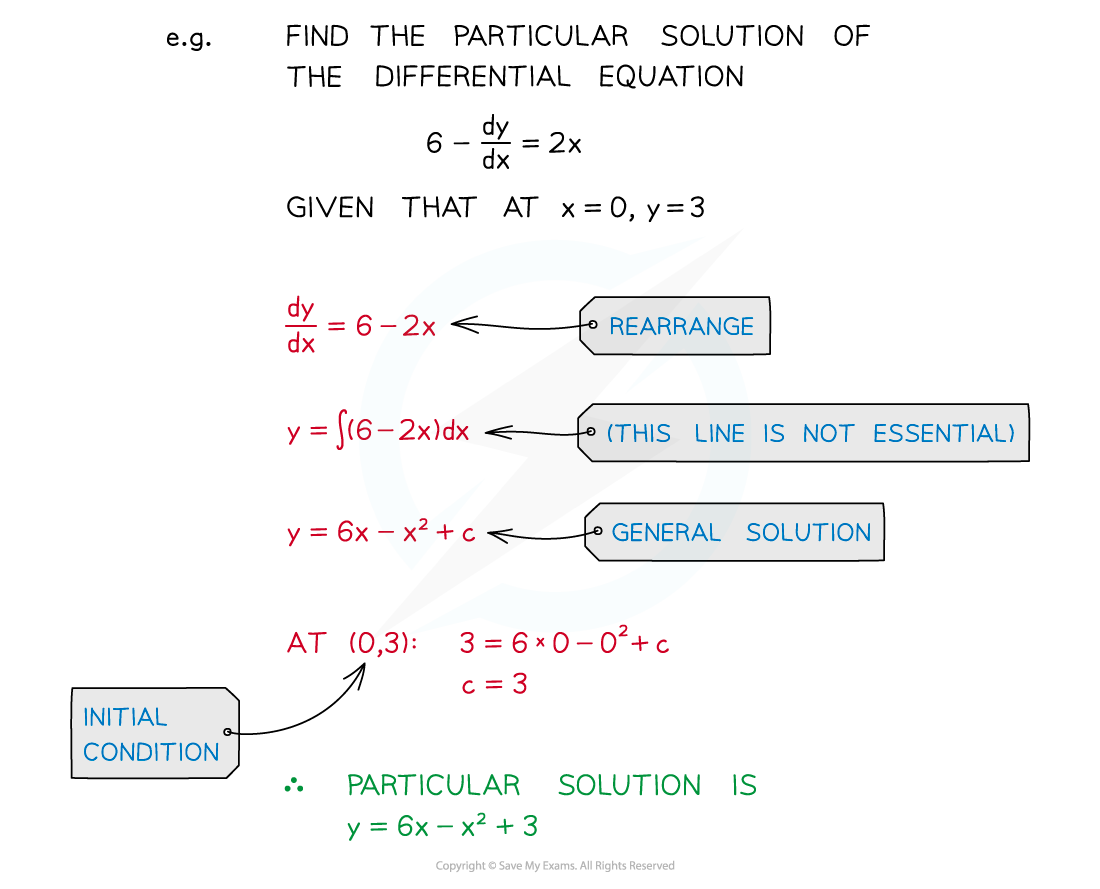
A boundary condition is a piece of extra information that lets you find the particular solution
For example knowing y = 4 when x = 0 in the preceding example
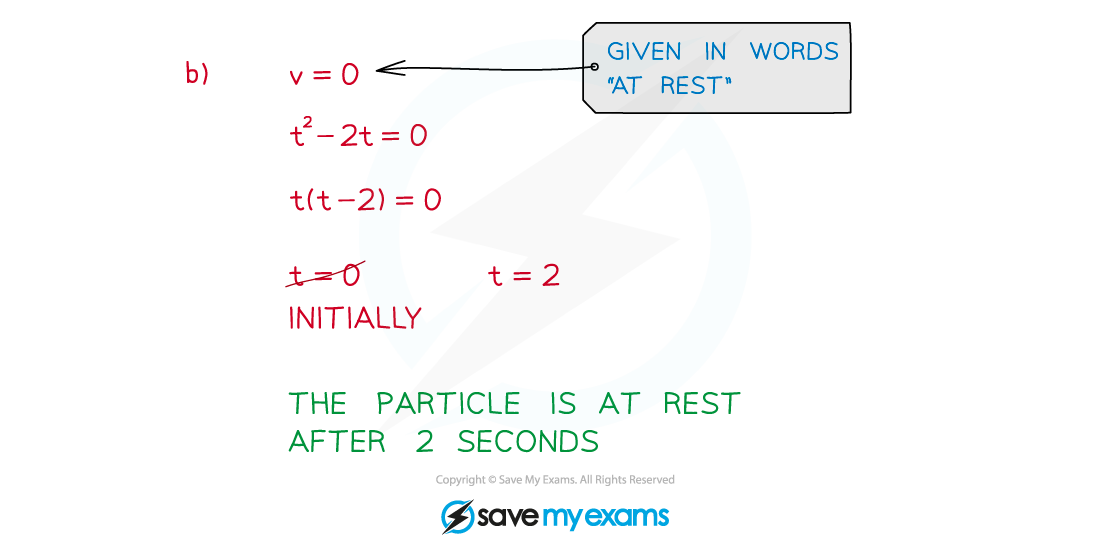
In a model this could be a particle coming to rest after a certain time, ie v = 0 at time t
Differential equations are used in modelling, experiments and real-life situations
A boundary condition is often called an initial condition when it gives the situation at the start of the model or experiment
This is often linked to time, so t = 0
It is possible to have two boundary conditions
eg a particle initially at rest has velocity, v = 0 and acceleration, a = 0 at time, t = 0
for a second order differential equation you need two boundary conditions to find the particular solution
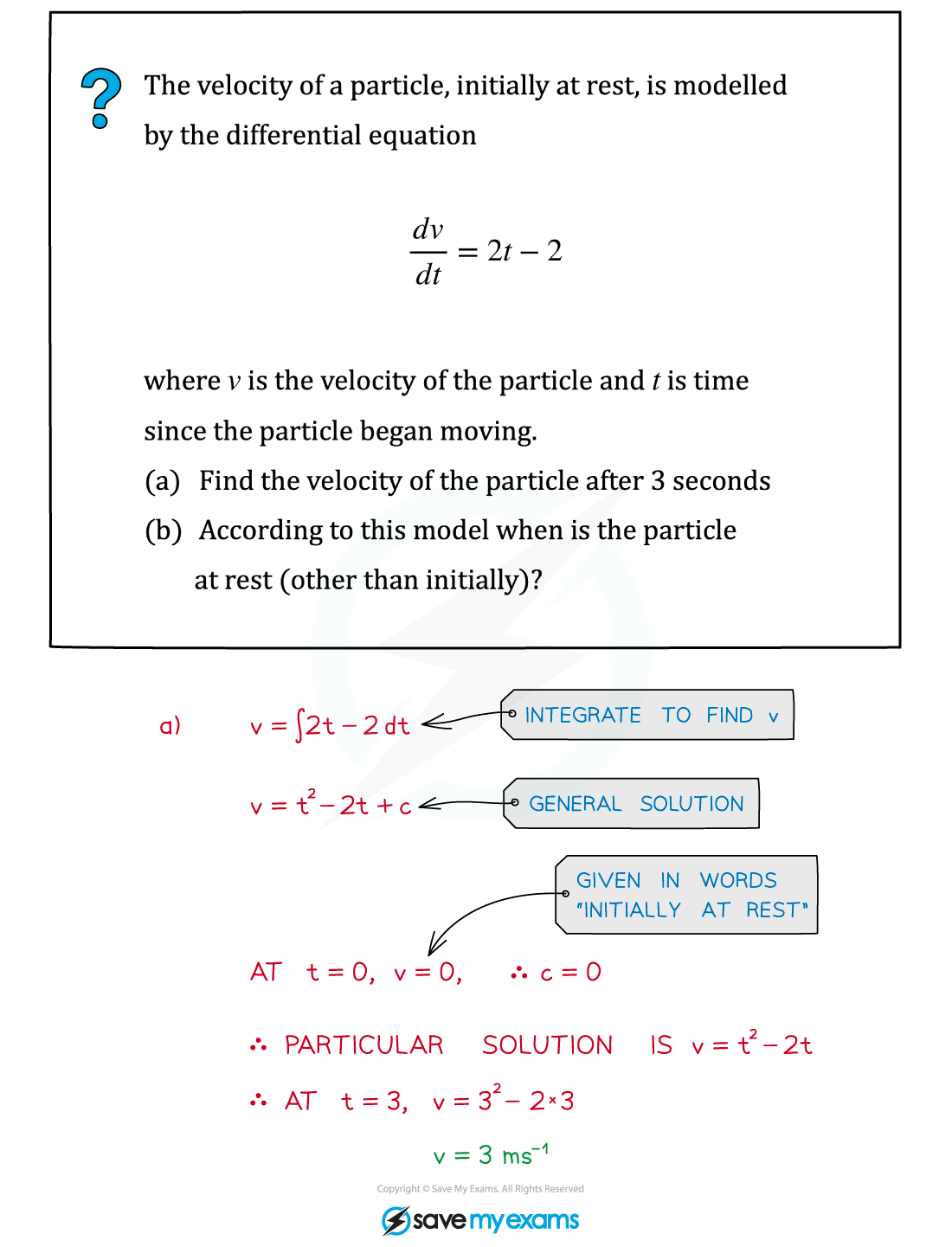
Worked Example

Unlock more, it's free!
Did this page help you?