Figure 1
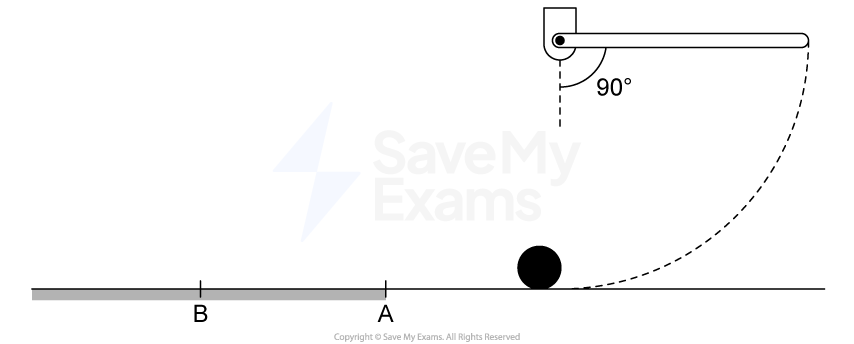
In Experiment 1, students release a disk of mass and radius
from rest at the top of a ramp that makes an angle
with the horizontal. The disk rolls without slipping, as shown in Figure 1. The rotational inertia of the disk is
. The frictional force exerted on the disk is
.
i) On the diagram in Figure 2, draw and label arrows that represent the forces (not components) that are exerted on the disk as it rolls down the ramp, which is indicated by the dashed line. Each force in your diagram must be represented by a distinct arrow starting on, and pointing away from, the point at which the force is exerted on the disk.

Figure 2
ii) Determine an expression for the net torque exerted on the disk around the center in terms of ,
,
,
and physical constants as appropriate.
iii) Determine an expression for the net force exerted on the disk in terms of ,
,
,
and physical constants as appropriate.
iv) Derive an expression for the translational acceleration of the center of mass of the disk. Express your answer in terms of ,
,
and physical constants as appropriate. Begin your derivation by writing a fundamental physics principle or an equation from the reference information.

Figure 3
In Experiment 1, the disk reaches the bottom of the ramp in time . In Experiment 2, the students release a block of ice from rest from the same height on a ramp inclined at the same angle
, as shown in Figure 3. Friction between the block and the ramp is negligible. The block reaches the bottom of the ramp in time
.
Indicate whether is greater than, less than, or equal to
.
⎽⎽⎽⎽⎽⎽⎽⎽⎽⎽ ⎽⎽⎽⎽⎽⎽⎽⎽⎽⎽
⎽⎽⎽⎽⎽⎽⎽⎽⎽⎽
Justify your reasoning.
Did this page help you?