IGCSEFurther MathsCambridge (CIE)Additional MathsRevision NotesAlgebra & FunctionsFactors of PolynomialsSolving Cubic Equations

Solving Cubic Equations (Cambridge (CIE) IGCSE Additional Maths): Revision Note

Exam code: 0606

Written by: Dan Finlay

Reviewed by: Lucy Kirkham

Updated on 2 July 2026

Solving cubic equations

What is a cubic equation?

A cubic function is an polynomial of degree 3
- i.e. the highest power of $x$ is 3
A cubic equation can be written in the form
- $a x^{3} + b x^{2} + c x + d = 0$
Solving a cubic equation involves factorising the cubic function first.

How do I factorise a cubic function?

Factorising a cubic (function) combines the factor theorem with the method of polynomial division
The example below shows the steps for factorising a cubic

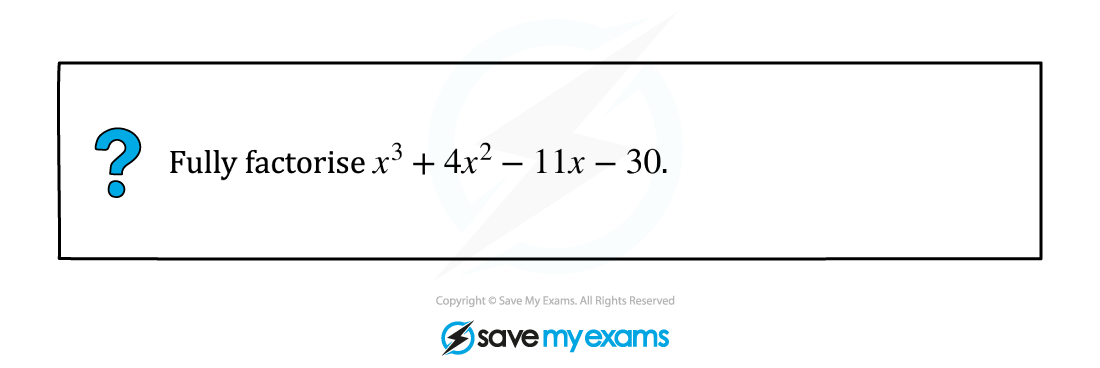
STEP 1
Use factor theorem.
Find a value $p$ such that $f (p) = 0$ .

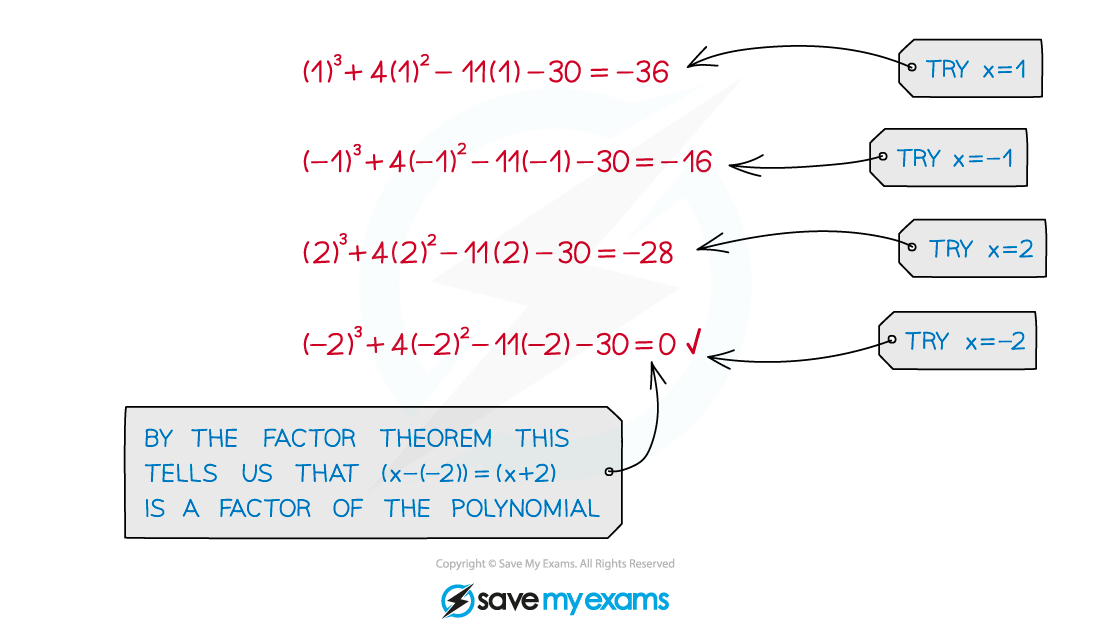
STEP 2
Use polynomial division.
Divide $f (x)$ by $(x - p)$ .
(It is possible to do this step 'by inspection', see the worked example below)

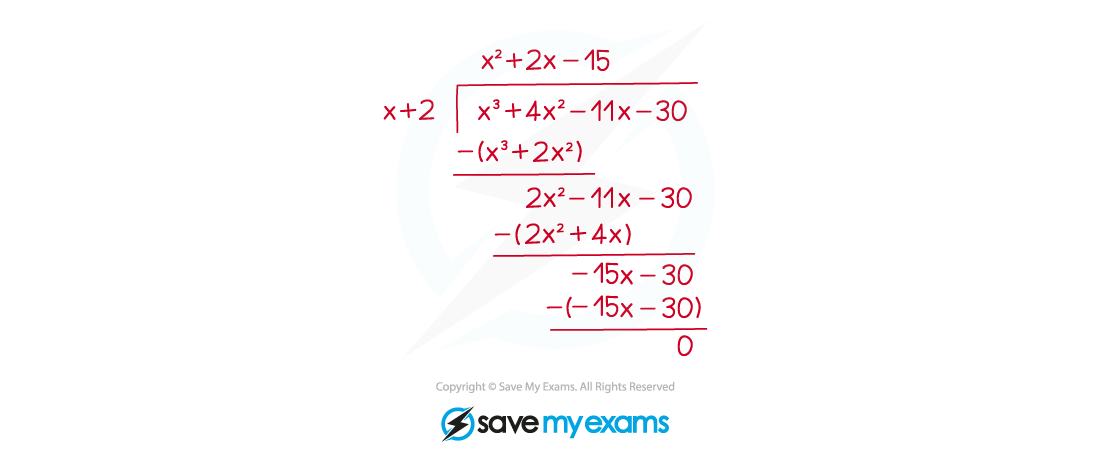
STEP 3
Use the result of your division to write $f (x) = (x - p) (a x^{2} + b x + c)$ .

STEP 4
If the quadratic $(a x^{2} + b x + c)$ can be factorised, do so.
$f (x)$ can then be written as the product of three linear factors.
If the quadratic cannot be factorised, then the result from STEP 3 is the final factorisation.

How do I solve a cubic equation?

A cubic equation will have either 1, 2 or 3 (real) solutions
- (The cubic function will have either 1, 2 or 3 (real) roots)
Once the cubic function is factorised using the four steps above, there is one more step to carry out

STEP 5 Find the solutions to the cubic equation by making each factor equal to zero

For each linear factor, $(x - p)$
- $x - p = 0$
- so $x = p$ is a solution
- This is the factor theorem!
- For a quadratic factor, $(a x^{2} + b x + c)$
  - $a x^{2} + b x + c = 0$
  - use either the quadratic formula or completing the square (as it won't factorise)
    - this will give two of the solutions to the cubic equation
  - if there are no solutions to the quadratic equation there are no solutions other than that from the linear factor

From the example above,
- $x^{3} + 4 x^{2} - 11 x - 30 = (x + 2) (x + 5) (x - 3)$
- so the solutions to the cubic equation $x^{3} + 4 x^{2} - 11 x - 30 = 0$ are
  - $x = - 2, x = - 5$ and $x = 3$
Cubic equations can have equal (repeated) solutions
- e.g. ${(x - 2)}^{2} (x + 1)$ has two (equal and real) roots, $x = 2$ (repeated) and $x = - 1$
- e.g. ${(2 x - 1)}^{3}$ has three (equal and real) roots, $x = \frac{1}{2}$

Examiner Tips and Tricks

When $d = 0$ (i.e. there is no constant term) then $x$ is a factor of the cubic function, and so $x = 0$ is a solution
- This is a special case of factor theorem, where $f (0) = 0$
  - spotting the factor of $x$ means there is no need to test values
- Take out a factor of $x$ and a quadratic function will remain
- Deal with the quadratic in any of the usual ways

Worked Example

a) Solve the cubic equation $x^{3} - 10 x^{2} + 12 x + 8 = 0$ .

STEP 1 - use factor theorem with $f (x) = x^{3} - 10 x^{2} + 12 x + 8$

$f (1) = {(1)}^{3} - 10 {(1)}^{2} + 12 (1) + 8 = 11 \neq 0$

$f (- 1) = {(- 1)}^{3} - 10 {(- 1)}^{2} + 12 (- 1) + 8 = - 15 \neq 0$

$f (2) = {(2)}^{3} - 10 {(2)}^{2} + 12 (2) + 8 = 0$

$∴ (x - 2)$ is a factor of $f (x)$

STEP 2 - polynomial division ( $f (x) \div (x - 2)$ ) or 'by inspection' By inspection ...

$f (x) = (x - 2) (a x^{2} + b x + c)$

('cubic' ÷ 'linear' = 'quadratic')

$a = 1$

(because the $x^{3}$ is generated only from $x \times a x^{2}$ )

$c = - 4$

(because the constant term is generated only from $- 2 \times c$ )

Equate coefficients of $x$ (or $x^{2}$ ) terms to find $b$ ,

$12 = c - 2 b$

$b = \frac{- 4 - 12}{2} = - 8$

STEP 3

$f (x) = (x - 2) (x^{2} - 8 x - 4)$

STEP 4 - the quadratic does not factorise

STEP 5 - Use the factors to find the solutions

$\begin{array}{rcl} x - 2 & = & 0, x = 2 \end{array}$

$x^{2} - 8 x - 4 = 0, x = \frac{8 \pm \sqrt{64 + 16}}{2}, x = 4 \pm 2 \sqrt{5}$

(using the quadratic formula)

The solutions to $x^{3} - 10 x^{2} + 12 x + 8 = 0$ are $x = 2, x = 4 + 2 \sqrt{5}$ and $x = 4 - 2 \sqrt{5}$ .

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

Beth
IGCSE Student

This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

Fathima
A Level Student

Incredible! SO worth my money, the revision notes have everything I need to know and are so easy to understand. I actually enjoy revising! It makes me feel a lot more confident for my GCSEs in a few months.

Kate
GCSE Student

Absolutely brilliant, both my girls used it for A levels and GCSE. It's saves on paper copies, also beneficial exam questions ranked from easy to hard. It's removed a lot of stress from the exams.

Sameera
Parent

Just to say that your resources are the best I have seen and I have been teaching chemistry at different levels for about 40 years

Mark
Chemistry Teacher

Excellent