Resolving Vectors (Edexcel International A Level (IAL) Physics): Revision Note
Exam code: YPH11
Resolving Vectors
Vectors are represented by an arrow
The arrowhead indicates the direction of the vector
The length of the arrow represents the magnitude
Component vectors are sometimes drawn with a dotted line and a subscript indicating horizontal or vertical for example Fv is the vertical component of the force F
Resolving Vectors
A single resultant vector can be resolved
This means it can be represented by two vectors, which in combination have the same effect as the original one
Resolving vectors makes calculating much easier when working with projectiles or any motion or force at an angle
When a single resultant vector is broken down into its parts, those parts are called components
A vector of magnitude F at angle theta to the horizontal is shown

Resolving Vectors by Scale Drawing
To resolve vectors by scale drawing means carefully producing a scale drawing with all lengths and angles correct
This should be done using a sharp pencil, ruler and protractor
Follow these steps
Choose a scale which fits to the page. For example, if resolving a resultant displacement of 100 m, use 1 cm = 10 m so that the resultant drawing is around 10 cm high
Clearly mark the starting point and a line to represent either the vertical or horizontal direction. This will depend on the angle given in the question, for example if the direction is '30o east of north' then start with a vertical line representing north
Carefully measure the angle and start by drawing the resultant vector
Draw a triangle using two of the sides of this rectangle
Worked Example
A hiker walks a distance of 6 km due east and 10 km due north.
By making a scale drawing of their route, find the magnitude of their displacement and its direction from the horizontal.
Answer:
Step 1: Choose a sensible scale
The distances are 6 and 10 km, so a scale of 1 cm = 1 km will fit easily on the page, but be large enough for an accurate scale drawing
Step 2: Draw the two components using a ruler and making the measurements accurate to 1 mm
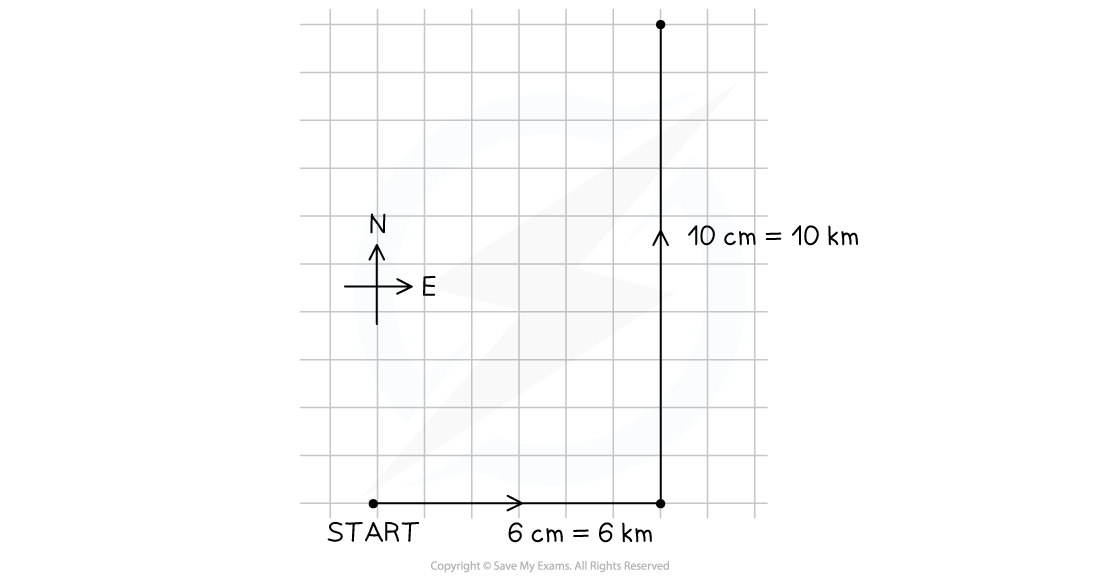
Step 3: Add the resultant vector, remembering the start and finish points of the journey

Step 4: Carefully measure the length of the resultant and convert using the scale

Step 5: Measure the angle between the vector and the horizontal line

Step 6: Write the complete answer, giving both magnitude and direction
R = 11.7 = 12 km
θ = 59°east and upwards from the horizontal
Resolving Vectors by Calculation
In this method a diagram is still essential but it does not need to be exactly to scale
The diagram can take the form of a sketch, as long as the resultant, component and sides are clearly labelled
Use trigonometry to resolve the two sides
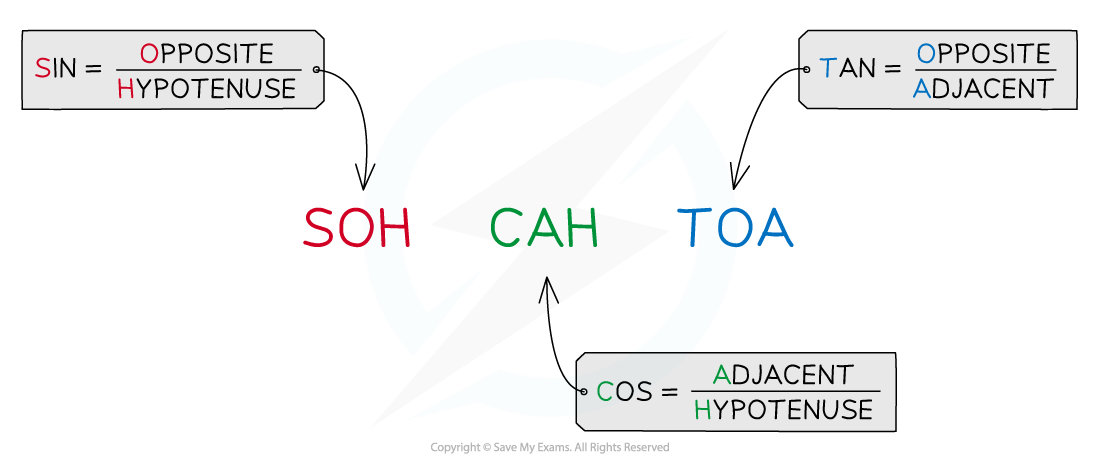
The mnemonic 'soh-cah-toa' is used to remember how to apply sines and cosines to resolving sides of a triangle
It is possible to resolve this vector into its horizontal and vertical components using trigonometry

The resultant force F can be split into its horizontal and vertical components
For the horizontal component, Fx = F cos θ
For the vertical component, Fy = F sin θ
Worked Example
A hiker walks a distance of 6 km due east and 10 km due north.
Calculate the magnitude of their displacement and its direction from the horizontal.
Answer:


Step 4: State the final answer complete with direction
R = 2√34 = 11.66 = 12 km
θ = 59°east and upwards from the horizontal
Examiner Tips and Tricks
Did you notice that the two worked examples above were the same question, being solved in two different ways?
If the question specifies calculation or scale drawing you must solve the problem as asked. However, if the choice is left up to you then any correct method will lead to the correct answer.
Scale drawing sometimes feels easier than calculating, but once you are confident with trigonometry and Pythagoras you will find calculating quicker and more accurate.
A quick tip to remember whether to use sin or cos is that when the resultant is closing down onto the angle, use cos.


You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?