Uncertainties (Edexcel International A Level (IAL) Physics): Revision Note

Uncertainties

• Uncertainties can be represented in a number of ways:

  • Absolute Uncertainty: where uncertainty is given as a fixed quantity

  • Fractional Uncertainty: where uncertainty is given as a fraction of the measurement

  • Percentage Uncertainty: where uncertainty is given as a percentage of the measurement

• Percentage uncertainty is defined by the equation:

Percentage uncertainty = $\frac{uncertainty}{measuredvalue}$× 100 %

• To find uncertainties in different situations:

• The uncertainty in a reading: ± half the smallest division

• The uncertainty in a measurement: at least ±1 smallest division

• The uncertainty in repeated data: half the range i.e. ± ½ (largest - smallest value)

• The uncertainty in digital readings: ± the last significant digit unless otherwise quoted

How to calculate absolute, fractional and percentage uncertainty

• Always make sure your absolute or percentage uncertainty is, at a maximum, to the same number of significant figures as the reading

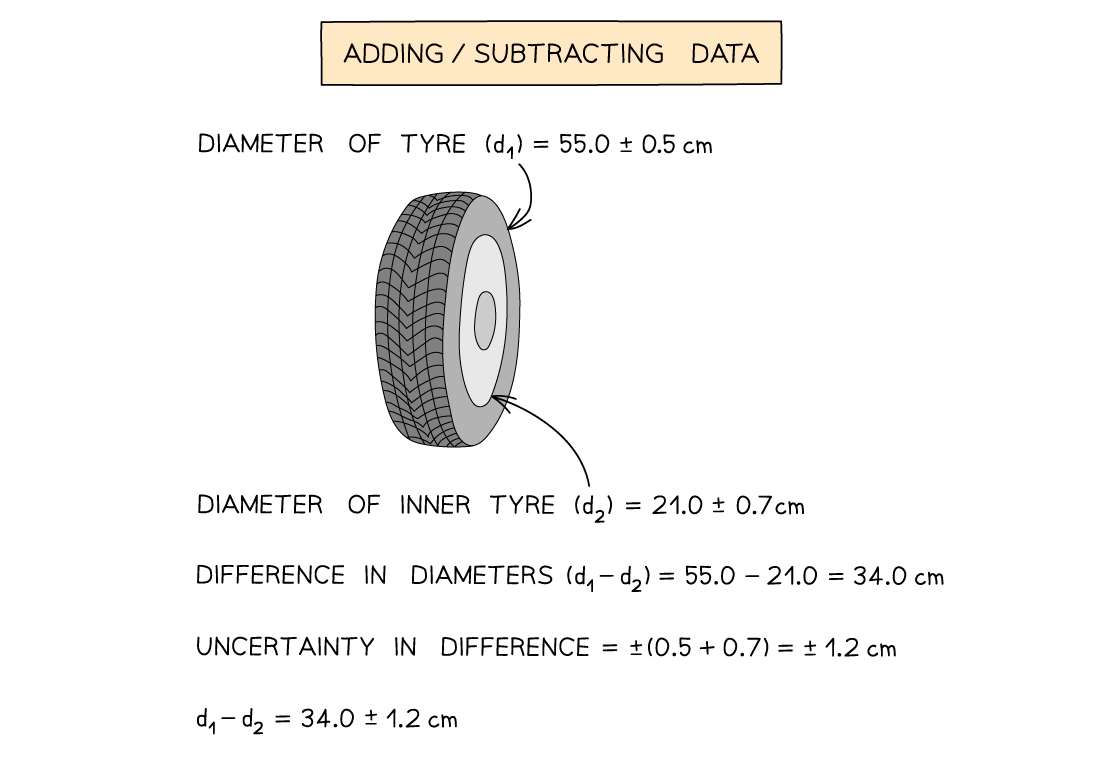
• Absolute uncertainties are compounded when adding or subtracting data 

Adding / Subtracting Data

• Add together the absolute uncertainties