IBMathsDPApplications & Interpretation (AI)HLRevision Notes1. Number & AlgebraExponentials & LogsExponents

Exponents (DP IB Applications & Interpretation (AI)): Revision Note

Last updated

DP Applications & Interpretation (AI): HLDP Analysis & Approaches (AA): HLDP Analysis & Approaches (AA): SL

Did this video help you?

Laws of Indices

What are the laws of indices?

  • Laws of indices (or index laws) allow you to simplify and manipulate expressions involving exponents

    • An exponent is a power that a number (called the base) is raised to

    • Laws of indices can be used when the numbers are written with the same base

  • The index laws you need to know are:

    • left parenthesis x y right parenthesis to the power of m equals x to the power of m end exponent y to the power of m

    • stretchy left parenthesis x over y stretchy right parenthesis to the power of m equals x to the power of m over y to the power of m

    • x to the power of m cross times x to the power of n equals x to the power of m plus n end exponent

    • x to the power of m divided by x to the power of n equals x to the power of m minus n end exponent

    • stretchy left parenthesis x to the power of m stretchy right parenthesis to the power of n equals x to the power of m n end exponent

    • x to the power of 1 equals x

    • x to the power of 0 equals 1

    • 1 over x to the power of m equals x to the power of negative m end exponent

    • x to the power of 1 over n end exponent equals blank n-th root of x

    • x to the power of m over n end exponent equals blank n-th root of x to the power of m end root

  • These laws are not in the formula booklet so you must remember them

How are laws of indices used?

  • You will need to be able to carry out multiple calculations with the laws of indices

    • Take your time and apply each law individually

    • Work with numbers first and then with algebra

  • Index laws only work with terms that have the same base, make sure you change the base of the term before using any of the index laws

    • Changing the base means rewriting the number as an exponent with the base you need

    • For example, 9 to the power of 4 equals left parenthesis 3 squared right parenthesis to the power of 4 equals 3 to the power of 2 cross times 4 end exponent equals 3 to the power of 8

    • Using the above can them help with problems like 9 to the power of 4 divided by 3 to the power of 7 equals 3 to the power of 8 divided by 3 to the power of 7 equals 3 to the power of 1 equals 3

Examiner Tips and Tricks

  • Index laws are rarely a question on their own in the exam but are often needed to help you solve other problems, especially when working with logarithms or polynomials

  • Look out for times when the laws of indices can be applied to help you solve a problem algebraically 

Worked Example

Simplify the following equations:

i) fraction numerator left parenthesis 3 x squared right parenthesis left parenthesis 2 x cubed y squared right parenthesis over denominator left parenthesis 6 x squared y right parenthesis end fraction.

 

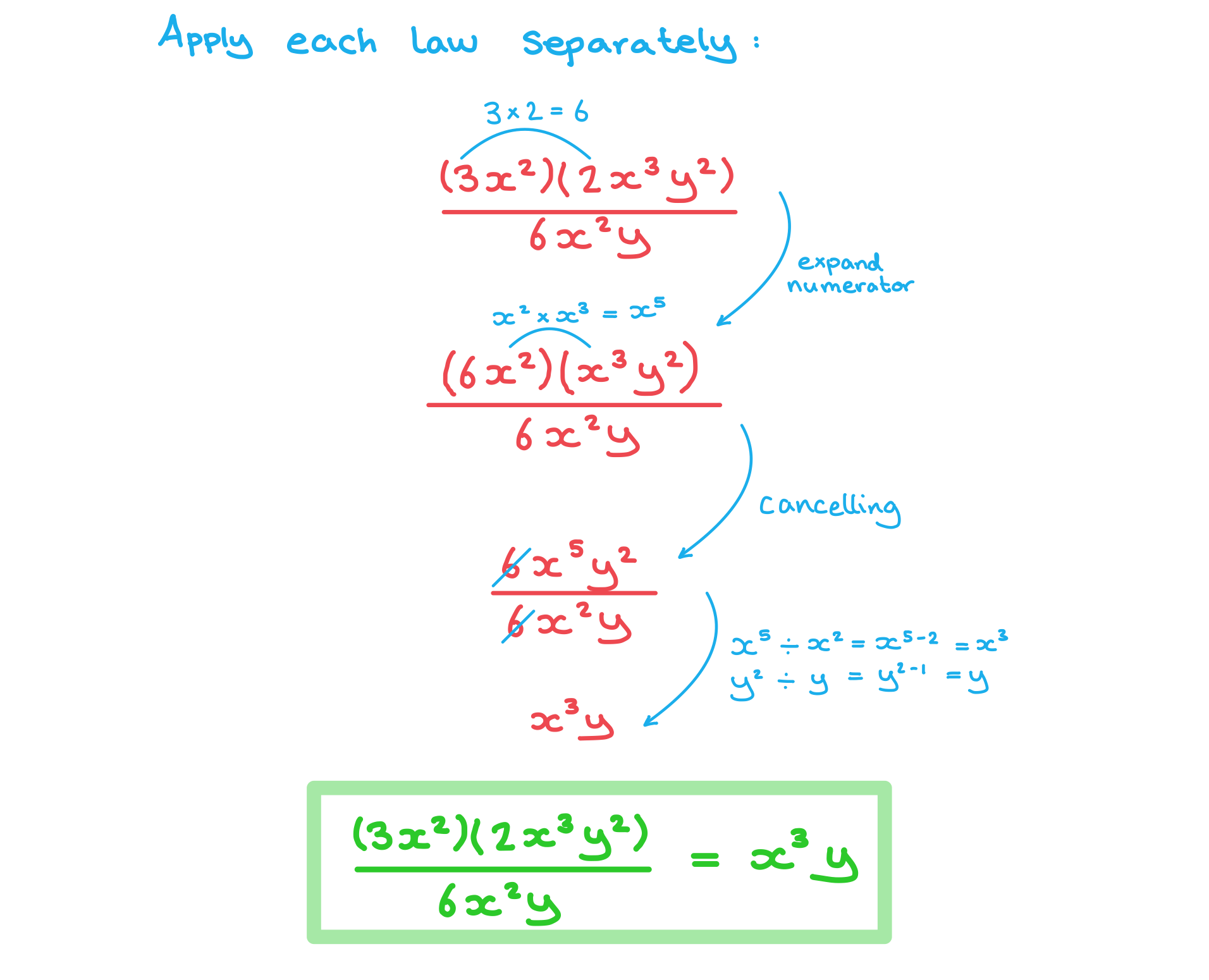
ai-sl-1-1-2-laws-of-indices-we-i

ii) left parenthesis 4 x squared y to the power of negative 4 end exponent right parenthesis cubed left parenthesis 2 x cubed y to the power of negative 1 end exponent right parenthesis to the power of negative 2 end exponent.

 

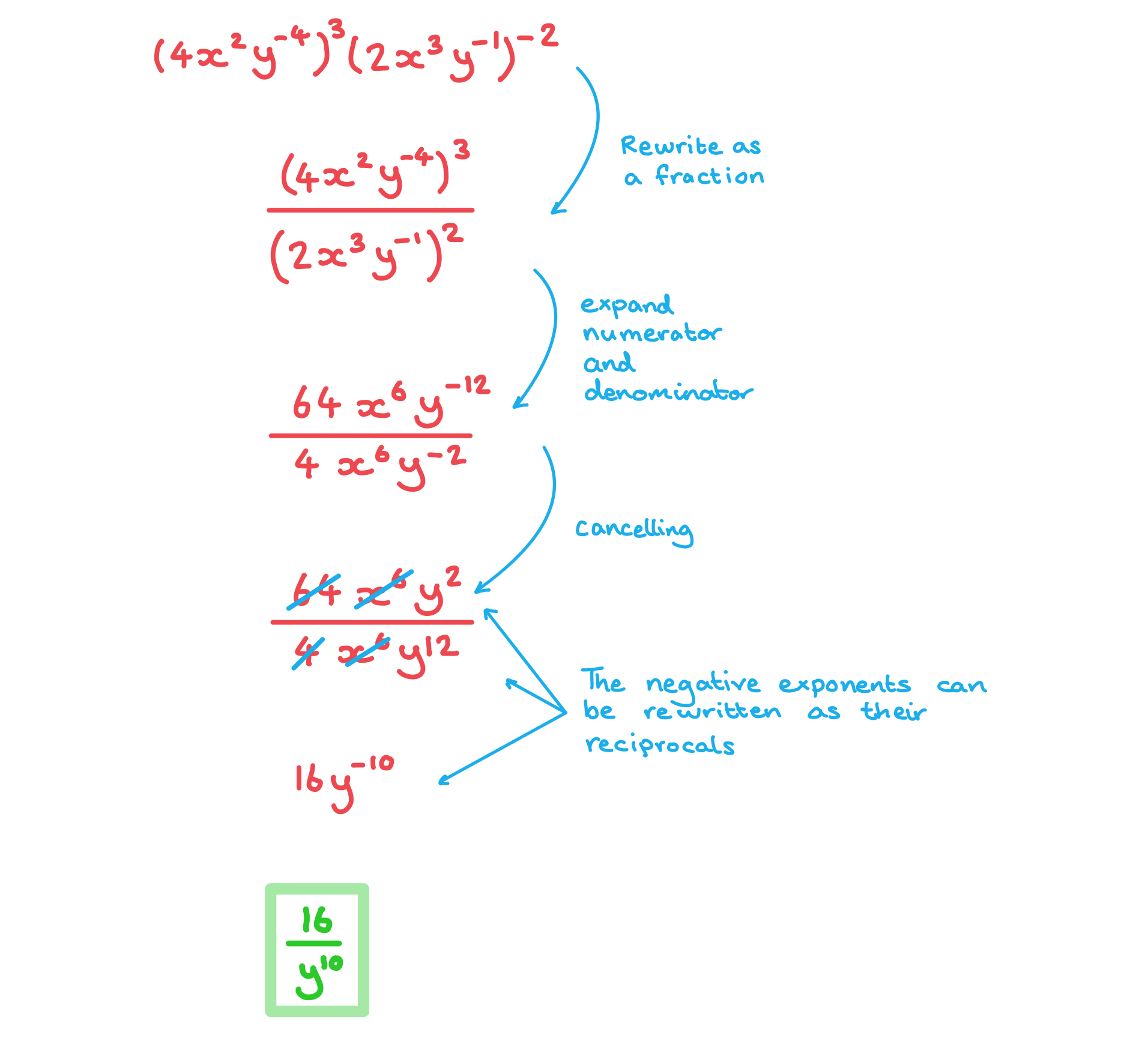
ai-sl-1-1-2-laws-of-indices-we-ii


You've read 1 of your 5 free revision notes this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

  • I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

    Beth
    IGCSE Student

  • This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

    Fathima
    A Level Student
Test yourselfFlashcards

Did this page help you?

Previous:Solving Equations using a GDCNext:Logarithms
Download notes on Exponents