GCSEPhysicsAQARevision NotesForcesDescribing MotionAcceleration

Acceleration (AQA GCSE Physics): Revision Note

Exam code: 8463

Ashika

Written by: Ashika

Reviewed by: Caroline Carroll

Updated on

AQA PhysicsAQA Combined Science: Synergy: Physical SciencesAQA Combined Science: Trilogy: Physics

Acceleration

  • Acceleration is defined as

the rate of change of velocity

  • In other words, it describes how much an object's velocity changes every second

  • The equation below is used to calculate the average acceleration of an object:

acceleration space equals space fraction numerator change space in space velocity over denominator time space taken end fraction

a space equals space fraction numerator increment v over denominator t end fraction

  • Where:

    • a = acceleration in metres per second squared (m/s2)

    • increment v = change in velocity in metres per second (m/s)

    • t = time taken in seconds (s)

  • The change in velocity is found by the difference between the initial and final velocity, as written below:

change in velocity = final velocity − initial velocity

increment v space equals space v space minus space u

  • Where:

    • v = final velocity in metres per second (m/s)

    • u = initial velocity in metres per second (m/s)

  • The equation for acceleration can be rearranged with the help of a formula triangle as shown:

Formula triangle for acceleration, change in velocity and time: change in velocity (Δv) at the top, acceleration (a) and time (t) at the bottom
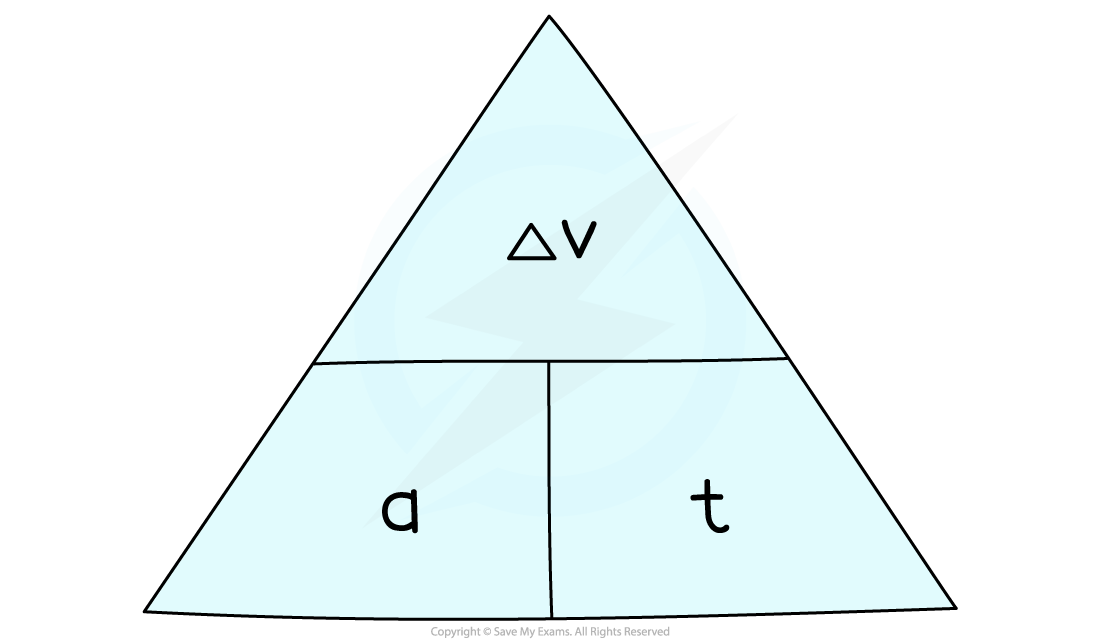
Acceleration, change in velocity, and time formula triangle

Speeding Up and Slowing Down

  • An object that speeds up is accelerating

  • An object that slows down is decelerating

  • The acceleration of an object can be positive or negative, depending on whether the object is speeding up or slowing down

    • If an object is speeding up, its acceleration is positive

    • If an object is slowing down, its acceleration is negative (sometimes called deceleration)

  • Two examples of accelerating and decelerating objects are shown in the image below

Two examples of acceleration: a rocket speeding up (positive acceleration) and a car braking (negative acceleration/deceleration), with arrows indicating direction of motion and velocity change
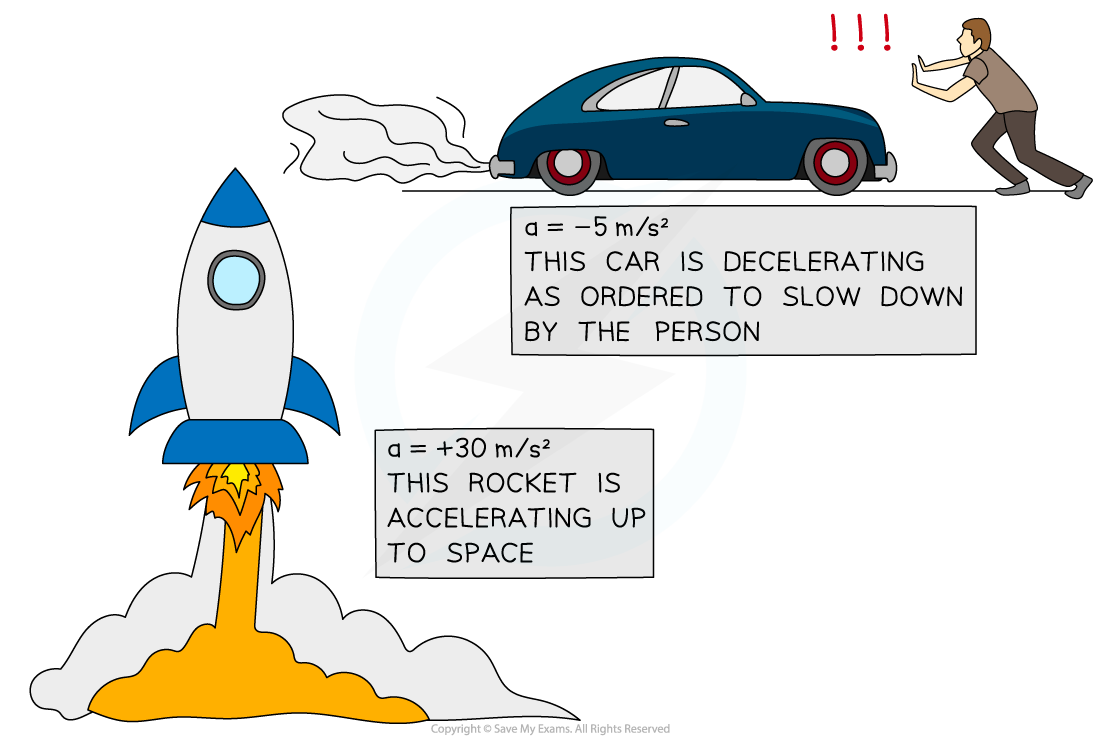
A rocket speeding up (accelerating) and a car slowing down (decelerating)

Worked Example

A Japanese bullet train decelerates at a constant rate in a straight line. The velocity of the train decreases from 50 m/s to 42 m/s in 30 seconds.

(a) Calculate the change in velocity of the train.

(b) Calculate the deceleration of the train, and explain how your answer shows the train is slowing down.

Answer:

Part (a)

Step 1: List the known quantities

  • Initial velocity, u space equals space 50 space straight m divided by straight s

  • Final velocity, v space equals space 42 space straight m divided by straight s

Step 2: Write down the relevant equation

change in velocity = final velocity − initial velocity

increment v space equals space v space minus space u

Step 3: Substitute values for final and initial velocity

increment v space equals space 42 space minus space 50 space equals space minus 8 space straight m divided by straight s

  • The velocity of the train decreases by 8 m/s

Part (b)

Step 1: List the known quantities

  • Change in velocity, increment v space equals space minus 8 space straight m divided by straight s

  • Time taken, t space equals space 30 space straight s

Step 2: Write down the relevant equation

acceleration space equals space fraction numerator change space in space velocity over denominator time space taken end fraction

a space equals space fraction numerator increment v over denominator t end fraction

Step 3: Substitute the values for change in velocity and time

a space equals space fraction numerator negative 8 over denominator 30 end fraction space equals space minus 0.27 space straight m divided by straight s squared

Step 4: Interpret the value for deceleration

  • The answer is negative, which indicates the train is slowing down

Examiner Tips and Tricks

Remember the units for acceleration are metres per second squared, m/s2

In other words, acceleration measures how much the velocity (in m/s) changes every second, m/s/s.

Estimating Accelerations

  • The acceleration of an object is a measure of how quickly its velocity changes

  • A typical family car, for example, takes around 10 seconds to go from 0 m/s to 27 m/s (roughly 60 mph)

    • This is an acceleration of about 2.7 m/s2

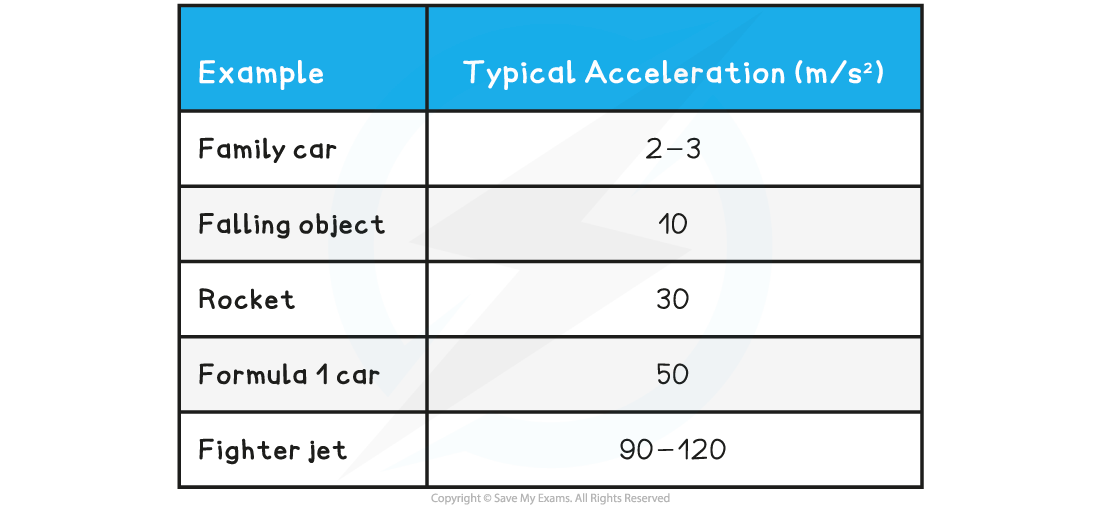
    • The table below gives some other typical accelerations:

Example

Typical acceleration (m/s2)

Family car

2 - 3

Falling object

10

Rocket

30

Formula 1 car

50

Fighter jet

90 - 120

Examiner Tips and Tricks

You should be able to estimate the magnitude of everyday accelerations. Memorise the examples given in the table to develop a sense of the magnitude of different accelerating objects.

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

Beth
IGCSE Student

This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

Fathima
A Level Student

Incredible! SO worth my money, the revision notes have everything I need to know and are so easy to understand. I actually enjoy revising! It makes me feel a lot more confident for my GCSEs in a few months.

Kate
GCSE Student

Absolutely brilliant, both my girls used it for A levels and GCSE. It's saves on paper copies, also beneficial exam questions ranked from easy to hard. It's removed a lot of stress from the exams.

Sameera
Parent

Just to say that your resources are the best I have seen and I have been teaching chemistry at different levels for about 40 years

Mark
Chemistry Teacher

Excellent

Our rating represented in Trustpilot stars
Read more reviews

Build on this topic

Check your understandingTry an Exam Question on this topicTest your recallReinforce key facts and definitions with Flashcards
Previous:Instantaneous SpeedNext:Velocity-Time Graphs
Author
Reviewer
Ashika

Author: Ashika

Expertise: Physics Content Creator

Ashika graduated with a first-class Physics degree from Manchester University and, having worked as a software engineer, focused on Physics education, creating engaging content to help students across all levels. Now an experienced GCSE and A Level Physics and Maths tutor, Ashika helps to grow and improve our Physics resources.

Caroline Carroll

Reviewer: Caroline Carroll

Expertise: Head of Content Delivery

Caroline graduated from the University of Nottingham with a degree in Chemistry and Molecular Physics. She spent several years working as an Industrial Chemist in the automotive industry before retraining to teach. Caroline has over 12 years of experience teaching GCSE and A-level chemistry and physics. She is passionate about delivering high-quality resources to help students achieve their full potential.