Critical Angle (Edexcel International A Level (IAL) Physics): Revision Note
Exam code: YPH11
Critical Angle
As the angle of incidence at the boundary between a more dense and a less dense medium is increased, the angle of refraction also increases until it gets to 90°
When the angle of refraction is exactly 90° the light is refracted along the boundary (if the boundary is straight)
At this point, the angle of incidence is known as the critical angle C
This can only occur when light passes from a more dense to a less dense material
This angle can be found using the formula:
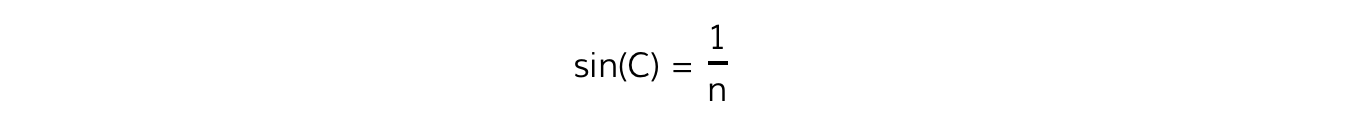
This can easily be derived from Snell’s law:
n1 sin θ1 = n2 sin θ2
Where:
θ1 = C
θ2 = 90°
n1 = n
n2 = 1 (air)
Worked Example
A glass cube is held in contact with a liquid and a light ray is directed at the vertical face of the cube. The angle of incidence at the vertical face is 39° and the angle of refraction is 25° as shown in the diagram. The light ray is totally internally reflected at X. The refractive index of the glass cube is 1.45 and the refractive index of the liquid is 1.32.
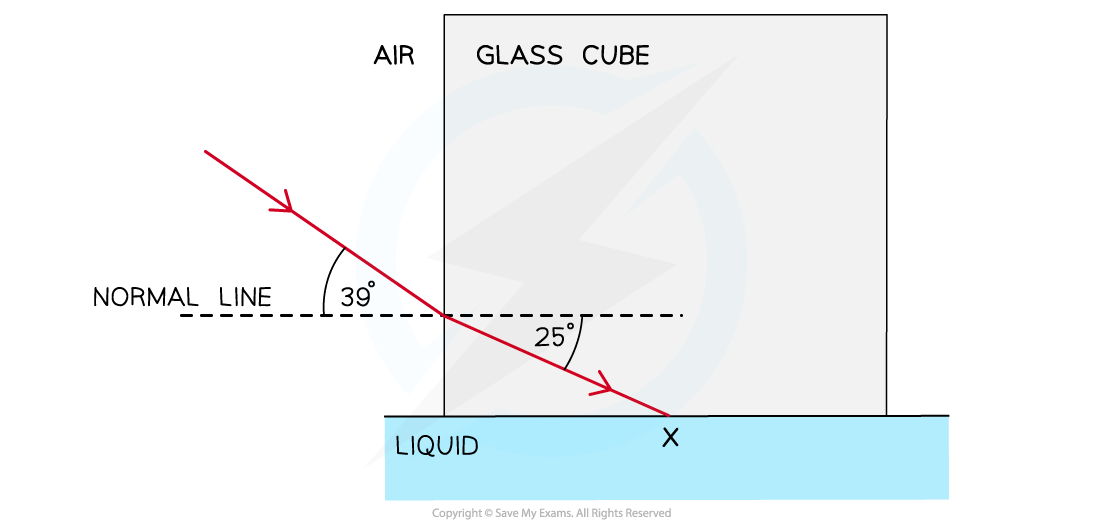
a) Complete the diagram to show the path of the ray beyond X
b) Calculate the critical angle for the ray at the glass-liquid boundary
Answer:
Part (a)
Complete the diagram to show the path of the ray beyond X
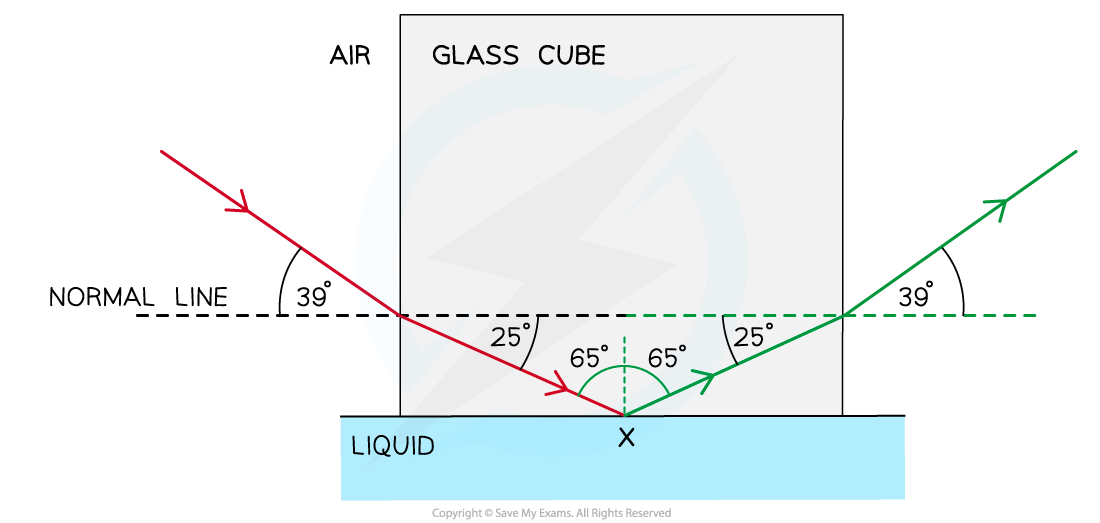
Step 1: Draw the reflected angle at the glass-liquid boundary
When a light ray is reflected, the angle of incidence = angle of reflection
Therefore, the angle of incidence (and reflection) is 90° – 25° = 65°
Step 2: Draw the refracted angle at the glass-air boundary
At the glass-air boundary, the light ray refracts away from the normal
Due to the reflection, the light rays are symmetrical to the other side
Part (b)
Calculate the critical angle for the ray at the glass-liquid boundary:
Step 1: Recall Snell's Law and rearrange to make critical angle the subject
n1 sin θ1 = n2 sin θ2
Step 2: Substitute in the known quantities
n1 = refractive index of glass cube = 1.45
n2 = refractive index of liquid = 1.32
θ1 = C
θ2 = 90° (The angle of refraction is 90° when at the critical angle)
Step 3: Calculate the critical angle
Examiner Tips and Tricks
Always draw ray diagrams with a ruler, and make sure you're comfortable calculating unknown angles. The main rules to remember are:
Angles in a right angle add up to 90°
Angles on a straight line add up to 180°
Angles in any triangle add up to 180°
For angles in parallel lines, such as alternate and opposite angles, take a look at the OCR GCSE maths revision notes '7.1.1 Angles in Parallel Lines'

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?