The Unit Circle (DP IB Applications & Interpretation (AI)): Revision Note
Did this video help you?
Defining sin, cos and tan
What is the unit circle?
The unit circle is a circle with radius 1 and centre (0, 0)
Angles are always measured from the positive x-axis and turn:
anticlockwise for positive angles
clockwise for negative angles
How can the unit circle be used to define sin, cos and tan?
Measure the angle
from the positive x-axis
Label the point on the circle
The trigonometric ratios can be found using SOHCAHTOA
is the y-coordinate of the point
is the x-coordinate of the point
is the gradient of the line segment from the centre to the point
You can use these definitions to calculate sin, cos and tan for angles that are:
greater than 90°
negative
measure the angle clockwise
greater than 360°
go round the circle again

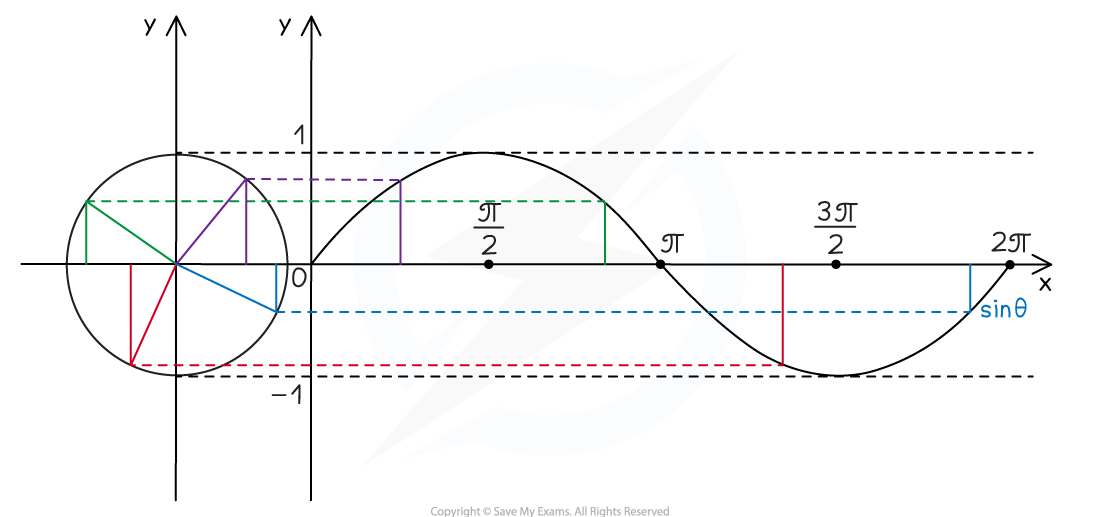
How can I use the unit circle to construct the sine graph?
The y-coordinates of the points on the unit circle give the values of sine
You can plot the y-coordinates against the angles to form the sine graph
You should remember the general shape
The sine graph starts at 0
It then increases and is equal to 1 when the angle is
It then decreases and returns to 0 when the angle is
It continues to decrease and is equal to -1 when the angle is
It then increases and returns to 0 when the angle is
The graph then repeats itself
How can I use the unit circle to construct the cosine graph?
The x-coordinates of the points on the unit circle give the values of cosine
You can plot the x-coordinates against the angles to form the cosine graph
You should remember the general shape
The cosine graph starts at 1
It then decreases and is equal to 0 when the angle is
It continues to decrease and is equal to -1 when the angle is
It then increases and is returns to 0 when the angle is
It continues to increase and returns to 1 when the angle is
The graph then repeats itself

Worked Example
The coordinates of a point on a unit circle, to 3 significant figures, are (0.629, 0.777). The radius from the centre to the point forms the angle θ° between the positive -axis. Find θ° to the nearest degree.
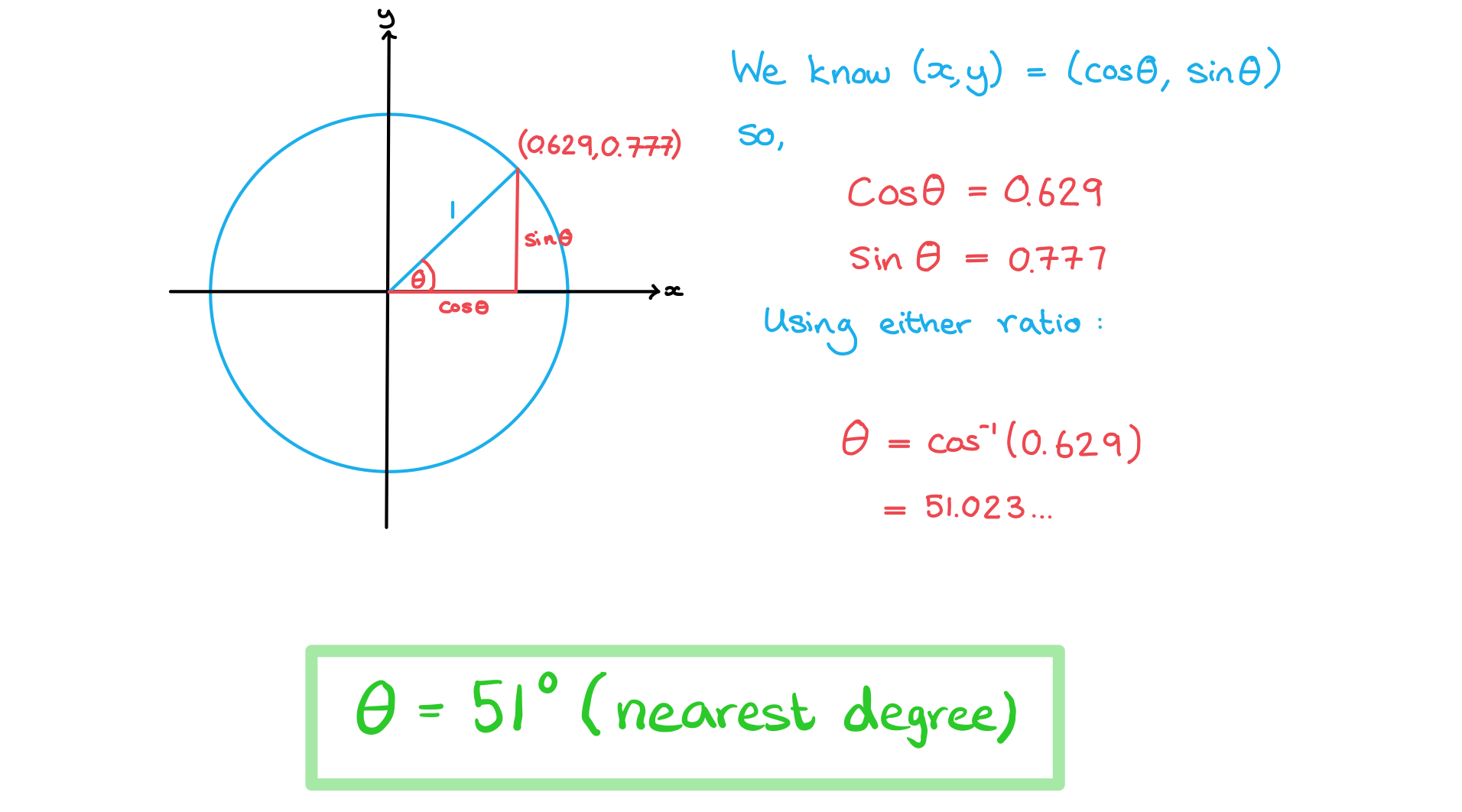
Did this video help you?
Using the unit circle
When are sin, cos and tan positive?
The unit circle can be split into four quadrants at every 90°
The first quadrant is for angles between 0 and 90°
All three of sinθ, cosθ and tanθ are positive in this quadrant
The second quadrant is for angles between 90° and 180°
sinθ is positive in this quadrant
The third quadrant is for angles between 180° and 270°
tanθ is positive in this quadrant
The fourth quadrant is for angles between 270° and 360°
cosθ is positive in this quadrant
Examiner Tips and Tricks
I tell my students to remember the phrase "All students take calculus" to help them remember the order.
What are the symmetries of the unit circle?
You can find symmetries by comparing the points on the unit circles which form the angles
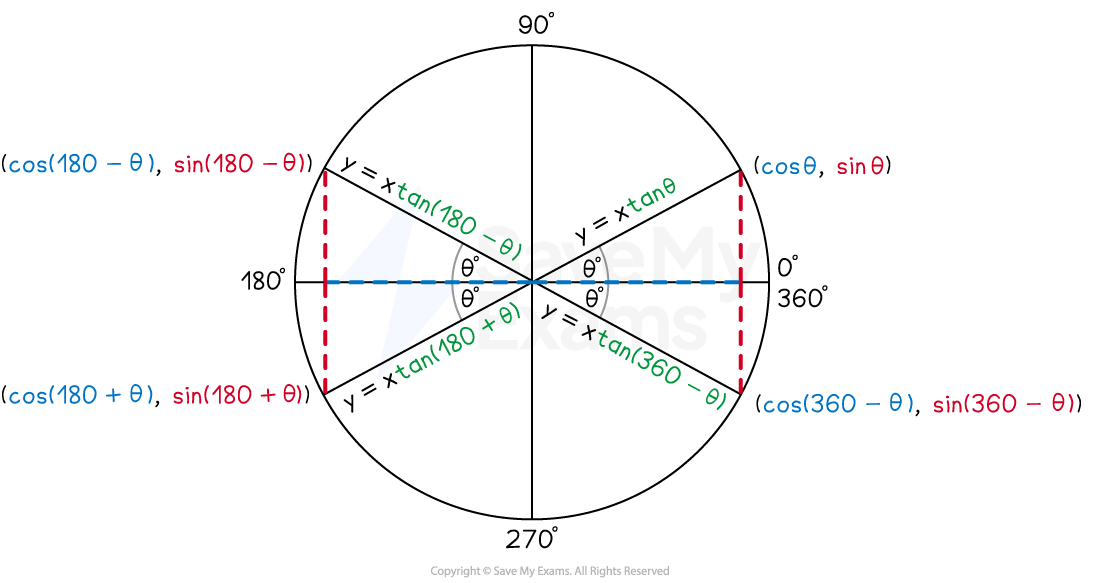
Adding 360° does not change the trig ratio
gives the same ratios as
How can I find the value of sin, cos and tan using the symmetries of the unit circle?
You can write a trig ratio of any angle in terms of a trig ratio of an acute angle
Compare the angle to 180° or 360° and use the symmetries
For example: 210° = 180° + 30°
For example: 315° = 360° - 45°
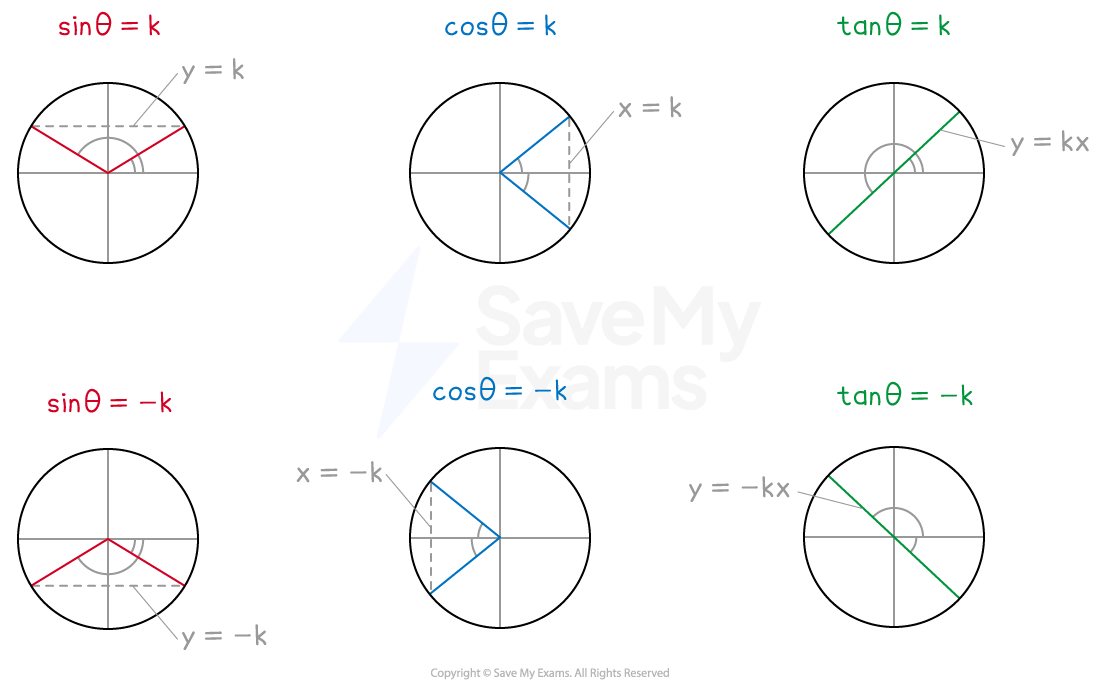
How can I use the unit circle to find solutions to simple trigonometric equations?
STEP 1
Use the inverse trig buttons on your calculator to find the principal valuee.g. to solve
, type in
STEP 2
Label this point on the unit circle and draw the radiusSTEP 3
Find the second point on the unit circle that also leads to a solution and draw the radiusFor the equation
The second point has the same y-coordinate
It is in the quadrant horizontal to the quadrant with the first radius
For the equation
The second point has the same x-coordinate
It is in the quadrant vertical to the quadrant with the first radius
For the equation
The second point has a radius with the same gradient
It is in the quadrant diagonal to the quadrant with the first radius
STEP 4
Find the angle from the positive x-axis to the second radius using symmetriese.g.
and
e.g.
and
e.g.
and
STEP 5
Use the two angles to find all solutions in the given intervalAdd or subtract multiples of 360° from the two angles
Examiner Tips and Tricks
The question could ask you to find the solutions in radians, so make sure you remember the main angles:
180° = π radians
360° = 2π radians
Worked Example
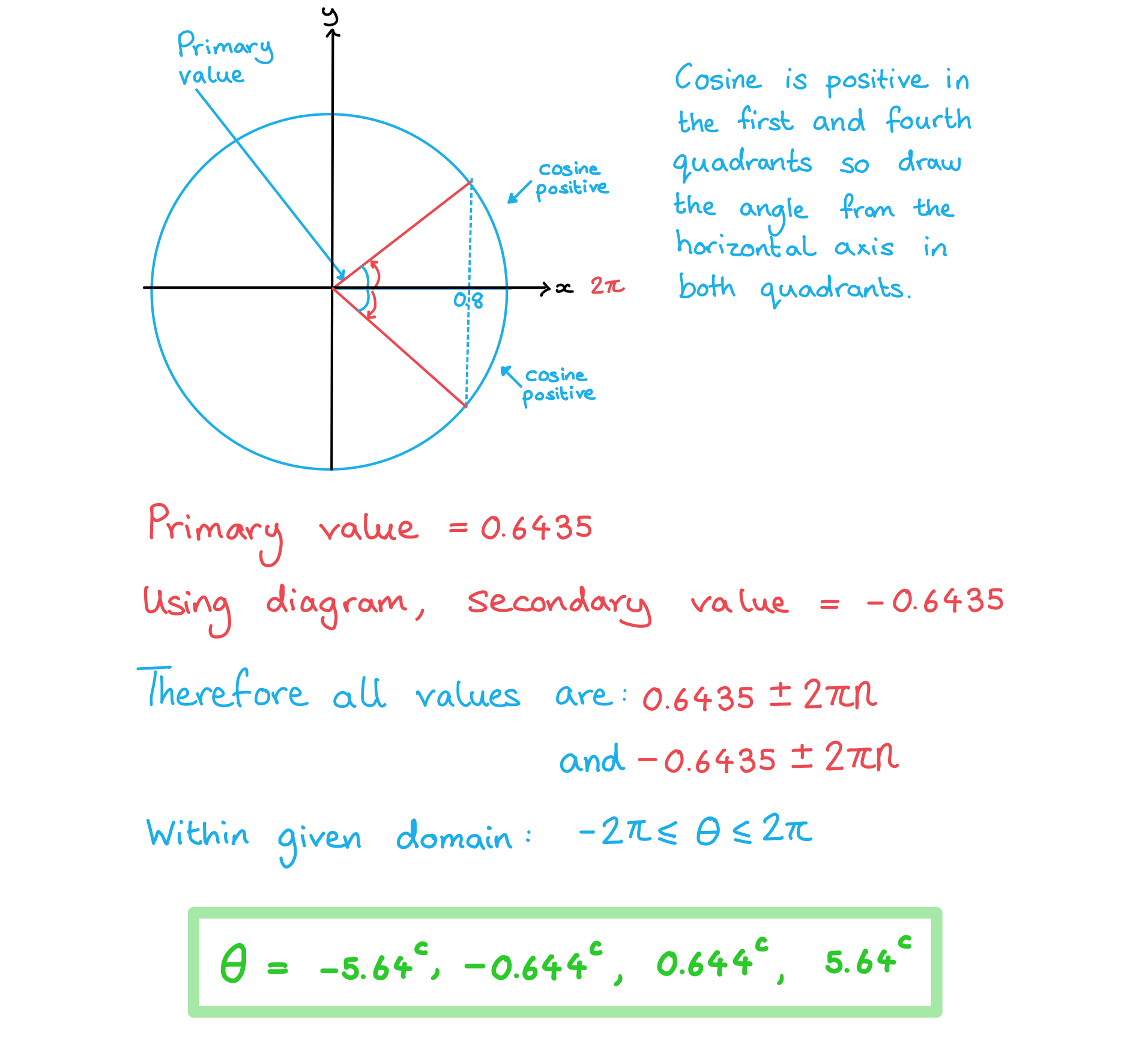
Given that one solution of cosθ = 0.8 is θ = 0.6435 radians correct to 4 decimal places, find all other solutions in the range -2π ≤ θ ≤ 2π. Give your answers correct to 3 significant figures.

Unlock more, it's free!
Did this page help you?