Composite Functions (Cambridge (CIE) O Level Additional Maths): Revision Note

Composite Functions

What is a composite function?

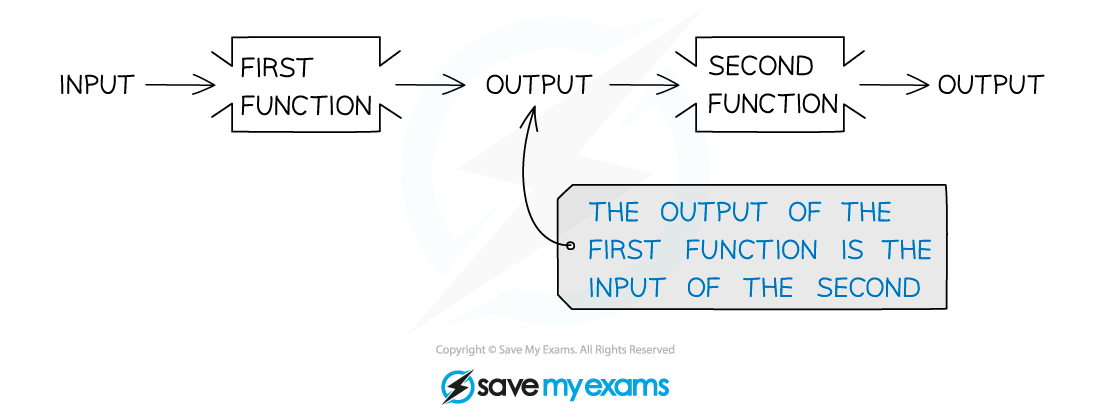
• A composite function is where one function is applied after another function

• The ‘output’ of one function will be the ‘input’ of the next one

• Sometimes called function-of-a-function

• A composite function can be denoted

  • 

  • 

  • All of these mean “ of ”

How do I work with composite functions?

• Recognise the notation

  •  means “f of g of x”

  The order matters

  • First apply  to  to get 

  • Then apply to  to get

  • Always start with the function closest to the variable

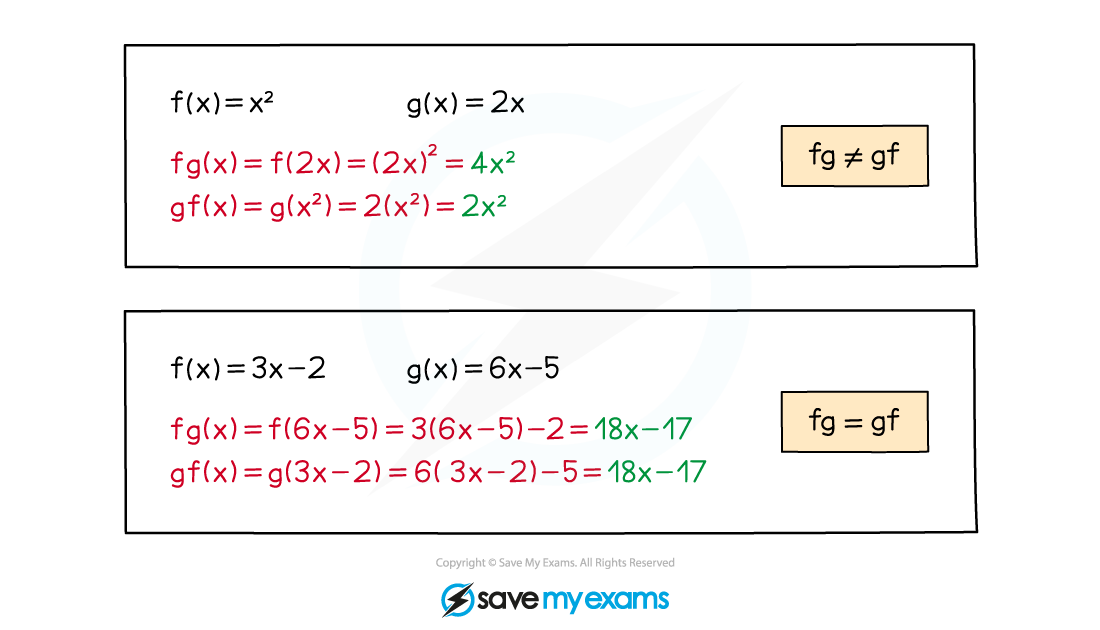
  • is not usually equal to 

Special cases

• and  are generally different but can sometimes be the same

•  is written as

  • Note that trig functions are exceptions to this rule

    • e.g.  means  not 

• For inverse functions, 

Domain & Range of Composite Functions

How do I find the domain and range of composite functions?

• Use logic to determine the domain and range of a composite function

• For  the first function to be applied will be 

  • So, at best, the domain of  will be the same as the domain of 

• However, for this to be the case, the range of  must be contained within the domain of

  • If this is not the case, then restrictions on the domain of  will be required

• Similarly, at best, the range of  will be the same as the range of 

  • But if the domain of  has been affected, the range of  will also be affected