Activity & Half-Life (DP IB Physics: SL): Revision Note
Activity & Half-Life
The activity of a radioactive sample is defined as:
The number of nuclei which decay in a given time
Activity is measured in becquerels (Bq)
One becquerel is equivalent to a nucleus decaying every second
It is impossible to know when a particular unstable nucleus will decay
But the rate at which the activity of a sample decreases can be predicted
This is known as the half-life
Half-life is defined as:
The time taken for half the undecayed nuclei to decay or the activity of a source to decay by half
In other words, the time it takes for the activity of a sample to fall to half its original level
Different isotopes have different half-lives, and these can vary from a fraction of a second to billions of years in length
Using Half-life
Scientists can measure the half-lives of different isotopes accurately:
Uranium-235 has a half-life of 704 million years
This means it would take 704 million years for the activity of a uranium-235 sample to decrease to half its original amount
Carbon-14 has a half-life of 5700 years
So after 5700 years, there would be 50% of the original amount of carbon-14 remaining
After two half-lives, or 11 400 years, there would be just 25% of the carbon-14 remaining
With each half-life, the amount remaining decreases by half
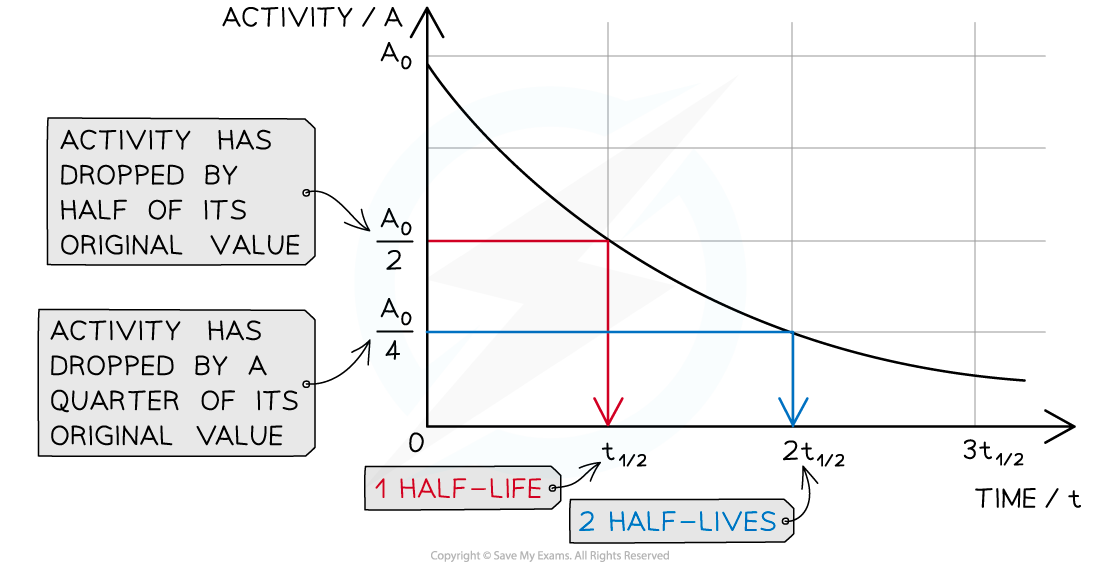
Graph showing how the activity of a radioactive sample changes over time. Each time the original activity halves, another half-life has passed
The time it takes for the activity of the sample to decrease from 100 % to 50 % is the half-life
It is the same length of time as it would take to decrease from 50 % activity to 25 % activity
The half-life is constant for a particular isotope
The proportion of an isotope remaining after half-lives has passed can be calculated using:
proportion of isotope remaining =
The following table shows that as the number of half-lives increases, both the proportion of the isotope remaining and the activity of the sample halves
Number of half-lives | Proportion of isotope remaining | Activity of sample |
|---|---|---|
0 | 1 | |
1 | ||
2 | ||
3 | ||
4 |
Worked Example
A radioactive sample has a half-life of 3 years. What is the ratio of decayed nuclei to original nuclei after 15 years?
Answer:
Step 1: Calculate the number of half-lives
The time period is 15 years
The half-life is 3 years
number of half-lives = = 5
Therefore, 5 half-lives have passed
Step 2: Determine the proportion of nuclei remaining
The proportion of nuclei remaining is:
So 1/32 of the original nuclei are remaining
Step 3: Write the ratio correctly
If 1/32 of the original nuclei are remaining, then 31/32 must have decayed
Therefore, the ratio is 31 decayed : 32 original, or 31:32
Worked Example
A particular radioactive sample contains 2 million un-decayed atoms. After a year, there are only 500 000 atoms left un-decayed.
Determine the half-life of the material.
Answer:
Step 1: Calculate how many times the number of un-decayed atoms has halved
There were 2 000 000 atoms to start with
1 000 000 atoms would remain after 1 half-life
500 000 atoms would remain after 2 half-lives
Therefore, the sample has undergone 2 half-lives
Step 2: Divide the time period by the number of half-lives
The time period is a year
The number of half-lives is 2
So 1 year represents two half-lives, and 6 months represents one half-life
Therefore, the half-life of the sample is 6 months
Decay Curves
To calculate the half-life of a sample, the procedure is:
Measure the initial activity, A0, of the sample
Measure how the activity changes with time
Determine the half-life of this original activity
The time taken for the activity to decrease to half its original value is the half-life
Worked Example
The radioisotope technetium is used extensively in medicine. The graph below shows how the activity of a sample varies with time.
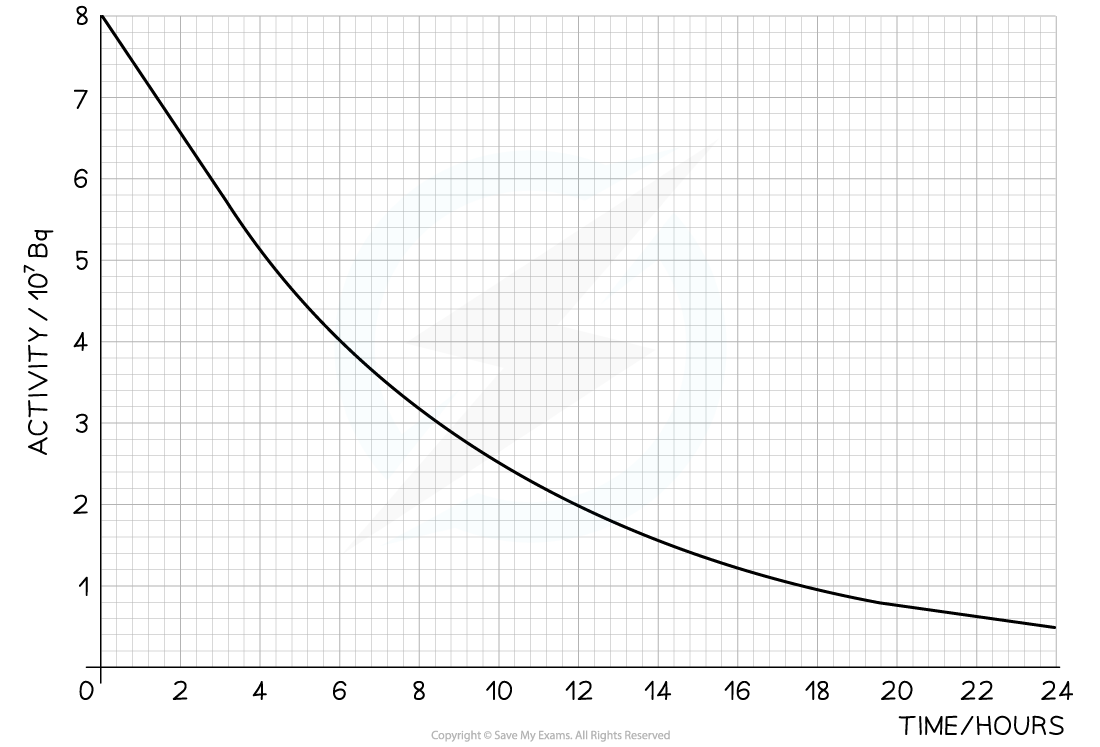
Determine the half-life of this material.
Answer:
Step 1: Draw lines on the graph to determine the time it takes for technetium to drop to half of its original activity
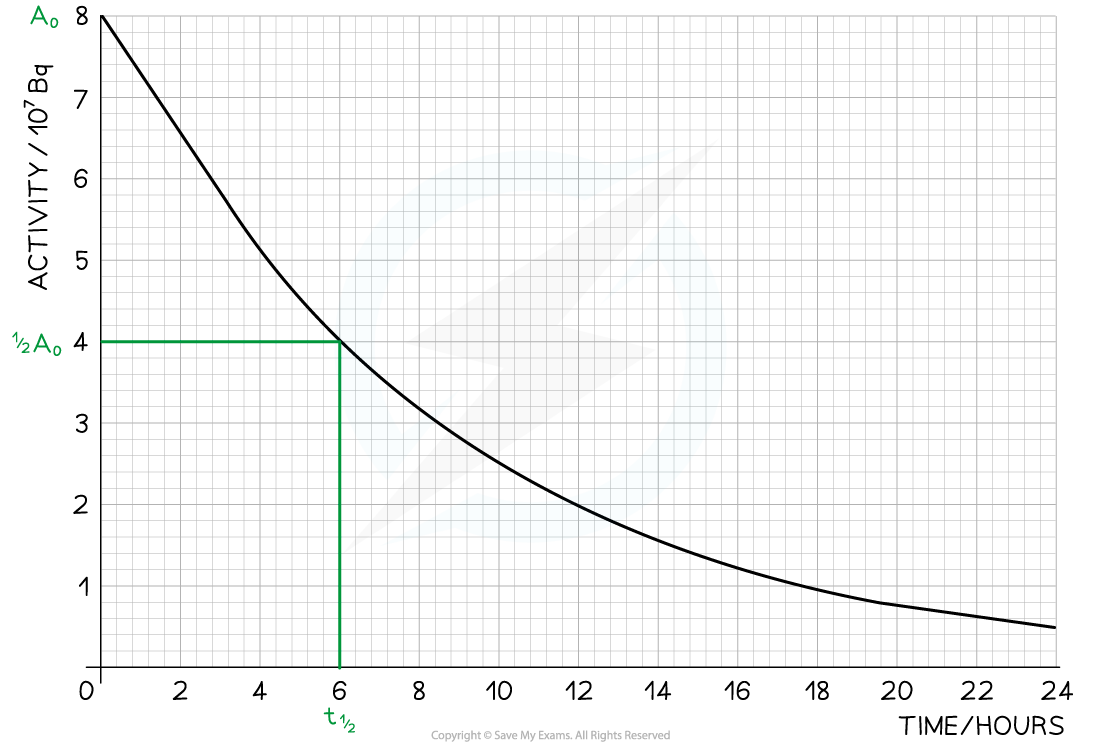
Step 2: Read the half-life from the graph
In the diagram above the initial activity, A0, is 8 × 107 Bq
The time taken to decrease to 4 × 107 Bq, or ½A0, is 6 hours
The time taken to decrease to 2 × 107 Bq is 6 more hours
The time taken to decrease to 1 × 107 Bq is 6 more hours
Therefore, the half-life of this isotope is 6 hours
Unlock more, it's free!
Join the 100,000+ Students that ❤️ Save My Exams
the (exam) results speak for themselves:
Was this revision note helpful?
Build on this topic
