Non-Uniform Circular Motion (DP IB Physics): Revision Note
Non-Uniform Circular Motion
Some bodies are in non-uniform circular motion
This happens when there is a changing resultant force such as in a vertical circle
An example of vertical circular motion is swinging a ball on a string in a vertical circle
The forces acting on the ball are:
the tension in the string
the weight of the ball
As the ball moves around the circle, the direction of the tension will change continuously
The magnitude of the tension will also vary continuously, reaching a maximum value at the bottom and a minimum value at the top
This is because the direction of the weight of the ball never changes, so the resultant force will vary depending on the position of the ball in the circle
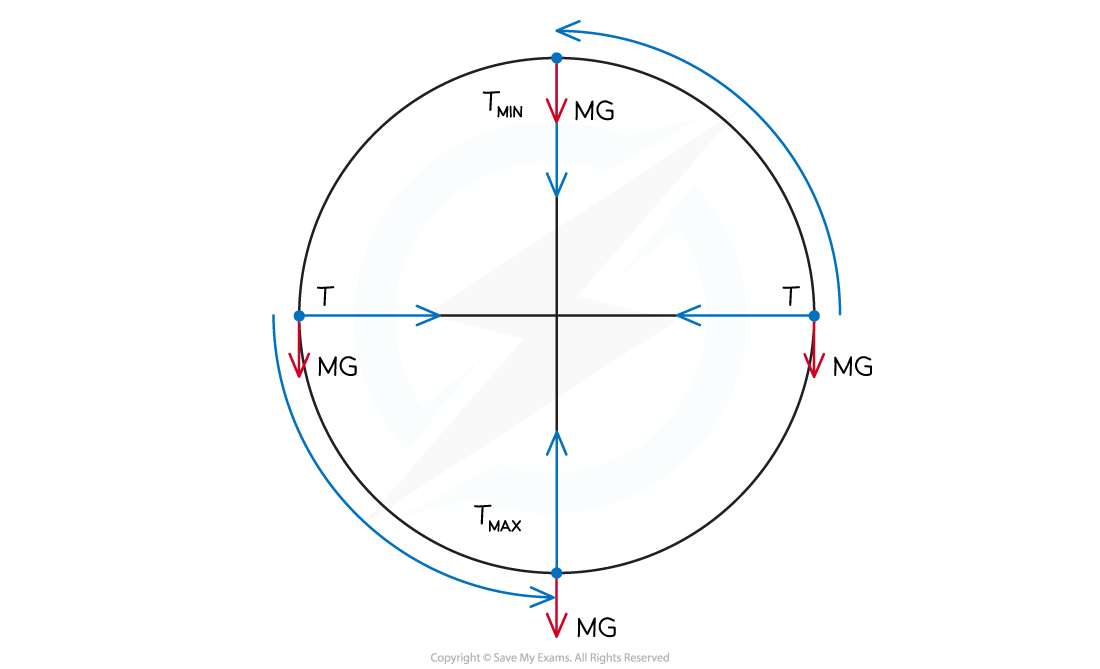
At the bottom of the circle, the tension must overcome the weight; this can be written as:
As a result, the acceleration, and hence, the speed of the ball, will be slower at the top
At the top of the circle, the tension and weight act in the same direction; this can be written as:
As a result, the acceleration, and hence, the speed of the ball, will be faster at the bottom
Worked Example
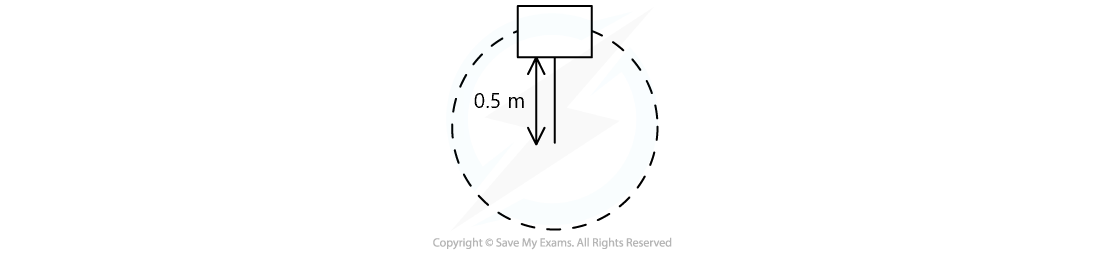
A bucket of mass 8.0 kg is filled with water and is attached to a string of length 0.5 m.
What is the minimum speed the bucket must have at the top of the circle so no water spills out?
Answer:
Step 1: Draw the forces on the bucket at the top

Although tension is in the rope, at the very top, the tension is 0
Step 2: Calculate the centripetal force
The weight of the bucket = mg
This is equal to the centripetal force since it is directed towards the centre of the circle
Step 3: Rearrange for velocity v
m cancels from both sides
Step 4: Substitute in values

Unlock more, it's free!
Did this page help you?