Representing Inequalities as Regions (Cambridge (CIE) O Level Maths): Revision Note
Exam code: 4024
Did this video help you?
Finding regions using inequalities
What are 2D inequalities?
Recall that an inequality in one variable (1D inequality) represents a relationship that is not equal
An inequality of x < 7, represents all values smaller than 7
There are an infinite number of values than can satisfy this inequality
A 2D inequality represents a relationship between two expressions that is not equal
The inequality y > x represents all pairs of numbers x and y where the y value is greater than the x value
There are an infinite number of pairs of values that would satisfy this inequality
These pairs of numbers can be thought of as coordinates
On a graph, all coordinates above the line y = x would satisfy this inequality
If a 2D inequality includes either the symbol ≤ or ≥, then coordinates on the line itself also satisfies the inequality
E.g. y ≤ 2x represents all of the pairs of numbers where the value of y is less than two lots of the value of x
This is the region below the line y = 2x, but also being on the line y = 2x satisfies the inequality
How do we draw inequalities on a graph?
A set of 2D inequalities can be shown graphically using straight lines and shaded regions
To draw the correct lines:
Replace the inequality sign with “=” and draw that line
Use a solid line for ≤ or ≥ (to indicate the line is included)
Use dotted line for < or > (to indicate the line is not included)
To decide which side of the line is the wanted side:
if "y ≤ ..." or "y < ..." then the wanted region is below the line
if "y ≥ ..." or "y > ..." then the wanted region is above the line
If you are unsure
substitute the coordinates from a point on one side of the line into the inequality
determine whether or not the inequality holds true on that side
For vertical lines:
the wanted region for
is to the left of
the wanted region for
is to the right of
To do the shading:
Shade the unwanted sides of each line (unless the question says otherwise)
You are shading away any parts you don't want
This will leave behind a clear region that is the wanted region (rather than trying to look for the wanted region under multiple shades)
Label the wanted region R (unless the question says otherwise)
(Be careful if using graphing software, as some shade the wanted sides)
Worked Example
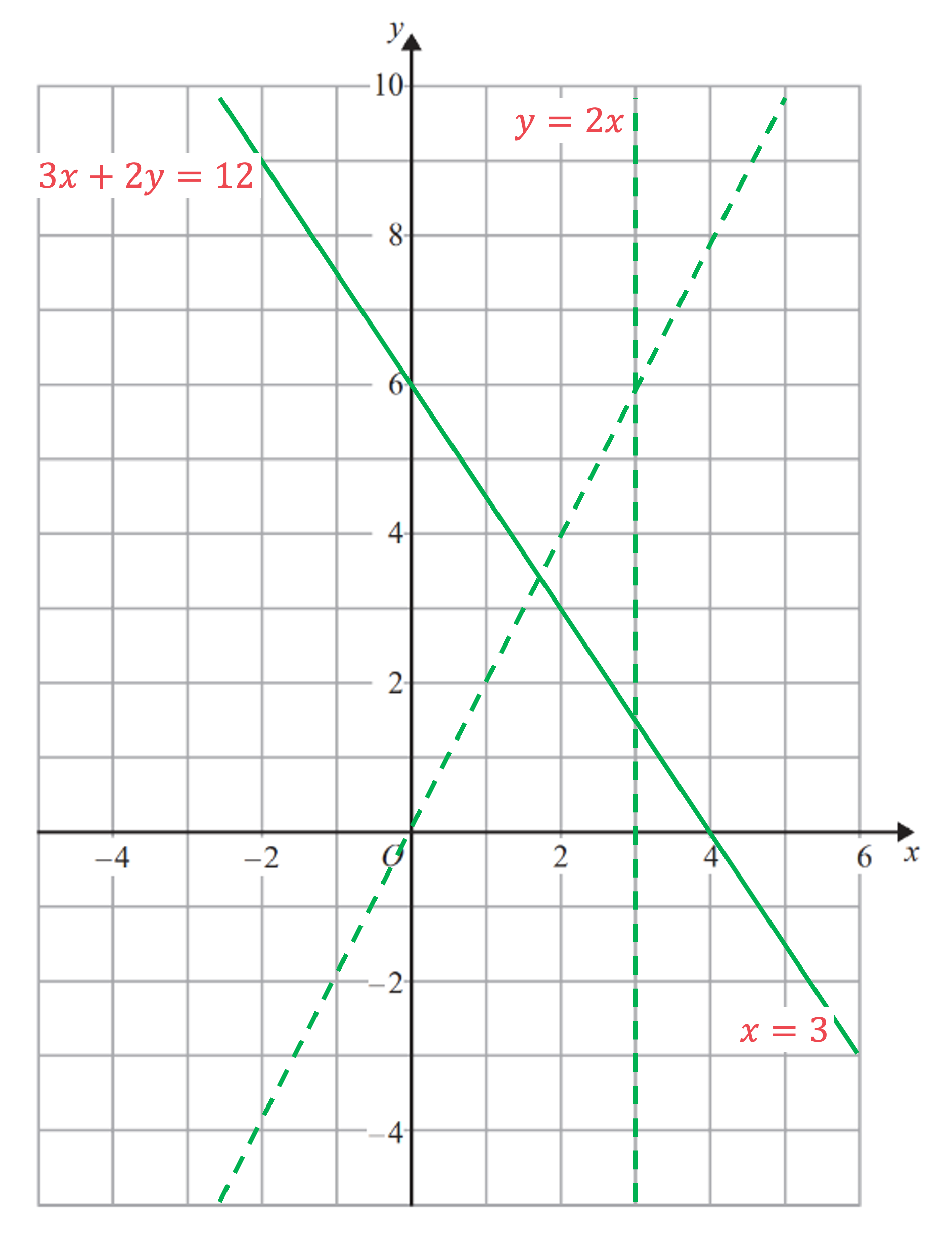
Show, graphically, the region that is satisfied by all three inequalities below:
Label this region R.
First draw the three straight lines: ,
and
Use your knowledge of Straight Line Graphs,
You may wish to rearrange to the form
first
The line is a solid line because of the "≥"
The lines and
are dotted lines because of the "<"
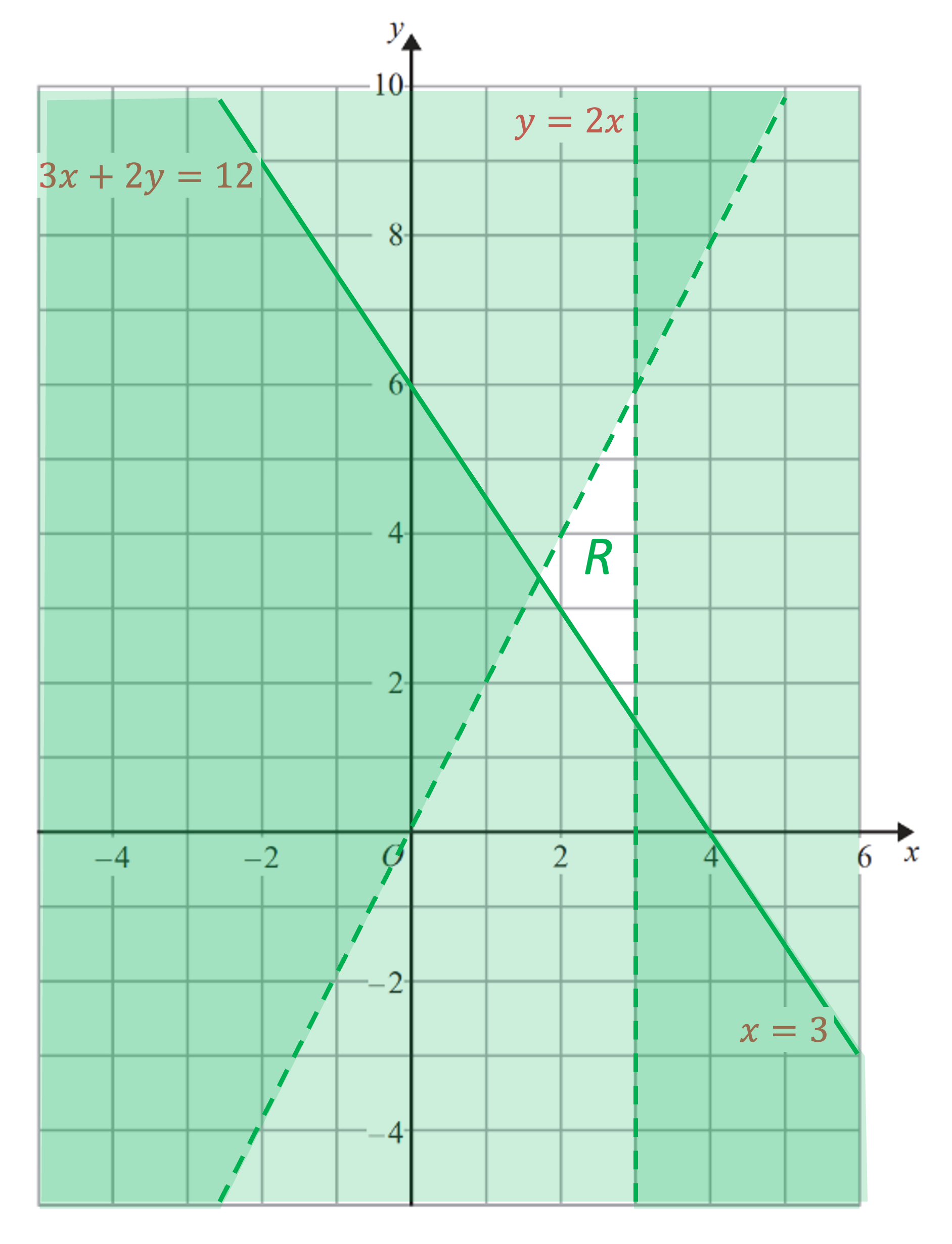
Now we need to shade the unwanted regions
For (or
), the unwanted region is below the line
We can check this with the point (0, 0)
is false therefore (0, 0) does lie in the unwanted region for
For , the unwanted region is above the line
Check with another point, for example (1, 0)
is true, so (1, 0) lies in the wanted (i.e. unshaded) region for
For , shade the unwanted region to the right of
If unsure, check with a point
Finally, don't forget to label the region R

Unlock more, it's free!
Did this page help you?