Solving Equations from Graphs (Cambridge (CIE) IGCSE Maths): Revision Note
Exam code: 0580 & 0980
Solving Equations Using Graphs
How do I find the coordinates of points of intersection?
Plot two graphs on the same set of axes
The points of intersection are where the two lines meet
For example, plot y = x2 + 3x + 1 and y = 2x + 1 on the same axes
They meet twice, as shown
The coordinates of intersection are (-1, -1) and (0, 1)

How do I solve simultaneous equations graphically?
The x and y solutions to simultaneous equations are the x and y coordinates of the point of intersection
For example, to solve 2x - y = 3 and 3x + y = 7 simultaneously
Rearrange them into the form y = mx + c
y = 2x - 3 and y = -3x + 7
Use a table of values to plot each line
Find the point of intersection, (2, 1)
The solutions are therefore x = 2 and y = 1

How do I use graphs to solve equations?
This is easiest explained through an example
You can use the graph of
to solve the following equations
The solutions are the two x-intercepts
This is where the curve cuts the x-axis (also called roots)
The solutions are the two x-coordinates where the curve intersects the horizontal line
the solutions are the two x-coordinates where the curve intersects the straight line
The straight line must be plotted on the same axes first
To solve a different equation like
add / subtract terms to both sides to get "graph = ..."
For example, subtract 5 from both sides
This can now be done as above
Examiner Tips and Tricks
When solving equations in x, only give x-coordinates as final answers
Include the y-coordinates if solving simultaneous equations
Worked Example
Use the graph of shown to estimate the solutions of each equation given below.
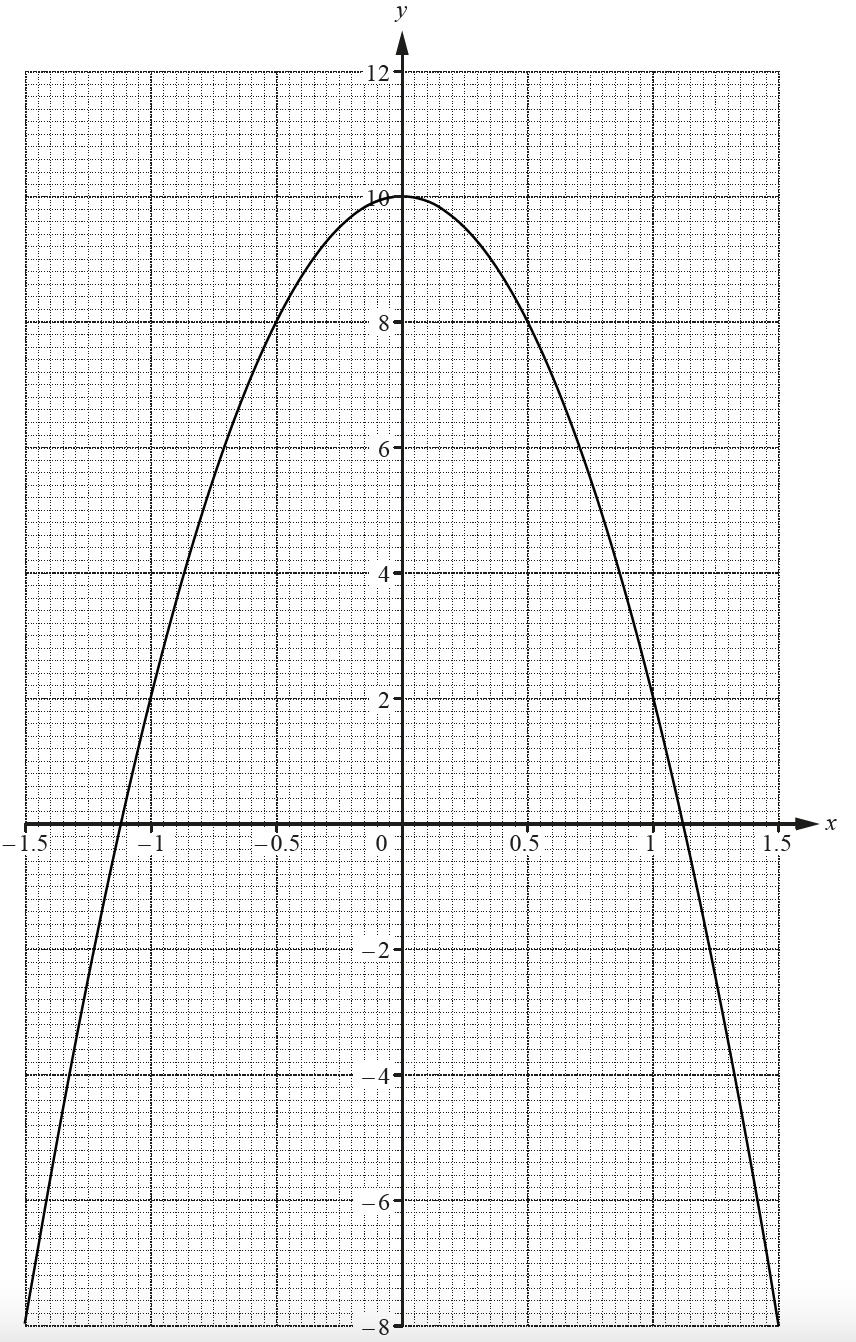
(a)
This equals zero, so the x-intercepts are the solutions
Read off the values where the curve cuts the x-axis
Use a suitable level of accuracy (no more than 2 decimal places from the scale of this graph)
-1.12 and 1.12
These are the two solutions to the equation
x = -1.12 and x = 1.12
A range of solutions are accepted, such as "between 1.1 and 1.2"
Solutions must be ± of each other (due to the symmetry of quadratics)
(b)
This equals 8, so draw the horizontal line y = 8
Find the x-coordinates where this cuts the graph
-0.5 and 0.5
These are the two solutions to the original equation
x = -0.5 and x = 0.5
The solutions here are exact

Unlock more, it's free!
Did this page help you?