AP®MathsCollege BoardCalculus ABStudy GuidesUnit 8: Applications of IntegrationAreasArea Between Two Curves

Area Between Two Curves (College Board AP® Calculus AB) : Study Guide

Jamie Wood

Written by: Jamie Wood

Reviewed by: Dan Finlay

Updated on

College Board Calculus ABCollege Board Calculus BC

Area between two curves in terms of x

How do I find the area between two curves?

  • Consider the diagram below where

    • The area between two curves, y equals f open parentheses x close parentheses and y equals g open parentheses x close parentheses is being found

      • bounded by the lines x equals a and x equals b

    • This region is labeled R

Graph showing two curves, y = f(x) and y = g(x), with a shaded region R between x = a and x = b. Integral expression for the area R is displayed.

  • The region R is the difference between the two areas found by:

    • Integrating y equals f open parentheses x close parentheses between x equals a and x equals b

    • Integrating y equals g open parentheses x close parentheses between x equals a and x equals b

  • As f open parentheses x close parentheses greater or equal than g open parentheses x close parentheses for all of this interval, this area can be calculated as

    • integral subscript a superscript b f open parentheses x close parentheses space italic d x space minus space integral subscript a superscript b g open parentheses x close parentheses space italic d x

  • This is equivalent to

    • integral subscript a superscript b open parentheses f open parentheses x close parentheses minus g open parentheses x close parentheses close parentheses space italic d x

  • It is essential to have the function which is "above" the other be first inside the integral

    • If the curves overlap and form multiple regions, see the method outlined in the 'Multiple Areas' study guide

  • Also note that if the graph of y equals f open parentheses x close parentheses is above the graph of y equals g open parentheses x close parentheses on open square brackets a comma space b close square brackets

    • then f open parentheses x close parentheses minus g open parentheses x close parentheses greater or equal than 0 everywhere on that interval

    • This means that you don't need to worry about negative integrals when integrating integral subscript a superscript b open parentheses f open parentheses x close parentheses minus g open parentheses x close parentheses close parentheses space italic d x

      • The integral will give the correct area value whether the area is above or below the x-axis

What if I am not told the limits?

  • If you are not told the limits, it is likely you are finding the area enclosed by two curves, f open parentheses x close parentheses and g open parentheses x close parentheses, which intersect each other

  • Find the bold italic x-values of the points of intersection of the two curves by solving f open parentheses x close parentheses equals g open parentheses x close parentheses

    • These will be the limits for the integral

Worked Example

Find the area of the region enclosed by the two curves with equations y equals 2 x squared minus 4 x plus 2 and y equals negative 2 x squared plus 8 x minus 6.

The curves are shown on the graph below.

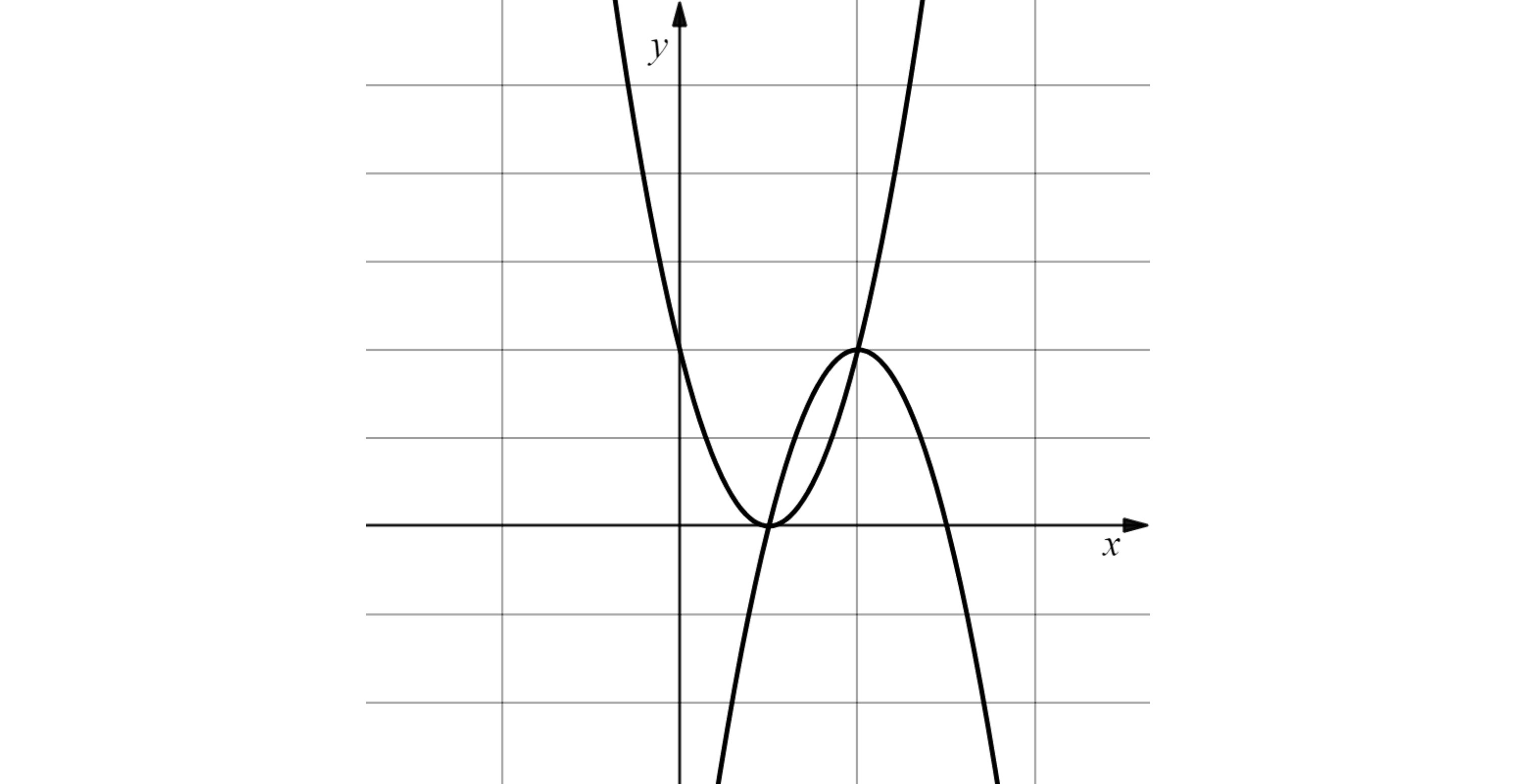
Two intersecting quadratic curves, one a negative quadratic, the other a positive quadratic. They intersect in two places.

Answer:

Find the points where the two curves intersect, by setting their equations equal to one another and solving

table row cell 2 x squared minus 4 x plus 2 end cell equals cell negative 2 x squared plus 8 x minus 6 end cell row cell 4 x squared minus 12 x plus 8 end cell equals 0 row cell x squared minus 3 x plus 2 end cell equals 0 row cell open parentheses x minus 2 close parentheses open parentheses x minus 1 close parentheses end cell equals 0 end table

x equals 2 or x equals 1

Between the intersections, the n-shaped graph (the negative quadratic) is above the u-shaped graph (the positive quadratic) so the area integral will be of the following form

integral subscript 1 superscript 2 open parentheses open parentheses negative 2 x squared plus 8 x minus 6 close parentheses minus open parentheses 2 x squared minus 4 x plus 2 close parentheses close parentheses space italic d x

Simplify and then find the value of the definite integral

table row cell integral subscript 1 superscript 2 minus 4 x squared plus 12 x minus 8 space italic d x end cell equals cell open square brackets negative 4 over 3 x cubed plus 6 x squared minus 8 x close square brackets subscript 1 superscript 2 end cell row blank equals cell open parentheses negative 4 over 3 open parentheses 2 close parentheses cubed plus 6 open parentheses 2 close parentheses squared minus 8 open parentheses 2 close parentheses close parentheses minus open parentheses negative 4 over 3 open parentheses 1 close parentheses cubed plus 6 open parentheses 1 close parentheses squared minus 8 open parentheses 1 close parentheses close parentheses end cell row blank equals cell open parentheses negative 8 over 3 close parentheses minus open parentheses negative 10 over 3 close parentheses end cell row blank equals cell 2 over 3 end cell end table

2 over 3 units squared

Area between two curves in terms of y

How do I find the area between two curves when the functions are in terms of y?

  • The same concepts apply as when the functions are in terms of x

    • but the process is followed relative to the y-axis instead of the x-axis

  • Consider the diagram below where

    • The area between two curves, x equals f open parentheses y close parentheses and x equals g open parentheses y close parentheses is being found

      • bounded by the lines y equals a and y equals b

    • This region is labeled R

Graph showing a shaded region R between curves x=f(y) and x=g(y) from y=a to y=b, with an integral formula for R: ∫[a to b] (f(y) - g(y)) dy.

  • The region R is the difference between the two areas found by:

    • Integrating x equals f open parentheses y close parentheses between y equals a and y equals b

    • Integrating x equals g open parentheses y close parentheses between y equals a and y equals b

  • As f open parentheses y close parentheses greater or equal than g open parentheses y close parentheses for all of this interval, this area can be calculated as

    • integral subscript a superscript b f open parentheses y close parentheses space italic d y space minus space integral subscript a superscript b g open parentheses y close parentheses space italic d y

    • I.e. g open parentheses y close parentheses is closer to the y-axis than f open parentheses y close parentheses is on this interval

  • This is equivalent to

    • integral subscript a superscript b open parentheses f open parentheses y close parentheses minus g open parentheses y close parentheses close parentheses space italic d y

  • It is essential to have the function which is further away from the y-axis, be first inside the integral

    • If the curves overlap and form multiple regions, see the method outlined in the 'Multiple Areas' study guide

  • In these scenarios we are integrating an equation for x in terms of y

    • If you are given an equation for y in terms of x

      • you need to rearrange the equation for xin terms of y

What if I am not told the limits?

  • If you are not told the limits, it is likely you are finding the area enclosed by the two curves, f open parentheses y close parentheses and g open parentheses y close parentheses, which intersect each other

  • Find the y-values of the points of intersection of the two curves by solving f open parentheses y close parentheses equals g open parentheses y close parentheses

    • These will be the limits for the integral

Worked Example

The graph below shows two curves with the following equations

y equals 1 over 8 e to the power of x and y equals 2 e to the power of negative x end exponent

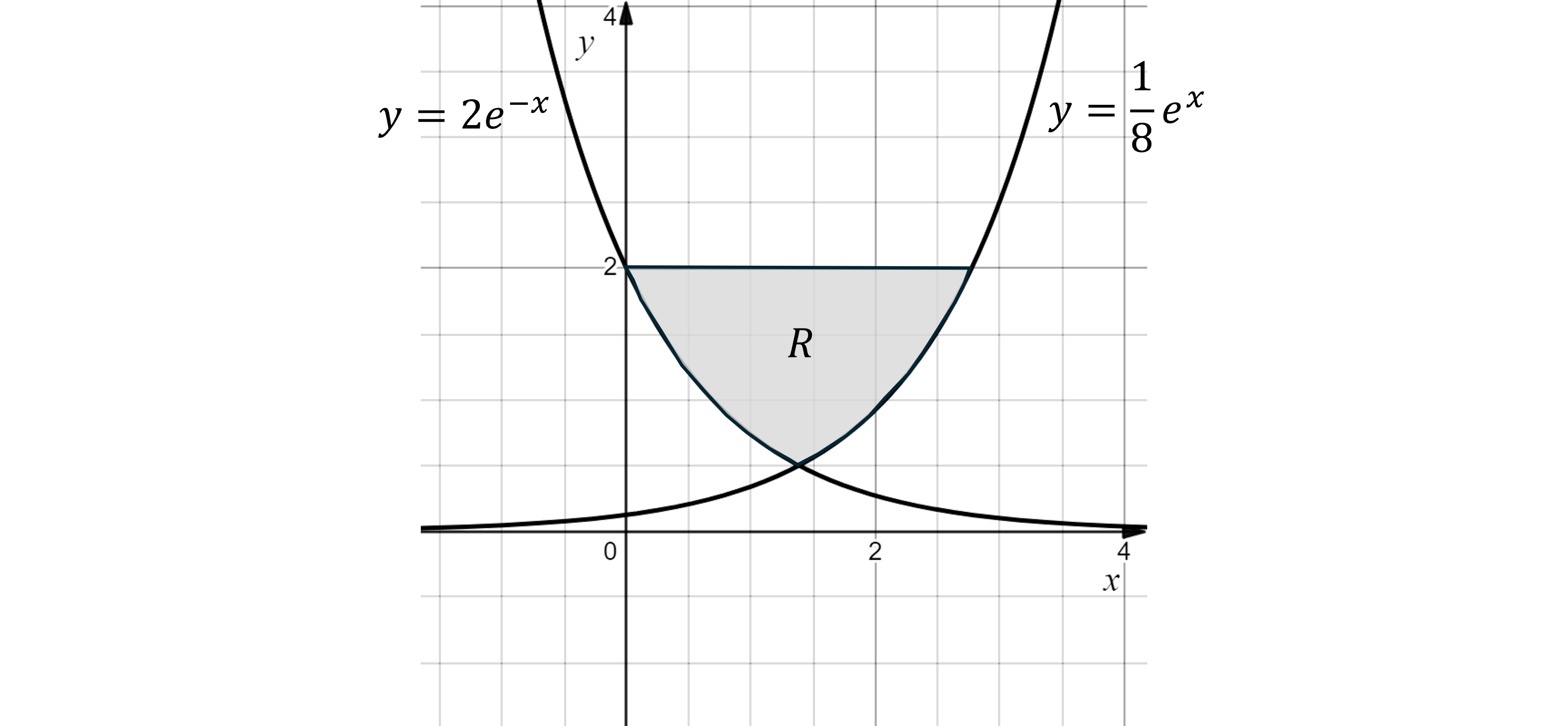
Graph of the functions y = 2e^(-x) and y = (1/8)e^(x) showing the area R bounded by the curves between x = 0 and x = 2, labeled and shaded in gray.

The region R is bounded by the line y equals 2 and the two curves. Find the area of region R.

Answer:

Note that this area could be found by using areas between the curves and the x-axis, and subtracting from the rectangular area underneath the line forming the upper boundary of region R

I.e.

table row cell Area space of space region space R end cell equals cell open parentheses 2 cross times ln 16 close parentheses minus integral subscript 0 superscript ln 4 end superscript 2 e to the power of negative x end exponent space d x space minus integral subscript ln 4 end subscript superscript ln 16 end superscript 1 over 8 e to the power of x space d x end cell row blank equals cell 2 ln 16 minus 3 end cell row blank equals cell 2.54517744... end cell end table

But here we'll work it out using areas between the curves and the y-axis, and integrating in terms of y

Start by working out the integral limits

The upper limit is y equals 2 and the lower limit will be the y value of the point of intersection of the two curves

As we are working in terms of y, rewrite each equation as x in terms of y

For y equals 1 over 8 e to the power of x

table row y equals cell 1 over 8 e to the power of x end cell row cell 8 y end cell equals cell e to the power of x end cell row cell ln open parentheses 8 y close parentheses end cell equals x end table

For y equals 2 e to the power of negative x end exponent

table row y equals cell 2 e to the power of negative x end exponent end cell row cell y over 2 end cell equals cell e to the power of negative x end exponent end cell row cell y over 2 end cell equals cell 1 over e to the power of x end cell row cell 2 over y end cell equals cell e to the power of x end cell row cell ln open parentheses 2 over y close parentheses end cell equals x end table

Find the y-value of the point of intersection by setting these equations equal to each other; this will be the lower limit for the integral

You could also use your calculator to find this

table row cell ln open parentheses 8 y close parentheses end cell equals cell ln open parentheses 2 over y close parentheses end cell row cell 8 y end cell equals cell 2 over y end cell row cell 8 y squared end cell equals 2 row cell y squared end cell equals cell 1 fourth end cell row y equals cell plus-or-minus 1 half end cell end table

y must be positive as neither graph has any negative y values

y equals 1 half

Use an integral of the form integral subscript a superscript b open parentheses f open parentheses y close parentheses minus g open parentheses y close parentheses close parentheses space italic d y

The curve with equation y equals 1 over 8 e to the power of x (or ln open parentheses 8 y close parentheses equals x) is furthest away from the y-axis, so will come first in the integral

integral subscript 1 half end subscript superscript 2 space open parentheses ln open parentheses 8 y close parentheses close parentheses minus open parentheses ln open parentheses 2 over y close parentheses close parentheses space italic d y

You could use your calculator at this point to evaluate the integral, or you can simplify first using laws of logarithms

table row cell ln open parentheses 8 y close parentheses minus ln open parentheses 2 over y close parentheses end cell equals cell ln open parentheses fraction numerator 8 y over denominator open parentheses 2 over y close parentheses end fraction close parentheses end cell row blank equals cell ln open parentheses 4 y squared close parentheses end cell row blank equals cell ln open parentheses 2 y close parentheses squared end cell row blank equals cell 2 ln open parentheses 2 y close parentheses end cell end table

Use your calculator to evaluate the integral

integral subscript 1 half end subscript superscript 2 space 2 ln open parentheses 2 y close parentheses space italic d y equals 2.54517744...

Round to 3 decimal places

2.545 units squared

You've read 1 of your 5 free study guides this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

  • I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

    Beth
    IGCSE Student

  • This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

    Fathima
    A Level Student
Test yourself

Did this page help you?

Previous:Area Between a Curve & y-AxisNext:Multiple Areas
Author
Reviewer
Jamie Wood

Author: Jamie Wood

Expertise: Maths Content Creator

Jamie graduated in 2014 from the University of Bristol with a degree in Electronic and Communications Engineering. He has worked as a teacher for 8 years, in secondary schools and in further education; teaching GCSE and A Level. He is passionate about helping students fulfil their potential through easy-to-use resources and high-quality questions and solutions.

Dan Finlay

Reviewer: Dan Finlay

Expertise: Maths Lead

Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.

Download notes on Area Between Two Curves