Gravitational Potential Energy (OCR A Level Physics)

• The gravitational potential V_g at a point is given by:

• Recall that potential V is defined as the energy required to bring unit mass m from infinity to a defined point in the field
  • Recall that the gravitational field is usually caused by 'big mass', M

• Therefore, the potential energy E  is given by:

E = mV_g

• Substituting the equation for gravitational potential V_g gives the equation for the gravitational potential energy E between two masses M and m:

• Where: 
  • G = Universal Gravitational Constant (N m² kg^–2)
  • M = mass causing the field (kg)
  • m = mass moving within the field of M (kg)
  • r = distance between the centre of m and M (m)

Calculating Changes in Gravitational Potential Energy

• When a mass is moved against the force of gravity, work is required
  • This is because gravity is attractive, therefore, energy is needed to work against this attractive force

• The work done (or energy transferred) ∆W to move a mass m between two different points in a gravitational potential ∆V is given by:

∆W = m ∆V

• Where:
  • ∆W = work done or energy transferred (J)
  • m = mass (kg)
  • ∆V = change in gravitational potential (J kg^-1)

• This work done, or energy transferred, is the change in gravitational potential energy (G.P.E) of the mass
  • When ∆V = 0, then the the change in G.P.E = 0

• The change in G.P.E, or work done, for an object of mass m at a distance r₁ from the centre of a larger mass M, to a distance of r₂ further away can be written as:

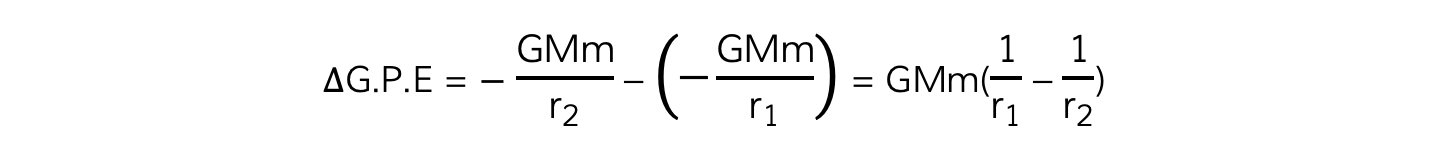
• Where:
  • M = mass that is producing the gravitational field (e.g., a planet) (kg)
  • m = mass that is moving in the gravitational field (e.g., a satellite) (kg)
  • r₁ = initial distance of m from the centre of M (m)
  • r₂ = final distance of m from the centre of M (m)

• Work is done when an object in a planet's gravitational field moves against the gravitational field lines, i.e., away from the planet
  • This is, again, because gravity is attractive
  • Therefore, energy is required to move against gravitational field lines

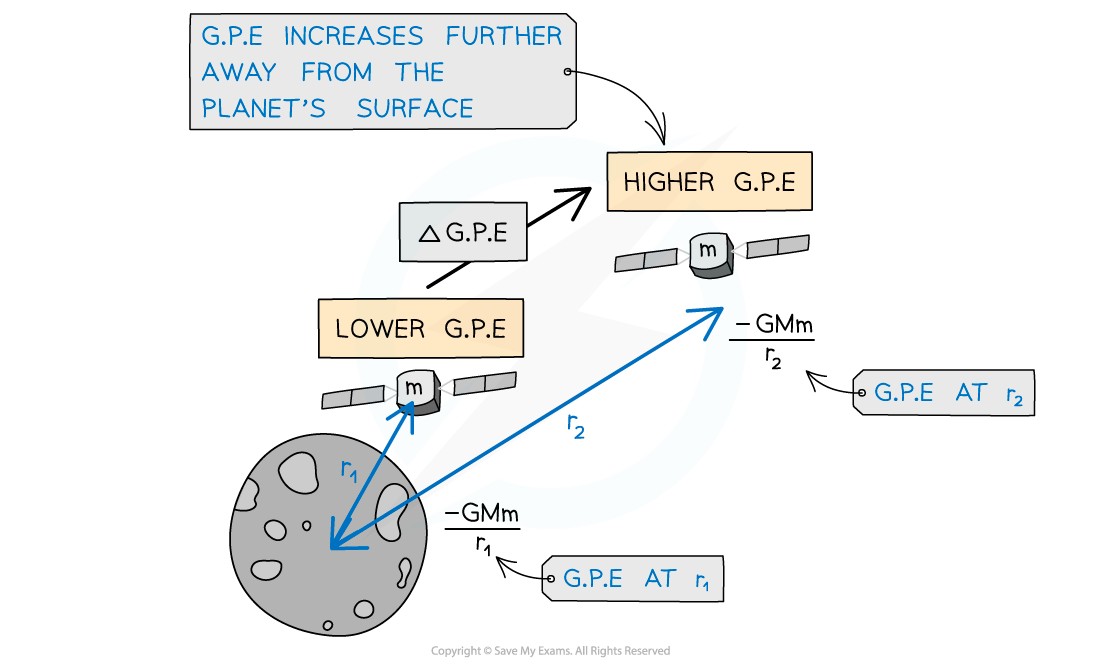
Gravitational potential energy increases as a satellite leaves the surface of the Moon

Step 1:            Write down the work done (or change in G.P.E) equation

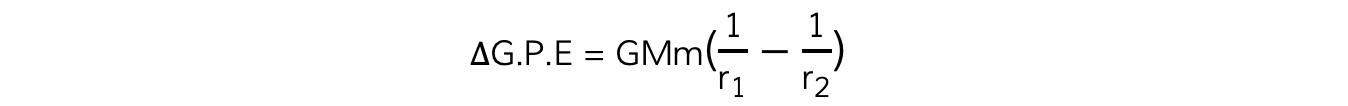
Step 2:            Determine values for r₁ and r₂

r₁ is the radius of Mars = 3400 km = 3400 × 10³ m

r₂ is the radius + altitude = 3400 + 700 = 4100 km = 4100 × 10³ m

Step 3:            Substitute in values

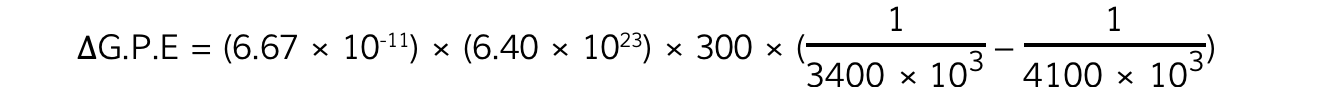
ΔG.P.E =  643.076 × 10⁶  = 640 MJ (2 s.f.)