First Principles Differentiation (DP IB Analysis & Approaches (AA)) : Revision Note

First Principles Differentiation

What is differentiation from first principles?

•  Differentiation from first principles uses the definition of the derivative of a function f(x)

• The definition is

•  means the 'limit as h tends to zero'

• When,  which is undefined

  • Instead we consider what happens as h gets closer and closer to zero

• Differentiation from first principles means using that definition to show what the derivative of a function is

• The first principles definition (formula) is in the formula booklet

How do I differentiate from first principles?

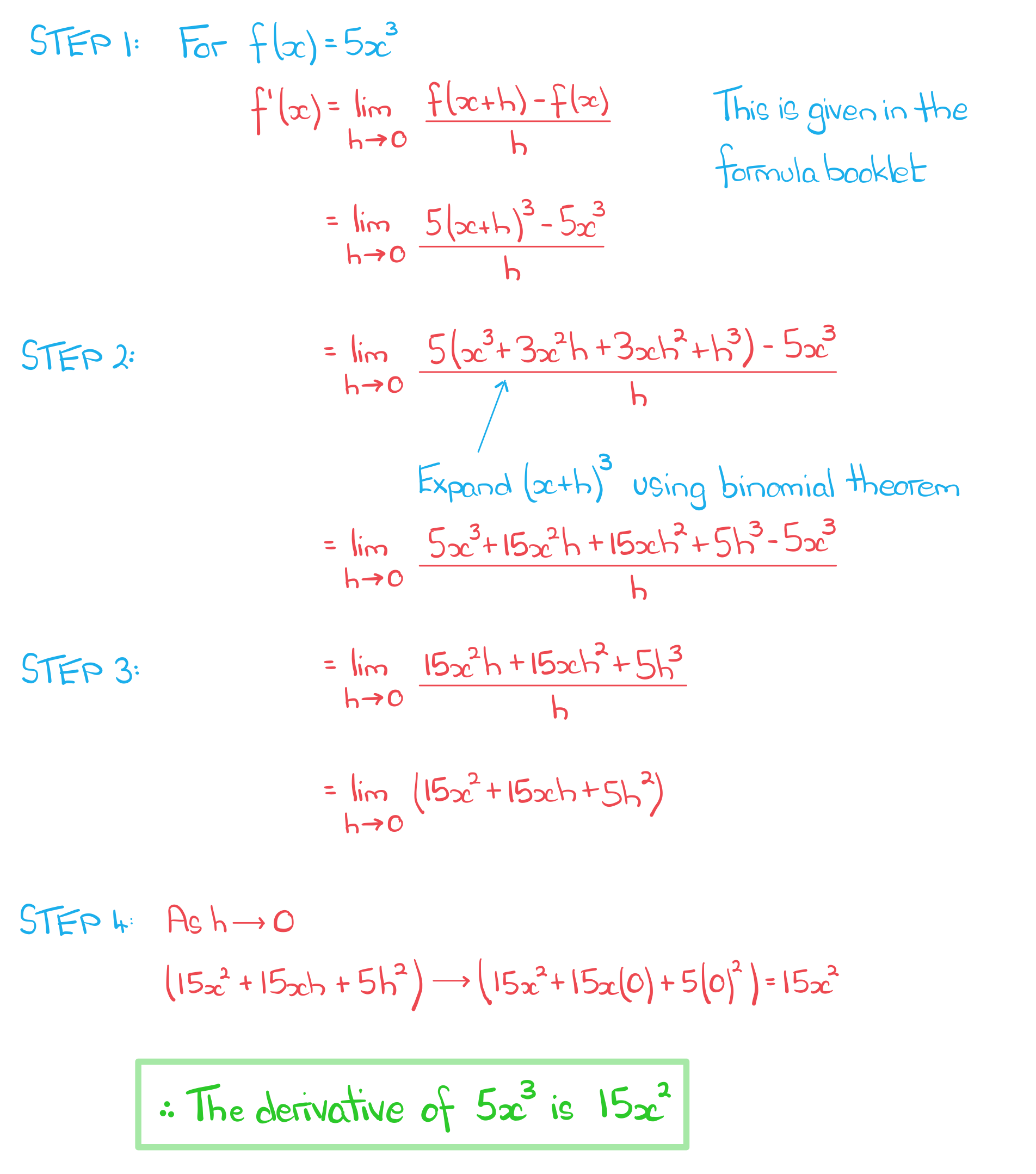
STEP 1: Identify the function f(x) and substitute this into the first principles formula   

   e.g.  Show, from first principles, that the derivative of 3x² is 6x   

    so 

STEP 2: Expand f(x+h) in the numerator   

   

STEP 3: Simplify the numerator, factorise and cancel h with the denominator   

STEP 4: Evaluate the remaining expression as h tends to zero   

   The derivative of  is