Intersecting Chord Theorem (Edexcel IGCSE Maths A): Revision Note
Exam code: 4MA1
Did this video help you?
Intersecting chord theorem
What is the intersecting chord theorem?
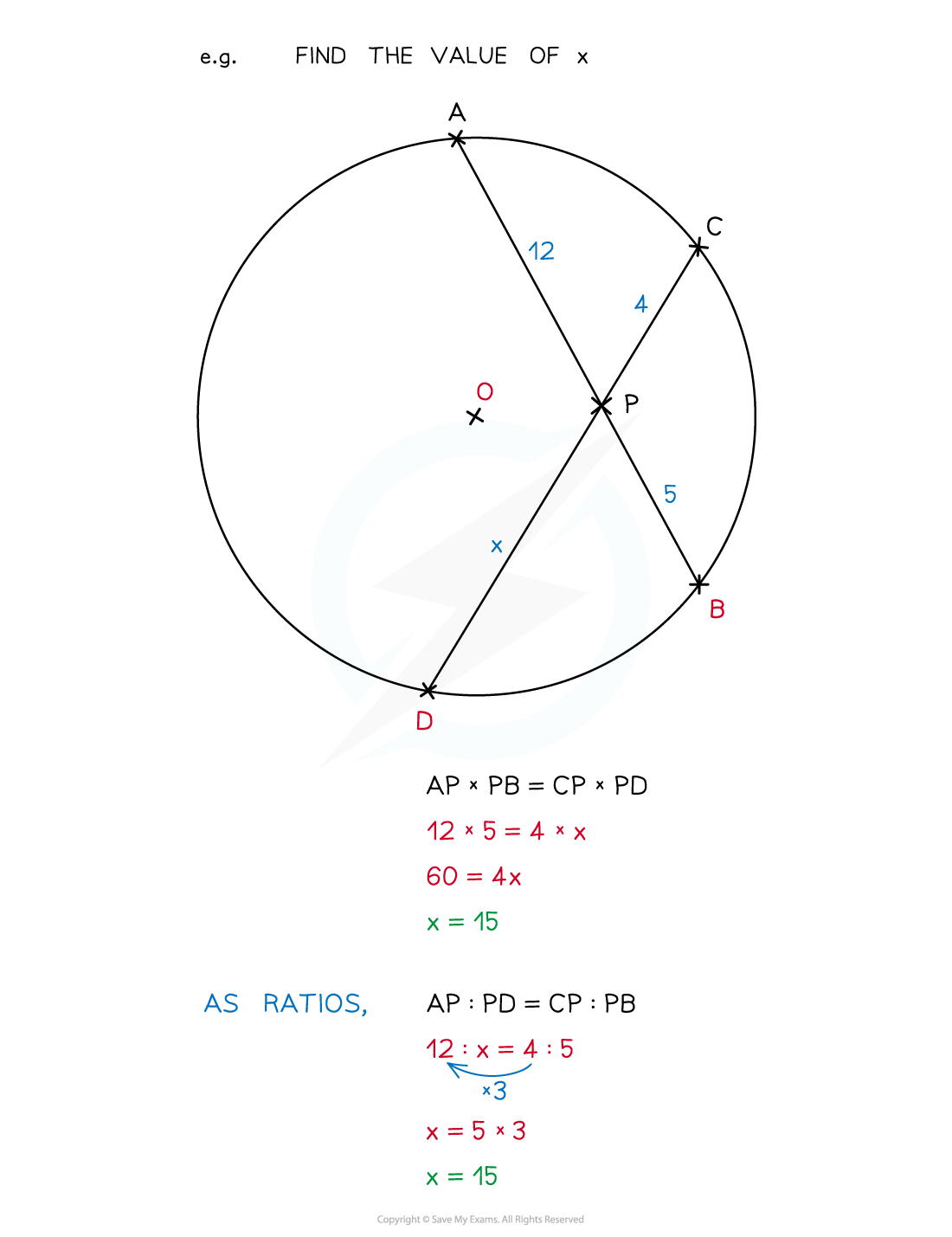
For two chords, AB and CD that meet at point P
AP : PD ≡ CP : PB
Ratio of longer lengths (of chords) ≡ Ratio of shorter lengths (of chords)
This can also be written as AP × PB = CP × PD
You do not need to know the proof of this theorem
This theorem is closely related to similar shapes

How do I use the intersecting chord theorem to solve problems?
If two chords intersect, you can find a missing length using the intersecting chord theorem
You can usually choose to solve the problem either using multiplication (AP × PB = CP × PD) or using ratio (AP : PD ≡ CP : PB)
Carefully keep track of which distance is associated with each part of each chord
What if the lengths are algebraic?
If any of the lengths are algebraic expressions, use the fact that AP × PB = CP × PD to form an equation and then solve it

Worked Example
The diagram below shows a circle with centre O and two chords, PQ and RS.
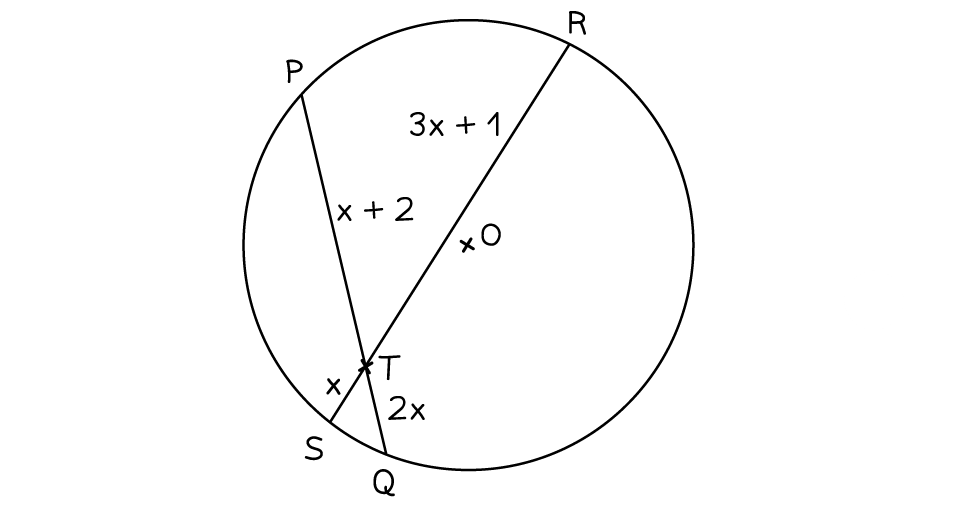
The chords PQ and RS meet at the point T.
(a) Find the possible value(s) of .
Using the intersecting chord theorem which states that
Fill in the information from the diagram
Simplify
Solve by factorising
Consider if both answers are possible
refers to a length, so cannot be zero
(b) Hence, or otherwise, write down the simplified ratio of PT:RT.
Method 1
Substitute the found value of into the expressions on the diagram for PT and RT
Write as a ratio PT:RT
Simplify
Method 2
Using the ratio form of the intersecting chord theorem, and the expressions on the diagram
This shows that the ratio of PT:RT is the same as the ratio of TS:TQ
Intersecting Chord Theorem (External)
What is the external case of the intersecting chord theorem?
The intersecting secant theorem is the mathematical name given to the external case of the intersecting chord theorem
A secant is a line which extends through a circle cutting the circumference at two points
It occurs when two chords intersect outside of the circle
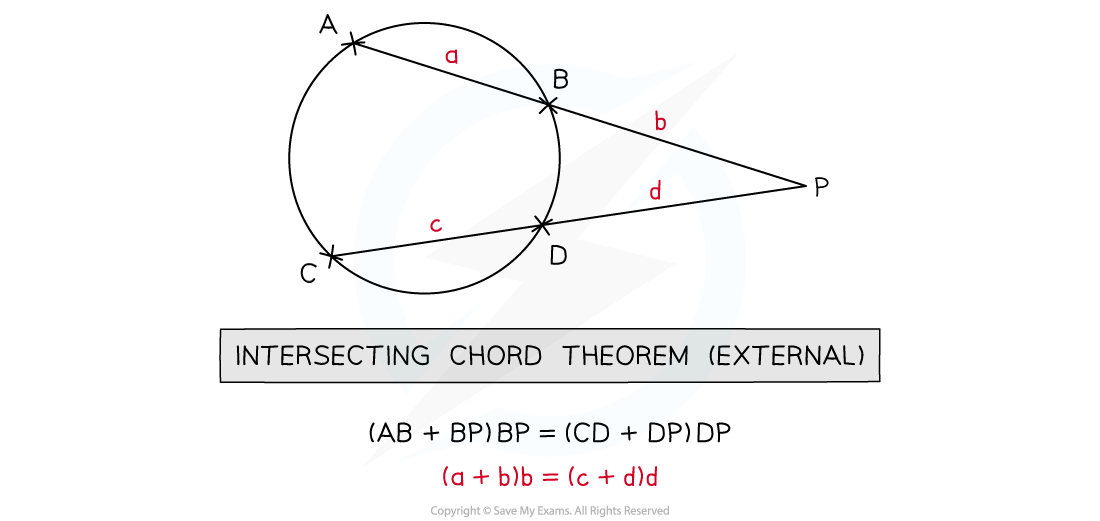
For two chords, AB and CD that extend and meet at point P outside of the circle
AP : PD ≡ CP : PB where AP = AB + BP and CP = CD + DP
Therefore (AB + BP) : PD ≡ (CD + DP) : PB
A more practical way to deal with most problems involving the intersecting secant theorem is
BP(AB + BP) = DP(CD + DP)
How do I use the intersecting secant theorem to solve problems?
If two chords intersect outside of a circle, you can find a missing length using the intersecting secant theorem
Substitute the values into the multiplication formula
BP(AB + BP) = DP(CD + DP)
If any of the lengths are algebraic expressions, an equation will be formed which can then be solved
Worked Example
In the diagram below, A, B, C and D are points on a circle.
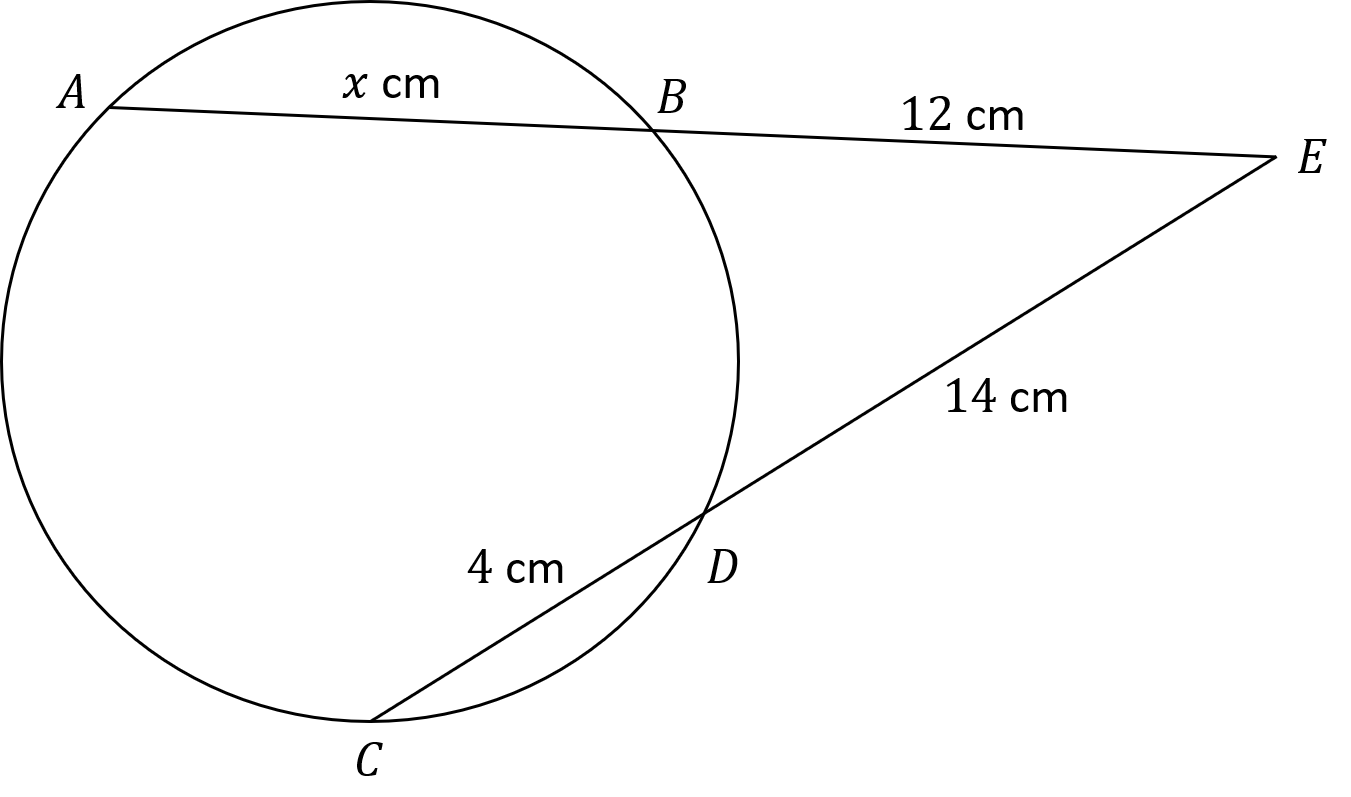
ABE and CDE are straight lines.
AB = cm
BE = 12 cm
CD = 4 cm
DE = 14 cm
Work out the value of .
Using the properties of Intersecting Chords (external intersection)
Or equivalently
Substitute the values from the diagram
Solve for
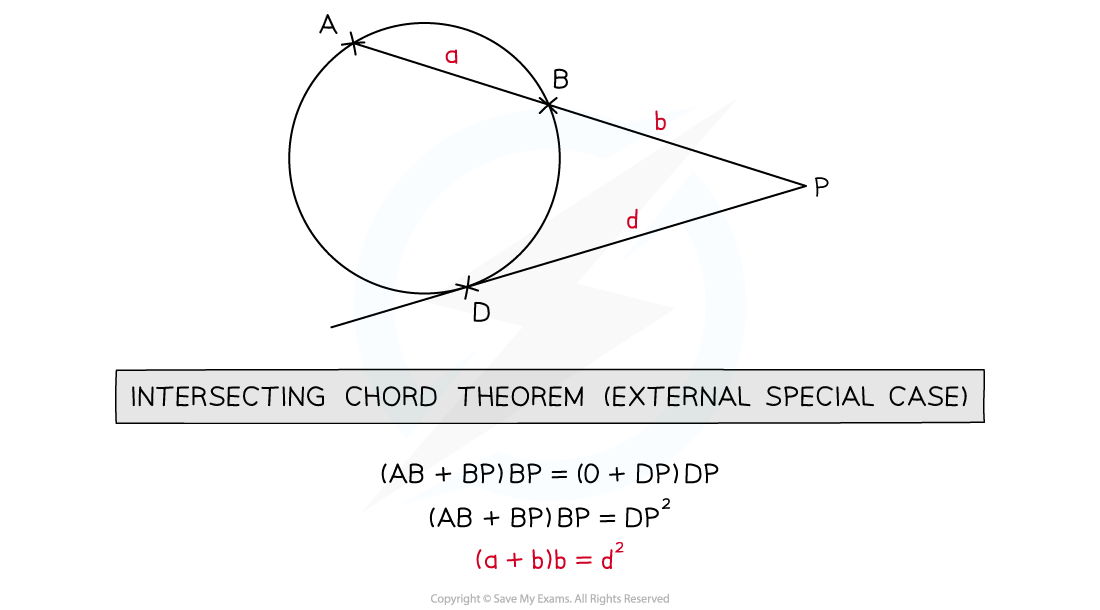
What if one of the lines is a tangent?
A special case of the intersecting secant theorem is when one of the lines is a tangent, rather than a secant
A tangent touches the circumference of the circle once, rather than intersecting it
In this case, one of the lengths becomes zero
BP(AB + BP) = DP(0 + DP)
BP(AB + BP) = DP2
Worked Example
In the diagram below, A, B, and C are points on a circle.
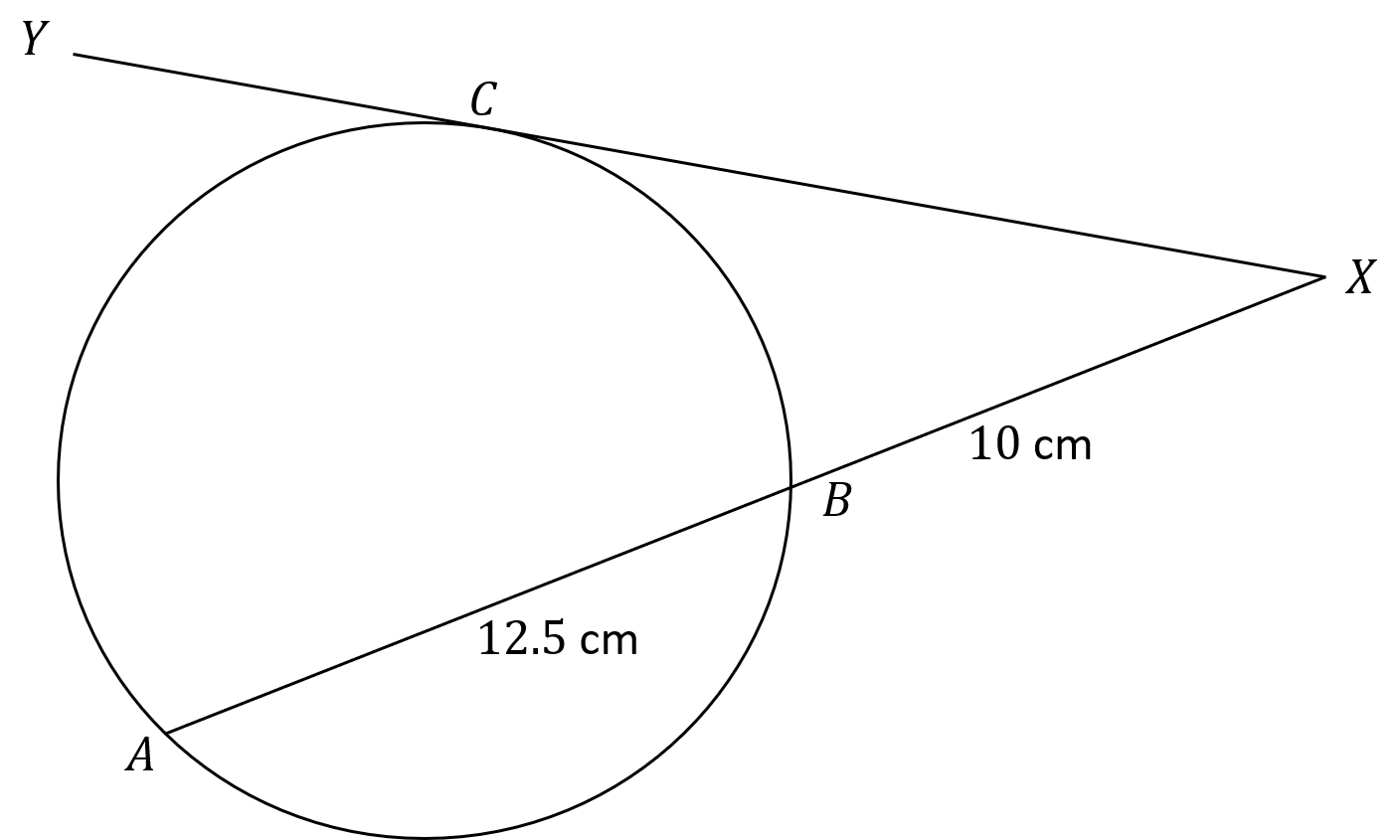
ABX is a straight line.
YCX is a tangent to the circle.
AB = 12.5 cm
BE = 10 cm
Work out the length of CX.
This is a special case of the properties of Intersecting Chords (external intersection), where one of the lines is a tangent, and therefore one of the line segments has length zero
Substitute the values from the diagram
Simplify and solve for
CX = 15 cm

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?