Geometric Proof with Vectors (DP IB Analysis & Approaches (AA)): Revision Note
Did this video help you?
Geometric proof with vectors
How can I prove geometric facts about a pair of vectors?
To show that two vectors are parallel:
Either show that they are scalar multiples of each other
Or show that their vector product is equal to the zero vector
To show that two vectors are perpendicular
Show that the scalar product of their vectors is equal to zero
To show that two vectors have equal length
Show that their magnitudes are equal
To show that two vectors have equal length and are parallel
Either show that they are equal
Or show that one is the negative of the other
They are the same lengths but in opposite directions
How can I use vectors to prove that line segments create a specific quadrilateral?
You can represent each side of a quadrilateral using displacement vectors
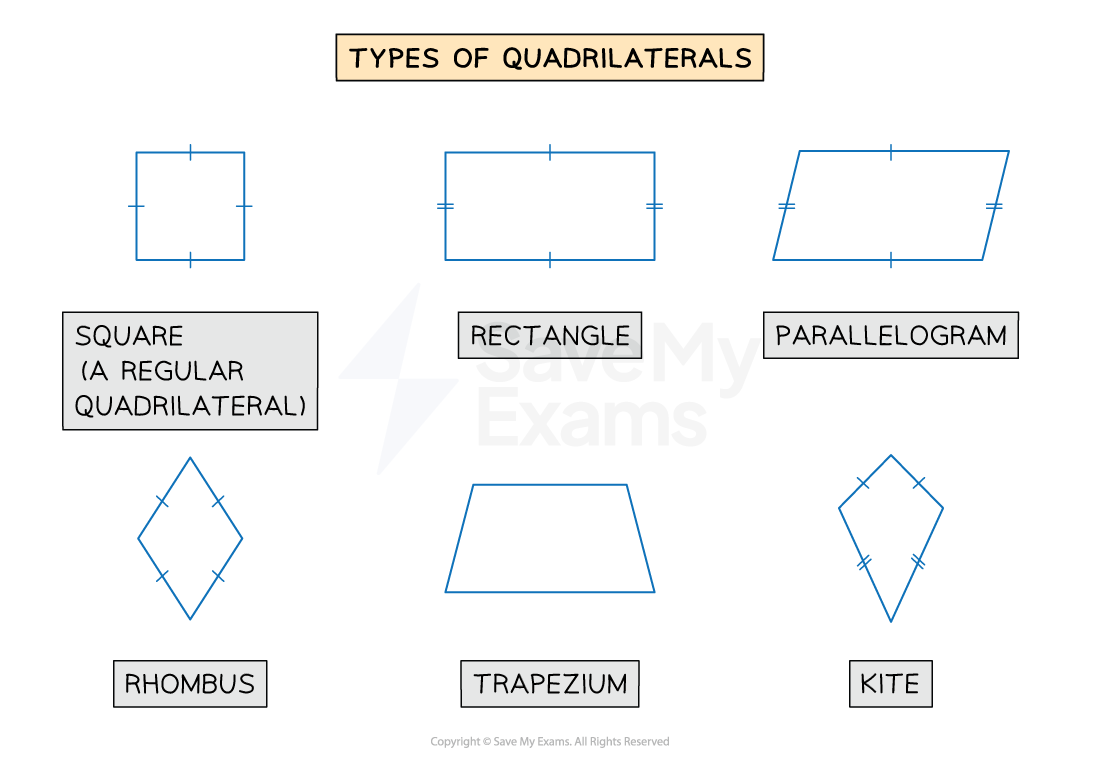
To prove that a 2D shape is a parallelogram
Either show that the vectors for both pairs of opposite sides are equal or opposite vectors
To prove that a 2D shape is a rectangle
Show that the vectors for both pairs of opposite sides are equal or opposite vectors
And show that the vectors for a pair of adjacent sides are perpendicular
To prove a 2D shape is a rhombus
Show that the vectors for both pairs of opposite sides are equal or opposite vectors
And show the vectors for a pair of adjacent sides have equal lengths
To prove a 2D shape is a square
Show that the vectors for both pairs of opposite sides are equal or opposite vectors
And show the vectors for a pair of adjacent sides have equal lengths
And show that the vectors for a pair of adjacent sides are perpendicular
To prove a 2D shape is a kite
Show that the vectors for both diagonals are perpendicular
And show that no pairs of vectors are parallel
To prove a 2D shape is a kite
Show that the vectors for only one pair of opposite sides are parallel
How do I find midpoints and points on a line using vectors?
If the point
is the midpoint of the line segment
then
More generally, if the point
divides a line segment
into the ratio
then
The position vector of the midpoint of points
and
is
is the position vector of
is the position vector of
How can vectors be used to prove that three points are collinear?
Three points are collinear if they all lie on the same line
To show that the points
,
and
are collinear
Show that any two of
,
and
are parallel
Examiner Tips and Tricks
Always sketch a diagram when working with vectors.
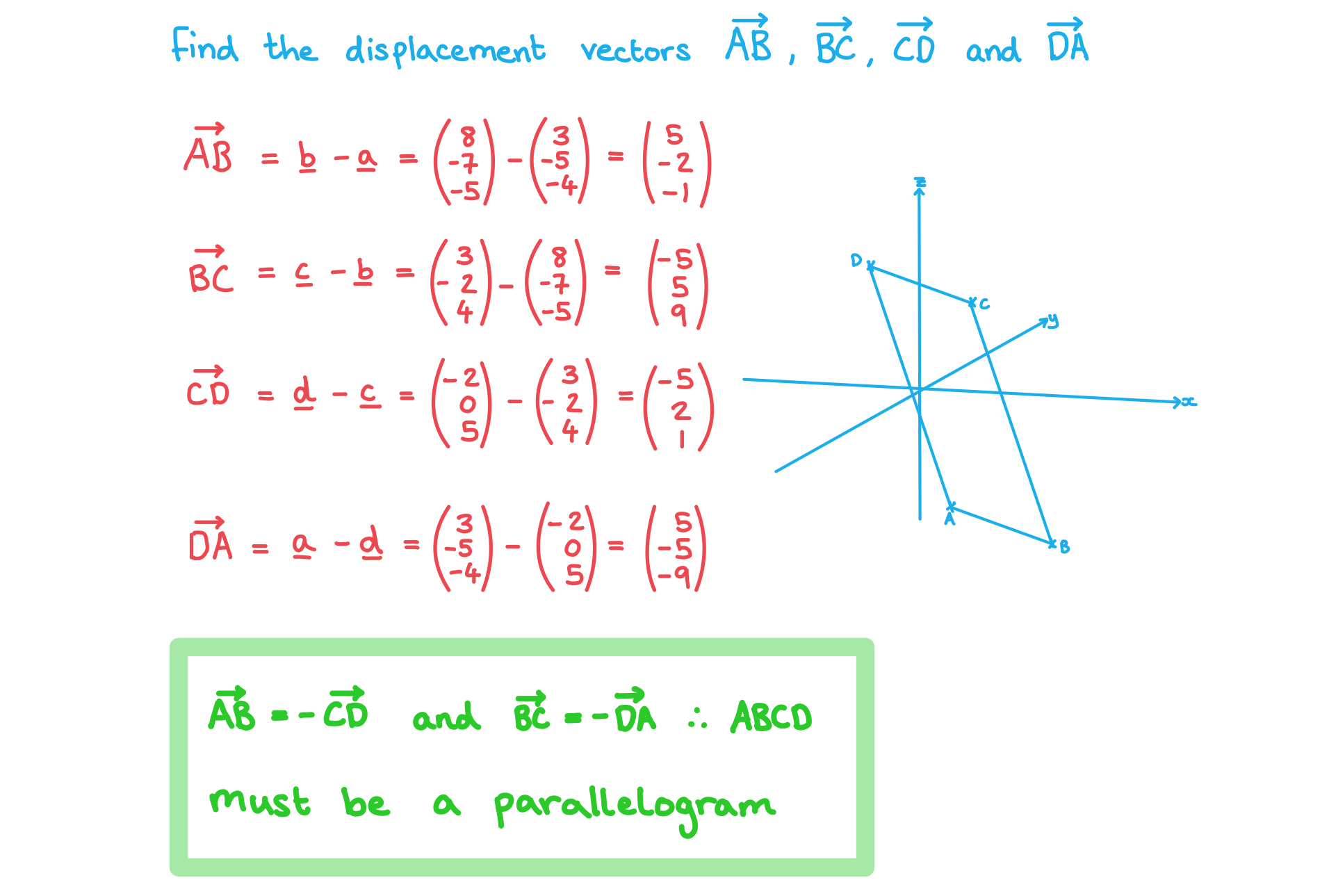
Worked Example
Use vectors to prove that the points A, B, C and D with position vectors ,
,
and
respectively, are the vertices of a parallelogram.

Unlock more, it's free!
Did this page help you?