Bayes' Theorem (DP IB Analysis & Approaches (AA)): Revision Note
Did this video help you?
Bayes' theorem
What is Bayes’ theorem?
Bayes’ theorem allows you switch the order of conditional probabilities
If you know
,
and
then Bayes’ theorem allows you to find
Essentially if you have a tree diagram you will already know the conditional probabilities of the second branches
Bayes’ theorem allows you to find the conditional probabilities if you switch the order of the events
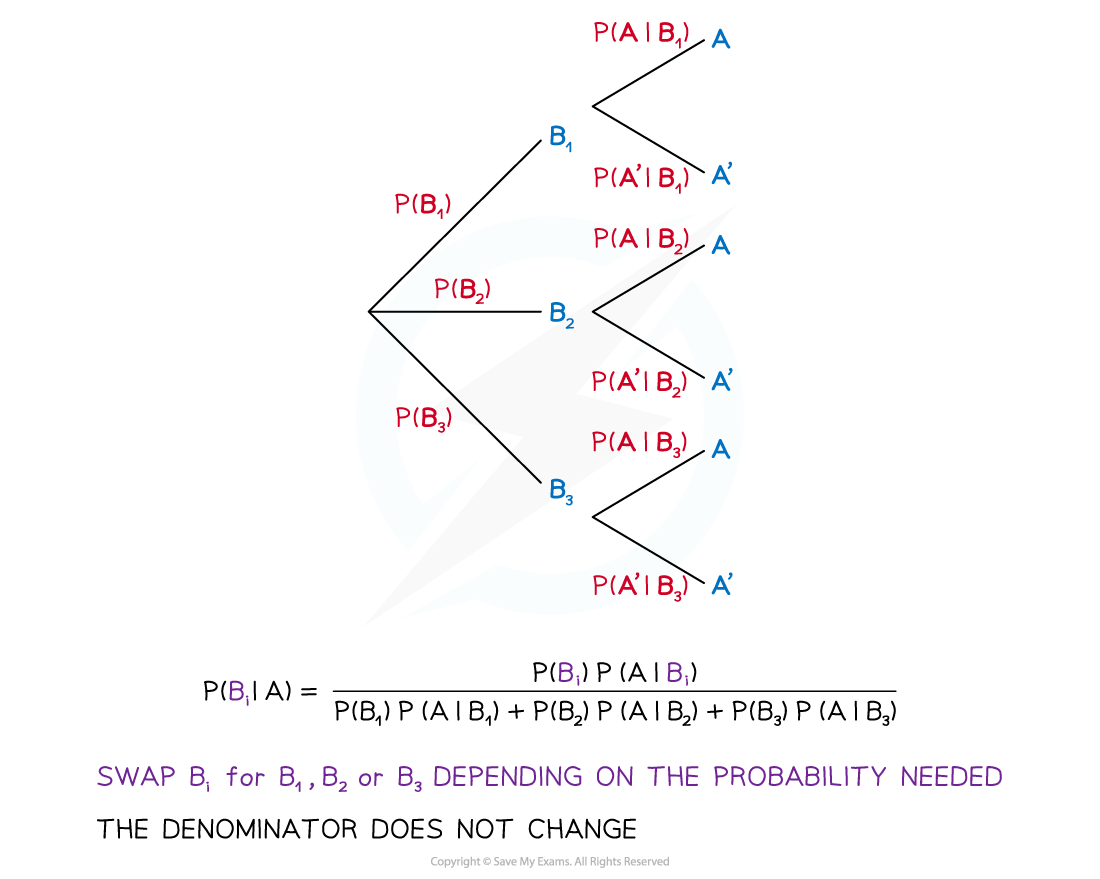
For any two events A and B Bayes’ theorem states:
This is given in the exam formula booklet
This formula is derived using the formulae:
and
Bayes’ theorem can be extended to mutually exclusive events B1, B2, ..., Bn and any other event A
In your exam you will have a maximum of three mutually exclusive events
This is given in the exam formula booklet
How do I calculate conditional probabilities using Bayes’ theorem?
Start by drawing a tree diagram
Label B1 & B2 (& B3 if necessary) on the first set of branches
Label A & A’ on the second set of branches
The questions will give you enough information to label the probabilities on this tree
Identify the probabilities needed to use Bayes’ theorem
The probabilities will come in pairs:
and
Examiner Tips and Tricks
In an exam you are less likely to make a mistake when using the formula if you draw a tree diagram first.
Worked Example
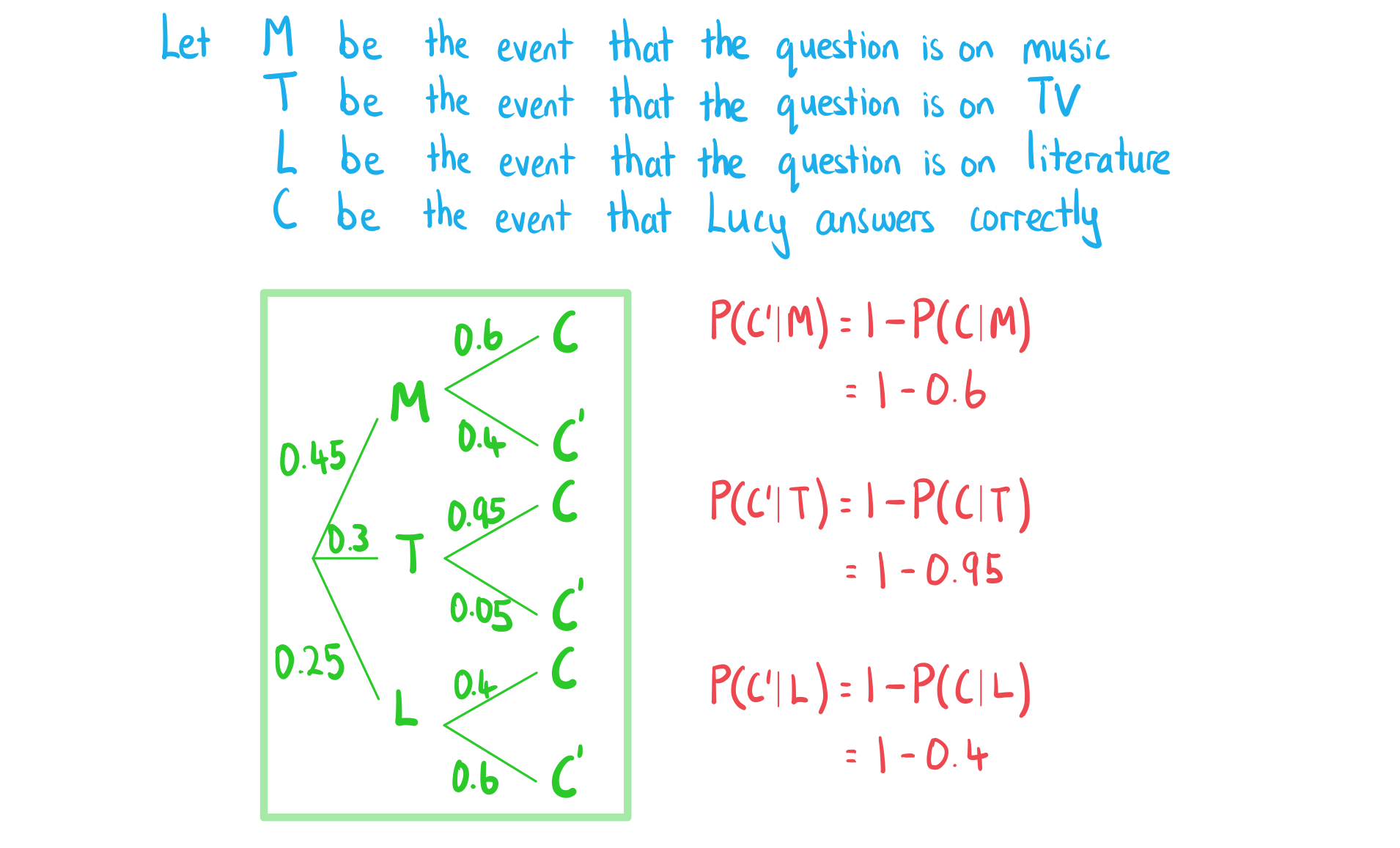
Lucy is doing a quiz. For each question there’s a 45% chance that it is about music, 30% chance that it is about TV and 25% chance that it is about literature. The probability that Lucy answers a question correctly is 0.6 for music, 0.95 for TV and 0.4 for literature.
a) Draw a tree diagram to represent this information.
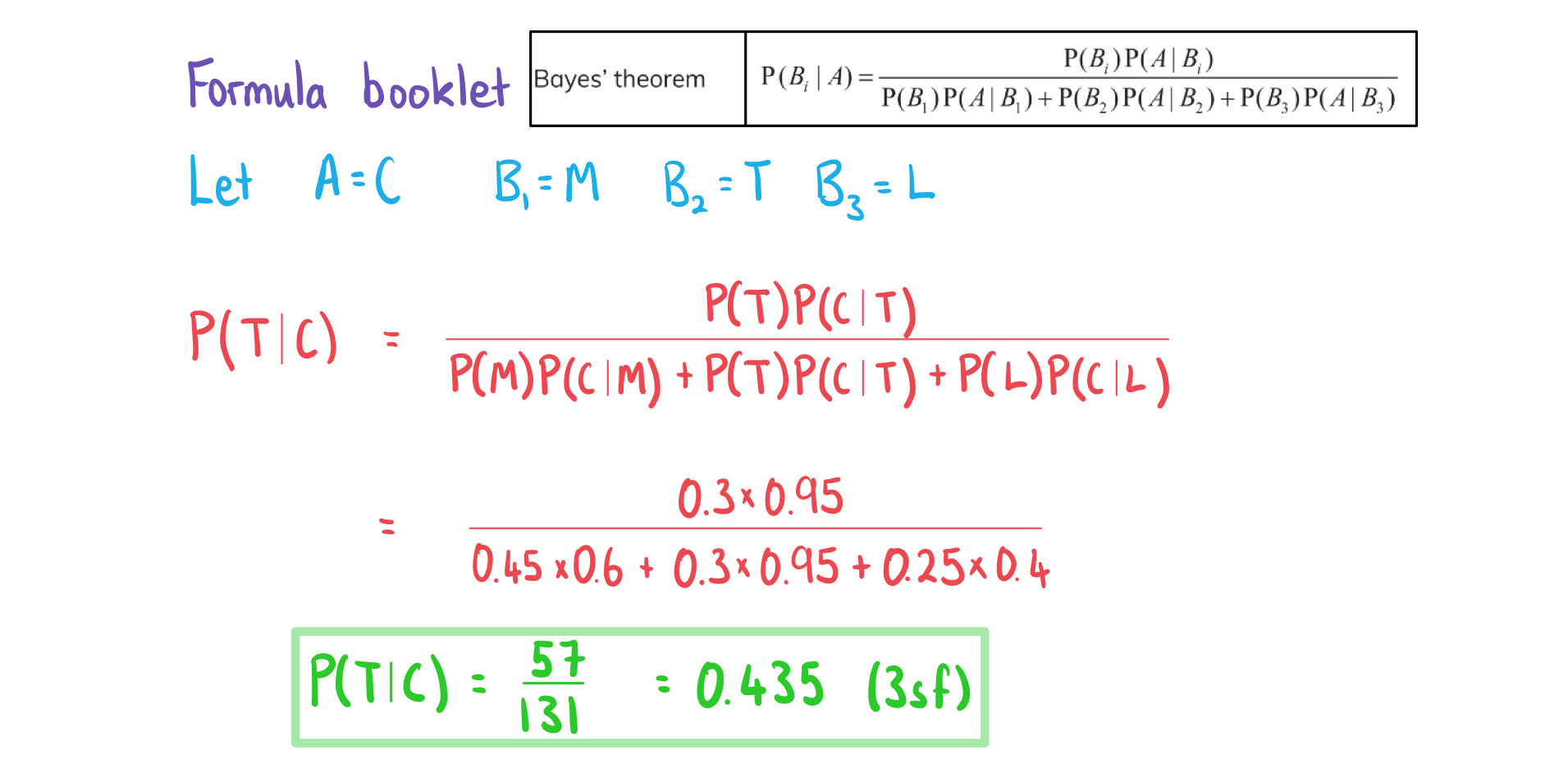
b) Given that Lucy answered a question correctly, find the probability that it was about TV.

Unlock more, it's free!
Did this page help you?