IBMathsDPAnalysis & Approaches (AA)HLRevision Notes1. Number & AlgebraBinomial TheoremExtension of The Binomial Theorem

Extension of The Binomial Theorem (DP IB Analysis & Approaches (AA)): Revision Note

Amber

Author

Amber

Last updated

Did this video help you?

Binomial Theorem: Fractional & Negative Indices

How do I use the binomial theorem for fractional and negative indices?

  • The formula given in the formula booklet for the binomial theorem applies to positive integers only

    • open parentheses a plus b close parentheses to the power of n equals a to the power of n plus blank scriptbase straight C subscript 1 end scriptbase presubscript blank presuperscript n a to the power of n minus 1 end exponent b plus horizontal ellipsis plus scriptbase straight C subscript r end scriptbase presubscript blank presuperscript n a to the power of n minus r end exponent b to the power of r plus horizontal ellipsis plus b to the power of n

    • where scriptbase straight C subscript r end scriptbase presubscript blank presuperscript n equals fraction numerator n factorial over denominator r factorial open parentheses n minus r close parentheses factorial end fraction

  • For negative or fractional powers the expression in the brackets must first be changed such that the value for a is 1

    • open parentheses a plus b close parentheses to the power of n equals a to the power of n open parentheses 1 plus b over a blank close parentheses to the power of n 

    • open parentheses a plus b close parentheses to the power of n equals a to the power of n open parentheses 1 plus n open parentheses b over a close parentheses space plus fraction numerator n left parenthesis n minus 1 right parenthesis over denominator 2 factorial end fraction open parentheses b over a close parentheses squared space plus space... space space blank close parentheses space comma space n element of straight rational numbers

    • This is given in the formula booklet 

  • If a = 1 and b = x the binomial theorem is simplified to

    • open parentheses 1 plus x close parentheses to the power of n equals 1 plus n x plus fraction numerator n open parentheses n minus 1 close parentheses over denominator 2 factorial end fraction x squared plus fraction numerator n open parentheses n minus 1 close parentheses open parentheses n minus 2 close parentheses over denominator 3 factorial end fraction x cubed plus horizontal ellipsis comma blank n element of straight rational numbers blank comma double-struck    open vertical bar x close vertical bar less than 1

    • This is not in the formula booklet, you must remember it or be able to derive it from the formula given

  • You need to be able to recognise a negative or fractional power

    • The expression may be on the denominator of a fraction

      • 1 over open parentheses a plus b close parentheses to the power of n equals open parentheses a plus b close parentheses to the power of negative n end exponent

    • Or written as a surd

      • n-th root of open parentheses a plus b close parentheses to the power of m end root equals open parentheses a plus b close parentheses to the power of m over n end exponent

  • For n blank not an element of blank straight natural numbers the expansion is infinitely long

    • You will usually be asked to find the first three terms

  • The expansion is only valid for open vertical bar x close vertical bar less than 1

    • This means negative 1 less than x less than 1

    • This is known as the interval of convergence

    • For an expansion open parentheses a blank plus blank b x close parentheses to the power of n the interval of convergence would be negative a over b less than x less than a over b

How do we use the binomial theorem to estimate a value?

  • The binomial expansion can be used to form an approximation for a value raised to a power

  • Since open vertical bar x close vertical bar less than 1 higher powers of x will be very small

    • Usually only the first three or four terms are needed to form an approximation

    • The more terms used the closer the approximation is to the true value

  • The following steps may help you use the binomial expansion to approximate a value

    • STEP 1: Compare the value you are approximating to the expression being expanded

      • e.g.  left parenthesis 1 space minus space x right parenthesis to the power of 1 half end exponent space equals space 0.96 to the power of 1 half end exponent

    • STEP 2: Find the value of x by solving the appropriate equation

      • e.g.  table attributes columnalign right center left columnspacing 0px end attributes row blank blank blank row cell 1 space minus space x space end cell equals cell space 0.96 end cell row cell space x space end cell equals cell space 0.04 end cell end table

  • STEP 3: Substitute this value of x into the expansion to find the approximation

    • e.g.  1 minus 1 half open parentheses 0.04 close parentheses minus 1 over 8 blank open parentheses 0.04 close parentheses squared equals 0.9798

  • Check that the value of x is within the interval of convergence for the expression

    • If x is outside the interval of convergence then the approximation may not be valid

Examiner Tips and Tricks

  • Students often struggle with the extension of the binomial theorem questions in the exam, however the formula is given in the formula booklet 

    • Make sure you can locate the formula easily and practice substituting values in

    • Mistakes are often made with negative numbers or by forgetting to use brackets properly

      • Writing one term per line can help with both of these

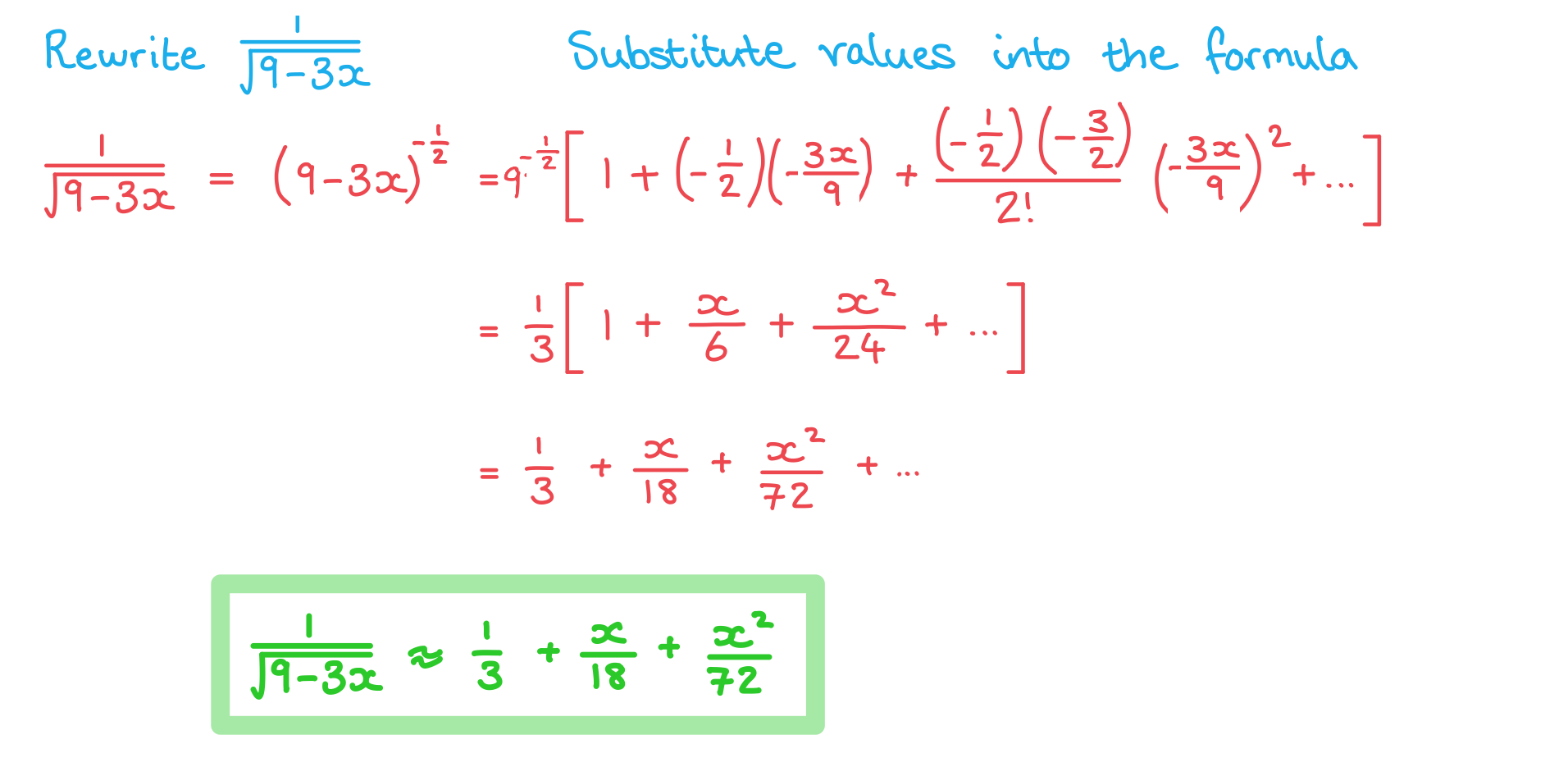
Worked Example

Consider the binomial expansion of  fraction numerator 1 over denominator square root of 9 blank minus blank 3 x end root end fraction.

a) Write down the first three terms.

1-6-2-ib-hl-aa-ext-bin-theorem-we-a

b) State the interval of convergence for the complete expansion.

1-6-2-ib-hl-aa-ext-bin-theorem-we-b

c) Use the terms found in part (a) to estimate begin mathsize 14px style fraction numerator 1 over denominator square root of 10 end fraction end style . Give your answer as a fraction.

1-6-2-ib-hl-aa-ext-bin-theorem-we-c

You've read 1 of your 5 free revision notes this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

  • I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

    Beth
    IGCSE Student

  • This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

    Fathima
    A Level Student
Test yourselfFlashcards

Did this page help you?

Previous:Binomial TheoremNext:Counting Principles
Amber

Author: Amber

Expertise: Maths Content Creator

Amber gained a first class degree in Mathematics & Meteorology from the University of Reading before training to become a teacher. She is passionate about teaching, having spent 8 years teaching GCSE and A Level Mathematics both in the UK and internationally. Amber loves creating bright and informative resources to help students reach their potential.

Download notes on Extension of The Binomial Theorem