Stretches of Graphs (Edexcel IGCSE Maths A) : Revision Note

Written by: Mark Curtis

Reviewed by: Dan Finlay

Updated on 31 March 2025

Did this video help you?

Stretches of Graphs

What are stretches of graphs?

Stretches of graphs are a type of transformation that pushes points away from, or towards, the $x$ -axis or $y$ -axis
- Graphs look like they have been stretched or squashed
  - either horizontally or vertically

A graph being stretched vertically (on the left) or horizontally (on the right). — **A graph being stretched vertically (left) or horizontally (right)**

How do I stretch graphs?

Let $y = f (x)$ be the equation of the original graph

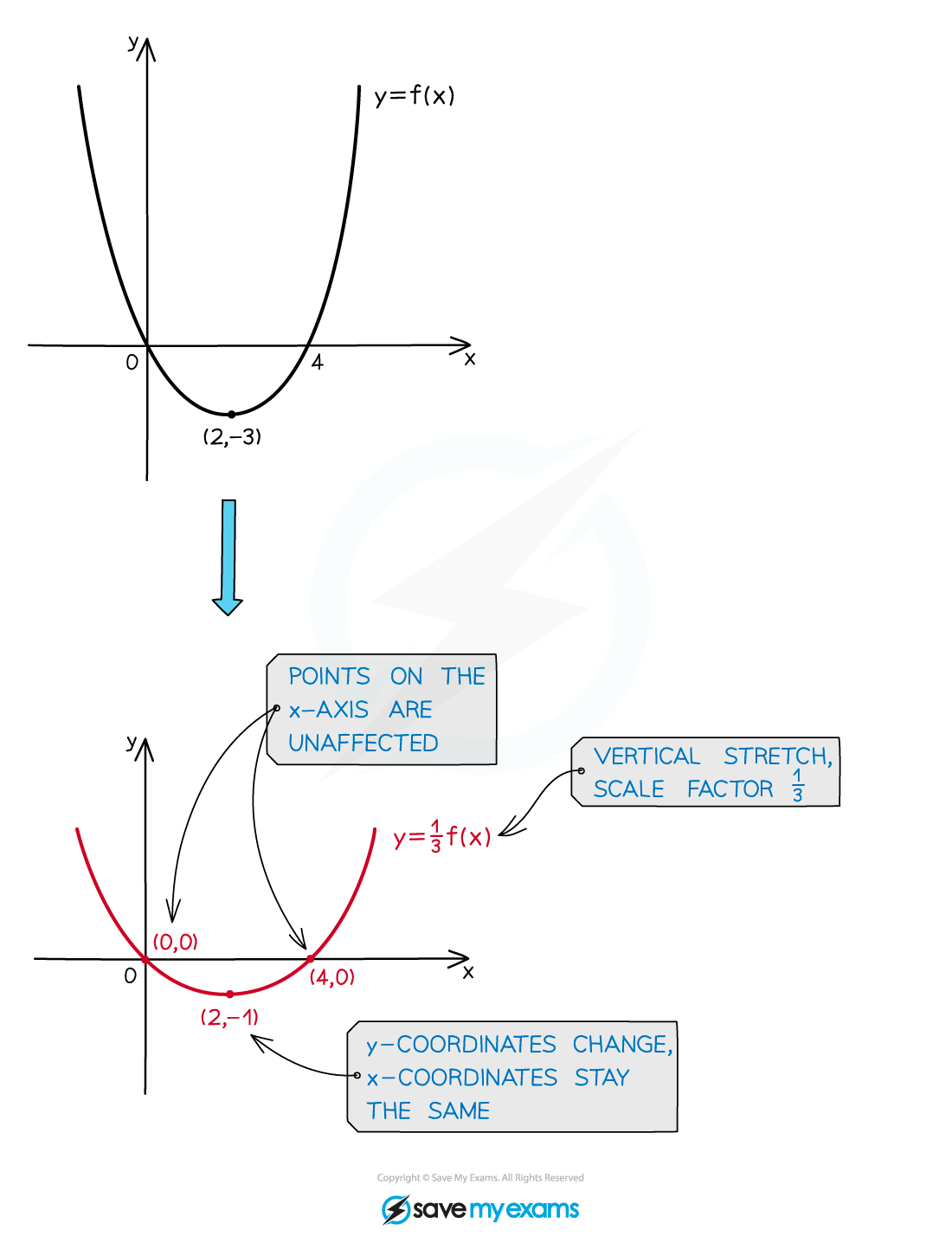
Vertical stretches: y=af(x)

$y = a f (x)$ is a vertical stretch (in the $y$ -direction) of scale factor $a$
- The $x$ -coordinates stay the same but the $y$ coordinates are multiplied by $a$
- Points appear to move parallel to the $y$ -axis
  - either stretching vertically away from the $x$ -axis if $a > 1$
  - or squashing vertically towards the $x$ -axis if $0 < a < 1$
- Points on the $x$ -axis stay where they are

Graph showing the function y = f(x) and its transformation y = (1/3)f(x), indicating vertical stretch by a factor of 1/3. Points (2, -3) and (2, -1) shown.

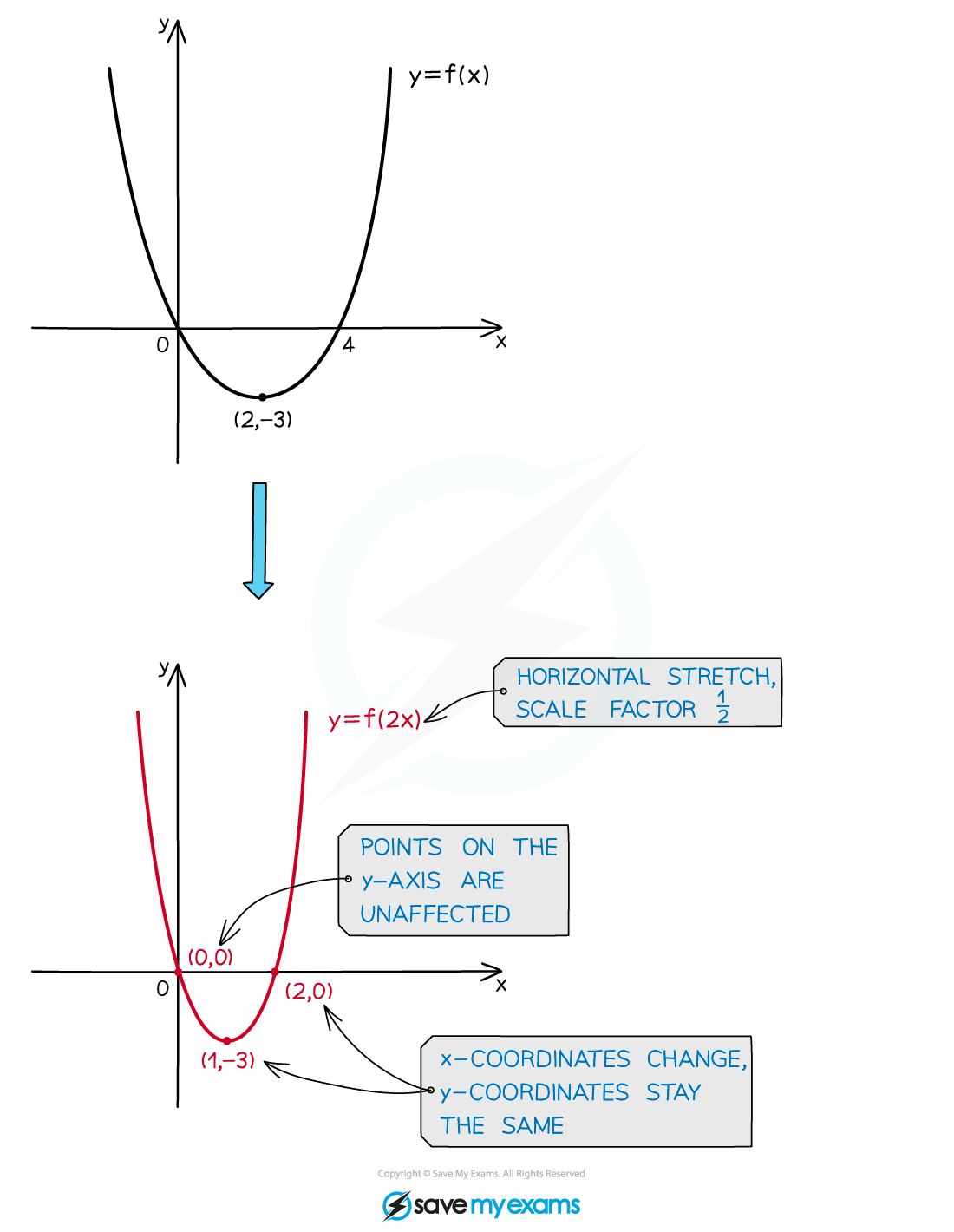
Horizontal stretches: y=fa(x)

$y = f (a x)$ is a horizontal stretch (in the $y$ -direction) of scale factor $\frac{1}{a}$ (not $a$ )
- The $y$ -coordinates stay the same but the $x$ coordinates are multiplied by $\frac{1}{a}$ (divided by $a$ )
- Points appear to move parallel to the $x$ -axis
  - either squashing horizontally towards the $y$ -axis if $a > 1$
  - or stretching horizontally away from the $y$ -axis if $0 < a < 1$
- Points on the $y$ -axis stay where they are
This means $y = f (2 x)$ is more like a horizontal squash of scale factor 2
- though the correct way to say this is a horizontal stretch of scale factor $\frac{1}{2}$
  - This also means that $f (\frac{x}{2})$ is a horizontal stretch of scale factor 2

Stretching a graph horizontally by scale factor one half. y-coordinates stay in same place, x-coordinates change

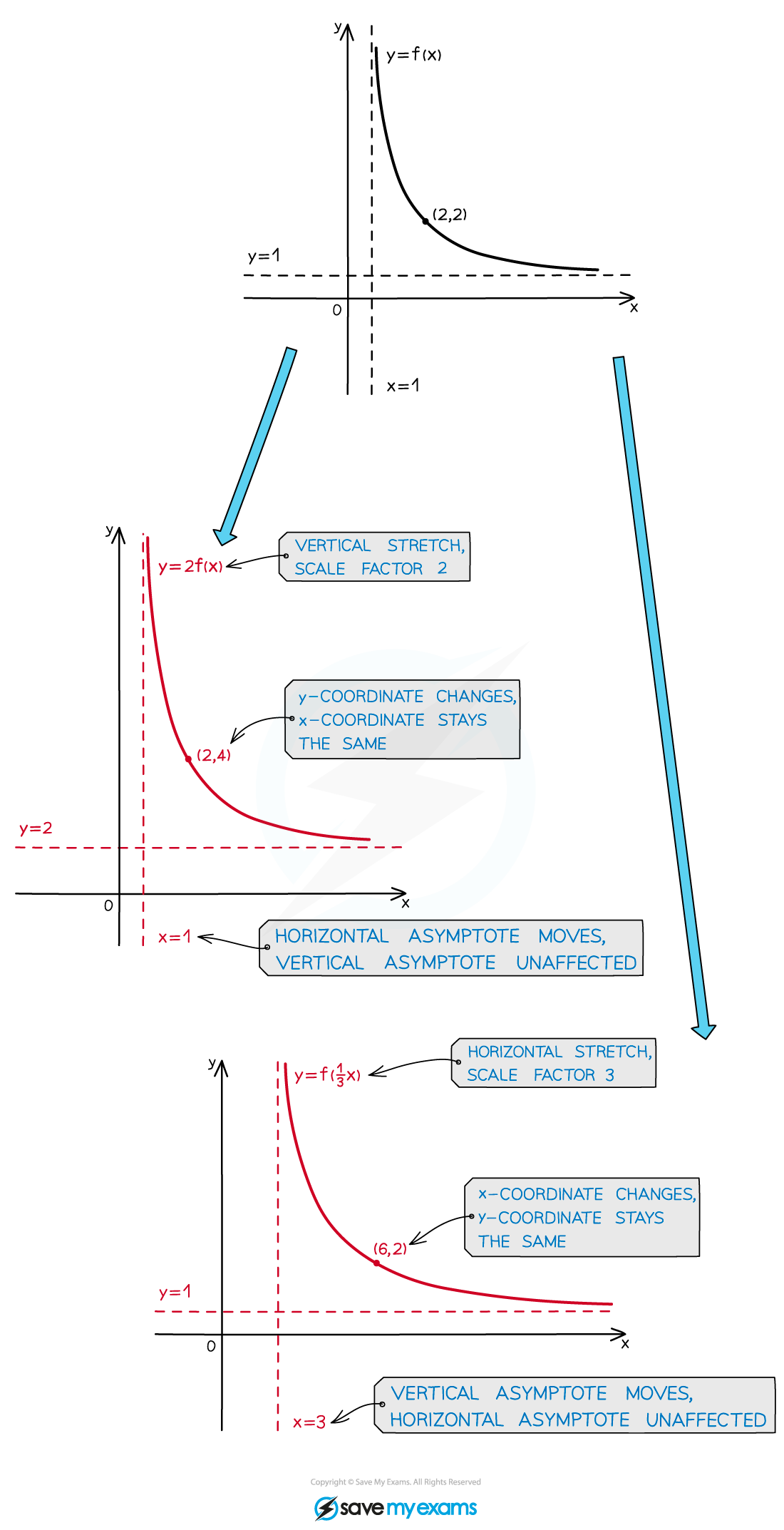
What happens to asymptotes when a graph is stretched?

Any asymptotes of $f (x)$ are also stretched

A diagram shows transformations of the function y = f(x). It illustrates vertical and horizontal stretches, their effects on asymptotes, and coordinate changes.

How does a stretch affect the equation of the graph?

When a graph is stretched, you can change its equation algebraically
- There is no need to sketch the graph
Stretching vertically by a scale factor of 3 puts a 3 in front of the whole equation
- For example, $y = x^{2} + 2 x$ becomes $y = 3 (x^{2} + 2 x)$
  - This simplifies to $y = 3 x^{2} + 6 x$
Stretching horizontally by a scale factor of $\frac{1}{3}$ ("squashing horizontally" by a scale factor of 3) replaces any $x$ with $(3 x)$ in the equation
- For example, $y = x^{2} + 2 x$ becomes $y = {(3 x)}^{2} + 2 (3 x)$
  - This simplifies to $y = 9 x^{2} + 6 x$

How do I apply a combined stretch?

The graph of $y = b f (a x)$ is a combined stretch_, both horizontally and vertically
- It does not matter which order you apply these in
  - For example, a horizontal stretch of scale factor $\frac{1}{a}$ followed by a vertical stretch of scale factor $b$

Worked Example

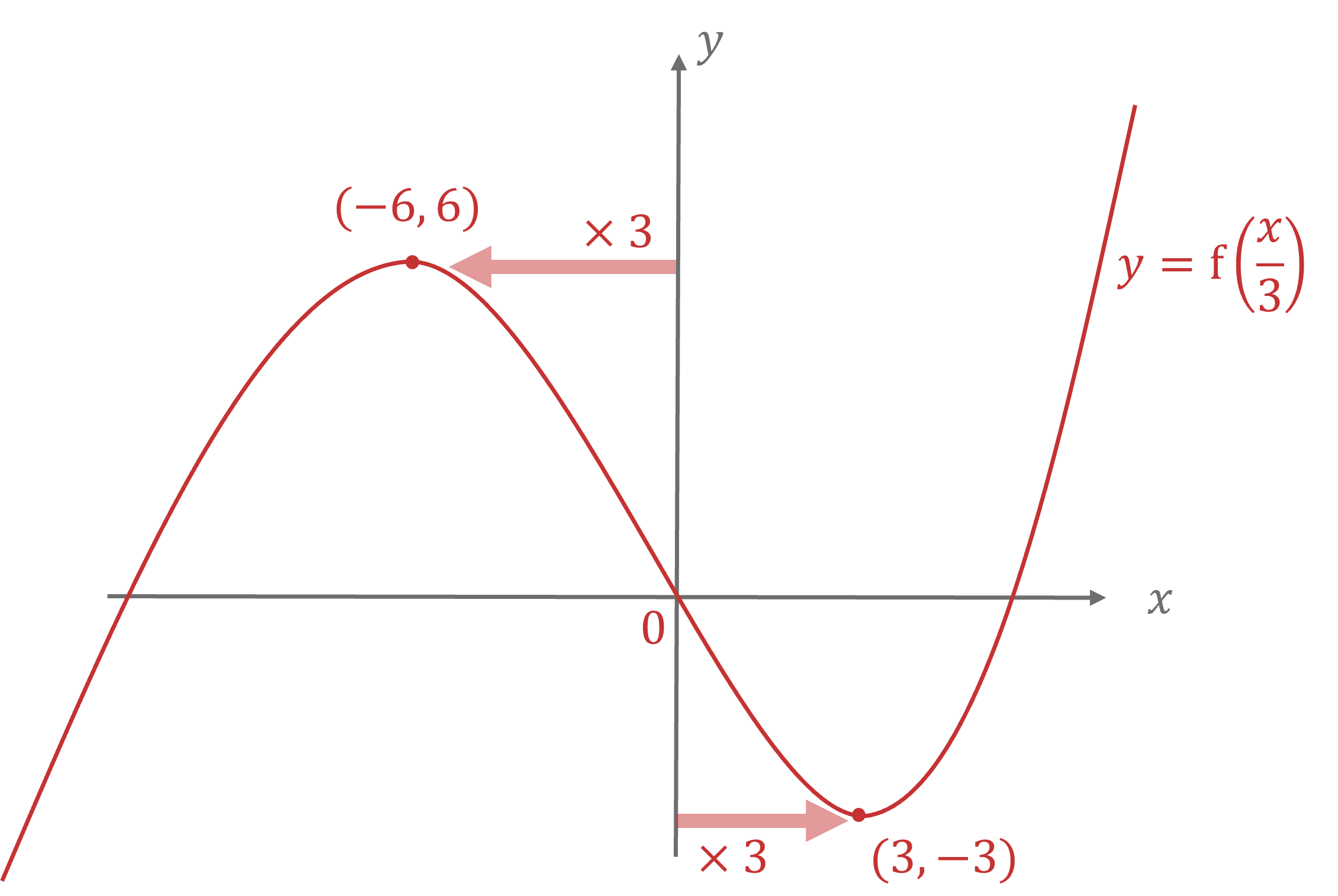
The diagram below shows the graph of $y = f (x)$ .

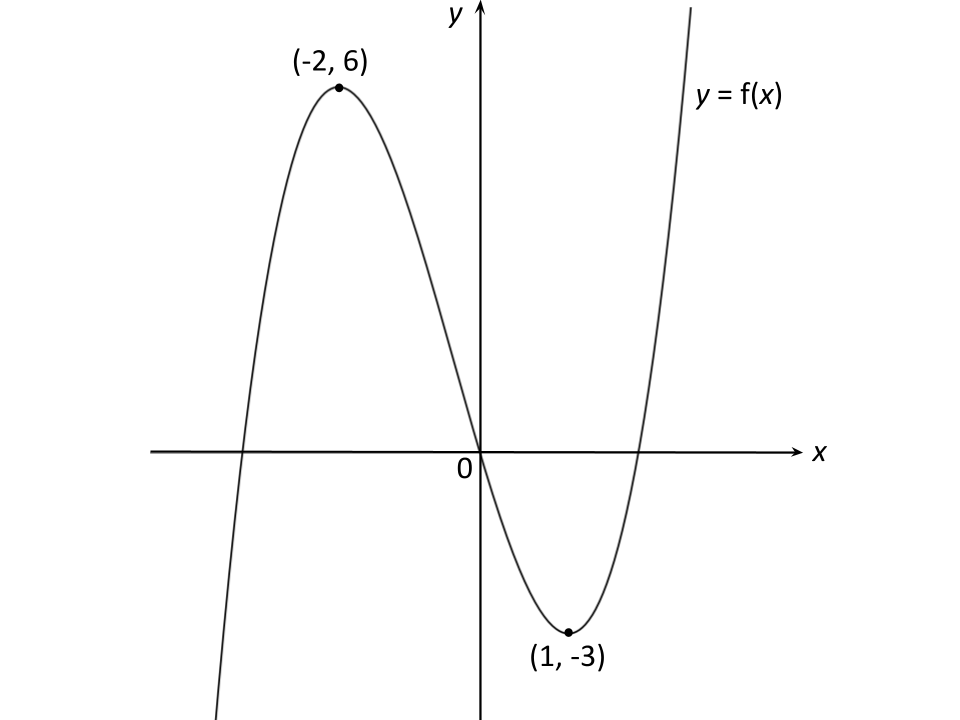
Sketch the graph of $y = 2 f (\frac{x}{3})$ .

$f (\frac{x}{3})$ represents a horizontal stretch of scale factor $\frac{1}{(\frac{1}{3})} = 3$
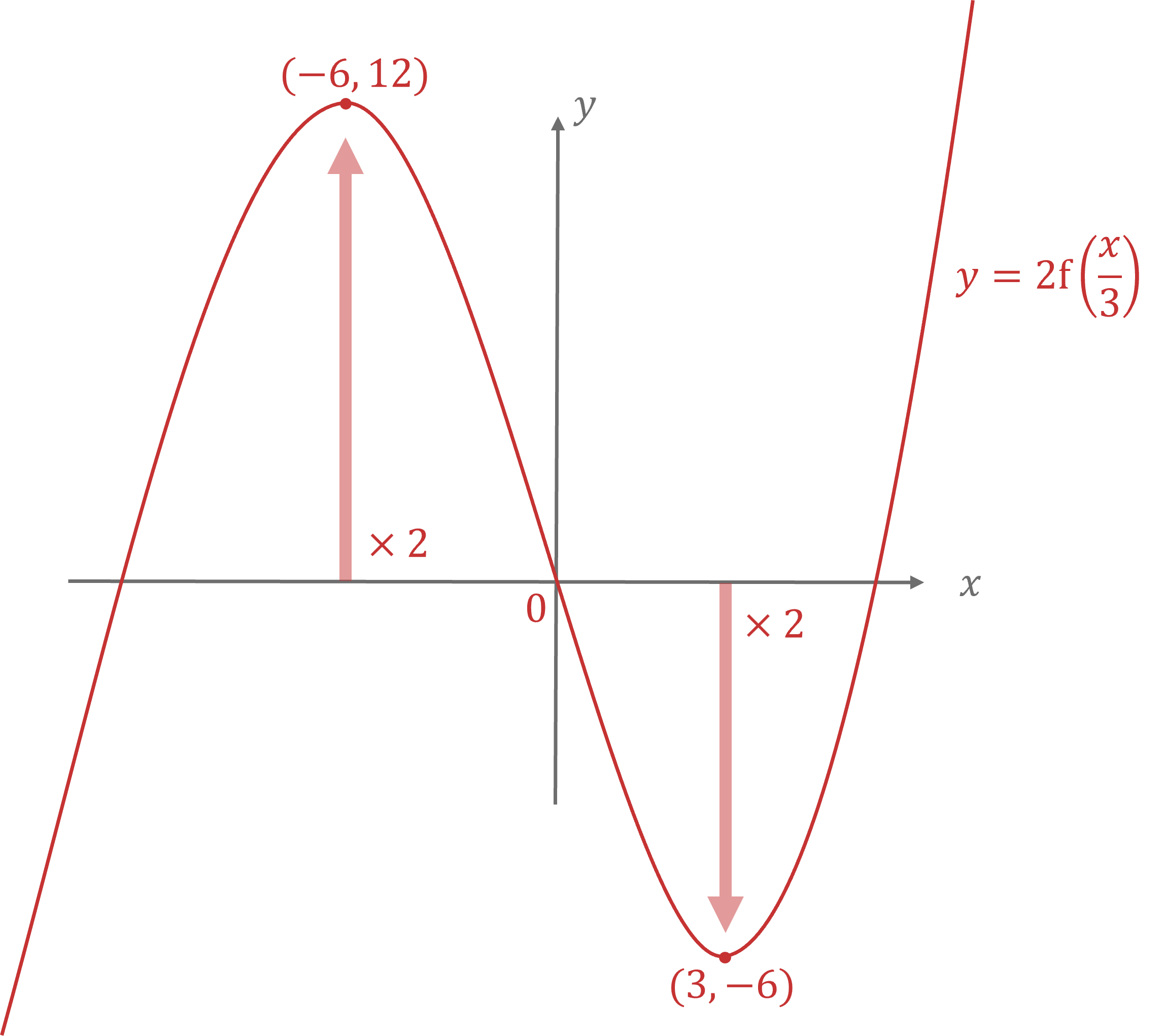
And $2 f (. . .)$ represents a vertical stretch of scale factor 2

Apply these in any order, e.g. start with the horizontal stretch, scale factor 3

Graph of the function y = f(x/3) with key points labeled (-6, 6) and (3, -3).

Now apply a vertical stretch of scale factor 2

Graph of the function y=2f(x/3) showing a curve with marked points at (-6,12) and (3,-6) and vertical stretches by a factor of 2 along y-axis.

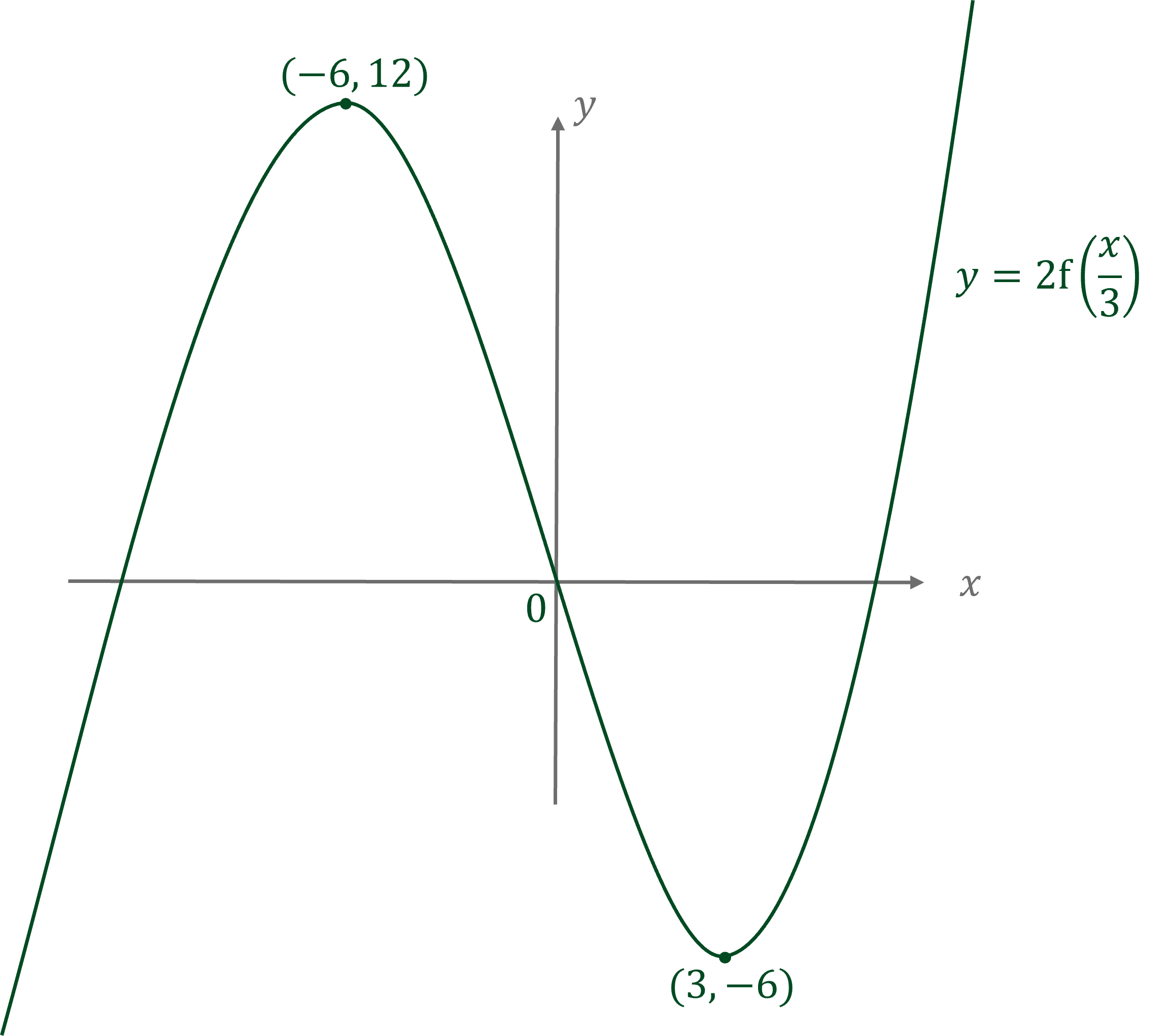
Show the coordinates of the new points clearly

Graph of the function y = 2f(x/3) with a peak at (-6,12) and a trough at (3,-6), crossing the y-axis at 0. Axes labelled x and y.

You've read 1 of your 5 free revision notes this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.
Beth
IGCSE Student
This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!
Fathima
A Level Student
Incredible! SO worth my money, the revision notes have everything I need to know and are so easy to understand. I actually enjoy revising! It makes me feel a lot more confident for my GCSEs in a few months.
Kate
GCSE Student
Absolutely brilliant, both my girls used it for A levels and GCSE. It's saves on paper copies, also beneficial exam questions ranked from easy to hard. It's removed a lot of stress from the exams.
Sameera
Parent
Just to say that your resources are the best I have seen and I have been teaching chemistry at different levels for about 40 years
Mark
Chemistry Teacher
Excellent
Read more reviews

Test yourself Flashcards

Did this page help you?

Previous:Reflections of GraphsNext:Differentiation

Numbers & the Number System

Number Toolkit

Mathematical Symbols

Order of Operations (BIDMAS/BODMAS)

Negative Numbers

Money Calculations

Addition & Subtraction

Multiplication & Division

Operations with Decimals

Related Calculations

Set Notation & Venn Diagrams

Set Notation & Venn Diagrams

Prime Factors, HCF & LCM

Types of Number

Prime Factor Decomposition

Uses of Prime Factor Decomposition

HCF & LCM

Powers, Roots & Standard Form

Powers & Roots

Laws of Indices

Converting To & From Standard Form

Operations with Standard Form

Fractions

Basic Fractions

Mixed Numbers & Improper Fractions

Adding & Subtracting Fractions

Multiplying & Dividing Fractions

Percentages

Basic Percentages

Percentage Increases & Decreases

Reverse Percentages

Compound Interest & Depreciation

Compound Interest

Depreciation

Fractions, Decimals & Percentages

Converting Fractions, Decimals & Percentages

Recurring Decimals

Ordering Fractions, Decimals & Percentages

Rounding, Estimation & Bounds

Rounding & Estimation

Upper & Lower Bounds

Surds

Simplifying Surds

Rationalising Denominators

Using a Calculator

Using a Calculator

Ratio Toolkit

Introduction to Ratios

Sharing in a Ratio

Working with Proportion

Ratio Problem Solving

Ratios & FDP

Multiple Ratios

Exchange Rates & Best Buys

Exchange Rates

Best Buys

Direct & Inverse Proportion

Direct Proportion

Inverse Proportion

Equations, Formulae & Identities

Algebra Toolkit

Algebraic Notation

Algebraic Vocabulary

Substitution

Collecting Like Terms

Algebraic Roots & Indices

Algebraic Roots & Indices

Expanding Brackets

Expanding & Simplifying Single Brackets

Expanding Double Brackets

Expanding Triple Brackets

Factorising

Factorising Out Terms

Factorising by Grouping

Factorising Simple Quadratics

Factorising Harder Quadratics

Difference Of Two Squares

Deciding the Factorisation Method

Completing the Square

Completing the Square