Language of Sequences & Series (DP IB Analysis & Approaches (AA)): Revision Note
Did this video help you?
Language of sequences & series
What is a sequence?
A sequence is an ordered set of numbers with a rule for finding all of the numbers in the sequence
For example 1, 3, 5, 7, 9, … is a sequence with the rule ‘start at one and add two to each number’
The numbers in a sequence are often called terms
The terms of a sequence are often referred to by the letter
with a subscript
So in the sequence above,
The nth term is
If a formula for the nth term is given, then each term in the sequence can be found by substituting the term number,
, into the formula
e.g. if
then the 5th term is
What is a series?
You get a series by summing up the terms in a sequence
E.g. For the sequence 1, 3, 5, 7, … the associated series is 1 + 3 + 5 + 7 + …
The notation
is used to refer to the sum of the first
terms in a series
For the series above,
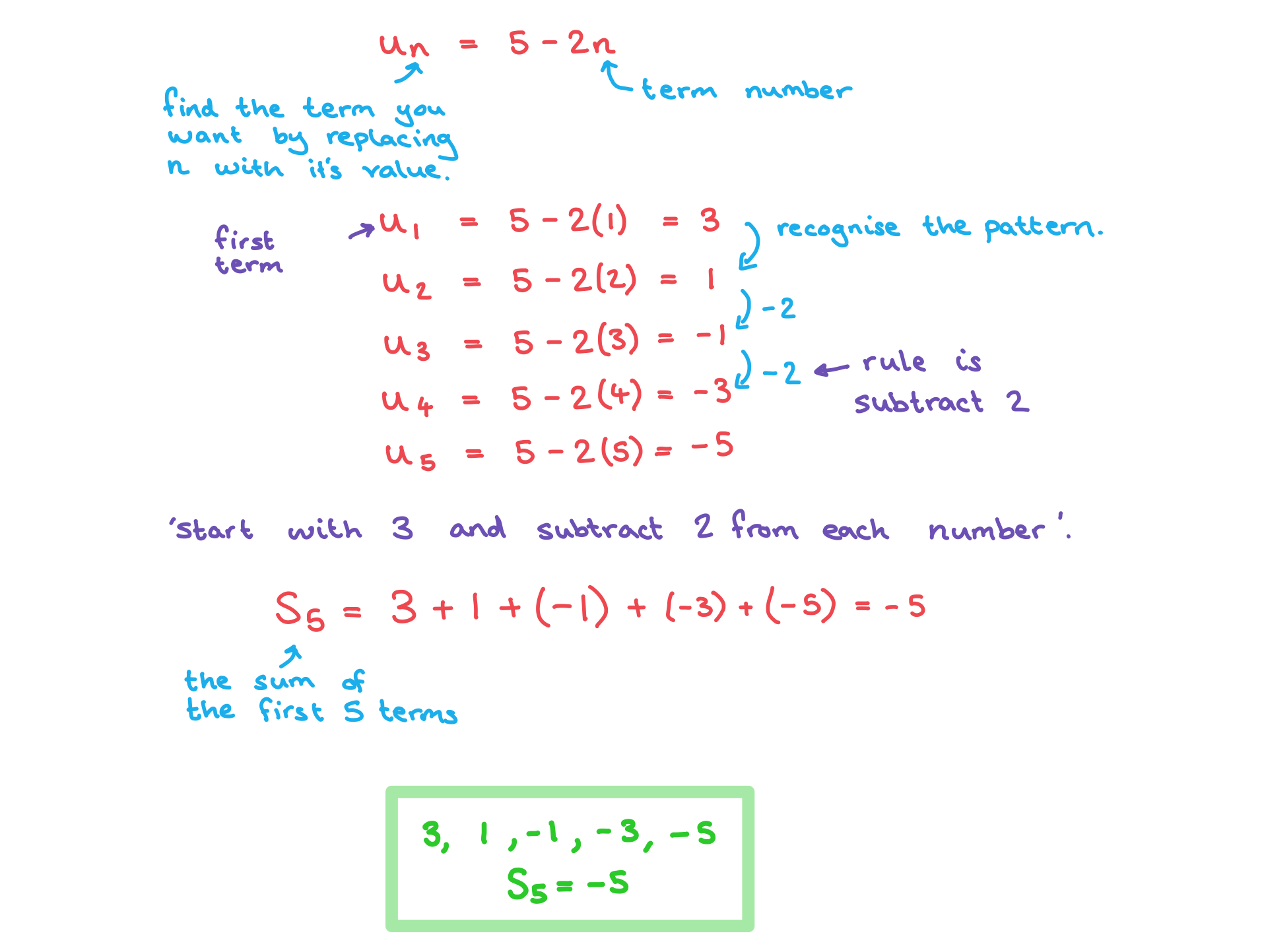
Worked Example
Determine the first five terms and the value of in the sequence given by
.

Unlock more, it's free!
Did this page help you?